Gauss’s Law

Gauss’s Law is a cornerstone of electromagnetism that relates the electric flux through a closed surface to the charge enclosed within it. In the context of AP Physics, understanding Gauss’s Law is crucial for analyzing electric fields in and around conductors, as well as in devices like capacitors. This law simplifies the calculation of electric fields in symmetrical charge distributions, making it a valuable tool for solving complex problems in electric circuits and ensuring a strong performance on the exam.

Learning Objectives

By studying Gauss’s Law in electric circuits for the AP Physics exam, you should aim to understand how to apply Gauss’s Law to analyze electric fields in conductors and capacitors. Focus on grasping the relationship between electric flux and enclosed charge, particularly in symmetrical charge distributions. Learn to calculate electric fields in and around conductors and capacitors, and understand the implications of these fields in circuit design and analysis. Master these concepts to confidently solve related problems on the exam.

Gauss’s Law in Electric Circuits

Gauss’s Law is a fundamental principle in electromagnetism, relating the distribution of electric charge to the resulting electric field. Although it is often discussed in the context of electrostatics, Gauss’s Law is also relevant in understanding electric circuits, particularly when analyzing electric fields within conductors and the behavior of capacitors.

1. Gauss’s Law: A Quick Recap

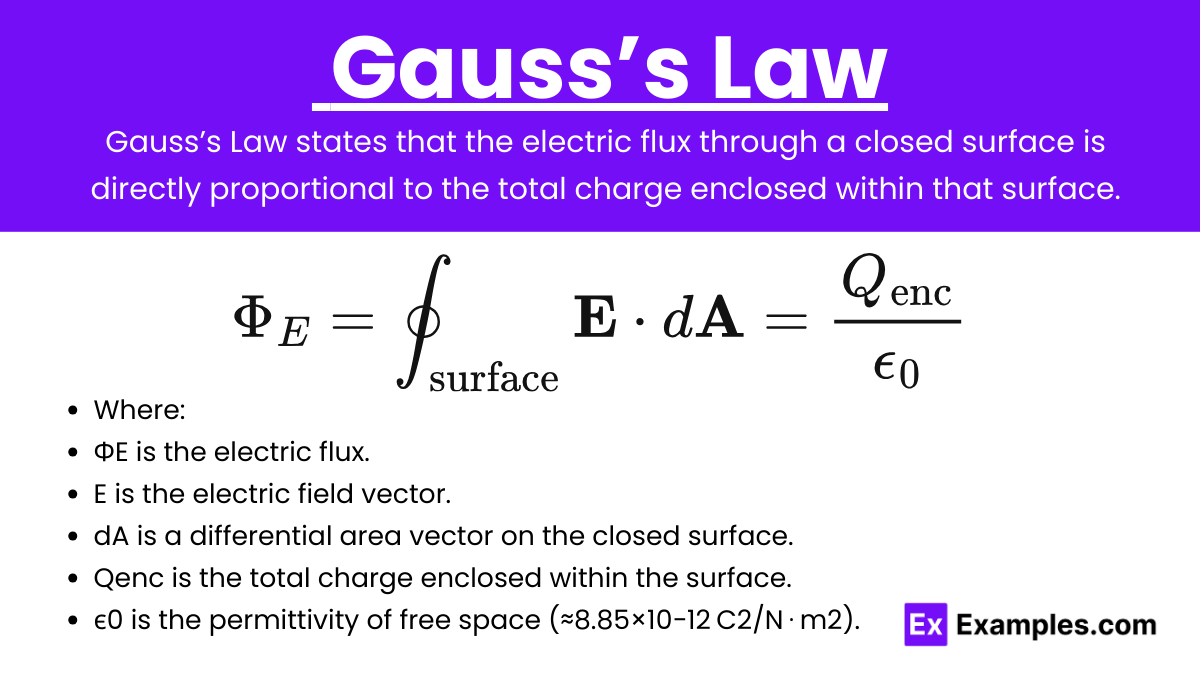
Gauss’s Law states that the electric flux through a closed surface (also known as a Gaussian surface) is directly proportional to the total charge enclosed within that surface. Mathematically, it is expressed as:

Where:

• Φ_E​ is the electric flux.
• E is the electric field vector.
• dA is a differential area vector on the closed surface.
• Q_enc is the total charge enclosed within the surface.
• ϵ₀​ is the permittivity of free space (≈8.85×10−¹² C²/N⋅m²).

2. Gauss’s Law and Conductors

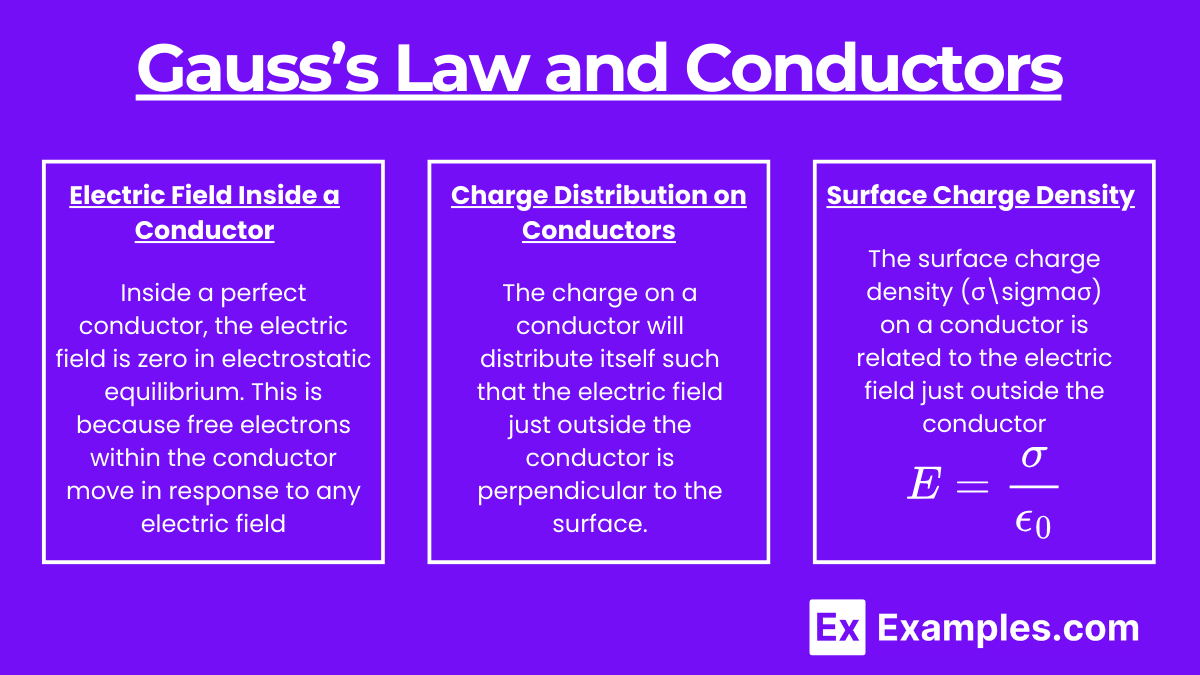
In electric circuits, conductors play a crucial role. Gauss’s Law helps us understand the distribution of electric charge in conductors:

• Electric Field Inside a Conductor: Inside a perfect conductor, the electric field is zero in electrostatic equilibrium. This is because free electrons within the conductor move in response to any electric field, redistributing themselves until the internal electric field cancels out. Thus, any net charge on a conductor resides on its surface.
• Charge Distribution on Conductors: The charge on a conductor will distribute itself such that the electric field just outside the conductor is perpendicular to the surface. Using a Gaussian surface just inside the conductor confirms that the electric field inside is zero, meaning any enclosed charge is zero.
• Surface Charge Density: The surface charge density (σ\sigmaσ) on a conductor is related to the electric field just outside the conductor by:

This relation is derived using Gauss’s Law and is particularly useful when analyzing capacitors.

3. Gauss’s Law in Capacitors

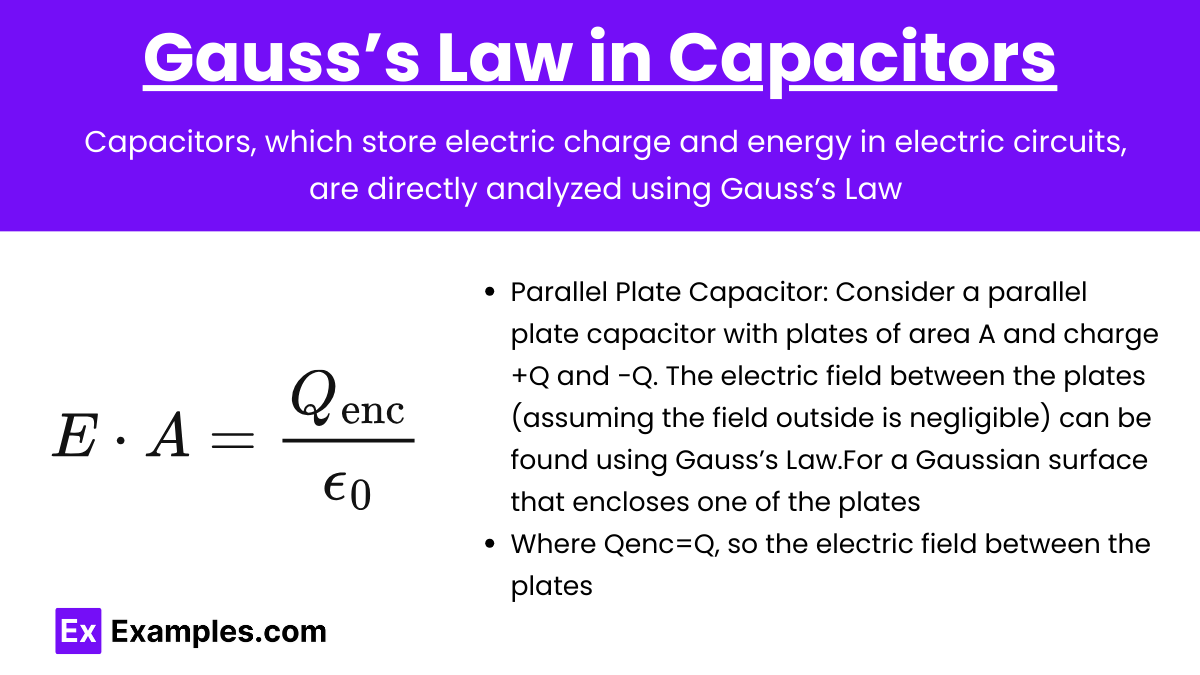
Capacitors, which store electric charge and energy in electric circuits, are directly analyzed using Gauss’s Law:

• Parallel Plate Capacitor: Consider a parallel plate capacitor with plates of area A and charge +Q and −Q. The electric field between the plates (assuming the field outside is negligible) can be found using Gauss’s Law.For a Gaussian surface that encloses one of the plates:

Where Q_enc=Q, so the electric field between the plates is:

• Capacitance: The capacitance C of the parallel plate capacitor is given by:

Where ddd is the separation between the plates.

4. Application of Gauss’s Law in Circuit Analysis

Gauss’s Law is particularly useful in analyzing circuits with symmetric charge distributions or when determining the electric field in and around conductors:

1. Electric Field Calculation in Symmetric Charge Distributions: Gauss’s Law simplifies the calculation of electric fields in circuits with spherical, cylindrical, or planar symmetry, aiding in the design and analysis of components like spherical conductors and coaxial cables.
2. Behavior of Conductors in Circuits: Helps in understanding how charges distribute themselves on conductors in a circuit, ensuring that the electric field inside a conductor is zero in electrostatic equilibrium.
3. Capacitor Analysis: Used to determine the electric field between the plates of a capacitor, which is crucial for calculating capacitance and understanding energy storage in circuits.
4. Electric Field in Shielding: Gauss’s Law is applied to analyze how conductive materials shield sensitive components in circuits by redistributing charges to cancel external electric fields.
5. Determining Surface Charge Density: Aids in calculating the surface charge density on conductors and how this relates to the electric field just outside the surface, important for understanding electrostatic potentials in circuits.
6. Designing Safe Circuits: Helps in assessing the strength and distribution of electric fields around high-voltage components, ensuring that circuit designs are safe and within operational limits.

Examples

Here are five examples of Gauss’s Law applied in electric circuits:

Example 1: Electric Field Due to a Charged Conductor

Consider a long, straight, uniformly charged conductor. By applying Gauss’s Law, we can determine the electric field at a point near the conductor. A cylindrical Gaussian surface is chosen, where the conductor is at the axis. Gauss’s Law relates the electric flux through the surface to the charge enclosed by the surface. This allows us to derive the electric field, which is crucial in analyzing the potential difference and behavior of charges in nearby circuits.

Example 2: Capacitor with a Dielectric

In a capacitor with a dielectric material between its plates, Gauss’s Law is used to calculate the electric field inside the dielectric. The Gaussian surface is chosen to enclose one of the capacitor’s plates. By applying Gauss’s Law, we can find the relationship between the charge on the plates, the dielectric constant, and the resulting electric field. This is essential for understanding how capacitors store energy in electric circuits.

Example 3: Electric Field Inside a Hollow Conductor

In circuits involving hollow conductors, Gauss’s Law is used to show that the electric field inside the conductor is zero. A Gaussian surface inside the hollow conductor reveals that no charge is enclosed within the surface, leading to zero electric flux. This principle is important for shielding sensitive components in electric circuits from external electric fields, ensuring stable operation.

Example 4: Electric Field Near a Charged Plate

Consider an infinite charged plate in a circuit, which can be idealized as a source of a uniform electric field. Using Gauss’s Law, we can determine that the electric field near the plate is constant and directed perpendicular to the surface. This example is useful in analyzing the behavior of parallel-plate capacitors, where the uniform electric field between the plates is a key factor in their functioning.

Example 5: Charge Distribution on Conductors in a Circuit

Gauss’s Law helps determine the distribution of charge on conductors within a circuit. For example, in a spherical conductor connected to a circuit, the charge resides on the outer surface, and the electric field inside is zero. By choosing a Gaussian surface inside the conductor, Gauss’s Law shows that no net charge is enclosed, confirming that the electric field is zero inside the conductor. This concept is crucial in understanding how charges behave in complex circuits, such as those involving coaxial cables.

Multiple Choice Questions

Question 1

What is Gauss’s Law?

A) The total electric flux through a closed surface is equal to the charge enclosed divided by the permittivity of free space.
B) The total electric flux through a closed surface is proportional to the square of the charge enclosed.
C) The electric field inside a conductor is always zero.
D) The electric flux through an open surface is proportional to the charge on the surface.

Correct Answer: A) The total electric flux through a closed surface is equal to the charge enclosed divided by the permittivity of free space.

Explanation: Gauss’s Law states that the electric flux through any closed surface (also called a Gaussian surface) is proportional to the total charge enclosed within that surface. Mathematically, this is expressed as:

where ΦE​ is the electric flux, Q_enc is the charge enclosed by the surface, and ϵ₀ is the permittivity of free space. This law is a fundamental principle in electromagnetism and is essential for calculating electric fields in various situations.

Question 2:

Which of the following is NOT a valid application of Gauss’s Law?

A) Calculating the electric field of a point charge.
B) Calculating the electric field inside a uniformly charged spherical shell.
C) Calculating the electric field due to an infinite line charge.
D) Calculating the magnetic field due to a current-carrying wire.

Correct Answer: D) Calculating the magnetic field due to a current-carrying wire.

Explanation: Gauss’s Law applies specifically to electric fields and charges. Option D refers to the magnetic field generated by a current-carrying wire, which is not related to Gauss’s Law but rather to Ampère’s Law. The other options are valid applications of Gauss’s Law: Option A deals with the field of a point charge, Option B with the field inside a spherical shell (which is zero due to symmetry), and Option C with the field from an infinite line charge.

Question 3:

A spherical Gaussian surface encloses a charge of +4 μC. What happens to the electric flux through the surface if the radius of the sphere is doubled?

A) The flux doubles.
B) The flux remains the same.
C) The flux halves.
D) The flux increases by a factor of four.

Correct Answer: B) The flux remains the same.

Explanation: According to Gauss’s Law, the electric flux through a Gaussian surface depends only on the total charge enclosed by the surface, not the size or shape of the surface itself. Even if the radius of the spherical surface is doubled, the total enclosed charge remains +4 μC, so the flux remains constant. The flux is given by:

Since Q_enc​ does not change, neither does ΦE​.