The Biot-Savart Law and Ampère’s Law

Last Updated: September 23, 2024

Notes

The Biot-Savart Law and Ampère’s Law are fundamental concepts in electromagnetism, crucial for understanding how electric currents generate magnetic fields. In AP Physics, these laws help describe the behavior of magnetic fields around current-carrying conductors, enabling students to analyze and solve problems related to magnetic field distributions. While the Biot-Savart Law provides a detailed method for calculating the magnetic field from small current elements, Ampère’s Law simplifies the process in highly symmetric situations, making it indispensable for mastering electromagnetism.

Free AP Physics C: Electricity and Magnetism Practice Test

Learning Objectives

When studying The Biot-Savart Law and Ampère’s Law for the AP Physics exam, you should focus on understanding how each law describes the generation of magnetic fields by electric currents. Learn to apply the Biot-Savart Law for calculating the magnetic field due to small current elements and complex geometries. Master Ampère’s Law for finding magnetic fields in symmetric situations, such as around wires and inside solenoids. Practice solving problems involving both laws, and understand the conditions under which each law is most effective.

The Biot-Savart Law and Ampère’s Law are fundamental principles in electromagnetism, describing how magnetic fields are generated by electric currents. Understanding these laws is crucial for solving problems related to magnetic fields in AP Physics.

The Biot-Savart Law

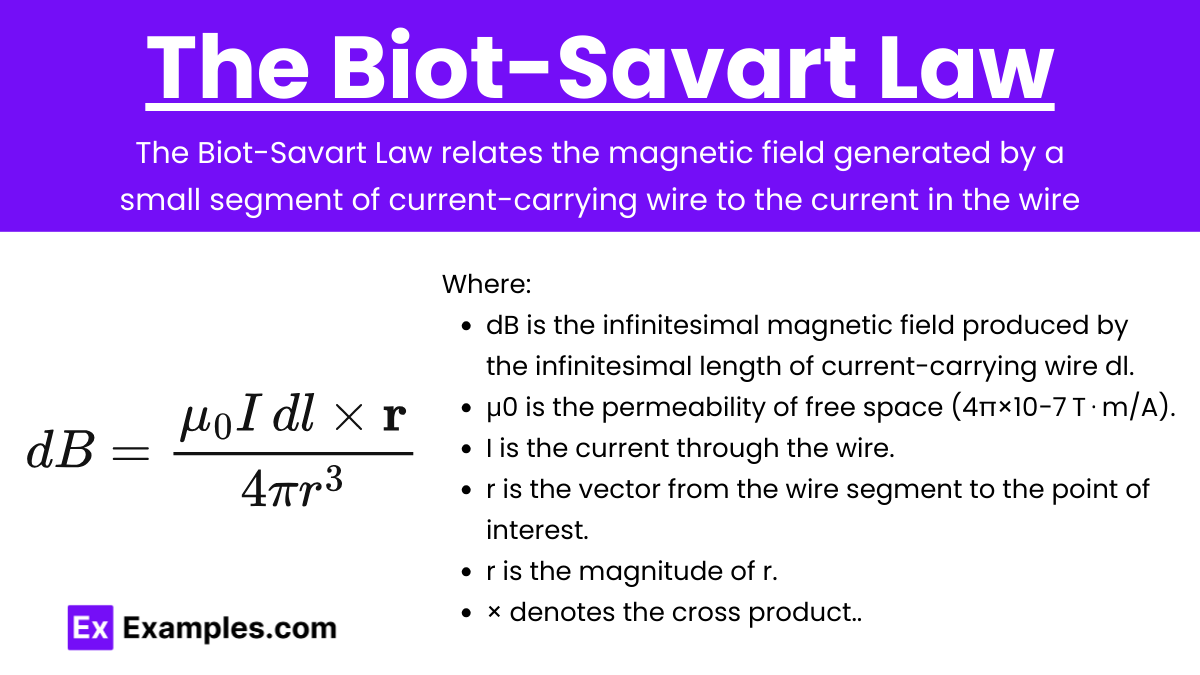
The Biot-Savart Law relates the magnetic field generated by a small segment of current-carrying wire to the current in the wire, the length of the segment, and the distance from the segment to the point where the magnetic field is being calculated.

Mathematical Expression

The Biot-Savart Law is given by:

$dB = \frac{\mu_{0} I \, dl \times \mathbf{r}}{4\pi r^{3}}$

Where:

dB is the infinitesimal magnetic field produced by the infinitesimal length of current-carrying wire dl.
μ₀is the permeability of free space (4π×10⁻⁷ T⋅m/A).
I is the current through the wire.
r is the vector from the wire segment to the point of interest.
r is the magnitude of r.
× denotes the cross product.

Key Points

The Biot-Savart Law is useful for calculating the magnetic field at a point due to a current element.
It is particularly helpful in scenarios involving complex current distributions, such as a loop of current or a segment of wire.
The direction of the magnetic field follows the right-hand rule: point your thumb in the direction of the current, and your fingers curl in the direction of the magnetic field.

Ampère’s Law

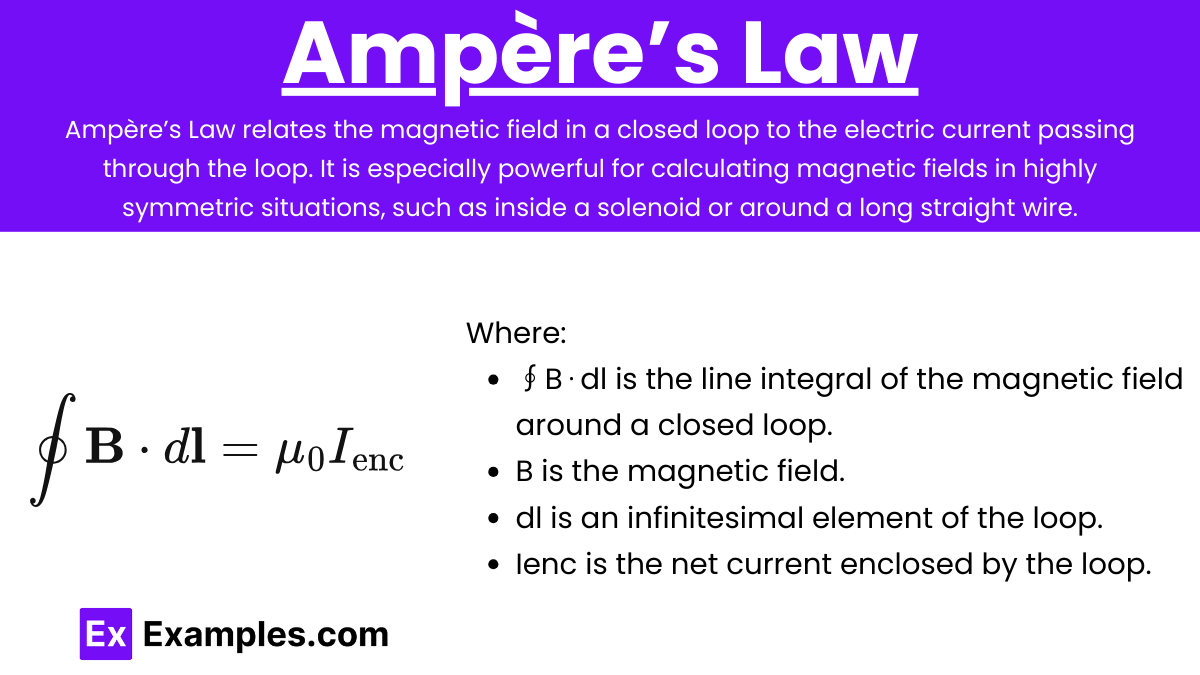
Ampère’s Law relates the magnetic field in a closed loop to the electric current passing through the loop. It is especially powerful for calculating magnetic fields in highly symmetric situations, such as inside a solenoid or around a long straight wire.

Mathematical Expression

Ampère’s Law is expressed as:

$\oint \mathbf{B} \cdot d\mathbf{l} = \mu_{0} I_{\text{enc}}$

Where:

∮B⋅dl is the line integral of the magnetic field around a closed loop.
B is the magnetic field.
dl is an infinitesimal element of the loop.
I_encis the net current enclosed by the loop.

Key Points

Ampère’s Law is particularly useful when the symmetry of the problem allows the magnetic field to be taken out of the integral.
It simplifies the calculation of magnetic fields in situations with high symmetry (e.g., infinite solenoids, toroids, straight wires).
The law applies to both steady and time-varying currents, but in AP Physics, we focus on steady currents.

Comparing the Biot-Savart Law and Ampère’s Law

Aspect	Biot-Savart Law	Ampère’s Law
Application	Used for calculating magnetic fields from small current elements or more complex geometries.	Used for calculating magnetic fields in cases of high symmetry (e.g., around a wire, inside a solenoid).
Mathematical Approach	Involves an integral over current elements to find the magnetic field.	Involves a line integral of the magnetic field around a closed loop.
Symmetry Requirement	No specific symmetry is required; can be used for any current distribution.	Requires symmetry to simplify calculations and remove the magnetic field from the integral.
Typical Use Cases	Magnetic field around loops, arcs, and short wire segments.	Magnetic field of infinite wires, solenoids, and toroids.

Applications of The Biot-Savart Law and Ampère’s Law

Magnetic Field of a Circular Loop: Use the Biot-Savart Law to calculate the magnetic field at the center of a current-carrying circular loop. This application is essential in understanding magnetic dipoles and their role in electromagnetism.
Magnetic Field of a Finite Straight Wire: The Biot-Savart Law helps determine the magnetic field near finite current-carrying wires, crucial for analyzing magnetic effects in circuits with straight wire segments.
Magnetic Field Inside a Solenoid: Apply Ampère’s Law to find the uniform magnetic field inside a solenoid. This is vital for creating strong, controllable magnetic fields used in electromagnets and transformers.
Magnetic Field Around a Long Straight Wire: Ampère’s Law simplifies the calculation of the magnetic field around an infinitely long, straight wire, fundamental to understanding basic electromagnetic principles.
Magnetic Field Around Arcs and Complex Geometries: The Biot-Savart Law is used to calculate the magnetic fields generated by current-carrying arcs and complex geometries, important in designing electromagnetic devices like motors and inductors.

Examples

Example 1: Magnetic Field Due to a Current-Carrying Straight Wire

Consider a long, straight wire carrying a current I. The Biot-Savart Law can be used to calculate the magnetic field at a point P located at a perpendicular distance rrr from the wire. According to the Biot-Savart Law, the magnetic field B at point P due to a small segment of the wire dl is given by $dB = \frac{\mu_{0} I \, dl \times \mathbf{r}}{4\pi r^{3}}$ . By integrating this expression along the length of the wire, we can derive the familiar expression $B = \frac{\mu_{0} I}{2\pi r}$ for the magnetic field around a long, straight conductor.

Example 2: Magnetic Field Inside a Solenoid

A solenoid is a coil of wire, often wound into a helical shape, carrying a current I. To find the magnetic field inside an ideal solenoid, Ampère’s Law is used. Considering a rectangular Amperian loop that encloses some turns of the solenoid, Ampère’s Law states $\oint \mathbf{B} \cdot d\mathbf{l} = \mu_{0} I_{\text{enc}}$ . For an ideal, infinitely long solenoid, the magnetic field inside is uniform and given by B=μ₀nI, where nnn is the number of turns per unit length. This field is directed along the axis of the solenoid and is zero outside the solenoid.

Example 3: Magnetic Field of a Circular Loop of Current

For a circular loop of radius R carrying a current III, the Biot-Savart Law can be used to calculate the magnetic field at the center of the loop. The symmetry of the loop allows simplification of the Biot-Savart Law, and the resulting magnetic field at the center of the loop is $B = \frac{\mu_{0} I}{2R}$ . This example shows how the Biot-Savart Law is useful in cases where the current distribution has a simple geometry.

Example 4: Magnetic Field Around a Toroid

A toroid is a doughnut-shaped coil of wire with N turns, carrying a current I. Ampère’s Law is particularly useful in this case. By applying Ampère’s Law around a circular path within the toroid, we find that the magnetic field inside the toroid is given by $B = \frac{\mu_{0} NI}{2\pi r}$ , where r is the radial distance from the center of the toroid. Outside the toroid, the magnetic field is nearly zero due to the circular symmetry and the closed nature of the coil.

Example 5: Magnetic Field of a Current Loop at an Axial Point

Consider a current loop of radius R carrying a current I. The Biot-Savart Law can be used to calculate the magnetic field at a point along the axis of the loop, a distance x from its center. By integrating the contributions of each small segment of the loop, the magnetic field at the axial point can be expressed as $B = \frac{\mu_{0} IR^{2}}{2(R^{2} + x^{2})^{3/2}}$ . This example demonstrates how the Biot-Savart Law can be applied to more complex configurations to find the magnetic field at specific points in space.

Multiple Choice Questions

Question 1

Which of the following statements correctly describes the Biot-Savart Law?

a) It relates the magnetic field at a point to the electric field and the displacement current.
b) It provides the relationship between the current in a conductor and the magnetic field it produces at a specific point.
c) It is used to calculate the electric field due to a point charge.
d) It relates the change in electric flux to the induced electromotive force (emf).

Answer: b) It provides the relationship between the current in a conductor and the magnetic field it produces at a specific point.

Explanation: The Biot-Savart Law describes how the magnetic field B is generated by a steady current I in a conductor. Specifically, it gives the magnetic field contribution dB at a point due to a small segment of current-carrying wire. The law is expressed mathematically as:

$dB = \frac{\mu_{0} I \, dl \times \mathbf{r}}{4\pi r^{3}}$

where:

μ₀is the permeability of free space,
I is the current,
dl is the differential length vector of the current element,
r is the position vector from the current element to the point where the field is being calculated,
r is the magnitude of r.

Thus, option b is correct as it accurately describes the law.

Question 2

Which of the following is a correct application of Ampère’s Law?

a) Calculating the magnetic field around a long, straight current-carrying wire.
b) Determining the electric potential difference between two points.
c) Estimating the electric field due to a uniformly charged sphere.
d) Measuring the force between two parallel current-carrying wires.

Answer: a) Calculating the magnetic field around a long, straight current-carrying wire.

Explanation: Ampère’s Law states that the line integral of the magnetic field B around a closed loop is proportional to the total current I_enc passing through the loop. Mathematically, it is expressed as:

$\oint \mathbf{B} \cdot d\mathbf{l} = \mu_{0} I_{\text{enc}}$

This law is particularly useful for calculating the magnetic field in highly symmetric situations, such as around a long, straight current-carrying wire, where the symmetry simplifies the calculation.

In contrast, options b) and c) pertain to electric fields, not magnetic fields, and option d) involves forces between currents, which is related to the Biot-Savart Law and not directly to Ampère’s Law.

Question 3:

Which of the following conditions must be satisfied for Ampère’s Law to be applicable in its simplest form?

a) The electric field must be constant in time.
b) The system must have cylindrical or planar symmetry.
c) The displacement current must be significant.
d) The magnetic field must be uniform.

Answer: b) The system must have cylindrical or planar symmetry.

Explanation: Ampère’s Law in its integral form is most easily applied in situations with high symmetry, such as cylindrical symmetry (e.g., around a long straight wire) or planar symmetry (e.g., in a current sheet). These symmetries allow the magnetic field B to be constant in magnitude along the path of integration, simplifying the evaluation of the integral.

Option a) refers to the time-dependence of the electric field, which is irrelevant to the simplicity of applying Ampère’s Law. Option c) would complicate the use of Ampère’s Law because it would require including the displacement current term, as in Maxwell-Ampère’s Law. Option d) is incorrect