Conservation of Energy

Last Updated: September 23, 2024

Notes

The Conservation of Energy is a fundamental principle in physics that asserts energy in an isolated system remains constant over time. This concept is central to understanding various phenomena in AP Physics, where energy can change forms, such as from kinetic to potential, but the total energy remains unchanged. Whether analyzing mechanical systems, thermal processes, or electrical circuits, mastering the conservation of energy helps predict system behavior and solve complex problems, making it a crucial topic for the AP Physics exam.

Free AP Physics C: Mechanics Practice Test

Learning Objectives

By studying Conservation of Energy for the AP Physics exam, you should aim to understand the principle that energy in an isolated system remains constant, even as it transforms between different forms like kinetic and potential energy. You should be able to apply the conservation of mechanical energy to solve problems involving objects in motion, pendulums, and systems with conservative and non-conservative forces. Additionally, mastering the Work-Energy Theorem and recognizing energy dissipation due to friction or other forces is essential.

What is the Conservation of Energy?

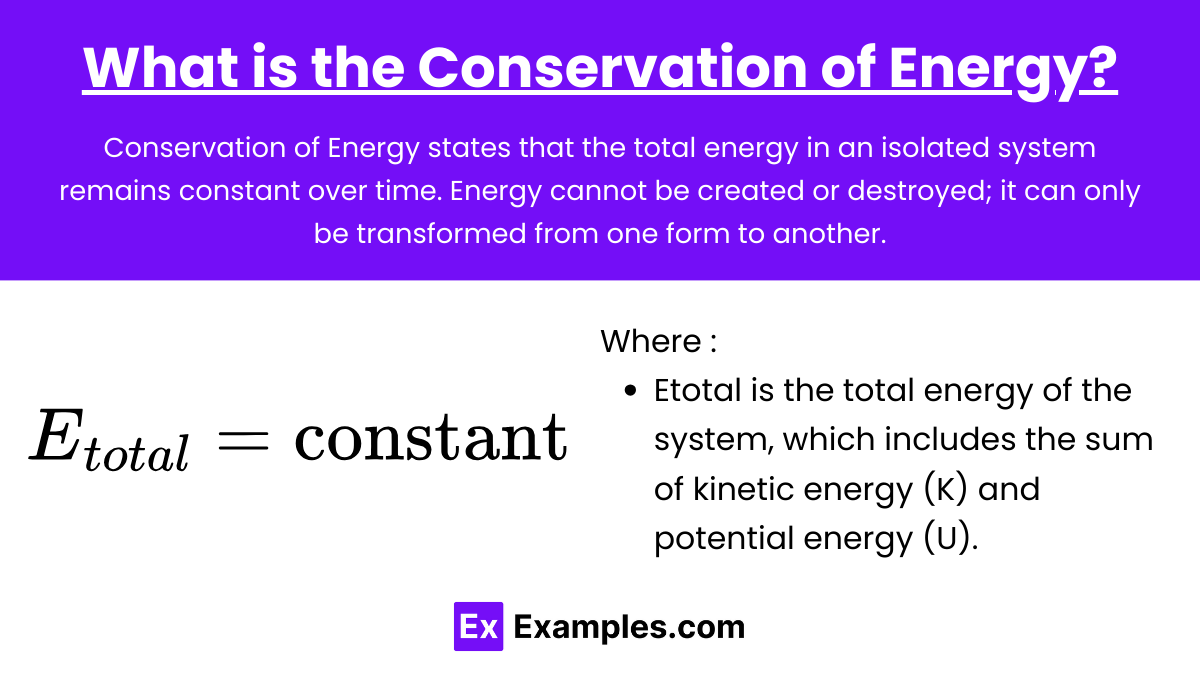
Conservation of Energy states that the total energy in an isolated system remains constant over time. Energy cannot be created or destroyed; it can only be transformed from one form to another. This principle applies to all types of energy, including kinetic, potential, thermal, chemical, and more.

Mathematical Expression

For an isolated system, the principle can be mathematically expressed as:

$E_{total} = \text{constant}$

Where:

Etotal is the total energy of the system, which includes the sum of kinetic energy (K) and potential energy (U).

In a more specific form, considering mechanical energy (which includes kinetic and potential energy):

$K_{i} + U_{i} = K_{f} + U_{f}$

Where:

K_iand U_iare the initial kinetic and potential energies, respectively.
K_fand U_f are the final kinetic and potential energies, respectively.

Key Concepts in Conservation of Energy

1. Mechanical Energy

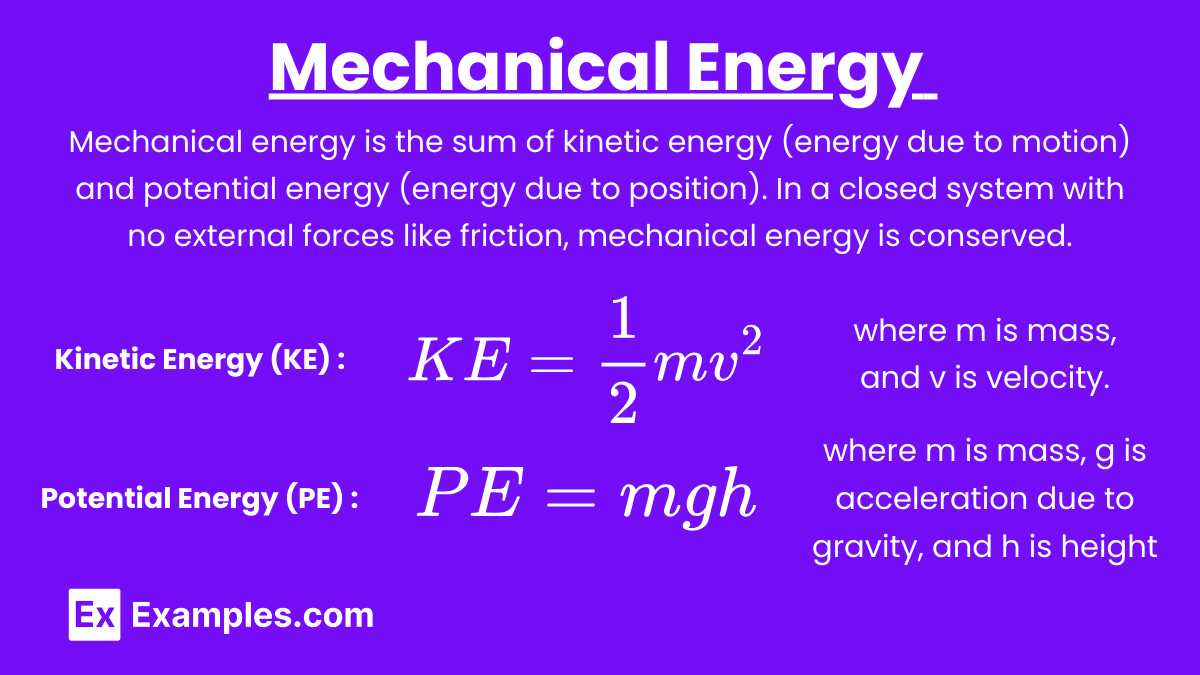
Mechanical energy is the sum of kinetic energy (energy due to motion) and potential energy (energy due to position). In a closed system with no external forces like friction, mechanical energy is conserved.

Kinetic Energy (KE): $KE = \frac{1}{2}mv^{2}$
where m is mass, and v is velocity.
Potential Energy (PE):
- Gravitational Potential Energy (GPE): $PE = mgh$
  where m is mass, g is acceleration due to gravity, and h is height above a reference point.
- Elastic Potential Energy (EPE) (for springs): $PE = \frac{1}{2}kx^{2}$
  where k is the spring constant, and x is the displacement from equilibrium.

2. Law of Conservation of Mechanical Energy

In the absence of non-conservative forces (like friction or air resistance), the total mechanical energy of a system remains constant:

$\text{Total Energy} = \text{Kinetic Energy} + \text{Potential Energy}$

3. Work-Energy Theorem

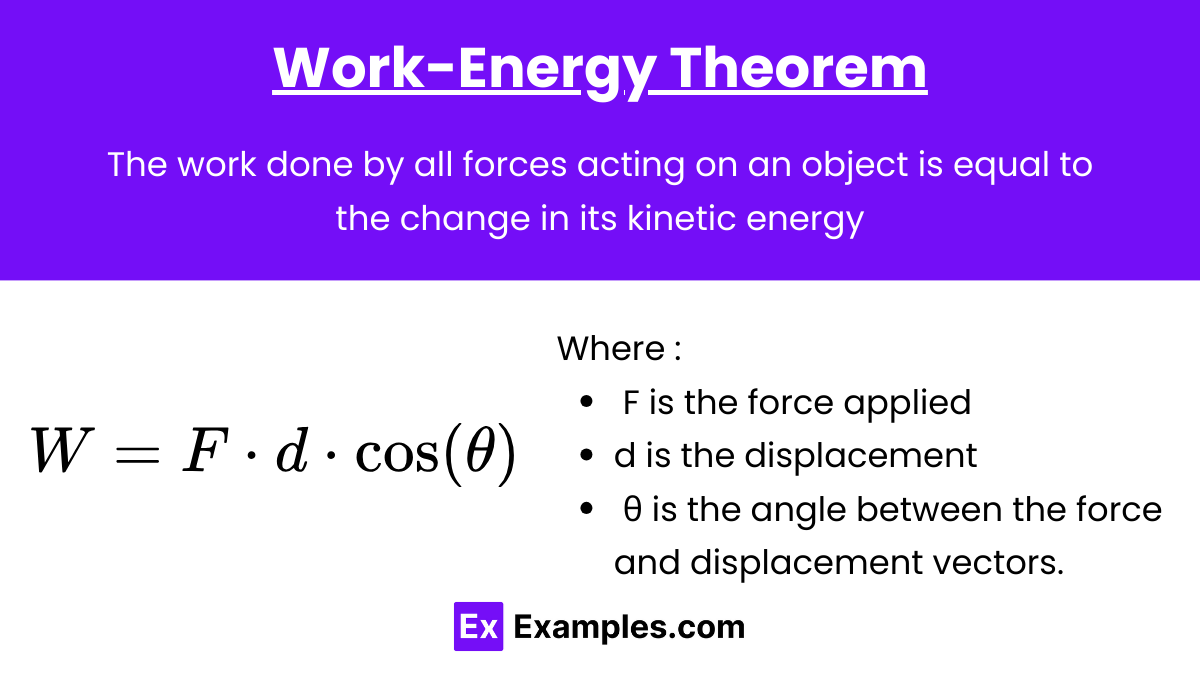
The work done by all forces acting on an object is equal to the change in its kinetic energy:

$W = \Delta KE = KE_{final} - KE_{initial}$

Work is calculated as:

$W = F \cdot d \cdot \cos(\theta)$

where F is the force applied, d is the displacement, and θ is the angle between the force and displacement vectors.

4. Non-Conservative Forces

Non-conservative forces, such as friction and air resistance, cause energy to be converted into other forms like heat, making mechanical energy non-conserved. The total energy, however, remains constant, taking into account all forms of energy.

Applications of Conservation of Energy

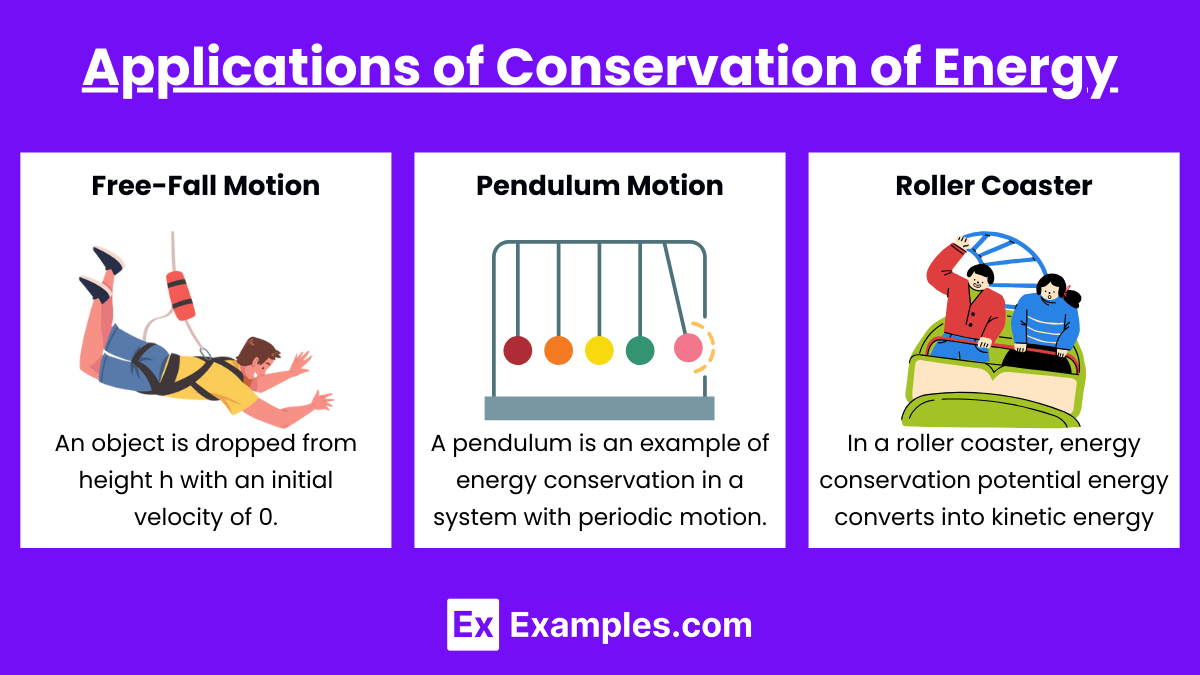
1. Free-Fall Motion : In free-fall, the only force acting on the object is gravity. The object’s potential energy decreases as its kinetic energy increases, but the total mechanical energy remains constant.

Example:

Initial State: An object is dropped from height h with an initial velocity of 0.
Final State: Just before hitting the ground, all potential energy is converted into kinetic energy.

$mgh = \frac{1}{2}mv^{2}$

2. Pendulum Motion : A pendulum is an example of energy conservation in a system with periodic motion. At the highest point, the energy is entirely potential, and at the lowest point, it’s entirely kinetic.

$mgh_{(max)} = \frac{1}{2}mv^{2}_{(max)}$

3. Roller Coaster : In a roller coaster, energy conservation explains how the potential energy at the top of a hill converts into kinetic energy as the coaster descends.

$mgh_{(top)} = \frac{1}{2}mv^{2}_{(bottom)}$

Problem-Solving Strategies

Identify the System: Determine the boundaries of the system and whether it is isolated or influenced by external forces.
Determine Energy Forms: Identify the different forms of energy involved (kinetic, potential, thermal, etc.).
Apply Conservation Laws: Use the law of conservation of energy to set up an equation where the total energy at the beginning equals the total energy at the end.
Include Non-Conservative Forces: If non-conservative forces like friction are present, account for energy loss or conversion to other forms.
Solve for Unknowns: Simplify the equation and solve for the unknown variable.

Examples

Example 1: Pendulum Motion

In a swinging pendulum, energy continuously transforms between kinetic and potential energy. At the highest point of the swing, the pendulum has maximum potential energy and minimum kinetic energy. As it swings down, potential energy converts into kinetic energy, reaching maximum kinetic energy at the lowest point. As it rises again, kinetic energy converts back to potential energy. Throughout this process, the total energy remains constant, illustrating the conservation of energy.

Example 2: Roller Coaster

A roller coaster at the top of a hill has maximum potential energy and minimal kinetic energy. As it descends, the potential energy is converted into kinetic energy, causing the coaster to accelerate. At the bottom of the hill, kinetic energy is at its peak, and potential energy is at its lowest. As the coaster ascends the next hill, kinetic energy converts back into potential energy. The total mechanical energy (potential + kinetic) remains constant throughout the ride, demonstrating energy conservation.

Example 3: Bouncing Ball

When a ball is dropped from a height, it has maximum potential energy and zero kinetic energy. As it falls, potential energy is converted into kinetic energy. When it hits the ground, some energy is temporarily stored as elastic potential energy, which then converts back into kinetic energy as the ball bounces back up. Although some energy is lost to heat and sound during the bounce, the principle of energy conservation still applies within the system.

Example 4: Hydroelectric Power Plant

Water stored in a dam has gravitational potential energy. When the water is released, this potential energy is converted into kinetic energy as the water flows down. The moving water then spins turbines, converting kinetic energy into mechanical energy, which is then transformed into electrical energy by generators. Throughout the process, the total energy is conserved, although it changes form.

Example 5: Photosynthesis

In plants, sunlight (radiant energy) is absorbed by chlorophyll and used to convert carbon dioxide and water into glucose and oxygen through photosynthesis. The energy from sunlight is stored in the chemical bonds of glucose, showing the transformation of radiant energy into chemical energy. While the form of energy changes, the total energy involved in the process remains conserved.

Multiple Choice Questions

Question 1

A roller coaster car is at the top of a hill with a potential energy of 5000 J. As it descends, its potential energy decreases to 2000 J. What is the kinetic energy of the car at this point?

A) 1000 J
B) 2000 J
C) 3000 J
D) 5000 J

Answer: C) 3000 J

Explanation:
The law of conservation of energy states that the total energy of an isolated system remains constant. In this case, the initial total energy of the roller coaster car is the sum of its potential energy (5000 J) and its kinetic energy (which is 0 J at the top of the hill). As the car descends, potential energy is converted into kinetic energy. The decrease in potential energy (from 5000 J to 2000 J) is equal to the increase in kinetic energy. Therefore, the kinetic energy at this point is 5000 J – 2000 J = 3000 J.

Question 2

A pendulum is released from a height where it has a gravitational potential energy of 10 J. Assuming no air resistance, what will be the kinetic energy of the pendulum at the lowest point of its swing?

A) 0 J
B) 5 J
C) 10 J
D) 20 J

Answer: C) 10 J

Explanation:
According to the conservation of energy principle, the total mechanical energy (sum of potential and kinetic energy) of the pendulum remains constant if there is no energy loss. At the highest point, the pendulum’s energy is all potential energy (10 J). As it swings down to the lowest point, this potential energy is completely converted into kinetic energy. Therefore, the kinetic energy at the lowest point is 10 J.

Question 3

A ball is thrown straight up with an initial kinetic energy of 15 J. At the peak of its motion, what is its kinetic energy and potential energy?

A) 15 J kinetic energy, 0 J potential energy
B) 0 J kinetic energy, 15 J potential energy
C) 7.5 J kinetic energy, 7.5 J potential energy
D) 15 J kinetic energy, 15 J potential energy

Answer: B) 0 J kinetic energy, 15 J potential energy

Explanation:
When the ball is thrown upwards, its kinetic energy is gradually converted into potential energy as it rises. At the peak of its motion, the ball momentarily stops, meaning its velocity is zero. Since kinetic energy depends on velocity (KE = 0.5 * mv²), the kinetic energy at this point is 0 J. However, the energy is conserved, so the initial kinetic energy (15 J) has been fully converted into potential energy, making the potential energy at the peak 15 J.