Conservation of Linear Momentum, Collisions

The Conservation of Linear Momentum is a fundamental concept in physics that plays a crucial role in understanding how objects interact in isolated systems. This principle states that the total momentum of a system remains constant if no external forces are acting on it. In the context of AP Physics, mastering the conservation of momentum is essential, especially when analyzing different types of collisions. Collisions, whether elastic or inelastic, illustrate how momentum is transferred between objects, and how energy can be conserved or transformed. A deep understanding of these concepts not only helps in solving complex problems but also provides insight into the behavior of objects in everyday situations, from car crashes to billiard games.

Free AP Physics C: Mechanics Practice Test

Learning Objectives

When studying Conservation of Linear Momentum and Collisions for the AP Physics exam, you should aim to understand how to apply the law of conservation of momentum to isolated systems, differentiate between elastic and inelastic collisions, and solve problems involving collisions in both one and two dimensions. You should be able to calculate final velocities after collisions, recognize when kinetic energy is conserved, and analyze real-world scenarios using momentum principles. Mastery of these concepts is essential for solving complex physics problems and achieving high scores.

Conservation of Linear Momentum

Conservation of Linear Momentum states that the total linear momentum of a closed system remains constant if no external forces act on it. Linear momentum is a vector quantity, defined as the product of an object’s mass and its velocity. Mathematically, it is expressed as: p = m v

Principle of Conservation of Linear Momentum

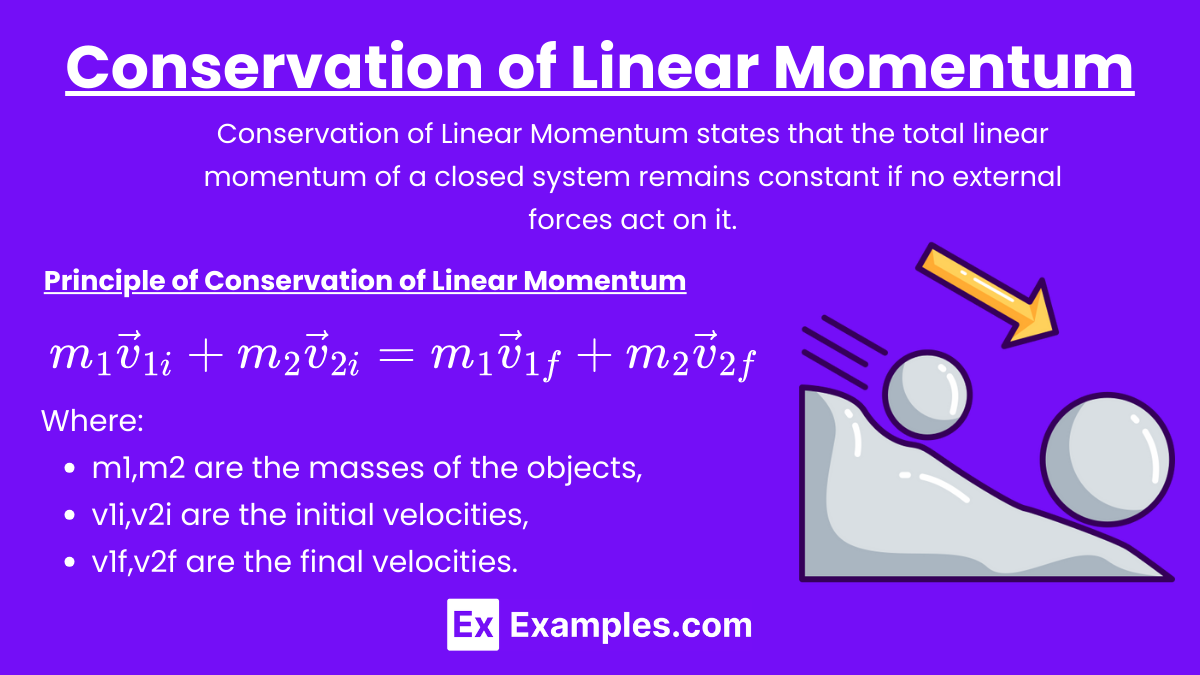
The principle of conservation of linear momentum can be understood through Newton’s Third Law of Motion, which states that every action has an equal and opposite reaction. When two objects interact, they exert equal and opposite forces on each other. This interaction causes a change in the momentum of both objects, but the total momentum of the system remains unchanged.

Mathematically, for two colliding objects in an isolated system:

p_initial = p_final

This can be expanded as:

\( m_1 \vec{v}_{1i} + m_2 \vec{v}_{2i} = m_1 \vec{v}_{1f} + m_2 \vec{v}_{2f} \)

Where:

m1,m2 are the masses of the objects,
v_1i,v_2i are the initial velocities,
v_1f,v_2f are the final velocities.

Types of Collisions

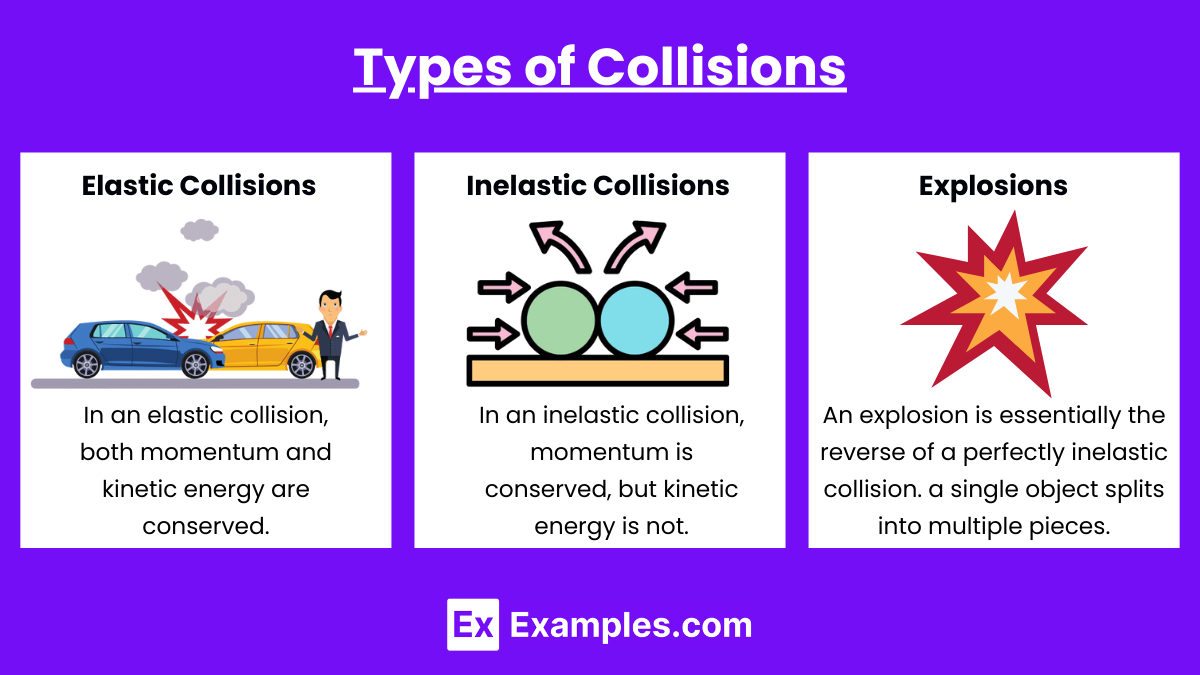
1. Elastic Collisions

In an elastic collision, both momentum and kinetic energy are conserved.

Kinetic energy: The total kinetic energy of the system before and after the collision is the same.
Example: Two identical billiard balls colliding head-on and rebounding without any loss of speed.

Key Equations:

Conservation of momentum :

\( m_1 \vec{V}_{\text{initial}} + m_2 \vec{V}_{2\text{initial}} = m_1 \vec{V}_{\text{final}} + m_2 \vec{V}_{2\text{final}} \)

Conservation of kinetic energy:

\( \frac{1}{2} m_1 v_{1\text{initial}}^2 + \frac{1}{2} m_2 v_{2\text{initial}}^2 = \frac{1}{2} m_1 v_{1\text{final}}^2 + \frac{1}{2} m_2 v_{2\text{final}}^2 \)

2. Inelastic Collisions

In an inelastic collision, momentum is conserved, but kinetic energy is not.

Some kinetic energy is transformed into other forms of energy, such as heat or sound.
In a perfectly inelastic collision, the colliding objects stick together after the collision.

Key Equations:

Conservation of momentum:

\( m_1 \vec{V}_{\text{initial}} + m_2 \vec{V}_{2\text{initial}} = (m_1 + m_2) \vec{V}_{\text{final}} \)

Kinetic energy is not conserved, but you can calculate the loss of kinetic energy as:

\( \text{Kinetic Energy Loss} = \text{Initial Kinetic Energy} – \text{Final Kinetic Energy} \)

3. Explosions

An explosion is essentially the reverse of a perfectly inelastic collision. In an explosion, a single object splits into multiple pieces.

The total momentum before and after the explosion remains zero if the object was initially at rest.
Example: A firework exploding in the air. The sum of the momenta of all fragments equals the momentum of the firework before the explosion.

Applications of Conservation of Linear Momentum in Collisions

1. Car Accidents and Safety Design : In car accidents, the principle of conservation of linear momentum helps in analyzing the impact forces and the resulting motion of vehicles. Understanding momentum conservation allows engineers to design safety features like crumple zones and airbags that minimize the force experienced by passengers by extending the collision time, thereby reducing the risk of injury.

2. Billiard Games: In billiard games, collisions between the balls are typically elastic, meaning that both momentum and kinetic energy are conserved. Players use the principles of momentum to control the direction and speed of the balls. By predicting how the balls will move after a collision, players can plan their shots to sink balls into pockets or position the cue ball strategically.

3. Particle Physics : In particle accelerators, such as the Large Hadron Collider, particles are collided at high speeds to study their fundamental properties. The conservation of momentum is crucial in analyzing the outcomes of these collisions, helping physicists identify new particles and understand the forces at work on a subatomic level.

4. Sports : In sports like football or hockey, collisions between players or between a player and a ball/puck involve the conservation of momentum. Coaches and players use this principle to improve performance by predicting the motion of the ball or puck after impact, optimizing passes, shots, and tackles.

5. Astrophysics and Celestial Collisions : The conservation of momentum is used to study collisions between celestial bodies, such as asteroids, comets, and planets. When two celestial objects collide, their combined momentum before and after the impact remains constant. This principle helps astronomers predict the outcomes of such events, including the formation of new celestial bodies or the alteration of orbital paths.

Examples

Example 1: Head-on Collision Between Two Cars

Imagine two cars, Car A and Car B, moving towards each other on a straight road. Car A has a mass of 1,200 kg and is moving at 15 m/s, while Car B has a mass of 1,500 kg and is moving at 10 m/s in the opposite direction. When they collide, if the collision is perfectly elastic, the total momentum before and after the collision will remain the same. The velocities of the cars will adjust such that the total momentum of the system is conserved, with each car rebounding in opposite directions.

Example 2: Recoil of a Gun

When a bullet is fired from a gun, the bullet moves forward while the gun recoils backward. Before the trigger is pulled, the total momentum of the gun and bullet system is zero because both are at rest. After the bullet is fired, the momentum of the bullet moving forward is equal in magnitude and opposite in direction to the momentum of the gun’s recoil. This example demonstrates the conservation of momentum in an inelastic collision, where the system’s total momentum remains zero.

Example 3: Billiard Balls Colliding on a Pool Table

Consider a moving billiard ball striking a stationary ball on a pool table. If the collision is perfectly elastic, the moving ball transfers some of its momentum to the stationary ball. The total momentum of both balls before and after the collision will be conserved. This example is often used to demonstrate how momentum is distributed between two objects in a collision.

Example 4: Explosion of a Firecracker in Space

Suppose a firecracker explodes in the vacuum of space, breaking into several pieces. Initially, the firecracker is stationary, so its total momentum is zero. After the explosion, the fragments fly off in different directions. The momentum of each fragment is such that when all are added together, the total momentum still equals zero. This is an example of the conservation of momentum in an explosive event where the internal forces cause the object to break apart.

Example 5: Elastic Collision Between Two Ice Skaters

Imagine two ice skaters gliding towards each other on a frictionless ice rink. One skater has a mass of 70 kg and is moving at 5 m/s, while the other skater has a mass of 60 kg and is moving at 4 m/s in the opposite direction. When they collide and push off each other, their total momentum before and after the collision is conserved. The skaters will move away from each other at velocities determined by their respective masses and the conservation of momentum principle.

Multiple Choice Questions

Question 1

When two objects collide in an isolated system, which of the following statements best describes the principle of conservation of linear momentum?

A) The total momentum of the system increases after the collision.
B) The total momentum of the system decreases after the collision.
C) The total momentum of the system remains constant before and after the collision.
D) The total momentum of each object remains the same before and after the collision.

Answer: C) The total momentum of the system remains constant before and after the collision.

Explanation: The principle of conservation of linear momentum states that in an isolated system (i.e., no external forces acting on it), the total linear momentum of the system remains constant during a collision. This means that the total momentum before the collision is equal to the total momentum after the collision, regardless of the types of collisions (elastic or inelastic). Momentum is conserved for the entire system, not necessarily for each individual object.

Question 2

Two carts with masses m1=2 kg and m2=3 are moving towards each other on a frictionless track. Cart 1 has a velocity of 4 m/s to the right, and Cart 2 has a velocity of 2 m/s to the left. After they collide and stick together, what is their combined velocity?

A) 0.4m/s to the right
B) 0.4m/s to the left
C) 2.8m/s to the right
D) 2.8m/s to the left

Answer: A) 0.4 m/s to the right

Explanation: This is a perfectly inelastic collision where the two objects stick together after colliding. According to the conservation of linear momentum:

Total initial momentum = Total final momentum

\( (m_1 \times v_1) + (m_2 \times v_2) = (m_1 + m_2) \times v_f \)

Substituting the given values:

(2×4)+(3×−2)=(2+3)×vf

8−6=5×vf

2=5×vf

vf=2/5=0.4 m/s to the right

Since the result is positive, the final velocity is to the right.

Question 3

In an elastic collision between two objects, which of the following quantities is conserved?

A) Only kinetic energy is conserved.
B) Only linear momentum is conserved.
C) Both kinetic energy and linear momentum are conserved.
D) Neither kinetic energy nor linear momentum is conserved.

Answer: C) Both kinetic energy and linear momentum are conserved.

Explanation: In an elastic collision, both kinetic energy and linear momentum are conserved. This means that the total kinetic energy of the system before the collision is equal to the total kinetic energy after the collision, and similarly, the total linear momentum remains unchanged. This contrasts with inelastic collisions, where only momentum is conserved, and kinetic energy is not.