Gravitational Forces

Last Updated: September 23, 2024

Notes

Gravitational forces are one of the four fundamental forces of nature, responsible for the attraction between objects with mass. These forces govern the motion of planets, stars, and galaxies, making them essential for understanding the universe’s structure. In AP Physics, the study of gravitational forces includes Newton’s Law of Universal Gravitation, gravitational fields, and the behavior of objects in orbits. Mastery of these concepts is crucial for solving complex problems and excelling in the exam.

Free AP Physics C: Mechanics Practice Test

Learning Objectives

For the AP Physics exam, your learning objectives on Gravitational Forces should include understanding Newton’s Law of Universal Gravitation and its application to calculate gravitational forces between objects. You should grasp the concepts of gravitational field strength, gravitational potential energy, and how they relate to the motion of objects in different contexts, such as orbits and escape velocity. Additionally, mastering Kepler’s Laws of Planetary Motion and applying these principles to solve problems involving planetary and satellite motion are crucial for excelling in this topic.

Overview of Gravitational Forces

Gravitational force is a fundamental force of nature that acts between any two objects with mass. It is always attractive and acts along the line joining the centers of mass of the two objects. The concept of gravitational force is central to understanding a wide range of physical phenomena, from the motion of planets to the behavior of objects near the Earth’s surface.

Newton’s Law of Universal Gravitation

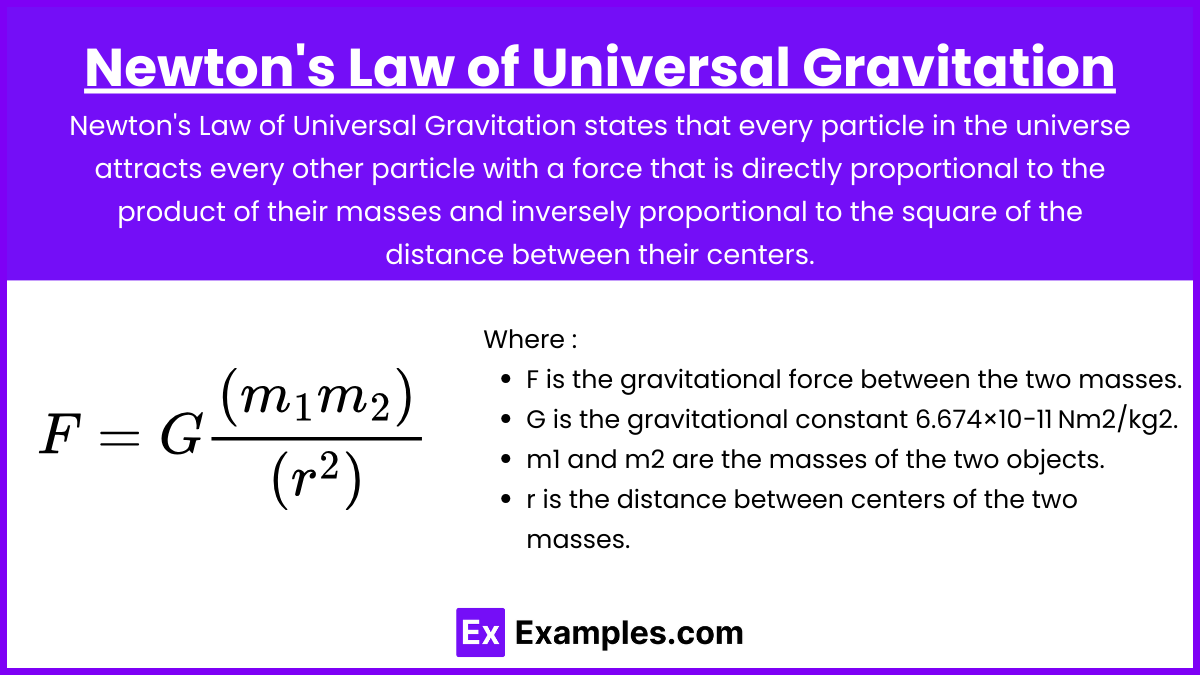
Newton’s Law of Universal Gravitation states that every particle in the universe attracts every other particle with a force that is directly proportional to the product of their masses and inversely proportional to the square of the distance between their centers. This can be mathematically expressed as:

$F = G \frac{(m_{1}m_{2})}{(r^{2})}$

Where:

F is the gravitational force between the two masses.
G is the gravitational constant 6.674×10−11 Nm²/kg².
m₁ and m₂ are the masses of the two objects.
r is the distance between the centers of the two masses.

Key Points:

Gravitational force is always attractive.
The magnitude of the force decreases with the square of the distance between the objects.
The gravitational force is central and acts along the line joining the two masses.

Gravitational Field

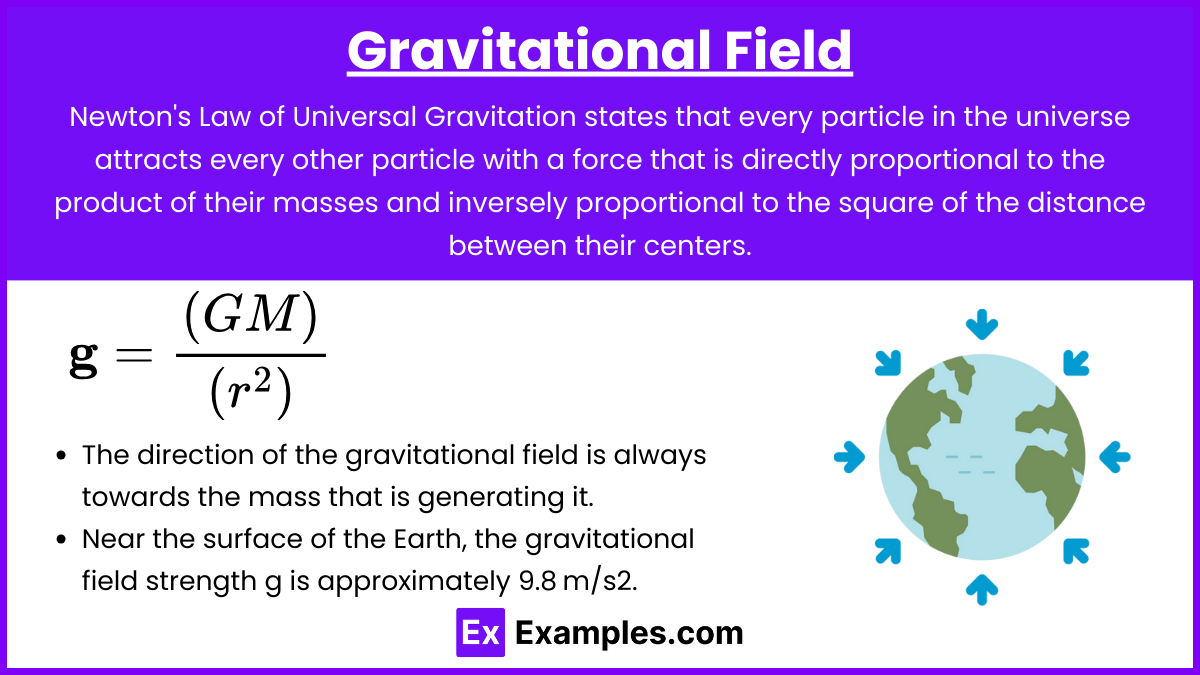
The gravitational field is a vector field that describes the gravitational force experienced by a unit mass at any point in space. The gravitational field strength g\mathbf{g}g at a distance rrr from a mass M is given by:

$\mathbf{g} = \frac{(GM)}{(r^{2})}$

The direction of the gravitational field is always towards the mass that is generating it.
Near the surface of the Earth, the gravitational field strength g is approximately 9.8 m/s².

Gravitational Field Due to Multiple Masses

When multiple masses are present, the total gravitational field at a point is the vector sum of the fields due to each mass. This is a direct application of the principle of superposition.

Gravitational Potential Energy

Gravitational potential energy U is the energy associated with the position of an object in a gravitational field. For two masses m₁and m₂ separated by a distance rrr, the gravitational potential energy is given by:

$U = -G \frac{(m_{1}m_{2})}{(r)}$

The negative sign indicates that the gravitational force is attractive.
As the distance r increases, the gravitational potential energy approaches zero from the negative side.

Gravitational Potential

The gravitational potential V at a distance r from a mass M is:

$V = -\frac{(GM)}{(r)}$

Gravitational potential is a scalar quantity.
It represents the work done per unit mass to bring an object from infinity to a point at distance r.

Circular Orbits and Kepler’s Laws

Objects in circular orbits around a planet or star are held in orbit by the gravitational force, which provides the necessary centripetal force. For an object of mass m in a circular orbit of radius r around a planet of mass M, the orbital speed v is given by:

$v = \sqrt{\frac{(GM)}{(r)}}$

Kepler’s Laws of Planetary Motion:

First Law (Law of Ellipses): Planets move in elliptical orbits with the Sun at one focus.
Second Law (Law of Equal Areas): A line segment joining a planet and the Sun sweeps out equal areas during equal intervals of time.
Third Law (Harmonic Law): The square of the orbital period T of a planet is proportional to the cube of the semi-major axis aaa of its orbit:

$T^{2} \propto a^{3}$

For circular orbits, this can be expressed as:

$T^{2} = \frac{(4\pi^{2}r^{3})}{(GM)}$

Gravitational Forces in Different Contexts

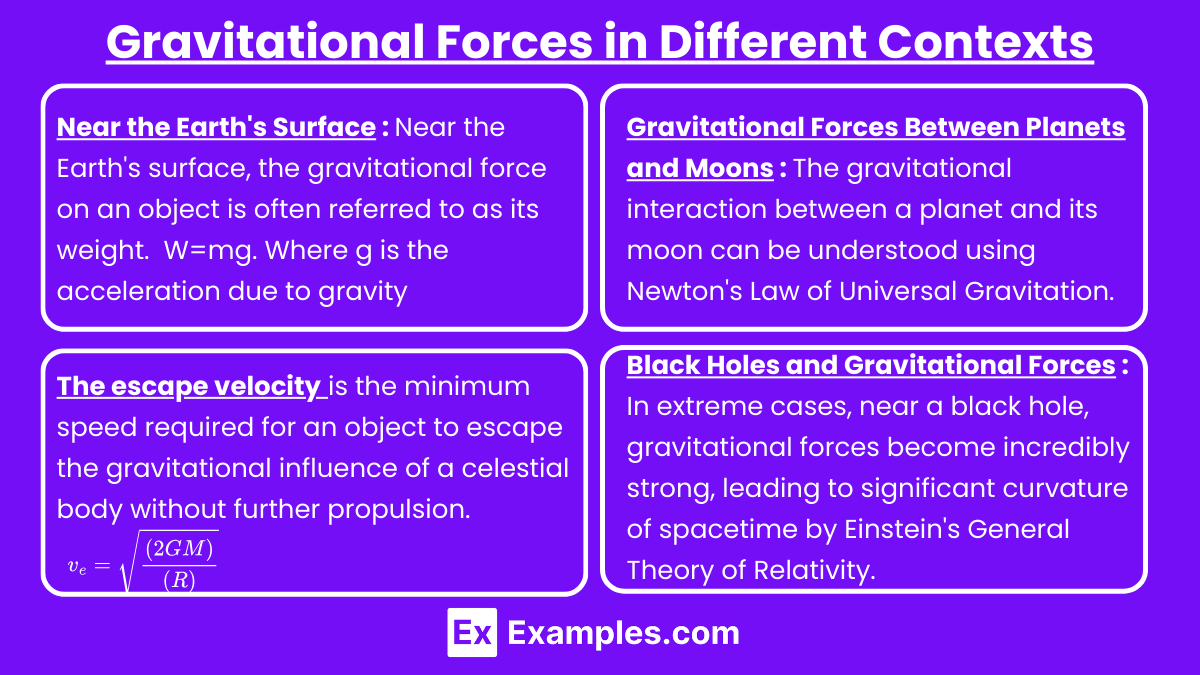
Near the Earth’s Surface

Near the Earth’s surface, the gravitational force on an object is often referred to as its weight. The weight W of an object with mass mmm is:

$W = mg$

Where g is the acceleration due to gravity.

Gravitational Forces Between Planets and Moons

The gravitational interaction between a planet and its moon can be understood using Newton’s Law of Universal Gravitation. For example, the gravitational force between the Earth and the Moon determines the tides on Earth.

Escape Velocity

The escape velocity is the minimum speed required for an object to escape the gravitational influence of a celestial body without further propulsion. For a planet of mass M and radius R, the escape velocity v_eis:

$v_{e} = \sqrt{\frac{(2GM)}{(R)}}$

Black Holes and Gravitational Forces

In extreme cases, such as near a black hole, gravitational forces become incredibly strong, leading to significant curvature of spacetime as described by Einstein’s General Theory of Relativity. The concept of an event horizon defines the boundary within which the escape velocity exceeds the speed of light.

Examples

Example 1: Earth’s Gravity on Objects

The most common example of gravitational force is the Earth pulling objects towards its center. When you drop a ball, it falls to the ground because Earth’s gravity pulls it downward. This force acts on everything near the Earth’s surface, keeping us grounded and causing objects to fall when released.

Example 2: Gravitational Pull of the Moon on Earth

The gravitational force between the Earth and the Moon causes ocean tides. The Moon’s gravity pulls on the Earth’s oceans, causing them to bulge out in the direction of the Moon. This pull creates the high and low tides experienced on Earth.

Example 3: Gravity Between the Sun and the Planets

The gravitational force from the Sun keeps the planets in our solar system in their orbits. The Sun’s gravity pulls the planets towards it, but because they are moving forward in their orbits, they do not fall into the Sun. Instead, they keep revolving around it in a balanced motion.

Example 4: Gravity on Astronauts in Space

Even in space, gravitational forces act on astronauts and spacecraft. While orbiting Earth, astronauts experience microgravity, meaning they feel weightless because they are in free fall towards Earth, but their forward motion keeps them in orbit.

Example 5: Gravitational Attraction Between Galaxies

Gravitational forces also act on a much larger scale between galaxies. The Milky Way and the Andromeda Galaxy, for example, are slowly being pulled towards each other due to their mutual gravitational attraction. Over billions of years, this force will cause the two galaxies to merge.

Multiple Choice Questions

Question 1

Which of the following factors affects the gravitational force between two objects?

A) The color of the objects
B) The distance between the objects
C) The speed of the objects
D) The temperature of the objects

Answer: B) The distance between the objects

Explanation: The gravitational force between two objects is directly related to their masses and inversely related to the square of the distance between them, as described by Newton’s Law of Universal Gravitation. The formula for gravitational force is:

$F = G \frac{(m_{1}m_{2})}{(r^{2})}$

Where:

F is the gravitational force,
G is the gravitational constant,
m₁ and m₂are the masses of the two objects, and
r is the distance between the centers of the two objects.

None of the other factors, such as the color, speed, or temperature of the objects, affect the gravitational force.

Question 2

If the mass of one object is doubled while the mass of the other object and the distance between them remain the same, how does the gravitational force between the two objects change?

A) It remains the same
B) It doubles
C) It halves
D) It quadruples

Answer: B) It doubles

Explanation: The gravitational force between two objects is directly proportional to the product of their masses. If the mass of one object is doubled, the force will also double, assuming the other mass and the distance remain unchanged. Mathematically:

$F \propto (m_{1} \times m_{2})$

If m₁ becomes 2m₁, then:

$F_{\text{new}} = \frac{G \cdot (2m_{1}) \cdot m_{2}}{(r^{2})} = 2 \times \frac{G \cdot m_{1} \cdot m_{2}}{(r^{2})} = 2F$

Thus, the gravitational force doubles.

Question 3

Which of the following best explains why astronauts in orbit around Earth experience “weightlessness”?

A) They are too far from Earth for gravity to act.
B) Earth’s gravity is balanced by the Sun’s gravity.
C) They are in free fall, accelerating towards Earth at the same rate as their spacecraft.
D) There is no gravity in space.

Answer: C) They are in free fall, accelerating towards Earth at the same rate as their spacecraft.

Explanation:
Astronauts in orbit experience “weightlessness” because they are in a continuous state of free fall towards Earth. However, because they are also moving forward at a high speed, they continuously fall around Earth rather than directly towards it. This creates the sensation of weightlessness, as both the astronauts and their spacecraft are accelerating towards Earth at the same rate. Gravity is still acting on them; it is not balanced or absent, but rather the forward motion keeps them in orbit, creating the sensation of floating.