Effortlessly convert between various mass units with the Examples.com Mass Converter. Input your measurements for quick and accurate conversions.
Mass Converters to Tonne (t)
| Kilogram to Tonne | Gram to Tonne | Milligram to Tonne |
| Microgram to Tonne | Imperial ton to Tonne | US ton to Tonne |
| Stone to Tonne | Pound to Tonne | Ounce to Tonne |
Mass Converters to Kilogram (kg)
| Tonne to Kilogram | Gram to Kilogram | Milligram to Kilogram |
| Microgram to Kilogram | Imperial ton to Kilogram | US ton to Kilogram |
| Stone to Kilogram | Pound to Kilogram | Ounce to Kilogram |
Mass Converters to Gram (g)
| Tonne to Gram | Kilogram to Gram | Milligram to Gram |
| Microgram to Gram | Imperial ton to Gram | US ton to Gram |
| Stone to Gram | Pound to Gram | Ounce to Gram |
Mass Converters to Milligram (mg)
| Tonne to Milligram | Kilogram to Milligram | Gram to Milligram |
| Microgram to Milligram | Imperial ton to Milligram | US ton to Milligram |
| Stone to Milligram | Pound to Milligram | Ounce to Milligram |
Mass Converters to Microgram (µg)
| Tonne to Microgram | Kilogram to Microgram | Gram to Microgram |
| Milligram to Microgram | Imperial ton to Microgram | US ton to Microgram |
| Stone to Microgram | Pound to Microgram | Ounce to Microgram |
Mass Converters to Imperial ton (It)
Mass Converters to US ton (tn)
| Tonne to US ton | Kilogram to US ton | Gram to US ton |
| Milligram to US ton | Microgram to US ton | Imperial ton to US ton |
| Stone to US ton | Pound to US ton | Ounce to US ton |
Mass Converters to Stone (s)
| Tonne to Stone | Kilogram to Stone | Gram to Stone |
| Milligram to Stone | Microgram to Stone | Imperial ton to Stone |
| US ton to Stone | Pound to Stone | Ounce to Stone |
Mass Converters to Pound (lb)
| Tonne to Pound | Kilogram to Pound | Gram to Pound |
| Milligram to Pound | Microgram to Pound | Imperial ton to Pound |
| US ton to Pound | Stone to Pound | Ounce to Pound |
Mass Converters to Ounce (oz)
| Tonne to Ounce | Kilogram to Ounce | Gram to Ounce |
| Milligram to Ounce | Microgram to Ounce | Imperial ton to Ounce |
| US ton to Ounce | Stone to Ounce | Pound to Ounce |
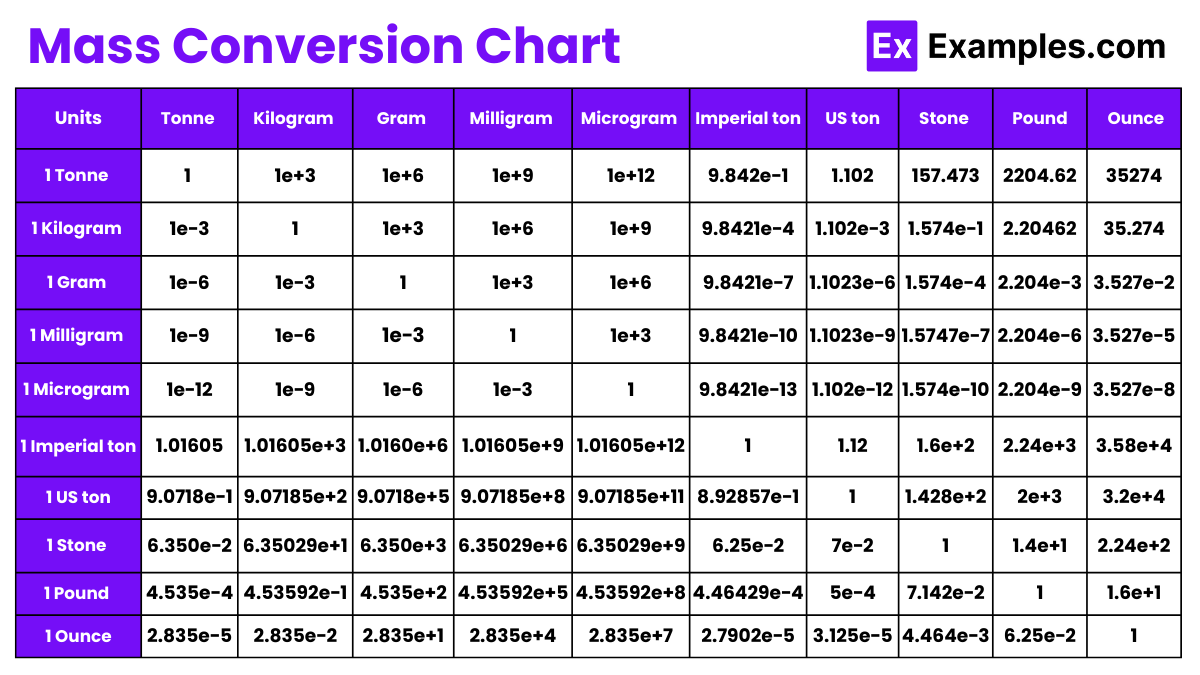
Mass Conversion Chart
How to use Mass Converter
Step 1: Select the Input Unit
In the mass converter tool, select the unit of mass you want to convert from. This could be units such as grams (g), kilograms (kg), pounds (lb), ounces (oz), etc.
Step 2: Enter the Value
Enter the numerical value of the mass you want to convert. For example, if you want to convert 10 kilograms, type “10” in the input field.
Step 3: Select the Output Unit
Choose the unit of mass you want to convert to. This could be grams (g), kilograms (kg), pounds (lb), ounces (oz), etc.
Step 4: Perform the Conversion
Click on the convert button or equivalent action in the tool to perform the conversion.
Step 5: View the Result
The converted value will be displayed in the output unit you selected. Note down the result for your reference.
Step 6: Repeat if Necessary
If you have more conversions to make, repeat the process by changing the input value and selecting different units as needed.
Are mass converters accurate?
Yes, mass converters are typically accurate as they use standard conversion factors. However, always double-check with reliable sources if you require precise measurements for critical tasks.
Can I convert very large or very small mass values?
Yes, most mass converters can handle a wide range of values, from very small masses like milligrams (mg) to very large masses like tons (t).
How can I verify the accuracy of a mass converter?
You can verify the accuracy by cross-referencing with standard conversion tables or using multiple mass converter tools to compare results.
Can I use a mass converter for scientific purposes?
Yes, mass converters are useful for scientific purposes, especially when you need quick and accurate conversions between different units of mass.
Is it possible to convert mass to volume using a mass converter?
No, mass converters specifically handle mass units. To convert mass to volume, you need to know the density of the substance and use a separate density-based conversion tool.
Can I use a mass converter for scientific purposes?
Yes, mass converters are useful for scientific purposes, especially when you need quick and accurate conversions between different units of mass.

