Orbits of Planets and Satellites

Last Updated: September 23, 2024

Notes

The orbits of planets and satellites are fundamental concepts in classical mechanics, crucial for understanding celestial motion in AP Physics. These orbits are governed by gravitational forces, leading to predictable paths around larger bodies like the Sun or Earth. The study involves applying Newton’s law of gravitation, Kepler’s laws, and analyzing circular and elliptical orbits. Mastery of these concepts is essential for solving problems related to planetary motion, satellite trajectories, and understanding the dynamics of our solar system in AP Physics.

Free AP Physics C: Mechanics Practice Test

Learning Objectives

In studying Orbits of Planets and Satellites for the AP Physics exam, you should aim to understand the application of Newton’s law of universal gravitation in orbital motion, analyze and apply Kepler’s laws of planetary motion, and calculate orbital velocity, period, and energy for both circular and elliptical orbits. You should also comprehend the concept of escape velocity, distinguish between different types of orbits (LEO, MEO, GEO), and apply these principles to solve problems involving gravitational forces, satellite motion, and planetary dynamics.

Orbits of Planets and Satellites

Understanding the orbits of planets and satellites is crucial for excelling in the AP Physics exam. This topic is rooted in classical mechanics and gravitation, and it involves the application of Newton’s laws, Kepler’s laws, and the concepts of circular and elliptical orbits.

1. Newton’s Law of Universal Gravitation

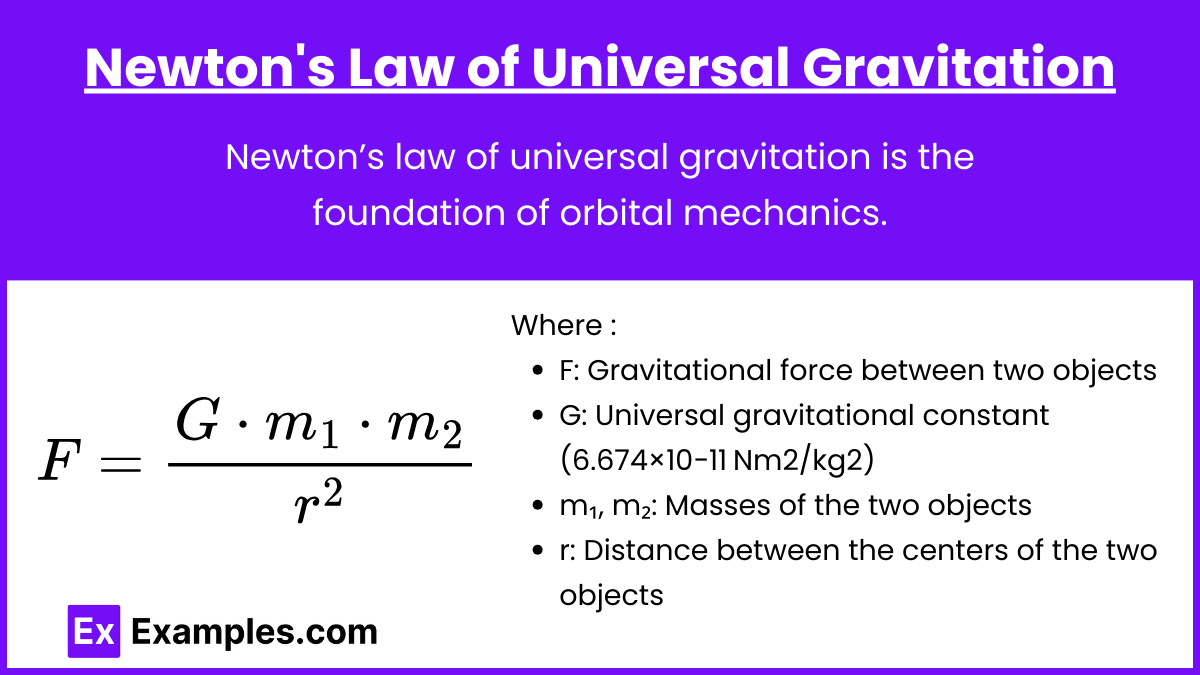
Newton's Law of Universal Gravitation (1)

Newton’s law of universal gravitation is the foundation of orbital mechanics. It states that every mass in the universe attracts every other mass with a force that is directly proportional to the product of their masses and inversely proportional to the square of the distance between them.

$F = \frac{G \cdot m_{1} \cdot m_{2}}{r^{2}}$

F: Gravitational force between two objects
G: Universal gravitational constant (6.674×10⁻¹¹ Nm²/kg²)
m₁, m₂: Masses of the two objects
r: Distance between the centers of the two objects

2. Kepler’s Laws of Planetary Motion

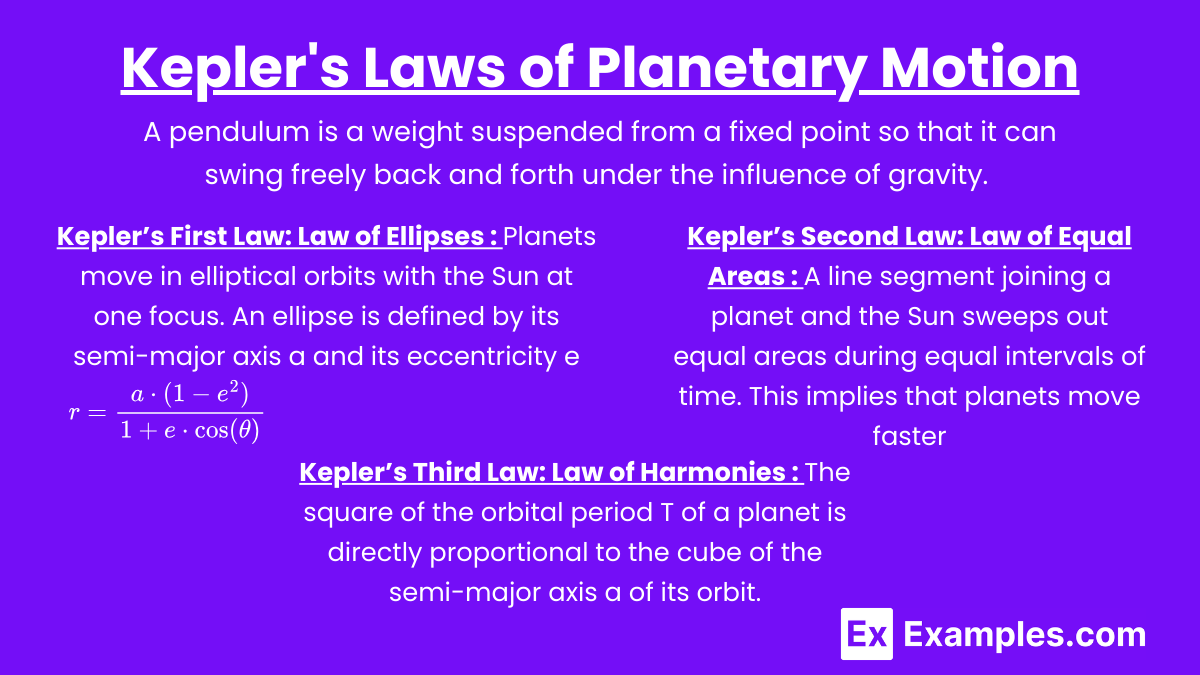
Johannes Kepler formulated three laws that describe the motion of planets around the Sun. These laws apply not only to planets but also to any satellite orbiting a larger body.

Kepler’s First Law: Law of Ellipses

Planets move in elliptical orbits with the Sun at one focus. An ellipse is defined by its semi-major axis a and its eccentricity e. The distance between the Sun and a planet varies as the planet travels along its orbit.

$r = \frac{a \cdot (1 - e^{2})}{1 + e \cdot \cos(\theta)}$

r: Distance from the Sun to the planet at any point in the orbit
a: Semi-major axis of the ellipse
e: Eccentricity of the orbit
θ: True anomaly (angle relative to the closest approach to the Sun)

Kepler’s Second Law: Law of Equal Areas

A line segment joining a planet and the Sun sweeps out equal areas during equal intervals of time. This implies that planets move faster when they are closer to the Sun (perihelion) and slower when they are farther from the Sun (aphelion).

Kepler’s Third Law: Law of Harmonies

The square of the orbital period T of a planet is directly proportional to the cube of the semi-major axis a of its orbit.

$T^{2} \propto a^{3}$

Or, in a more precise form for two bodies orbiting each other:

$T^{2} = \frac{4\pi^{2} \cdot a^{3}}{G(M + m)}$

T: Orbital period
a: Semi-major axis
M: Mass of the central body (e.g., the Sun)
m: Mass of the orbiting body (e.g., a planet)

3. Orbital Velocity

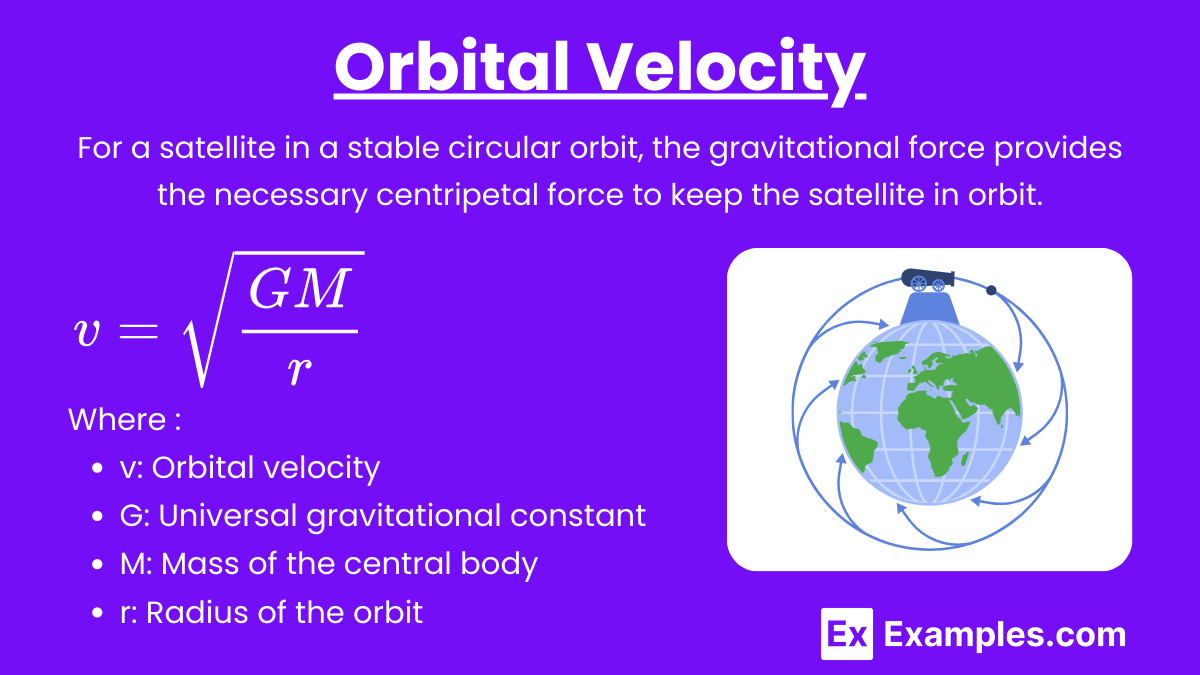
For a satellite in a stable circular orbit, the gravitational force provides the necessary centripetal force to keep the satellite in orbit. The orbital velocity v is given by:

$v = \sqrt{\frac{GM}{r}}$

v: Orbital velocity
G: Universal gravitational constant
M: Mass of the central body
r: Radius of the orbit (distance from the center of the central body to the satellite)

4. Geostationary Orbits

A geostationary orbit is a special type of orbit where a satellite remains stationary relative to a fixed point on the Earth’s surface. This requires the satellite to have an orbital period equal to the Earth’s rotational period (about 24 hours) and to orbit in the Earth’s equatorial plane.

Orbital radius for geostationary orbit: Approximately 42,164 km from the Earth’s center
Orbital period: 24 hours

5. Energy in Orbits

The total mechanical energy E of a satellite in orbit is the sum of its kinetic energy K and gravitational potential energy U

$E = K + U = \frac{1}{2}mv^{2} - \frac{GMm}{r}$

For a circular orbit, this simplifies to: $E = -\frac{GMm}{2r}$

Kinetic energy: K = 1/2 mv²
Potential energy: U = −GMm/r

In elliptical orbits, the energy considerations become more complex, but the total energy still remains constant.

6. Escape Velocity

Escape velocity is the minimum speed needed for an object to escape the gravitational pull of a planet or any other celestial body. It is derived from the condition that the total energy (kinetic + potential) of the object becomes zero at infinity.

$v_{\text{esc}} = \sqrt{\frac{2GM}{r}}$

vₑₛc: Escape velocity
G: Universal gravitational constant
M: Mass of the central body
r: Distance from the center of the body to the object

For Earth, the escape velocity is approximately 11.2 km/s.

7. Types of Orbits

Low Earth Orbit (LEO): Altitude of 160-2,000 km, orbital period around 90 minutes.
Medium Earth Orbit (MEO): Altitude of 2,000-35,786 km, includes GPS satellites.
Geostationary Orbit (GEO): Altitude of 35,786 km, orbital period of 24 hours.
Elliptical Orbits: Used for certain communication satellites, often have high eccentricity.

Examples

Example 1: Earth’s Orbit Around the Sun

Earth follows an elliptical orbit around the Sun, completing one full revolution in approximately 365.25 days. This orbit, known as the ecliptic, is slightly tilted relative to the Sun’s equator, contributing to the changing seasons. The gravitational pull of the Sun keeps Earth in this stable orbit, balancing the centrifugal force resulting from its motion.

Example 2: Moon’s Orbit Around Earth

The Moon orbits Earth in an elliptical path, taking about 27.3 days to complete one revolution. This orbit is inclined about 5 degrees to the plane of Earth’s orbit around the Sun. The gravitational interaction between Earth and the Moon causes tidal effects on Earth, and over time, it has led to the Moon being tidally locked, always showing the same face to Earth.

Example 3: Mars’ Orbit Around the Sun

Mars also orbits the Sun in an elliptical path, with an average distance of 228 million kilometers from the Sun. It takes Mars about 687 Earth days to complete one orbit. The elliptical nature of its orbit causes varying distances from the Sun, leading to significant differences in surface temperature between its perihelion (closest point to the Sun) and aphelion (farthest point).

Example 4: Geostationary Satellite Orbit

A geostationary satellite orbits Earth at an altitude of approximately 35,786 kilometers, directly above the equator. It takes exactly 24 hours to complete one orbit, matching Earth’s rotation. This synchronous orbit allows the satellite to remain fixed over a single point on Earth’s surface, making it ideal for communications, weather monitoring, and broadcasting.

Example 5: Jupiter’s Moon Io

Io, one of Jupiter’s largest moons, orbits the gas giant at an average distance of 421,700 kilometers. Io completes an orbit around Jupiter in about 1.77 Earth days. Its orbit is slightly elliptical and is heavily influenced by Jupiter’s strong gravitational field, as well as by the gravitational pulls of other moons like Europa and Ganymede. This results in significant tidal heating, making Io the most volcanically active body in the solar system.

Multiple Choice Questions

Question 1

Which of the following best describes the shape of the orbits of planets around the Sun according to Kepler’s First Law?

A) Circular
B) Elliptical
C) Parabolic
D) Hyperbolic

Correct Answer: B) Elliptical

Explanation: Kepler’s First Law, also known as the Law of Ellipses, states that the orbits of planets around the Sun are elliptical in shape, with the Sun located at one of the two foci of the ellipse. This means that the distance between the planet and the Sun changes as the planet moves along its orbit. The elliptical shape allows for varying orbital speeds and distances, which are key to understanding planetary motion.

Question 2

Which of the following factors primarily determines the orbital speed of a satellite orbiting Earth?

A) The mass of the satellite
B) The distance from the Earth
C) The mass of the Earth
D) The size of the satellite

Correct Answer: B) The distance from the Earth

Explanation: The orbital speed of a satellite is primarily determined by its distance from the center of the Earth. According to the laws of orbital mechanics, specifically Kepler’s Third Law, the closer a satellite is to Earth, the faster it must travel to remain in orbit. This is because the gravitational force exerted by Earth is stronger at shorter distances, requiring a higher speed to balance the gravitational pull and maintain a stable orbit.

Question 3

According to Newton’s Law of Universal Gravitation, if the mass of a planet were to double while the distance between the planet and a satellite remained the same, how would the gravitational force between them change?

A) It would remain the same
B) It would double
C) It would halve
D) It would quadruple

Correct Answer: B) It would double

Explanation: Newton’s Law of Universal Gravitation states that the gravitational force between two objects is directly proportional to the product of their masses and inversely proportional to the square of the distance between them. If the mass of the planet doubles, the gravitational force exerted on the satellite would also double, assuming the distance between the planet and the satellite remains constant. This is because the gravitational force F is given by the equation $F = \frac{G \cdot m_{1} \cdot m_{2}}{r^{2}}$ , where G is the gravitational constant, m₁ and m₂ are the masses of the two objects, and rrr is the distance between them.