Power

Last Updated: September 23, 2024

Notes

Power is a critical concept in AP Physics, representing the rate at which work is done or energy is transferred in a system. It quantifies how quickly energy is used, making it essential in understanding everything from mechanical engines to electrical circuits. Measured in watts, power combines force, motion, and energy, offering insights into the efficiency and performance of various physical processes. Mastery of this concept is vital for success on the AP Physics exam, as it appears across multiple topics and problem types.

Free AP Physics C: Mechanics Practice Test

Learning Objectives

When studying the topic of Power for the AP Physics exam, you should focus on understanding the definition of power as the rate of work done or energy transferred. Master the formulas for calculating power in both mechanical and electrical systems, including average and instantaneous power. Learn to apply these formulas in various contexts, such as force-velocity relationships and electrical circuits. Additionally, understand the significance of power efficiency and its practical applications, while also being able to solve related problems accurately and efficiently.

Power

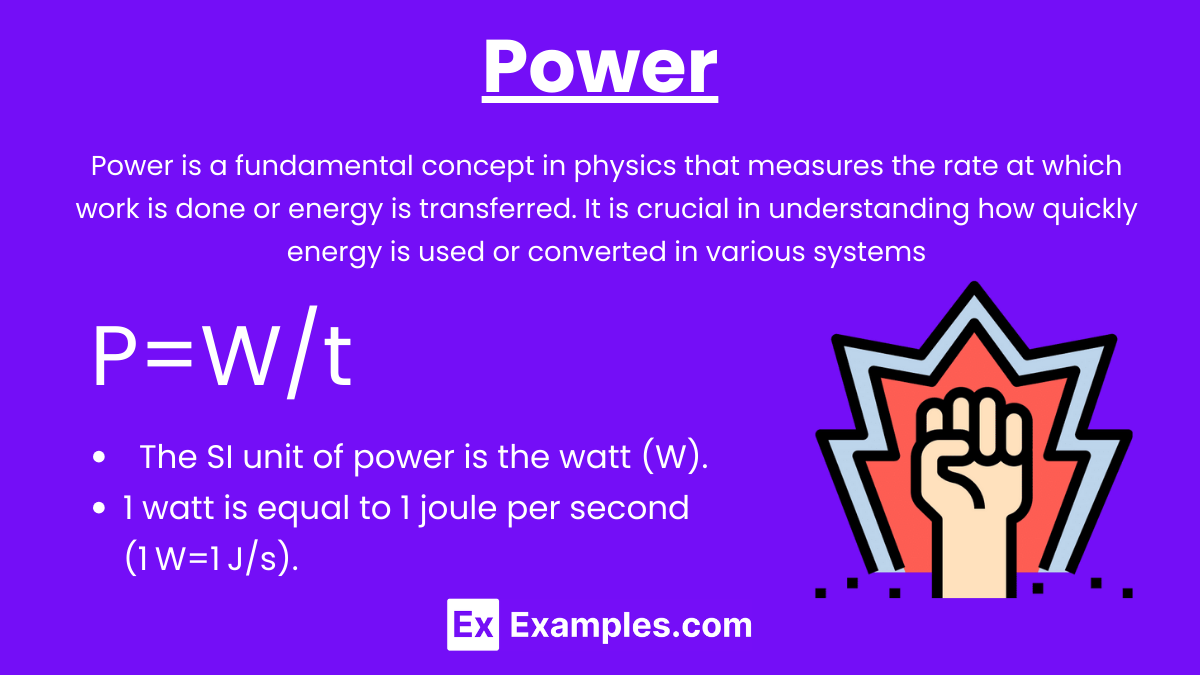
Power is a fundamental concept in physics that measures the rate at which work is done or energy is transferred. It is crucial in understanding how quickly energy is used or converted in various systems, from mechanical devices to electrical circuits.

Power (P) is defined as the amount of work done (W) or energy transferred (E) per unit of time (t).

$P = \frac{W}{t} (or) \frac{E}{t}$

SI Unit of Power

The SI unit of power is the watt (W).
1 watt is equal to 1 joule per second (1 W=1 J/s).

Dimensional Formula

The dimensional formula of power is M¹L²T⁻³, where:

M represents mass,
L represents length,
T represents time.

Types of Power

Mechanical Power
Electrical Power

Mechanical Power

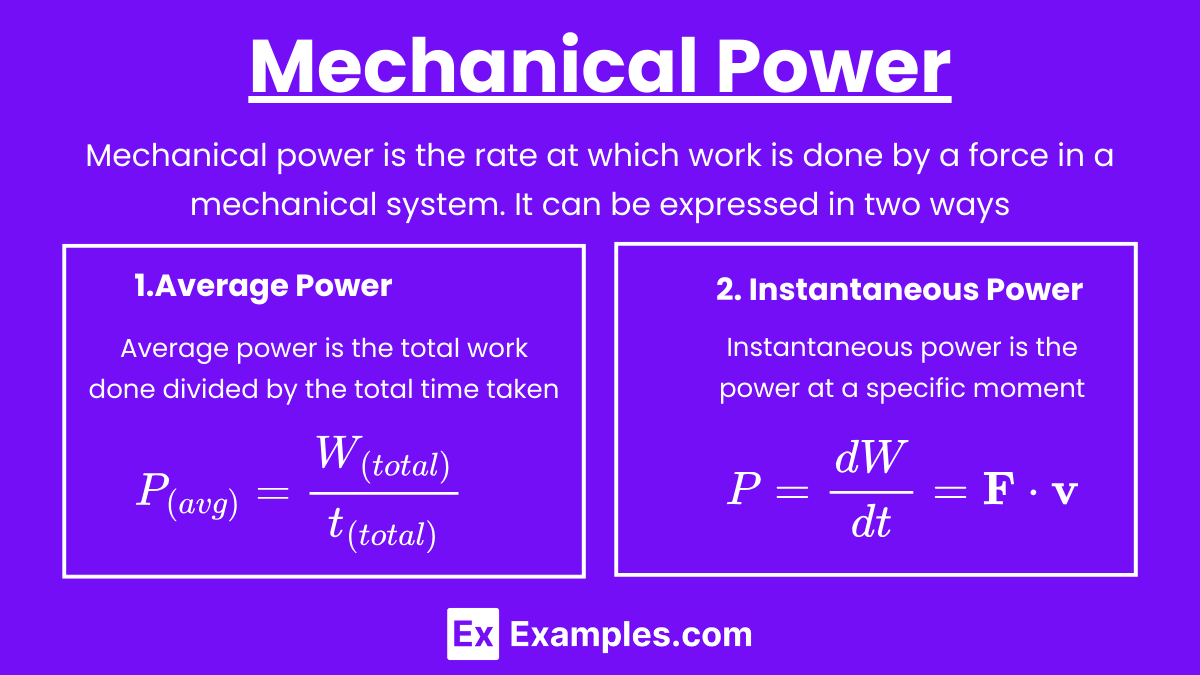
Mechanical power is the rate at which work is done by a force in a mechanical system. It can be expressed in two ways:

1. Average Power

Average power is the total work done divided by the total time taken:

$P_{(avg)} = \frac{W_{(total)}}{t_{(total)}}$

2. Instantaneous Power

Instantaneous power is the power at a specific moment and is calculated using:

$P = \frac{dW}{dt} = \mathbf{F} \cdot \mathbf{v}$

Here, F is the force applied, and v is the velocity of the object.
Instantaneous power can also be given by the dot product of force and velocity vectors.

Electrical Power

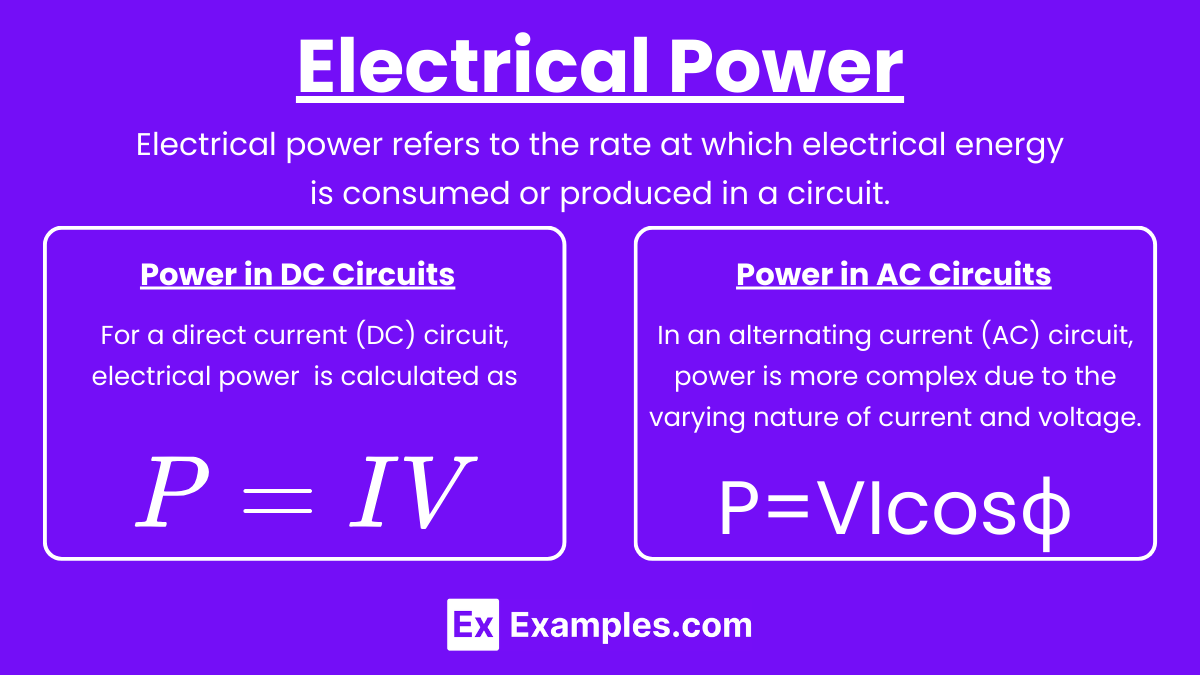
Electrical power refers to the rate at which electrical energy is consumed or produced in a circuit.

1. Power in DC Circuits

For a direct current (DC) circuit, electrical power is calculated as:

$P = IV$

I is the current (in amperes),
V is the voltage (in volts).

Using Ohm’s Law (V=IR), electrical power can also be expressed as:

$P = I^{2}R \quad \text{or} \quad P = \frac{V^{2}}{R}$

2. Power in AC Circuits

In an alternating current (AC) circuit, power is more complex due to the varying nature of current and voltage. Power in AC circuits is generally described using the following:

Apparent Power (S): The product of the root-mean-square (RMS) voltage and current. It is measured in volt-amperes (VA).
Real Power (P): The actual power consumed, calculated as P=VIcos⁡ϕ, where ϕ is the phase difference between current and voltage. It is measured in watts (W).
Reactive Power (Q): The power stored and then released by inductors and capacitors in the circuit, given by Q=VIsin⁡ϕ, measured in volt-amperes reactive (VAR).

Work-Energy Theorem and Power

According to the work-energy theorem, the work done on an object is equal to the change in its kinetic energy. Therefore, power can also be related to energy changes:

$P = \frac{\Delta E}{t}$

Power Efficiency

Efficiency of a system is the ratio of useful power output to total power input:

$\text{Efficiency}(\eta) = \frac{\text{Useful Power Output}}{\text{Total Power Input}} \times 100\%$

Applications of Power

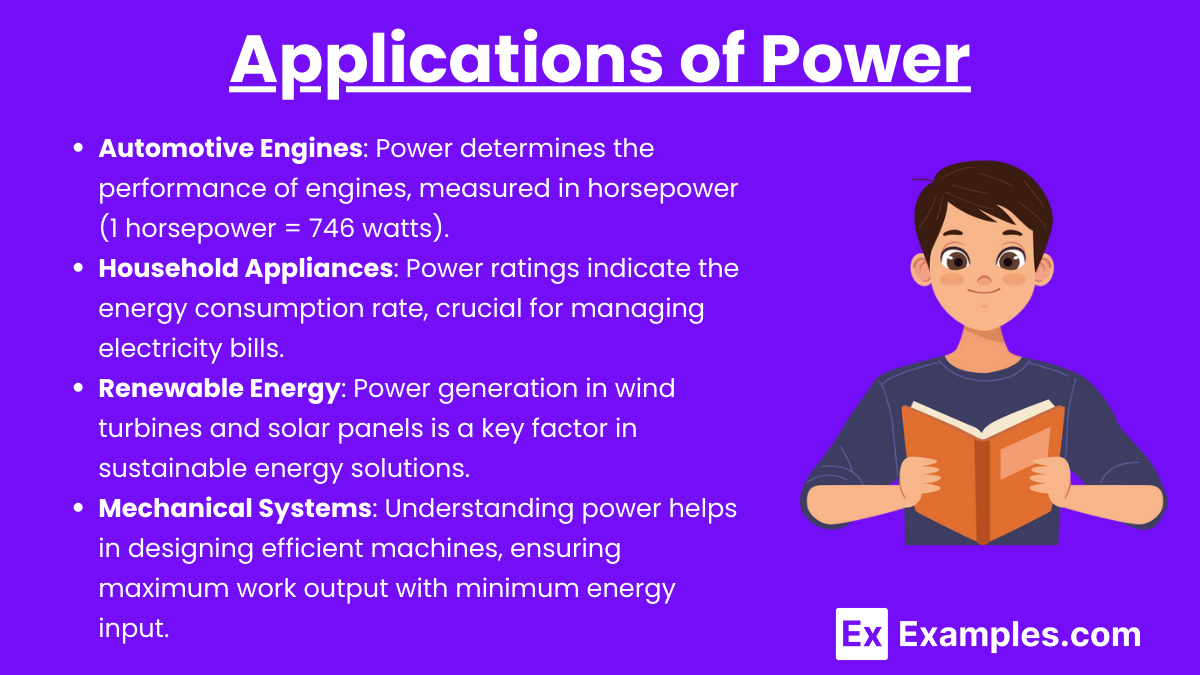
Automotive Engines: Power determines the performance of engines, measured in horsepower (1 horsepower = 746 watts).
Household Appliances: Power ratings indicate the energy consumption rate, crucial for managing electricity bills.
Renewable Energy: Power generation in wind turbines and solar panels is a key factor in sustainable energy solutions.
Mechanical Systems: Understanding power helps in designing efficient machines, ensuring maximum work output with minimum energy input.

Examples

Example 1: Electric Power in Households

The electricity that powers your home appliances, such as lights, refrigerators, and televisions, is a common example of power. This electrical power is generated by power plants and distributed to homes, allowing devices to operate and perform tasks that would otherwise require manual effort.

Example 2: Mechanical Power in Vehicles

The engine of a car produces mechanical power by converting fuel into energy. This power is used to turn the wheels and propel the vehicle forward. Without mechanical power, vehicles like cars, buses, and airplanes wouldn’t be able to move or perform their intended functions.

Example 3: Solar Power for Energy

Solar panels convert sunlight into electrical power through the photovoltaic effect. This renewable energy source is becoming increasingly popular as it provides a sustainable way to generate power for homes, businesses, and even spacecraft.

Example 4: Human Muscle Power

When you lift a heavy object, ride a bicycle, or push a lawnmower, you’re using your muscle power. This form of power is generated by the muscles in your body as they contract and exert force to perform physical tasks.

Example 5: Hydraulic Power in Construction Equipment

Machines like bulldozers, cranes, and excavators use hydraulic power to lift heavy loads and perform other demanding tasks. This power is generated by pressurized fluid in a hydraulic system, which allows the equipment to move with great force and precision.

Multiple Choice Questions

Question 1

A light bulb has a power rating of 60 watts. If it operates for 2 hours, how much energy does it consume?

A) 120 joules
B) 120 watt-hours
C) 30 joules
D) 30 watt-hours

Answer: B) 120 watt-hours

Explanation: Power is the rate at which energy is consumed or transferred. The power rating of 60 watts means the bulb uses 60 joules of energy per second. To find the total energy consumed, multiply the power by the time in hours:

$\text{Energy} = \text{Power} \times \text{Time} = 60\,\text{W} \times 2\,\text{hours} = 120\,\text{watt-hours}$

This means the light bulb consumes 120 watt-hours of energy in 2 hours.

Question 2

If a machine does 500 joules of work in 10 seconds, what is its power output?

A) 50 watts
B) 5 watts
C) 5000 watts
D) 0.02 watts

Answer: A) 50 watts

Explanation:

Power is defined as the amount of work done per unit of time. To calculate power:

$\text{Power} = \frac{\text{Work}}{\text{Time}} = \frac{500\,\text{joules}}{10\,\text{seconds}} = 50\,\text{watts}$

This means the machine has a power output of 50 watts, which indicates it transfers 50 joules of energy every second.

Question 3

Which of the following units is NOT a unit of power?

A) Watt
B) Joule per second
C) Newton-meter per second
D) Joule

Answer: D) Joule

Explanation:

Power is the rate at which energy is transferred or converted, and its SI unit is the watt (W), which is equivalent to joules per second (J/s). Newton-meter per second is also a unit of power because:

$1\,\text{Watt} = 1\,\text{Joule/second} = 1\,\text{Newton-meter/second}$

However, a joule (J) is a unit of energy, not power. Therefore, Joule is not a unit of power.