Work–Energy Theorem
The Work-Energy Theorem is a fundamental concept in physics that states the work done by the net force on an object equals the change in its kinetic energy. This theorem is crucial in solving problems related to motion and energy in AP Physics. By understanding the relationship between force, displacement, and energy, students can analyze various physical scenarios more effectively. Mastery of the Work-Energy Theorem is essential for excelling in AP Physics exams, as it forms the basis for more complex topics.
Learning Objectives
By studying the Work-Energy Theorem for the AP Physics exam, you should understand how to calculate work done by forces and relate it to changes in kinetic energy. You should be able to derive and apply the theorem in various contexts, such as determining the motion of objects under different forces. Additionally, focus on problem-solving techniques involving work, kinetic energy, and conservation of energy, ensuring you can accurately analyze scenarios like stopping distances, lifting objects, and projectile motion using the Work-Energy Theorem.
Introduction to Work-Energy Theorem
The Work–Energy Theorem is a fundamental principle in physics that connects the concepts of work and kinetic energy. It states that the work done by all the forces acting on an object is equal to the change in the object’s kinetic energy.This theorem states that the work done on an object by the net force acting on it is equal to the change in its kinetic energy. Mathematically, it is expressed as:
\( W_{net} = \Delta K \)
Where:
- Wnet is the net work done on the object.
- ΔK is the change in kinetic energy of the object.
This theorem is crucial for solving problems related to the motion of objects and understanding the relationship between forces and energy.
Understanding Work and Kinetic Energy
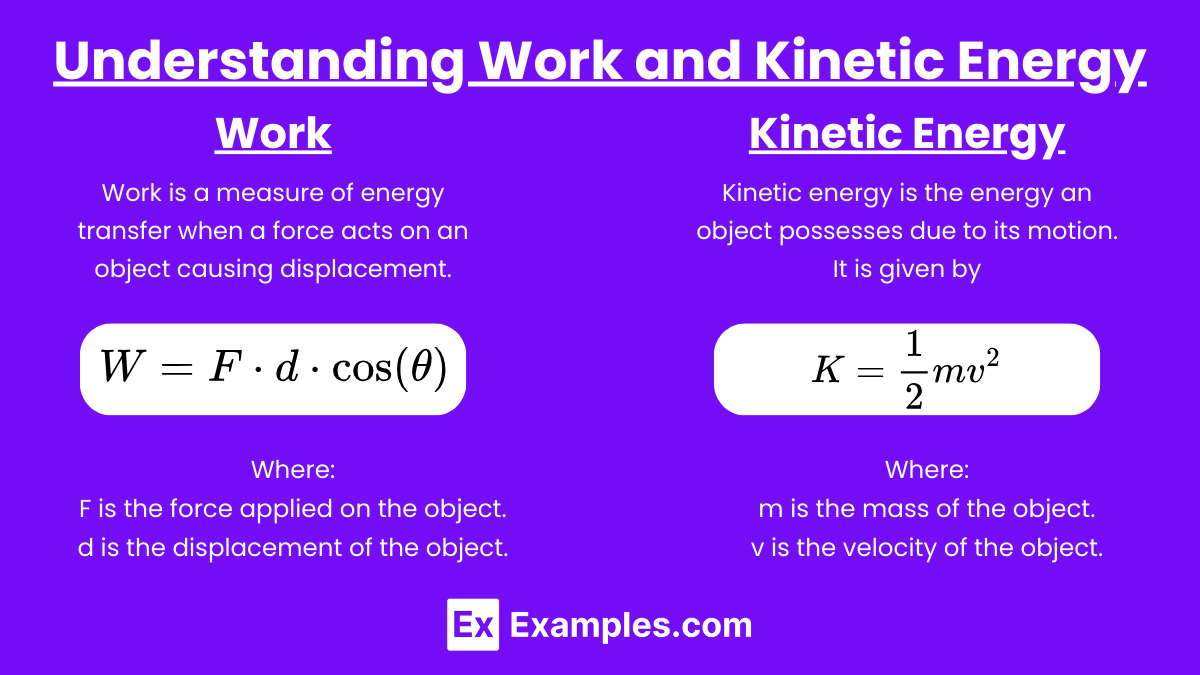
Work is a measure of energy transfer when a force acts on an object causing displacement. It is calculated using:
\( W = F \cdot d \cdot \cos(\theta) \)
Where:
- F is the force applied on the object.
- d is the displacement of the object.
- θ is the angle between the force and the displacement vectors.
- Positive Work: When the force component is in the direction of the displacement.
- Negative Work: When the force component is opposite to the displacement.
- Zero Work: When the force is perpendicular to the displacement or when there is no displacement.
Kinetic Energy (K): Kinetic energy is the energy an object possesses due to its motion. It is given by:
\( K = \frac{1}{2}mv^{2} \)
Where:
- m is the mass of the object.
- v is the velocity of the object.
Deriving the Work–Energy Theorem
To derive the Work–Energy Theorem, consider an object of mass mmm moving under the influence of a net force Fnet. By Newton’s Second Law:
\(\vec{F}_{\text{net}} = m \vec{a} \)
Where a is the acceleration. The net work done Wnet can be written as:
\( W_{\text{net}} = \int \vec{F}_{\text{net}} \cdot d\vec{s} \)
Substituting Fnet=ma and using the definition of acceleration a=dv/dt, we get:
\( W_{\text{net}} = m \int \frac{d\vec{v}}{dt} \cdot d\vec{s} \)
From the kinematic relationship, v dv=d(1/2 v2), the equation becomes:
\( W_{\text{net}} = \int m \, d\left(\frac{1}{2}v^2\right) \)
\( W_{\text{net}} = \Delta \left(\frac{1}{2}mv^2\right) \)
Thus, we arrive at the Work–Energy Theorem:
\( W_{\text{net}} = \Delta K \)
Applications of the Work-Energy Theorem
The Work-Energy Theorem is particularly useful in solving problems where forces and displacements are involved. Here are some common applications:
- Stopping Distance of a Car: When brakes are applied to a moving car, the work done by the frictional force reduces the car’s kinetic energy to zero, bringing it to a stop. The theorem helps calculate the stopping distance based on the initial speed and the braking force.
- Projectile Motion: The work-energy theorem can analyze the motion of a projectile, such as determining the speed of a projectile at different points in its trajectory.
- Elevators and Lifting Objects: The theorem can be used to determine the work required to lift an object to a certain height, considering gravitational forces.
Examples
Example 1: Falling Object
Consider a ball that is dropped from a height. As the ball falls, gravity does work on it, converting potential energy into kinetic energy. The work done by gravity equals the change in the ball’s kinetic energy. If air resistance is negligible, the increase in kinetic energy equals the loss of potential energy, perfectly demonstrating the Work-Energy Theorem.
Example 2: Car Braking
When a car comes to a stop, the brakes do negative work on the car. This work decreases the car’s kinetic energy to zero. The amount of work done by the braking force is equal to the loss of kinetic energy, showing that the work done on the car corresponds to the change in its energy.
Example 3: Compressing a Spring
If you compress a spring, you exert a force over a distance, doing work on the spring. This work is stored as elastic potential energy. According to the Work-Energy Theorem, the work you do is equal to the increase in the spring’s potential energy, illustrating the transfer of energy through work.
Example 4: Projectile Motion
A projectile launched at an angle follows a curved path under the influence of gravity. The work done by gravity as the projectile rises or falls changes its kinetic and potential energy. The Work-Energy Theorem explains that the net work done on the projectile by all forces is equal to its change in kinetic energy, accounting for both the horizontal and vertical components of motion.
Example 5: Lifting a Weight
When you lift a weight vertically at a constant speed, you do work against gravity. This work increases the gravitational potential energy of the weight. According to the Work-Energy Theorem, the work you do on the weight is equal to the increase in its potential energy, demonstrating the direct relationship between work and energy.
Multiple Choice Questions
Question 1
A 2 kg object is pushed with a constant force of 10 N along a frictionless horizontal surface for a distance of 5 meters. What is the increase in the object’s kinetic energy?
a) 20 J
b) 25 J
c) 50 J
d) 100 J
Answer: c) 50 J
Explanation: The Work–Energy Theorem states that the work done on an object is equal to the change in its kinetic energy. Work done W is given by:
W = F × d
where:
- F=10 N (force applied)
- d=5 m (distance)
So, the work done is: W=10 N×5 m=50 J
Since the surface is frictionless, all the work done goes into increasing the object’s kinetic energy. Therefore, the increase in kinetic energy is 50 J.
Question 2
A car accelerates from rest under the influence of a constant force. If the work done by the force is 500 J500 \, \text{J}500J, what is the final kinetic energy of the car?
a) 250 J
b) 500 J
c) 1000 J
d) 2000 J
Answer: b) 500 J
Explanation: According to the Work–Energy Theorem, the work done on an object is equal to its change in kinetic energy. The car starts from rest, so its initial kinetic energy KEi is 0. The work done by the force W is 500 J, which will be entirely converted into the car’s kinetic energy. Therefore, the final kinetic energy KEf is:
KEf = W = 500 J
Question 3
The Work–Energy Theorem states that the work done on an object is equal to what?
a) The object’s weight
b) The change in the object’s kinetic energy
c) The object’s potential energy
d) The object’s momentum
Answer: b) The change in the object’s kinetic energy
Explanation: The Work–Energy Theorem states that the work done by all forces acting on an object is equal to the change in the object’s kinetic energy. This means that if work is done on an object, its kinetic energy will increase or decrease depending on whether the work is positive or negative.