Describing how Quantities Change with respect to each other in a Parametric Function

Last Updated: September 23, 2024

Notes

In AP Precalculus, parametric functions are used to describe how two variables change with respect to a third parameter, often time. Instead of relating one variable directly to another, parametric equations express both variables as separate functions of the parameter. This allows for a more flexible representation of motion or other dynamic systems. By analyzing the rates of change of each variable through derivatives, students can understand how quantities vary in relation to each other, providing insights into curves, slopes, and paths traced by these functions.

Free AP Precalculus Practice Test

Learning Objectives

When studying how quantities change with respect to each other in a parametric function for AP Precalculus, you should focus on understanding how to express both x and y as functions of a parameter (often time,t), and how to compute their rates of change using derivatives. You should learn how to interpret the relationships between these variables, find the slope of parametric curves, and describe the overall behavior of the system. Additionally, you should understand real-world applications like circular motion and motion in planes.

Understanding Parametric Functions

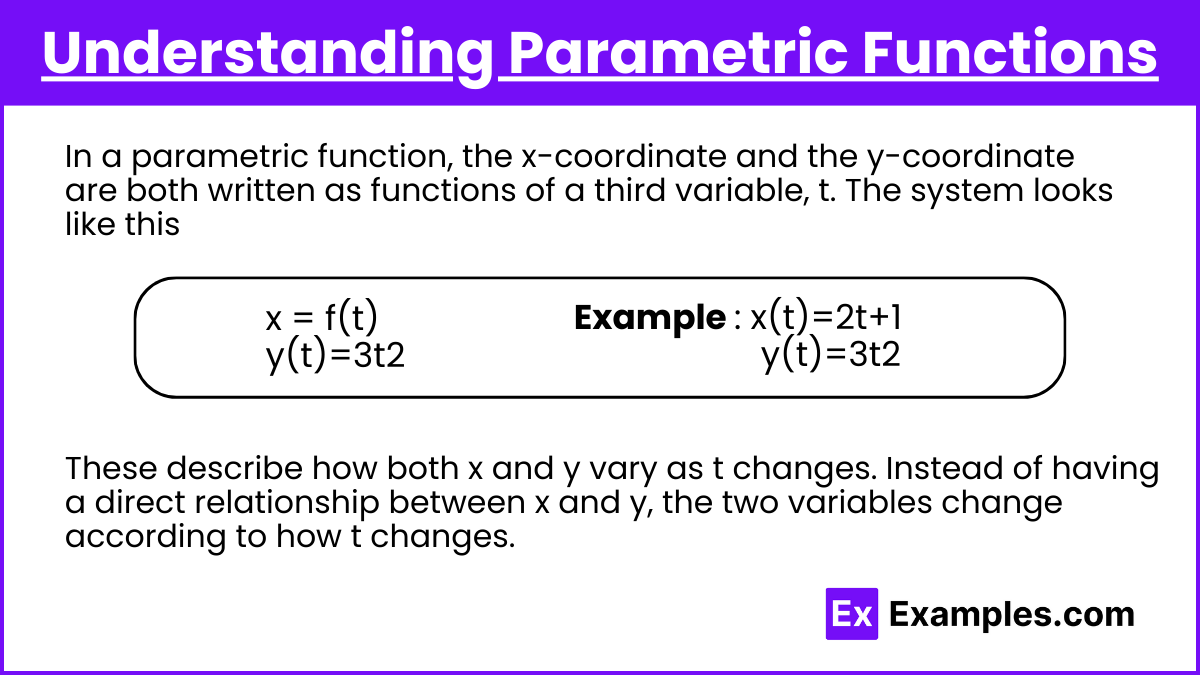
In a parametric function, the x-coordinate and the y-coordinate are both written as functions of a third variable, t. The system looks like this:

x = f(t)
y = g(t)

For example, if you have two parametric equations:

x(t)=2t+1
y(t)=3t²

These describe how both x and y vary as t changes. Instead of having a direct relationship between x and y, the two variables change according to how t changes.

Rates of Change in Parametric Equations

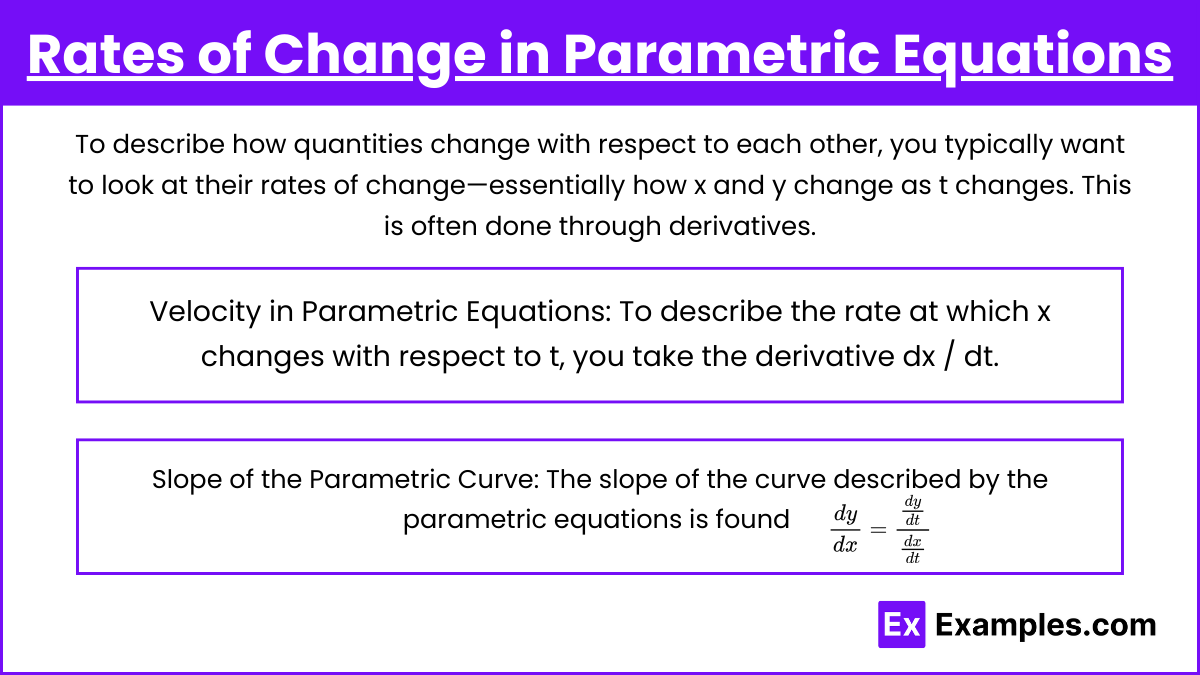
To describe how quantities change with respect to each other, you typically want to look at their rates of change—essentially how x and y change as t changes. This is often done through derivatives.

Velocity in Parametric Equations:
- To describe the rate at which x changes with respect to t, you take the derivative $\frac{dx}{dt}$ .
- Similarly, to describe the rate at which y changes with respect to t, you take the derivative $\frac{dy}{dt}$ .
Slope of the Parametric Curve: The slope of the curve described by the parametric equations is found
$\frac{dy}{dx} = \frac{\frac{dy}{dt}}{\frac{dx}{dt}}$ . This expresses how y changes with respect to x at any given point on the curve. It’s essentially the rate at which one quantity changes with respect to another, which provides insights into the behavior of the system described by the parametric function.

Relationships Between Quantities

By analyzing the derivatives of x(t) and y(t), you can understand how changes in one quantity (e.g., horizontal position) affect the other quantity (e.g., vertical position). For example, in the case of the circle:

When sin(t) is 0, cos(t) is at its maximum, meaning the horizontal position is changing fastest while the vertical position is stationary.

Examples

Example 1. Projectile Motion

In physics, the trajectory of a projectile can be described using parametric equations. Let $x(t) = v_0 \cos(\theta)t$ represent the horizontal distance and $y(t) = v_0 \sin(\theta)t - \frac{1}{2}gt^2$ represent the vertical height, where v₀ is the initial velocity, θ is the angle of projection, and g is the acceleration due to gravity. As t increases, both x and y change based on their dependence on time. The horizontal distance increases linearly while the vertical height follows a parabolic trajectory, showing a clear relationship between the two quantities.

Example 2. Circular Motion

A point moving along a circle with radius r can be described by the parametric equations x(t) = rcos(t) and y(t) = rsin(t), where t represents the angle (in radians). As t increases, the quantities x and y oscillate sinusoidally. The values of x and y are interdependent, with their rates of change being connected to the angular velocity. This relationship describes how the point moves around the circle, and the two quantities represent the horizontal and vertical positions that change with respect to time.

Example 3. Elliptical Orbit

The motion of a planet around the sun can be modeled using parametric equations of an ellipse. If a and b are the major and minor axes, the parametric equations are x(t) = acos(t) and y(t) = bsin(t). As t changes, the position of the planet changes in the elliptical path. The horizontal and vertical displacements vary at different rates, as described by the rates of change ( \frac{dx}{dt} \) and $\frac{dy}{dt}$ , reflecting the relationship between the two quantities as the planet moves through different parts of its orbit.

Example 4. Cycloid Motion

A cycloid describes the path traced by a point on the rim of a rolling circle. The parametric equations are x(t) = r(t−sin(t)) and y(t) = r(1−cos(t)), where r is the radius of the circle and t is the parameter representing time. As the circle rolls, both the horizontal and vertical displacements of the point change in a cyclical manner. The horizontal displacement increases steadily, while the vertical displacement oscillates as the point traces the curve. This example shows how the two quantities are related, as they change simultaneously.

Example 5. Ferris Wheel

The position of a person on a Ferris wheel can be described parametrically. If r is the radius of the Ferris wheel and ω is the angular velocity, then the parametric equations for the position are x(t) = rcos(ωt) and y(t) = rsin(ωt)+h, where h is the height of the wheel’s center. As the wheel rotates, the person’s horizontal and vertical positions change with time, with the two quantities being interdependent. The horizontal position follows a sinusoidal pattern, while the vertical position reflects the upward and downward motion of the ride.

Multiple Choice Questions

Question 1

Given the parametric equations x(t) = t² and y(t) = 3t+1, what is the slope $\frac{dy}{dx}$ at t = 1?

A) 1
B) 2
C) 3
D) 0.5

Answer: C) 3

Explanation:
To find $\frac{dy}{dx}$ , use $\frac{dy}{dx} = \frac{\frac{dy}{dt}}{\frac{dx}{dt}}$ :

$\frac{dx}{dt} = 2t \quad \text{and} \quad \frac{dy}{dt} = 3$ At t = 1:
$\frac{dx}{dt} = 2 \quad \text{and} \quad \frac{dy}{dt} = 3$

$\frac{dy}{dx} = \frac{3}{2} = 1.5$

Question 2

For the parametric equations x(t) = 4cos⁡(t) and y(t) = 4sin⁡(t), what is the value of $\frac{dy}{dx}$ at t = 0?

A) 0
B) 1
C) -1
D) Undefined

Answer: A) 0

Explanation:
At t = 0:

$\frac{dx}{dt} = -4\sin(0) = 0 \quad \text{and} \quad \frac{dy}{dt} = 4\cos(0) = 4$
Since $\frac{dy}{dx} = \frac{4}{0}$ , the slope is 0.

Question 3

Given x(t) = t³ and y(t) = t²−1, at which value of t does the slope $\frac{dy}{dx} = 0$ ?

A) t = 0
B) t = 1
C) t = -1
D) t = 2

Answer: A) t = 0

Explanation:
To Find $\frac{dy}{dx} = 0$

$\frac{dy}{dx} = \frac{2t}{3t^2}$
Set this equal to 0: $\frac{2t}{3t^2} = 0 \quad \Rightarrow \quad t = 0$