Graphing Conic Sections Using Implicitly defined Functions and Parametric Functions

Last Updated: September 23, 2024

Notes

Graphing conic sections involves visualizing curves like circles, ellipses, parabolas, and hyperbolas, which can be represented using implicitly defined functions or parametric equations. Implicit equations define relationships between x and y, capturing these shapes through a general quadratic form. Parametric equations introduce a third variable, allowing the conic’s position to be defined more flexibly, especially useful when traditional methods fall short. Mastering both approaches is crucial for understanding the geometric properties and practical applications of conic sections in AP Precalculus.

Free AP Precalculus Practice Test

Learning Objectives

When studying “Graphing Conic Sections Using Implicitly Defined Functions and Parametric Functions” for AP Precalculus, focus on understanding how to represent circles, ellipses, parabolas, and hyperbolas using both implicit equations and parametric equations. Learn how to convert between these forms, interpret the geometric meaning of the parameters, and graph conic sections accurately. Also, focus on the flexibility parametric equations offer in modeling complex curves and how they can be used to represent non-function relationships. Practice applying these concepts to real-world scenarios.

Conic sections are curves formed by the intersection of a plane with a cone, resulting in various shapes like circles, ellipses, parabolas, and hyperbolas. These sections can be represented both as implicitly defined functions and through parametric equations.

Implicitly Defined Functions

Conic sections can often be described by an equation of the form Ax²+Bxy+Cy²+Dx+Ey+F = 0, where different values of the constants determine the specific conic section:

Circle: The equation of a circle has the form x²+y² = r², representing a set of points equidistant from the center.
Ellipse: The general equation for an ellipse is a2x²+b²y² = 1, where a and b are the lengths of the semi-major and semi-minor axes.
Parabola: A parabola is represented by y = ax²+bx+c or, in implicit form, Ax²+Dx+Ey+F = 0.
Hyperbola: The equation for a hyperbola is $\frac{x^2}{a^2} + \frac{y^2}{b^2} = 1$ , which involves two separate curves.

In implicit form, solving the equation for y in terms of x (or vice versa) might not always yield a single function, which is why conic sections often require more sophisticated approaches for graphing, such as parametric equations.

Parametric Equations for Conic Sections

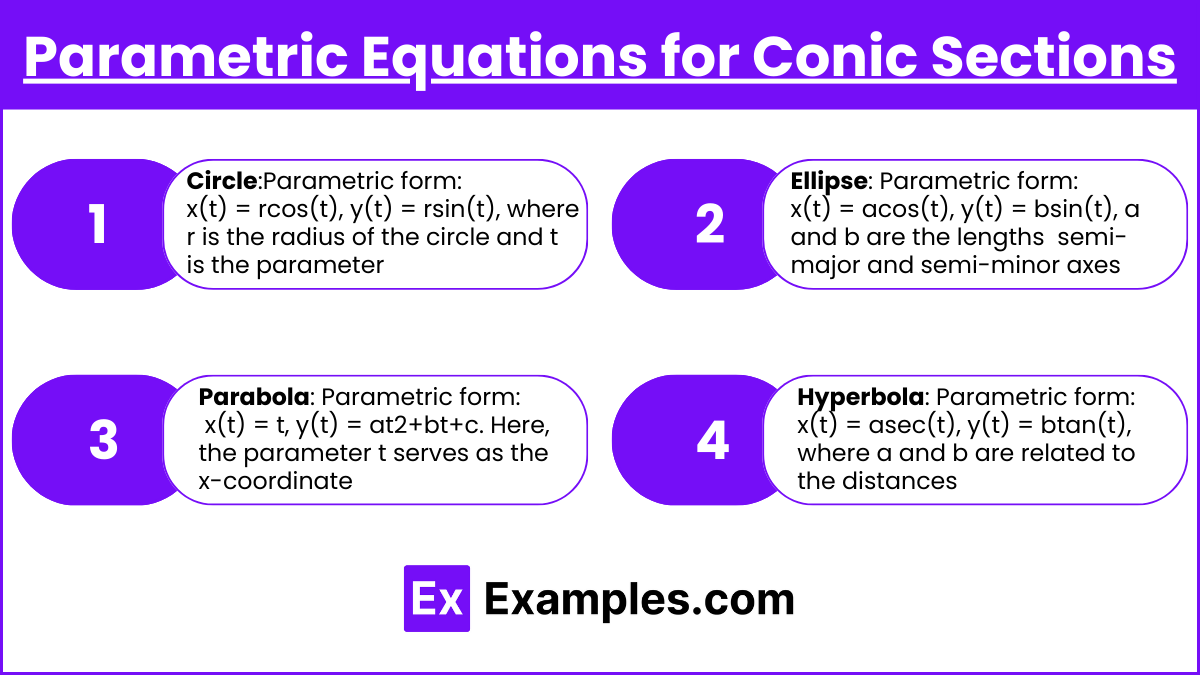
Parametric equations allow you to define the position of a point on a conic section as a function of a third variable, called the parameter, usually denoted t. This is especially useful when graphing conic sections that do not pass the vertical line test or when dealing with curves that are difficult to solve explicitly.

Circle:
- Parametric form: x(t) = rcos(t), y(t) = rsin(t), where r is the radius of the circle and t is the parameter (usually 0 ≤ t < 2π).
Ellipse:
- Parametric form: x(t) = acos(t), y(t) = bsin(t), where a and b are the lengths of the semi-major and semi-minor axes, respectively.
Parabola:
- Parametric form: x(t) = t, y(t) = at²+bt+c. Here, the parameter t serves as the x-coordinate, and y is computed based on the quadratic form.
Hyperbola:
- Parametric form: x(t) = asec(t), y(t) = btan(t), where a and b are related to the distances between the center and the vertices or foci of the hyperbola.

Why Parametric Equations are Useful

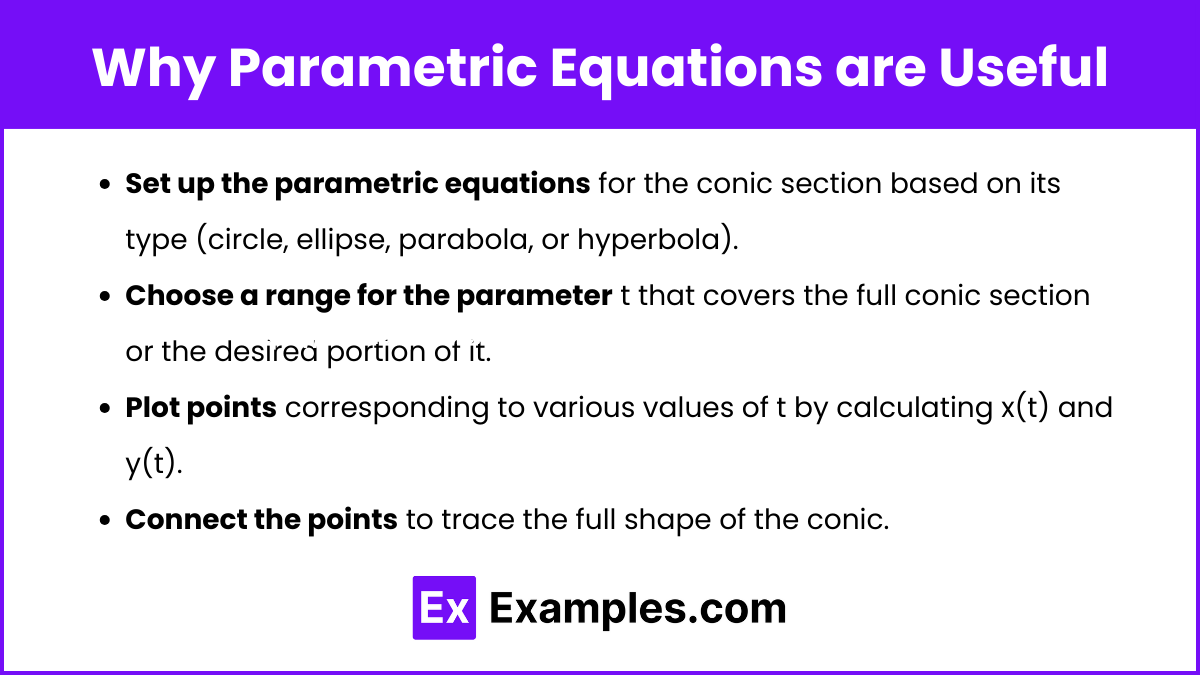
Parametric equations allow us to easily represent the curve even when the equation may not have a unique function form in Cartesian coordinates. They also enable the modeling of the motion of objects along the curve by varying the parameter t. This is particularly useful in real-world applications like physics and engineering, where time (or another parameter) is an essential part of the system being modeled.Steps to Graph Conic Sections Parametrically:

Set up the parametric equations for the conic section based on its type (circle, ellipse, parabola, or hyperbola).
Choose a range for the parameter t that covers the full conic section or the desired portion of it.
Plot points corresponding to various values of t by calculating x(t) and y(t).
Connect the points to trace the full shape of the conic.

Examples

Example 1: Circle

A circle can be implicitly defined by the equation x²+y²=25, representing a circle with a radius of 5 and centered at the origin. The parametric form for this circle is given by x(t) = 5cos(t) and y(t) = 5sin(t), where t ranges from 0 to 2π. Graphing this parametric function produces the same circular shape, but it allows us to visualize how points move around the circle as t changes.

Example 2: Ellipse

An ellipse is represented implicitly by the equation 16x²+9y² = 1, with the semi-major axis length a = 4 and semi-minor axis length b = 3. The parametric equations for this ellipse are x(t) = 4cos(t) and y(t) = 3sin(t), where t ranges from 0 to 2π. The parametric form not only traces the entire ellipse but also allows for precise control over how the curve is generated.

Example 3: Parabola

A parabola can be implicitly defined by the equation y = 2x²−3x+1, a standard quadratic form. The parametric form for this parabola can be written as x(t) = t and y(t) = 2t²−3t+1, where t is a parameter that directly replaces the x-value. By varying t, the parametric form generates the same parabolic curve as the implicit equation, giving a more dynamic perspective on the graphing process.

Example 4: Hyperbola

A hyperbola can be implicitly defined by the equation $\frac{x^2}{9} - \frac{y^2}{4} = 1$ , which describes a hyperbola centered at the origin with vertices at (±3,0). The parametric form of this hyperbola is x(t) = 3sec(t) and y(t) = 2tan(t), where t is the parameter. This parametric equation can be used to trace the two branches of the hyperbola as t varies, avoiding the difficulty of solving for y explicitly in the implicit form.

Example 5: Rotated Conic Section (Ellipse with Tilt)

Consider the implicitly defined equation for a rotated ellipse: x²+xy+y² = 10. This represents an ellipse that is rotated, making it challenging to graph using standard methods. However, by using parametric equations, we can express this rotated ellipse as x(t) = 3cos(t)−sin(t) and y(t) = 2cos(t)+sin(t), where t is the parameter. The parametric form simplifies graphing this complex curve, providing an efficient way to represent the rotation and maintain the shape of the ellipse.

Multiple Choice Questions

Question 1

Which of the following is the parametric equation for a circle centered at the origin with radius r?

A) x(t) = rcos(t), y(t) = rsin(t)
B) x(t) = rsin(t), y(t) = rcos(t)
C) x(t) = rcosh(t), y(t) = rsinh(t)
D) x(t) = rsec(t), y(t) = rtan(t)

Answer: A) x(t) = rcos(t), y(t) = rsin(t)

Explanation: The parametric equation of a circle is derived from the standard equation x²+y²=r². This can be represented parametrically as x = rcos(t) and y = rsin(t), where t is the parameter (usually in radians), representing the angle around the circle from the positive x-axis. Choices B and D are incorrect because they reverse the cosine and sine or represent incorrect functions. Choice C represents a hyperbolic function, not a circular function.

Question 2

Which of the following equations represents a hyperbola in implicit form?

A) $\frac{x^2}{a^2} + \frac{y^2}{b^2} = 1$
B) $\frac{x^2}{a^2} - \frac{y^2}{b^2} = 1$
C) $x^2 + y^2 = r^2$
D) $y = ax^2 + bx + c$

Answer: B) $\frac{x^2}{a^2} - \frac{y^2}{b^2} = 1$

Explanation: A hyperbola is represented by the implicit equation $\frac{x^2}{a^2} - \frac{y^2}{b^2} = 1$ , where the minus sign indicates the subtraction of the y²-term from the x²-term. This reflects the two branches of a hyperbola, which open either horizontally or vertically. Option A is the equation of an ellipse, option C is the equation of a circle, and option D is the equation of a parabola, making B the correct answer.

Question 3

Which of the following statements is true about parametric equations for conic sections?

A) Parametric equations can only describe circles.
B) Parametric equations are useful when the relation between x and y cannot be described by a single function.
C) Parametric equations always describe hyperbolas.
D) Parametric equations are rarely used in graphing conic sections.

Answer: B) Parametric equations are useful when the relation between x and y cannot be described by a single function.

Explanation: Parametric equations are powerful tools for describing curves where the relationship between x and y may not be a function (i.e., when the graph fails the vertical line test, as is the case with circles and ellipses). In parametric form, both x and y are functions of a third variable (the parameter, usually denoted t), allowing for more flexibility in graphing. Option A is incorrect because parametric equations can describe many conic sections, not just circles. Option C is incorrect because parametric equations can describe all types of conic sections (circles, ellipses, hyperbolas, and parabolas), and option D is incorrect because parametric equations are frequently used in graphing complex curves.