Geometric sequences and exponential functions are closely related mathematical concepts that describe patterns of growth or decay. In a geometric sequence, each term is found by multiplying the previous term by a fixed ratio, while exponential functions model continuous growth or decay using a constant base raised to a variable exponent. Both concepts share a similar structure, with geometric sequences representing discrete data points and exponential functions describing continuous changes. Understanding this relationship is key to analyzing patterns and solving problems in AP Precalculus, particularly in modeling real-world scenarios.
Free AP Precalculus Practice Test
Learning Objectives
In studying "Relating Geometric Sequences and Exponential Functions" for AP Precalculus, you should focus on understanding how geometric sequences are defined by a common ratio and how they correspond to exponential functions through their multiplicative structure. Learn to transition between discrete geometric sequences and continuous exponential functions, identifying similarities and differences. Practice modeling real-world problems, such as population growth or decay, using both geometric sequences and exponential functions. Additionally, recognize how changes in parameters like the common ratio or base affect the overall behavior of the function or sequence.
Relating Geometric Sequences and Exponential Functions
Geometric Sequences
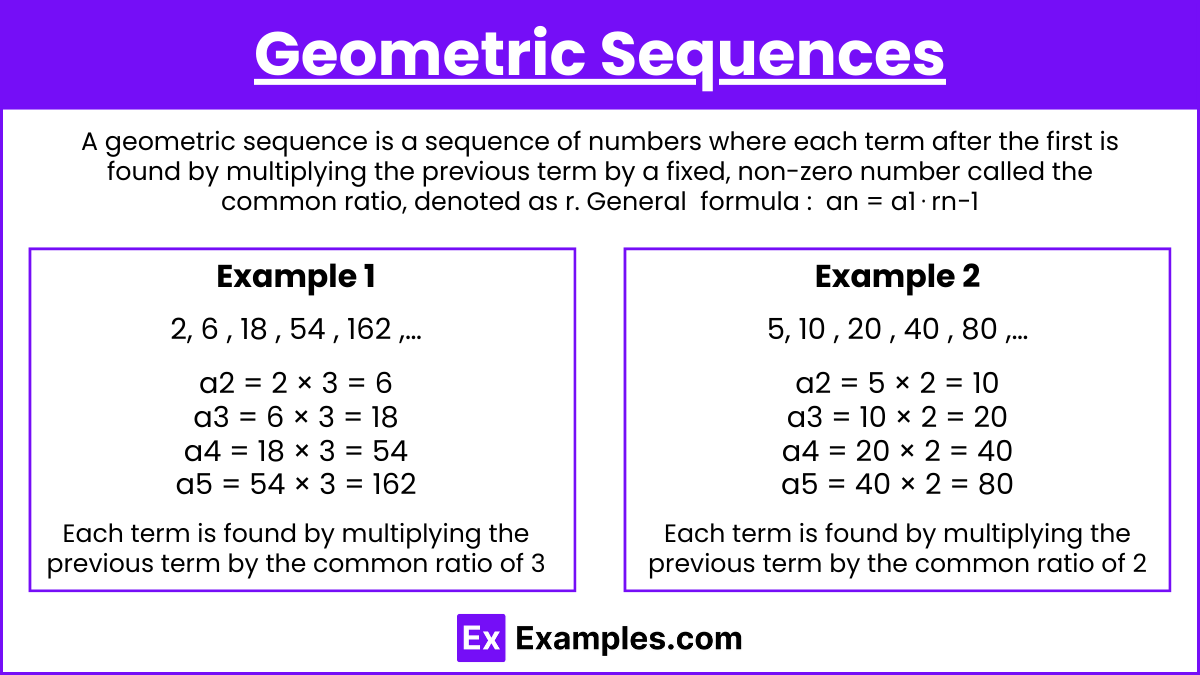
A geometric sequence is a sequence of numbers where each term after the first is found by multiplying the previous term by a fixed, non-zero number called the common ratio, denoted as r. The general form for the n-th term of a geometric sequence is:
an = a1⋅rn−1
where:
an is the n-th term,
a1 is the first term, and
r is the common ratio.
Example 1
Geometric Sequence: 2,6,18,54,162,…
First term (a1) = 2
Common ratio (r) = 3
Each term is found by multiplying the previous term by the common ratio of 3:
a2 = 2 × 3 = 6
a3 = 6 × 3 = 18
a4 = 18 × 3 = 54
a5 = 54 × 3 = 162
The general formula for the n-th term of this sequence is: an = 2⋅3n−1
Example 2
Geometric Sequence: 5,10,20,40,80,…
First term (a1) = 5
Common ratio (r) = 2
Each term is found by multiplying the previous term by the common ratio of 2:
a2 = 5 × 2 = 10
a3 = 10 × 2 = 20
a4 = 20 × 2 = 40
a5 = 40 × 2 = 80
The general formula for the n-th term of this sequence is: an=5⋅2n−1
Exponential Functions
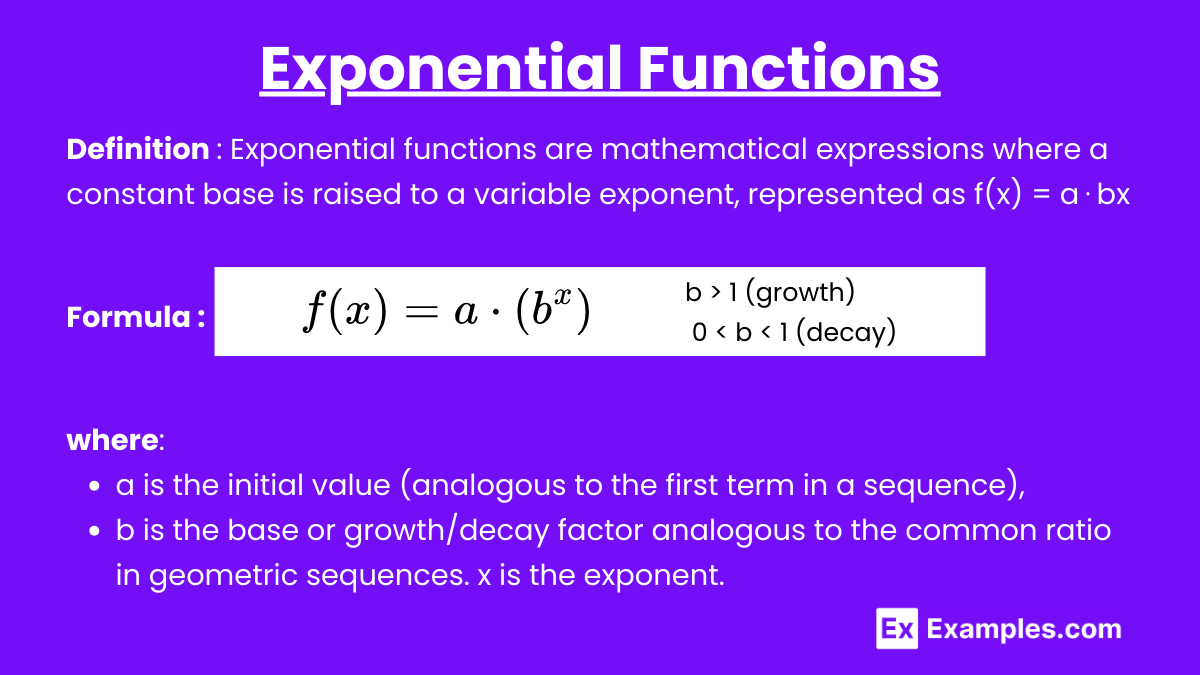
Exponential functions are mathematical expressions where a constant base is raised to a variable exponent, represented as f(x) = a⋅bx. These functions model growth or decay, depending on whether the base b > 1 (growth) or 0 < b < 1 (decay). They are used to describe phenomena like population growth, radioactive decay, and compound interest.
f(x) = a⋅bx
where:
a is the initial value (analogous to the first term in a sequence),
b is the base or growth/decay factor (analogous to the common ratio in geometric sequences),
x is the exponent.
Relationship Between Geometric Sequences and Exponential Functions
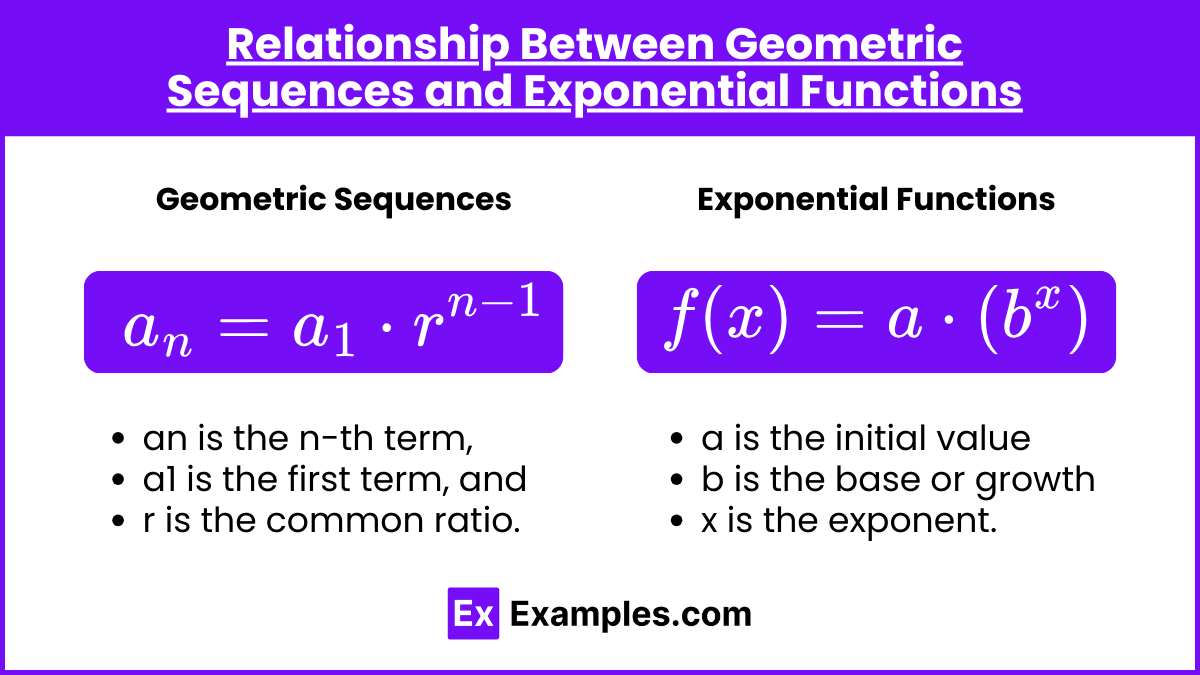
Common Structure: The relationship between geometric sequences and exponential functions lies in their similar structure. The formula for the n-th term of a geometric sequence is very much like an exponential function, where the sequence’s term number corresponds to the exponent in the exponential function.
Discrete vs. Continuous: Geometric sequences are a discrete form, where terms are only defined at integer values of n, whereas exponential functions are continuous and can take any real value of x.
Real-Life Applications: Both geometric sequences and exponential functions model real-world scenarios involving exponential growth or decay, such as population growth, interest rates, radioactive decay, or anything that involves proportional changes over time.
Transformation: Any geometric sequence can be represented as an exponential function by extending the sequence’s rule to a continuous domain. For instance, if a geometric sequence has the form an = 5⋅2n−1, the corresponding exponential function would be f(x) = 5⋅2x.
Rate of Change: The rate of change in a geometric sequence corresponds to the multiplicative factor (common ratio), while in exponential functions, this factor corresponds to the base of the exponent. For example, in both cases, the value multiplies by a fixed rate (r or b) over each step or unit of time.
Recursive Form of Geometric Sequences: Geometric sequences can also be written in a recursive form, where each term is defined in terms of the previous one: an = an−1⋅r. This highlights the multiplicative nature of change, similar to the continuous growth in exponential functions.
Examples
Example 1: Population Growth
In a town, the population is 1,000 people and grows by 5% each year. The growth follows a geometric sequence because each year’s population is 1.05 times the previous year’s population. The population after n years can be modeled by the geometric sequence formula Pn = 1000⋅(1.05)n. This is equivalent to the exponential function P(t) = 1000⋅1.05t, where t represents time in years.
Example 2: Investment Growth
An initial investment of $500 earns 8% interest annually. The value of the investment after n years follows the geometric sequence An = 500⋅(1.08)n. This represents the growing value over time, where the 8% increase per year is reflected as the common ratio of the geometric sequence. The same scenario is described by the exponential function A(t) = 500⋅1.08t, with t in years.
Example 3: Radioactive Decay
A radioactive substance decays at a rate of 10% per year. If the initial mass is 200 grams, the amount remaining after n years follows a geometric sequence Mn = 200⋅(0.9)n, where the common ratio is 0.9 (representing 90% of the substance left each year). This decay can also be modeled as an exponential function M(t) = 200⋅0.9t, showing the continuous nature of decay over time.
Example 4: Bacterial Growth
A bacteria culture starts with 50 cells and doubles every hour. The number of bacteria after n hours is given by the geometric sequence Bn = 50⋅2n, where the common ratio is 2, indicating the doubling. The continuous version of this growth is modeled by the exponential function B(t) = 50⋅2t, describing how the bacteria population grows over time.
Example 5: Depreciation of a Car's Value
The value of a new car depreciates by 15% annually. If the car’s initial value is $30,000, the value after n years is given by the geometric sequence Vn=30,000⋅(0.85)n, where the common ratio is 0.85, representing the 15% depreciation each year. The corresponding exponential function is V(t)=30,000⋅0.85t, showing how the car’s value declines over time.
Multiple Choice Questions
Question 1
Which of the following represents the relationship between a geometric sequence and an exponential function?
A. A geometric sequence and an exponential function always have a linear relationship.
B. A geometric sequence represents a discrete set of values, while an exponential function is continuous.
C. A geometric sequence is always a decreasing function, while exponential functions are always increasing.
D. A geometric sequence and an exponential function are both quadratic in nature.
Answer: B. A geometric sequence represents a discrete set of values, while an exponential function is continuous.
Explanation: A geometric sequence consists of terms defined for integer values of n, while an exponential function can take any real value of x. The structure of both is similar, but the geometric sequence is discrete, and the exponential function is continuous. The other options are incorrect because geometric sequences and exponential functions are not inherently linear, and they can be either increasing or decreasing, depending on the common ratio or base.
Question 2
Given the geometric sequence 3,6,12,24,…, what is the corresponding exponential function that represents this sequence?
A. f(x) = 3⋅2x
B. f(x) = 2⋅3x
C. f(x) = 3⋅2x−1
D. f(x) = 3⋅2x+1
Answer: A. f(x) = 3⋅2x
Explanation: The common ratio r in the geometric sequence is 2, since each term is multiplied by 2 to get the next term. The first term is 3. Therefore, the general form of the exponential function representing this sequence is f(x) = 3⋅2x. Option C uses x−1, which is not needed in the continuous version, and options B and D have incorrect initial values or growth factors.
Question 3
Which of the following statements about geometric sequences and exponential functions is true?
A. The sum of a geometric sequence is always equal to an exponential function.
B. Both geometric sequences and exponential functions describe situations where the change between successive terms is additive.
C. Geometric sequences and exponential functions describe multiplicative change, where each term or value is multiplied by a constant.
D. An exponential function cannot represent a geometric sequence with a negative common ratio.
Answer: C. Geometric sequences and exponential functions describe multiplicative change, where each term or value is multiplied by a constant.
Explanation:
Geometric sequences and exponential functions both describe multiplicative relationships. In geometric sequences, the common ratio r is the factor that determines how each term relates to the previous one, and in exponential functions, the base b plays this role. The sum of a geometric sequence is not always related to an exponential function (Option A is false), and these functions describe multiplicative rather than additive change (Option B is false). Option D is incorrect because exponential functions can represent geometric sequences with any real number as a ratio, including negative values.