Using Inverse Trigonometric Functions to Solve Trigonometric Equations

Last Updated: September 23, 2024

Notes

Inverse trigonometric functions are essential tools for solving trigonometric equations, particularly when the angle is unknown. These functions, including arcsin, arccos, and arctan, allow us to determine angles from known trigonometric ratios. In Precalculus, solving equations like sin⁡(θ) = x, cos⁡(θ) = y, or tan⁡(θ)=z involves isolating the trigonometric function and applying the corresponding inverse function. Understanding the domain, range, and periodic nature of trigonometric functions is crucial for finding all possible solutions within a specified interval.

Free AP Precalculus Practice Test

Learning Objectives

In studying “Using Inverse Trigonometric Functions to Solve Trigonometric Equations” for AP Precalculus, you should focus on learning how to apply arcsin, arccos, and arctan to solve for unknown angles in equations. You will understand the domains and ranges of inverse trigonometric functions, determine general solutions within specific intervals, and handle periodicity to find all possible solutions. Additionally, you should be able to interpret the meaning of these solutions in the context of trigonometric equations and solve equations involving composite functions with inverse trig functions.

Using Inverse Trigonometric Functions

Inverse trigonometric functions are critical tools for solving trigonometric equations. These functions are the inverses of the basic trigonometric functions—sine, cosine, and tangent—denoted as arcsin (sin⁻¹), arccos (cos⁻¹), and arctan (tan⁻¹). In trigonometric equations, inverse trigonometric functions allow us to find angles (often referred to as theta, θ) when given the value of a trigonometric ratio.

Basics of Inverse Trigonometric Functions

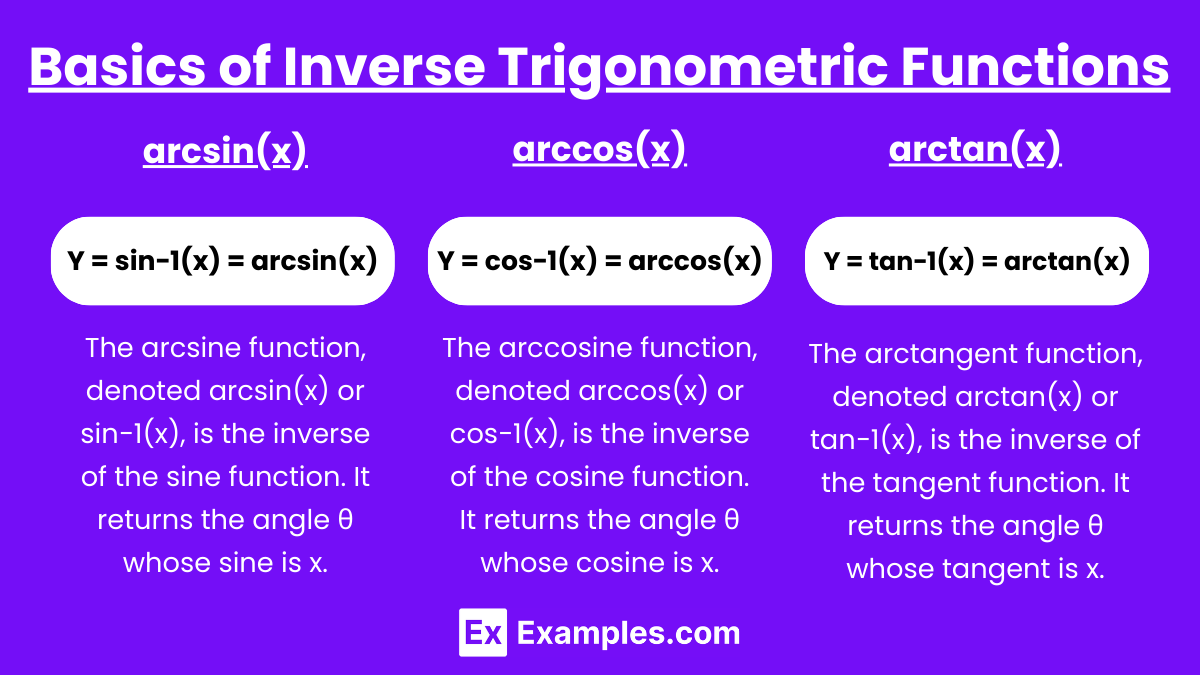
arcsin(x): The arcsine function, denoted arcsin(x) or sin−1(x), is the inverse of the sine function. It returns the angle θ whose sine is x. In other words, if sin(θ) = x, then arcsin(x) = θ.

Domain: −1 ≤ x ≤ 1 (The sine function only takes values between -1 and 1).
Range: −2π≤θ≤2π (quadrants I and IV).
Graphical Meaning: When finding arcsin(x), you are determining the angle θ for which the sine value is x, where the angle lies in the first or fourth quadrant.

arccos(x): The arccosine function, denoted arccos(x) or cos−1(x), is the inverse of the cosine function. It returns the angle θ whose cosine is x. In other words, if cos(θ) = x, then arccos(x) = θ.

Domain: −1≤ x ≤1 (Cosine also only takes values between -1 and 1).
Range: 0≤ θ ≤π (quadrants I and II).
Graphical Meaning: When finding arccos(x), you are determining the angle θ for which the cosine value is x, where the angle lies in the first or second quadrant.

arctan(x): The arctangent function, denoted arctan(x) or tan−1(x), is the inverse of the tangent function. It returns the angle θ whose tangent is x. In other words, if tan(θ) = x, then arctan(x) = θ.

Domain: All real numbers (−∞ < x < ∞).
Range: −2π < θ < 2π (quadrants I and IV).
Graphical Meaning: When finding arctan(x), you are determining the angle θ for which the tangent value is x, where the angle lies in the first or fourth quadrant.

Each of these inverse functions is defined on a specific restricted range, ensuring they return unique values:

Solving Trigonometric Equations Using Inverse Functions

When solving an equation like sin⁡(θ) = x, the solution can be found using θ = arcsin⁡(x), provided that −1 ≤ x ≤ 1, as the sine function has a restricted range of [−1,1]. Similarly, the general form of solutions for inverse cosine and tangent functions is given by θ = arccos⁡(x) and θ = arctan⁡(x), with their respective domain restrictions.

In solving trigonometric equations using inverse functions:

Isolate the trigonometric expression: First, rearrange the equation so that the trigonometric term is by itself on one side of the equation. For example, if you have an equation 2sin(x) = 1, first divide by 2 to get sin(x) = 0.5.
Apply the inverse function: Use the appropriate inverse trigonometric function to find the angle that corresponds to the trigonometric value. For example, if sin(x) = 0.5, then x = arcsin(0.5).
Consider the general solution: Since trigonometric functions are periodic, there are usually multiple solutions within a given range. For sine and cosine, you must consider the periodic nature of the functions. For example, if x = arcsin(0.5) gives you x = 30∘ or $\frac{\pi}{6}$ , you also need to consider that x = 180∘−30∘ = 150∘ or $\frac{5\pi}{6}$ is another solution.
Account for all solutions within the interval: If solving over a specific interval (e.g., [0,2π]), ensure you include all possible solutions by adding multiples of the period to the initial solution. For sine, the general solution is θ = arcsin(x) + 2kπ or θ = π−arcsin(x) + 2kπ, where k is any integer.

Special Considerations

When solving trigonometric equations with inverse functions, keep in mind:

Multiple solutions: Since trigonometric functions are periodic, there are often infinitely many solutions.
Principal values: The inverse functions return a principal value, but you must account for all possible angles that satisfy the equation.
Quadrants: Be mindful of which quadrants the solutions lie in, as the signs of the trigonometric functions change depending on the quadrant.

Examples

Example 1

Solve the equation sin(θ) = 0.5 for θ in the interval [0,2π].

To solve, use the inverse sine function: θ = arcsin(0.5). The principal solution is $\theta = \frac{\pi}{6}$ . However, since sine is positive in both the first and second quadrants, the second solution is $\theta = \pi - \frac{\pi}{6} = \frac{5\pi}{6}$ . Therefore, the solutions in the given interval are θ = 6π and θ = 65π.

Example 2

Solve $\cos(\theta) = -\frac{1}{2}$ for θ in the interval [0,2π].

Using the inverse cosine function, $\theta = \arccos\left(-\frac{1}{2}\right)$ , the principal value is $\theta = \frac{2\pi}{3}$ . Since cosine is negative in both the second and third quadrants, the second solution is $\theta = 2\pi - \frac{2\pi}{3} = \frac{4\pi}{3}$ . Thus, the solutions are $\theta = \frac{2\pi}{3}$ and $\theta = \frac{4\pi}{3}$ .

Example 3

Solve tan(θ)=1 for θ in the interval [0,2π].

The inverse tangent function gives $\theta = \arctan(1) = \frac{\pi}{4}$ . Since tangent is positive in both the first and third quadrants, the second solution is $\theta = \frac{\pi}{4} + \pi = \frac{5\pi}{4}$ . Therefore, the solutions are $\theta = \frac{\pi}{4}$ and $\theta = \frac{5\pi}{4}$ .

Example 4

Solve $\sin(\theta) = -\frac{\sqrt{3}}{2}$ for θ in the interval [0,2π].

Using the inverse sine function, $\theta = \arcsin\left(-\frac{\sqrt{3}}{2}\right)$ , the principal value is $\theta = -\frac{\pi}{3}$ , but adjusting to the interval [0,2π], the first solution is $\theta = 2\pi - \frac{\pi}{3} = \frac{5\pi}{3}$ . Since sine is negative in the third and fourth quadrants, the second solution is $\theta = \pi + \frac{\pi}{3} = \frac{4\pi}{3}$ . Thus, the solutions are $\theta = \frac{4\pi}{3}$ and $\theta = \frac{5\pi}{3}$ .

Example 5

Solve cos(θ) = 0.75 for θ in the interval [0,2π].

First, use the inverse cosine function: θ = arccos(0.75). The principal value is θ ≈ 0.7227. Since cosine is positive in both the first and fourth quadrants, the second solution is θ = 2π−0.7227 ≈ 5.5605. Therefore, the solutions are θ ≈ 0.7227 and θ≈5.5605.

Multiple Choice Questions

Question 1

What is the value of θ in the equation sin(θ)=0.5, where 0 ≤ θ ≤ 2π?

A. $\frac{\pi}{6}$
B. $\frac{\pi}{3}$
C. $\frac{\pi}{6} ) and ( \frac{5\pi}{6}$
D. $\frac{7\pi}{6}$

Answer is C

Explanation for Answer:

To solve sin(θ) = 0.5, you use the inverse sine function:

$\theta = \arcsin(0.5) = \frac{\pi}{6}$

Since sine is positive in both the first and second quadrants, the second solution within [0,2π] is found by using $\pi - \frac{\pi}{6} = \frac{5\pi}{6}$ . Thus, the two solutions are 6π and 65π.

Question 2

Solve cos(θ) = −0.5 for 0 ≤ θ ≤ 2π?

A. $\frac{2\pi}{3}$
B. $\frac{\pi}{3}$
C. $\frac{2\pi}{3}$ and $\frac{4\pi}{3}$
D. π

Answer is C

Explanation for Answer:

To solve cos(θ)=−0.5, use the inverse cosine function:

$\theta = \arccos(-0.5) = \frac{2\pi}{3}$

Cosine is negative in both the second and third quadrants. The additional solution in the third quadrant is:

$\theta = 2\pi - \frac{2\pi}{3} = \frac{4\pi}{3}$

Therefore, the solutions are $\frac{2\pi}{3}$ and $\frac{4\pi}{3}$ .

Question 3

Find the principal solution of the equation tan(θ) = 1, where 0 ≤ θ < π ?

A. $\frac{\pi}{4}$
B. $\frac{3\pi}{4}$
C. $\frac{\pi}{2}$
D. π

Answer is A

Explanation for Answer:

To solve tan(θ)=1, use the inverse tangent function:

$\theta = \arctan(1) = \frac{\pi}{4}$

Since the tangent function is positive in the first and third quadrants, the principal solution (the smallest positive solution within the given range) is 4π. There is no additional solution in [0,π) since the problem specifies the principal solution within the interval [0,π).