Using Vectors to Describe Motion of an Object

Last Updated: September 23, 2024

Notes

In AP Precalculus, vectors play a crucial role in describing the motion of objects by representing both magnitude and direction. Vectors are used to model physical quantities such as displacement, velocity, and acceleration. By breaking motion into components along the x, y, and z axes, vectors allow for a clear analysis of movement in two- and three-dimensional spaces. Understanding vectors helps in solving real-world problems, such as projectile motion and relative motion, by analyzing how objects move in different directions over time.

Free AP Precalculus Practice Test

Learning Objectives

In studying the topic “Using Vectors to Describe Motion of an Object” for AP Precalculus, you should focus on understanding how vectors represent both the magnitude and direction of quantities like displacement, velocity, and acceleration. You should be able to decompose vectors into components, calculate vector magnitudes, and determine direction angles. Additionally, you should learn to model real-world scenarios involving motion, such as projectile motion and relative motion, using vector operations and to interpret how velocity and acceleration vectors describe changes in motion over time.

Using Vectors to Describe Motion of an Object

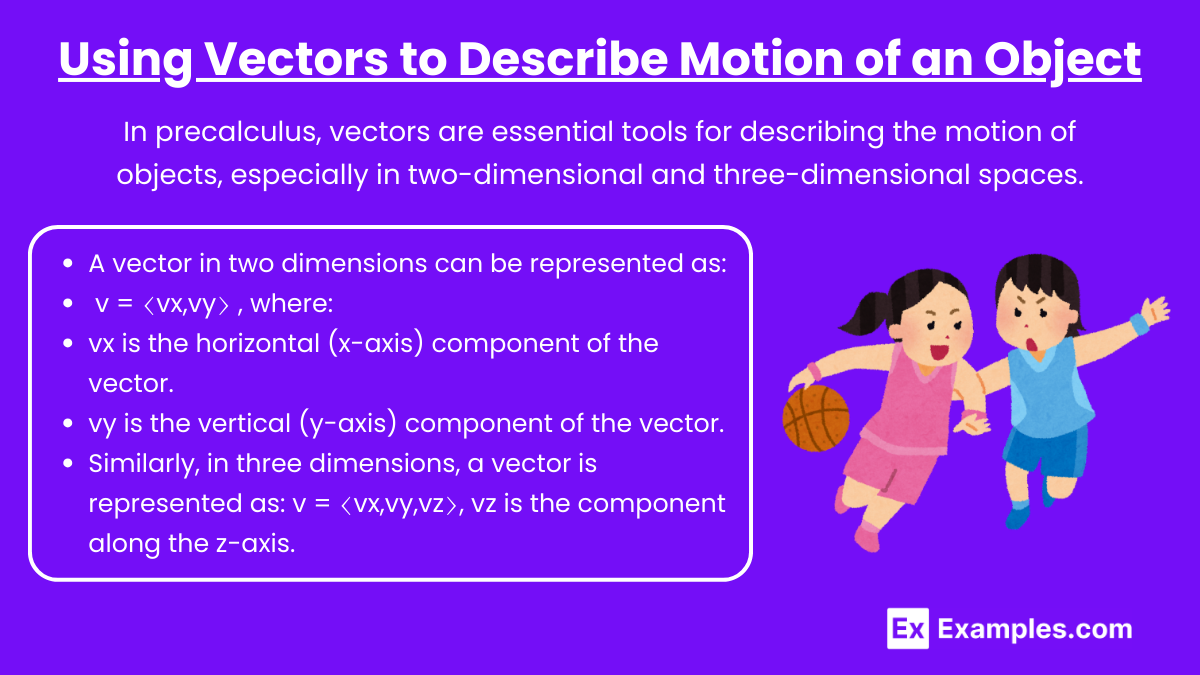
In precalculus, vectors are essential tools for describing the motion of objects, especially in two-dimensional and three-dimensional spaces. A vector has both magnitude (length) and direction, making it ideal for representing physical quantities like velocity and acceleration, which describe how an object moves.Components of a Vector

A vector in two dimensions can be represented as: v = ⟨vx,vy⟩

where:

vx is the horizontal (x-axis) component of the vector.
vy is the vertical (y-axis) component of the vector.

Similarly, in three dimensions, a vector is represented as: v = ⟨vx,vy,vz⟩, vz is the component along the z-axis.

Describing Motion

When an object moves, its position changes with time, and this change can be described using a position vector, typically denoted as r(t). This vector depends on time and provides the position of the object at any given instant. The position vector can be broken into its components as:

r(t) = ⟨x(t),y(t),z(t)⟩

Here, x(t),y(t),z(t) are the functions of time representing the object’s position in each direction.

Velocity Vector and Acceleration Vector

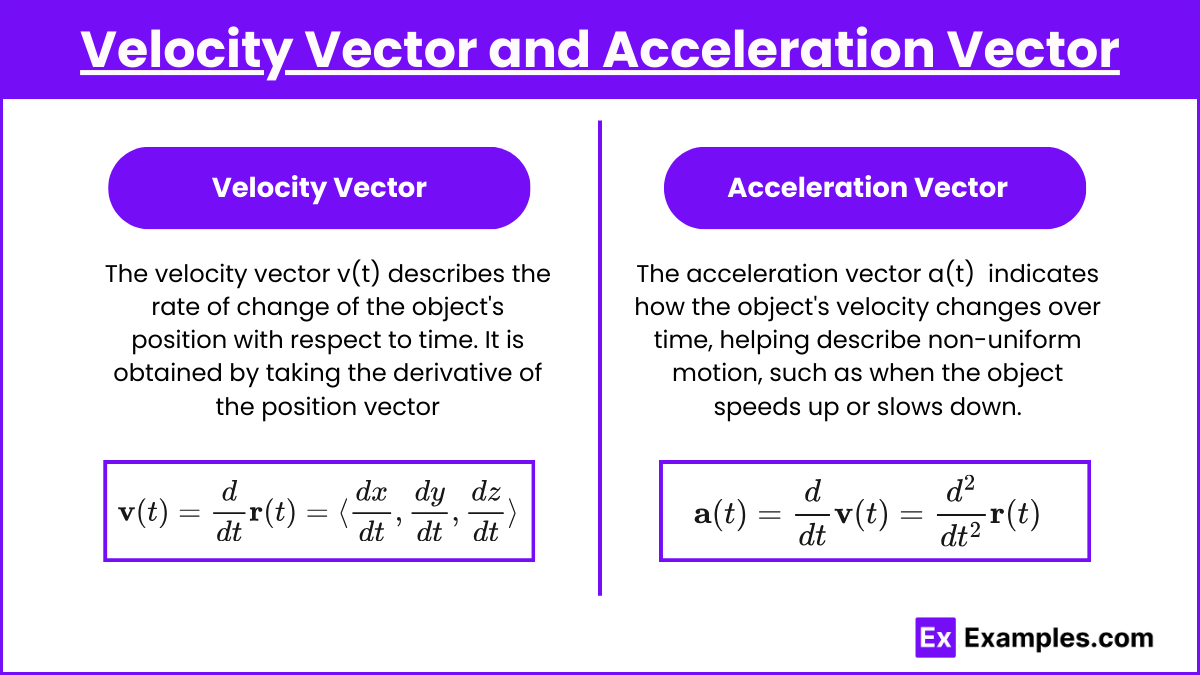
Velocity Vector

The velocity vector v(t) describes the rate of change of the object’s position with respect to time. It is obtained by taking the derivative of the position vector:

$\mathbf{v}(t) = \frac{d}{dt} \mathbf{r}(t) = \langle \frac{dx}{dt}, \frac{dy}{dt}, \frac{dz}{dt} \rangle$

The magnitude of the velocity vector gives the speed of the object.

Acceleration Vector

The acceleration vector a(t) represents the rate of change of the velocity vector:

$\mathbf{a}(t) = \frac{d}{dt} \mathbf{v}(t) = \frac{d^2}{dt^2} \mathbf{r}(t)$

This vector indicates how the object’s velocity changes over time, helping describe non-uniform motion, such as when the object speeds up or slows down.

Magnitude and Direction

The magnitude (or length) of a vector v = ⟨vx,vy,vz⟩ can be calculated using the Pythagorean theorem:

$|\mathbf{v}| = \sqrt{v_x^2 + v_y^2 + v_z^2}$

The direction of the vector is given by the angle(s) it forms with the coordinate axes, and in two dimensions, it can be determined by:

$\theta = \tan^{-1}\left(\frac{v_y}{v_x}\right)$

Applications in Describing Motion

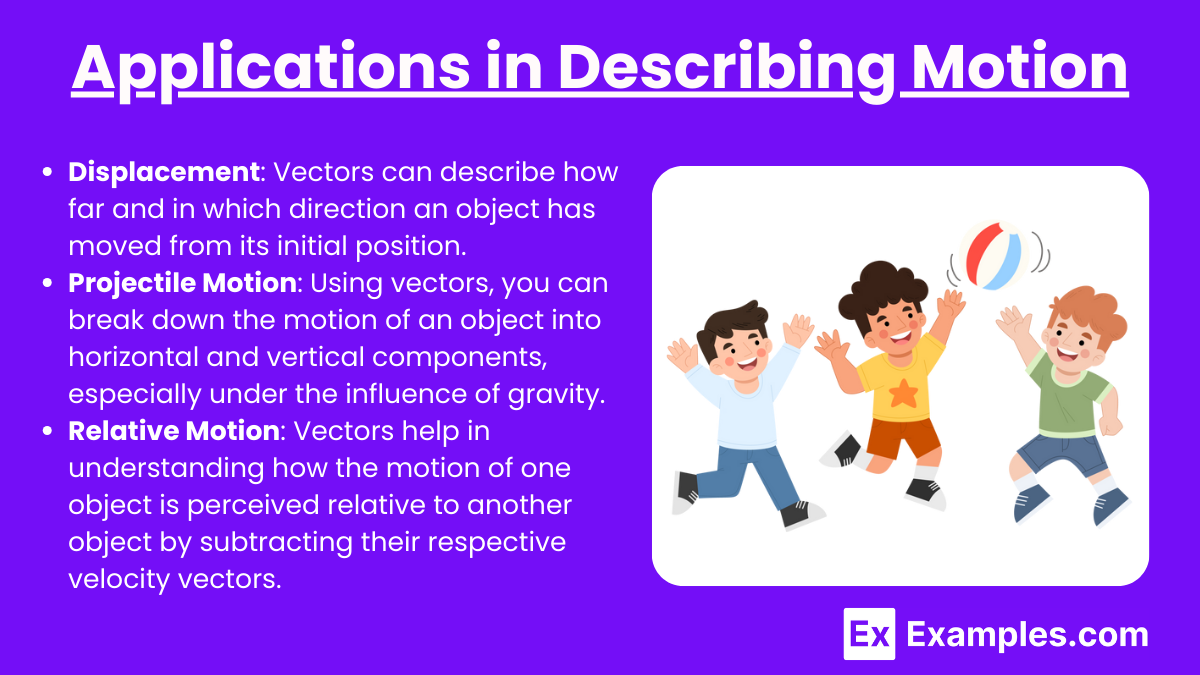
Displacement: Vectors can describe how far and in which direction an object has moved from its initial position.
Projectile Motion: Using vectors, you can break down the motion of an object into horizontal and vertical components, especially under the influence of gravity.
Relative Motion: Vectors help in understanding how the motion of one object is perceived relative to another object by subtracting their respective velocity vectors.

Examples

Example 1: Projectile Motion

When a ball is thrown, its motion can be described using a vector with both horizontal and vertical components. The initial velocity vector has a certain magnitude and direction. As the ball moves through the air, the horizontal velocity remains constant (assuming no air resistance), but the vertical component changes due to gravity. The overall position of the ball at any point in time can be described using a position vector, with time determining the horizontal and vertical displacement.

Example 2: Car Moving on a Road

Suppose a car is traveling along a curved road. The car’s position at any point in time can be described using a vector that points from a reference point (like the starting point) to the car’s current position. The velocity vector at each point describes how fast the car is moving and in which direction, and the acceleration vector tells us if the car is speeding up, slowing down, or changing direction.

Example 3: Airplane in Flight

An airplane moving through the air can have its motion broken into vectors. For example, its velocity vector can be split into components that represent its speed in the northward and eastward directions. If the wind is blowing, another velocity vector is added, and the resultant vector gives the true motion of the airplane relative to the ground. The pilot uses vectors to adjust the airplane’s course and maintain the desired direction of travel.

Example 4: Boat Crossing a River

A boat crossing a river must account for the current of the river. The boat has a velocity vector that represents its speed and direction across the water, and the current has its own velocity vector. To determine the boat’s actual path across the river, the two vectors are added, and the resulting vector shows the boat’s true direction and speed relative to the riverbank.

Example 5: Spacecraft Orbiting a Planet

A spacecraft in orbit around a planet has a position vector pointing from the center of the planet to the spacecraft’s location. The velocity vector describes its speed and direction as it moves around the planet. When adjustments are made to the spacecraft’s orbit, such as firing thrusters, this introduces an acceleration vector, which changes its velocity and can alter the orbit.

Multiple Choice Questions

Question 1

An object moves in the plane and its position at time t is given by the vector r(t)=⟨3t,4t⟩. What is the magnitude of the object’s velocity vector?

A) 5t
B) 3t
C) 5
D) 7

Answer: C)

Explanation: The velocity vector v(t) is the derivative of the position vector r(t). Since r(t)=⟨3t,4t⟩, its derivative is: $\mathbf{v}(t) = \frac{d}{dt} \mathbf{r}(t) = \langle 3, 4 \rangle$

The magnitude of the velocity vector is given by: $|\mathbf{v}(t)| = \sqrt{3^2 + 4^2} = \sqrt{9 + 16} = \sqrt{25} = 5$

Thus, the magnitude of the velocity is 5, independent of t, and the correct answer is C.

Question 2

An object is moving in a straight line with a constant velocity. Which of the following statements is true about the acceleration vector of the object?

A) The acceleration vector points in the same direction as the velocity vector.
B) The acceleration vector is always zero.
C) The acceleration vector is constant and non-zero.
D) The acceleration vector changes direction over time.

Answer: B)

Explanation: When an object is moving with constant velocity, it means its speed and direction do not change. Since acceleration is the rate of change of velocity, if the velocity is constant, the acceleration must be zero. Hence, the correct answer is B.

Question 3

A car moves along the path described by the position vector r(t) = ⟨2t²,3t⟩. What is the car’s acceleration vector?

A) ⟨4t,3⟩
B) ⟨4,3⟩
C) ⟨4,0⟩
D) ⟨2t,3t⟩

Answer: C)

Explanation: The acceleration vector is the second derivative of the position vector r(t). First, we find the velocity vector by taking the derivative of the position vector:

$\mathbf{v}(t) = \frac{d}{dt} \mathbf{r}(t) = \langle 4t, 3 \rangle$

Next, the acceleration vector is the derivative of the velocity vector: $\mathbf{a}(t) = \frac{d}{dt} \mathbf{v}(t) = \langle 4, 0 \rangle$

Thus, the correct answer is C.