The Binomial Distribution
In AP Statistics, the binomial distribution is essential for analyzing experiments with two possible outcomes: success or failure. It applies to scenarios where a fixed number of independent trials occur, each with the same probability of success. Understanding the binomial distribution helps students calculate probabilities of obtaining a specific number of successes and interpret statistical results in various contexts, such as quality control, survey analysis, and game theory. This foundational knowledge is crucial for success in statistics.
Learning Objectives
By studying the binomial distribution in AP Statistics, you will learn to analyze scenarios with two possible outcomes: success or failure. You will understand how to calculate probabilities of a specific number of successes in fixed, independent trials, and interpret these probabilities in real-world contexts. This knowledge will help you solve problems related to quality control, surveys, and experiments, enhancing your ability to make data-driven decisions and deepen your understanding of statistical concepts.
Definition and Key Concepts
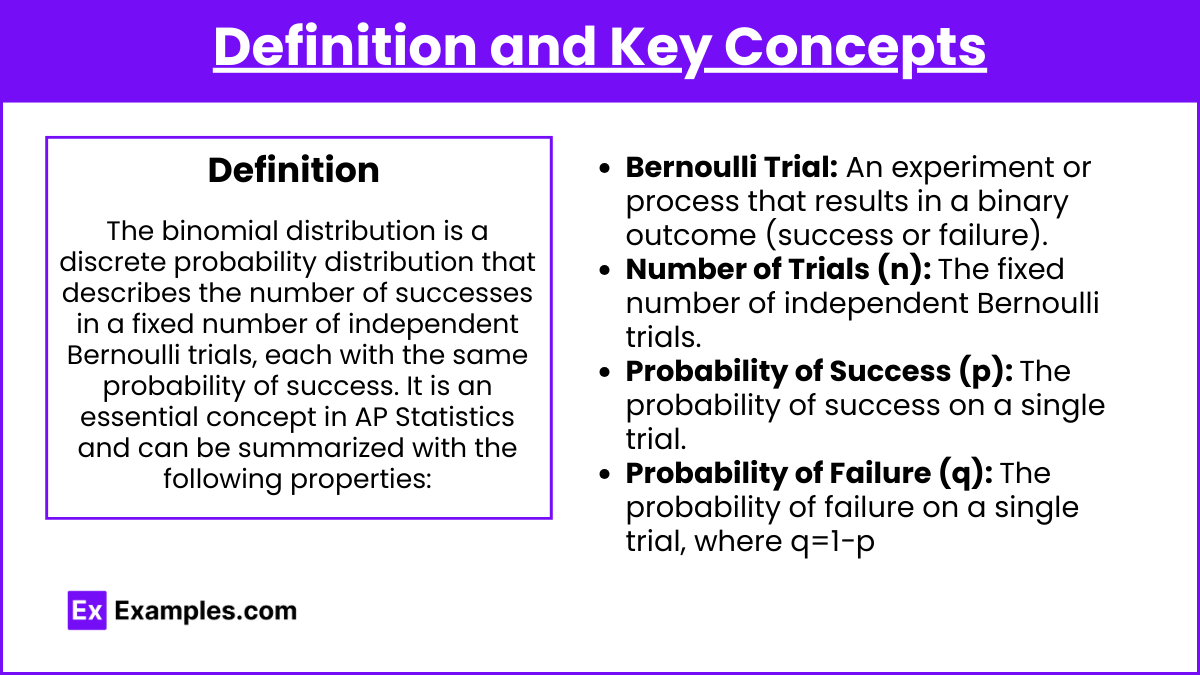
The binomial distribution is a discrete probability distribution that describes the number of successes in a fixed number of independent Bernoulli trials, each with the same probability of success. It is an essential concept in AP Statistics and can be summarized with the following properties:
- Bernoulli Trial: An experiment or process that results in a binary outcome (success or failure).
- Number of Trials (n): The fixed number of independent Bernoulli trials.
- Probability of Success (p): The probability of success on a single trial.
- Probability of Failure (q): The probability of failure on a single trial, where q=1−p
Binomial Probability Formula
The probability of obtaining exactly k successes in n trials is given by the binomial probability formula:
\(P(X = k) = \binom{n}{k} p^k (1 – p)^{n – k}\)
Where:
- \(\binom{n}{k}\) is the binomial coefficient, calculated as \(\frac{n!}{k!(n – k)!}\)
- p is the probability of success
- (1 – p) is the probability of failure
- n is the number of trials
- k is the number of successes
Mean and Variance of Binomial Distribution
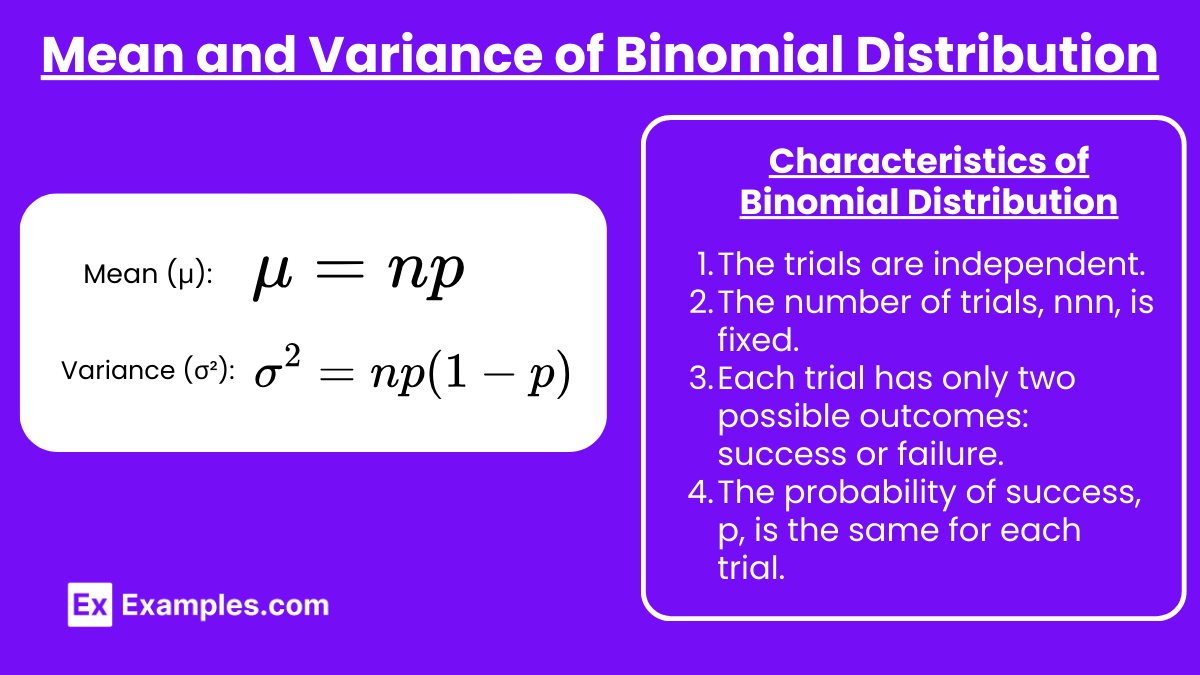
- Mean (μ): \( \mu = np\)
- Variance (σ²): \((\sigma^2 = np(1 – p)\)
Characteristics of Binomial Distribution
- The trials are independent.
- The number of trials, nnn, is fixed.
- Each trial has only two possible outcomes: success or failure.
- The probability of success, ppp, is the same for each trial.
Examples
Example 1: Coin Tossing
Suppose you toss a fair coin 10 times. What is the probability of getting exactly 6 heads?
\(
\begin{aligned}
n &= 10 \
p &= 0.5 \
k &= 6 \
P(X = 6) &= \binom{10}{6} (0.5)^6 (0.5)^4 = \frac{10!}{6!4!} (0.5)^{10} \approx 0.2051
\end{aligned}
\)
Example 2: Quality Control
A factory produces light bulbs with a 2% defect rate. What is the probability that in a sample of 20 bulbs, exactly 1 is defective?
\(
\begin{aligned}
n &= 20 \
p &= 0.02 \
k &= 1 \
P(X = 1) &= \binom{20}{1} (0.02)^1 (0.98)^{19} \approx 0.2702
\end{aligned}
\)
Example 3: Exam Questions
A multiple-choice exam has 15 questions, each with 4 choices. If a student guesses on all questions, what is the probability of getting exactly 5 questions correct?
\(
\begin{aligned}
n &= 15 \
p &= 0.25 \
k &= 5 \
P(X = 5) &= \binom{15}{5} (0.25)^5 (0.75)^{10} \approx 0.2023
\end{aligned}
\)
Example 4: Marketing Survey
In a survey, 40% of respondents prefer brand A. If 8 people are surveyed, what is the probability that exactly 3 prefer brand A?
\(
\begin{aligned}
n &= 8 \
p &= 0.4 \
k &= 3 \
P(X = 3) &= \binom{8}{3} (0.4)^3 (0.6)^5 \approx 0.2787
\end{aligned}
\)
Example 5: Disease Testing
A disease test has a 95% accuracy rate. If 5 people are tested, what is the probability that exactly 4 tests are accurate?
\(
\begin{aligned}
n &= 5 \
p &= 0.95 \
k &= 4 \
P(X = 4) &= \binom{5}{4} (0.95)^4 (0.05)^1 \approx 0.2023
\end{aligned}
\)
Multiple Choice Questions (MCQs)
MCQ 1
If a fair die is rolled 6 times, what is the probability of getting exactly 2 fours?
A. 0.2001
B. 0.0332
C. 0.0322
D. 0.2346
Answer: C
Explanation:
\(
\begin{aligned}
n &= 6 \
p &= \frac{1}{6} \
k &= 2 \
P(X = 2) &= \binom{6}{2} \left(\frac{1}{6}\right)^2 \left(\frac{5}{6}\right)^4 \approx 0.0322
\end{aligned}
\)
MCQ 2
A basketball player makes 70% of their free throws. If they take 10 free throws, what is the probability they make exactly 7?
A. 0.2335
B. 0.2668
C. 0.7000
D. 0.8670
Answer: B
Explanation:
\(
\begin{aligned}
n &= 10 \
p &= 0.7 \
k &= 7 \
P(X = 7) &= \binom{10}{7} (0.7)^7 (0.3)^3 \approx 0.2668
\end{aligned}
\)
MCQ 3
In a game, the probability of winning is 0.2. If you play the game 15 times, what is the probability of winning exactly 4 times?
A. 0.2503
B. 0.1863
C. 0.2051
D. 0.1036
Answer: D
Explanation:
\(
\begin{aligned}
n &= 15 \
p &= 0.2 \
k &= 4 \
P(X = 4) &= \binom{15}{4} (0.2)^4 (0.8)^{11} \approx 0.1036
\end{aligned}
\)