Distributive Property
Numbers can be easily distributed into other numbers in a bracket to create a new equation. This is because of the distributive property of an integer.
1. Distributive Property Template
2. Standard Algorithm Distributive Property
3. Geometry Distributive Property Grade
4. Exploring Distributive Property
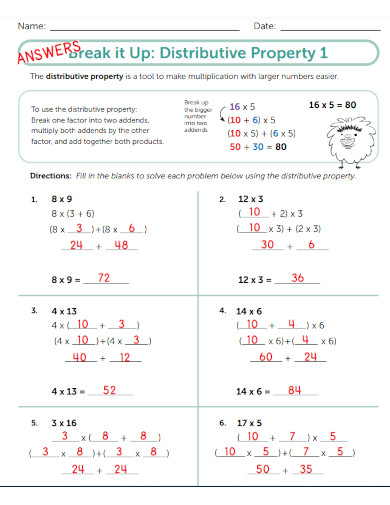
5. Distributive Property Break it Up
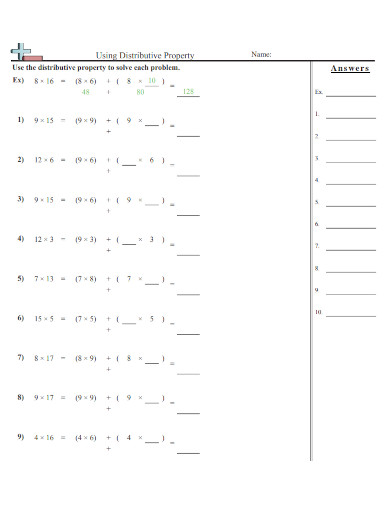
6. Using the Distributive Property
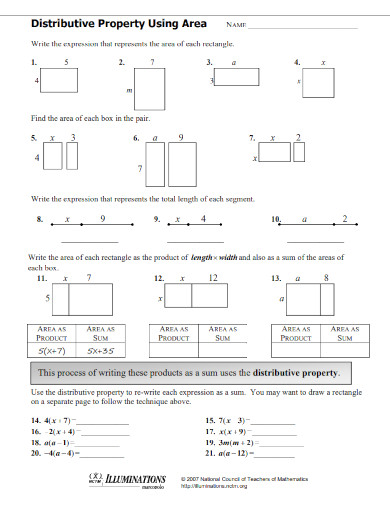
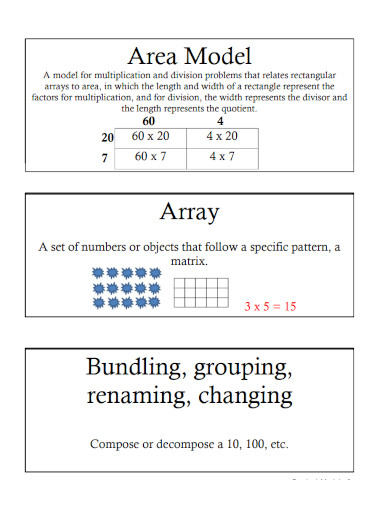
7. Distributive Property Using Area
8. Distributive Property Example
9. Sample Distributive Property
10. Associative Property Distributive Property
11. Students’ Awareness of Distributive Property
12. Students Distributive Property
13. Distributive Property Multiplication
14. Distributive Property Notebook
15. Distributive Property Pattern
16. Distributive Property Notes
17. Determining Distributive Property
18. Representing the Distributive Property
19. Distributive Property and Factoring Worksheet
20. Applying Distributive Property
21. Factoring Using Distributive Property
22. Distributive Property Lesson Review
23. Distributive Property Questions
24. Distributive Property Lesson Plan Template
25. Distributive Property Homework Practice
26. Distributive Property Equivalent Expression
What Is a Distributive Property
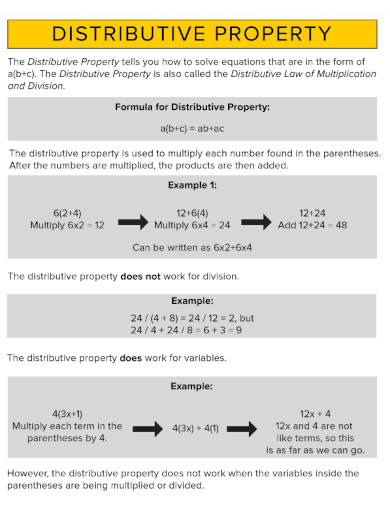
The distributive property is the property of an integer or a real number to be distributed amongst other numbers or equations sequestered inside a closed bracket [()]. If you want to learn more, you may use any of the Distributive Property articles above for a deeper understanding of this property of integer. (Good reads include Students’ Awareness of Distributive Property, Exploring Distributive Property, and Distributive Property Template)
How to Use the Distributive Property of Multiplication
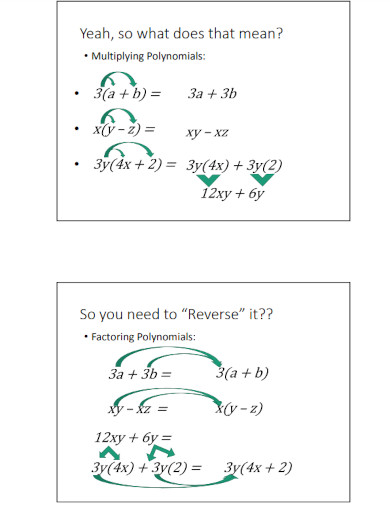
The distributive property of multiplication can come in two different methods, which are called the distributive property of multiplication over subtraction or the distributive property of multiplication over addition. The difference between both types of distributive properties is the operation of the numbers in the brackets. You can easily visualize both types by using this example, x(y+z) = xy + xz (Distributive property of multiplication over addition) and x(y-z) = xy – xz. (the distributive property of multiplication over subtraction) You can use any of the distributive property worksheets, distributive lesson plans, and distributive property lesson reviews on the links above to practice this property.
1.) Write the Whole Equation
Begin by writing the whole equation on a piece of paper or digital note-taking software. This will help you create a visual representation of the whole equation and will help you distribute the number.
2.) Distribute the Number Outside of the Brackets
You must distribute the number from the outside of the brackets to the numbers inside the bracket. For example, if the equation is 0 = 8(2x + 9) then the resulting equation should look like 0 = (8(2x) + 8(9)).
3.) Multiply the Number by the Bracketed Numbers
To distribute the outside number inside the bracketed numbers, you must multiply the said outside number with all the numbers inside the brackets. Using the same example, it would result in the equation of 0 = 16x + 72.
4.) Solve the Equation
After you have finished all the steps above in the equation, you must simplify and solve the new equation. Using the example above, we can solve it by moving 16x to the other side of the equation resulting in -16x = 72. By isolating the unknown number, we can obtain its value by dividing both sides of the equation by -16, resulting in x = 72/-16. Afterward, all that is left is to simplify the equation, which gives us x = (-4.5).
FAQs
What are the practical uses of distributive property?
The distributive property of integers has a lot of real-life applications. This is possible because the distributive property of integers presents itself as a shortcut in mental mathematics. One can use the distributive property of multiplication to help create an easy summation of the income obtained from rent. Another practical use of the distributive property is via the summation of the costs incurred by the creation of the pilot’s uniform.
What are the properties of integers, and how does it relate to the distributive property?
There are four properties of integers, and these are commutative, associative, identity, and distributive properties of the integer. People can apply the four properties of integers to the mathematical operations of addition and multiplication.
What is the difference between distributive and associative property?
The distributive property of an integer is the property that denotes the ability of a specific number to be distributed to a mathematical equation or expression in a closed bracket. The associative property of the integer, on the other hand, expresses that the position of the number in the equation is unimportant and will not affect the final answer.
The distributive property of integers is a subtype of the properties of integers. This property helps us understand the number’s ability to be distributed amongst other equations or numbers in a bracket.