Interest Rate Risk and Return

Preparing for the CFA Exam requires a solid grasp of Interest Rate Risk and Return, a fundamental component of fixed-income analysis. Mastery of interest rate fluctuations, their impact on bond prices, and measures of duration and convexity is essential. This knowledge equips candidates to assess risk, predict returns, and make informed investment decisions.

Learning Objective

In studying “Interest Rate Risk and Return” for the CFA Exam, you should learn to analyze the impact of interest rate fluctuations on fixed-income securities, focusing on concepts such as duration, convexity, and yield curve shifts. Understand how interest rate changes influence bond prices, returns, and portfolio performance. Evaluate the use of duration and convexity as tools for measuring and managing interest rate risk, and explore how these metrics apply in various market environments. Additionally, learn to interpret risk and return metrics, assess interest rate risk strategies, and apply these concepts to CFA-level problem-solving and portfolio management scenarios.

Understanding Interest Rate Risk in Fixed-Income Securities

Interest rate risk is the risk that changes in market interest rates will affect the value of fixed-income securities, such as bonds. This risk arises because bond prices and interest rates have an inverse relationship: as interest rates increase, bond prices tend to decrease, and vice versa. This is a core concept in fixed-income investing, as fluctuations in interest rates can lead to substantial changes in the value of bonds and overall portfolio performance.

The sensitivity of a bond’s price to changes in interest rates depends on several factors:

1. Bond Maturity: Bonds with longer maturities are more sensitive to interest rate changes than those with shorter maturities. This is because a longer time horizon exposes the bond to greater variability in rates over its life, impacting its discounted cash flows more significantly.
2. Coupon Rate: Bonds with lower coupon rates are generally more sensitive to interest rate changes compared to high-coupon bonds. This is because the cash flows are more heavily weighted towards the bond’s maturity date, making the bond’s price more vulnerable to rate changes.
3. Yield to Maturity (YTM): The yield to maturity reflects the total return anticipated on a bond if held until it matures. Higher YTM can reduce a bond’s sensitivity to interest rate changes, whereas lower YTM increases sensitivity.

Understanding these factors allows investors to assess the level of interest rate risk within their fixed-income portfolios and take steps to manage this risk. By selecting bonds with appropriate maturities and coupon rates, as well as utilizing hedging techniques, investors can better align their portfolios with expected changes in interest rates.

Duration and Convexity as Measures of Interest Rate Risk

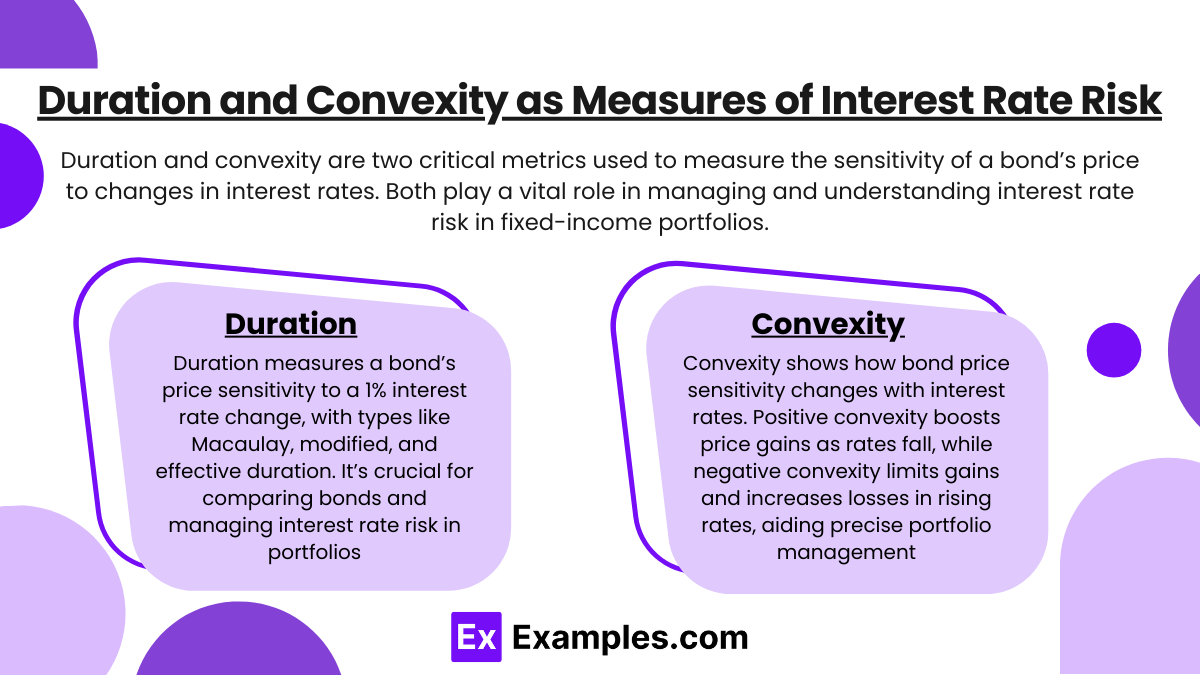
Duration and convexity are two critical metrics used to measure the sensitivity of a bond’s price to changes in interest rates. Both play a vital role in managing and understanding interest rate risk in fixed-income portfolios. Here’s a detailed look at each:

1. Duration:

• Definition: Duration is a measure of the weighted average time until a bond’s cash flows (coupon and principal payments) are received. It reflects how much a bond’s price is expected to change with a 1% change in interest rates.
• Types of Duration:
  • Macaulay Duration: It calculates the time-weighted present value of a bond’s cash flows. Though more academic, it’s foundational and serves as a basis for other duration measures.
  • Modified Duration: Modified duration adjusts Macaulay duration to measure a bond’s price sensitivity more directly to yield changes, estimating the percentage price change for a 1% yield movement.
  • Effective Duration: Effective duration is used for bonds with embedded options (e.g., callable or putable bonds) to account for interest rate changes’ effects on bond features.
• Use: Duration is particularly useful in comparing bonds with different maturities, coupons, and structures, as it allows investors to estimate price sensitivity and adjust portfolios according to their interest rate outlook.

2. Convexity:

• Definition: Convexity measures the degree to which duration changes as interest rates change. It provides a second-order approximation of how a bond’s price responds to interest rate changes, accounting for the curvature of the price-yield relationship.
• Positive vs. Negative Convexity:
  • Positive Convexity: Most bonds exhibit positive convexity, meaning their prices increase at an accelerating rate as interest rates decline and decrease at a decelerating rate as rates rise. Higher convexity generally indicates a bond will perform better in volatile interest rate environments.
  • Negative Convexity: Some bonds, such as callable bonds, have negative convexity at certain yield levels because they are less sensitive to interest rate decreases (as issuers might call them). In rising rate environments, these bonds lose value faster.
• Use: Convexity is an essential tool for managing bond portfolios, as it allows for more accurate predictions of bond price changes across large interest rate shifts, beyond what duration alone can provide. By understanding convexity, investors can choose bonds with characteristics that suit their risk tolerance and market outlook.

Why Duration and Convexity Matter Together?

While duration provides a linear estimate of price sensitivity, convexity accounts for the curvature in the price-yield relationship, making it especially valuable when interest rates are expected to move significantly. Combining both measures, investors can obtain a more comprehensive view of how bond prices will likely respond to interest rate changes. This combination is crucial for strategies in portfolio immunization, hedging, and aligning bond selection with anticipated market conditions

Yield Curve Shifts and Their Impact on Bond Portfolios

The yield curve represents the relationship between bond yields and maturities at a given point in time, typically displaying yields on bonds of varying maturities but with similar credit quality. Yield curve shifts, or changes in the shape or level of the yield curve, significantly affect bond prices and portfolio values. Understanding different types of yield curve shifts is crucial for managing bond portfolios, as each shift can impact bonds differently based on their maturity, coupon, and other characteristics.

1. Types of Yield Curve Shifts

• Parallel Shifts: In a parallel shift, all yields along the curve move up or down by the same amount. For example, if the entire yield curve rises by 50 basis points (0.5%), it’s considered a parallel upward shift. Parallel shifts affect all maturities equally in terms of yield change, but bonds with longer maturities will experience greater price fluctuations due to their higher duration.
• Steepening and Flattening Shifts: When short-term and long-term yields move by different amounts, it results in steepening or flattening of the yield curve:
  • Steepening Shift: This occurs when long-term rates rise more than short-term rates, increasing the difference (or “spread”) between short-term and long-term yields. This typically benefits long-maturity bonds relative to short-term bonds.
  • Flattening Shift: Flattening happens when short-term rates increase more than long-term rates, reducing the spread. A flatter yield curve may signal economic uncertainty and can lead to decreased returns on long-term bonds relative to short-term bonds.
• Curvature Shift: A change in the shape or “bend” of the curve, where mid-term rates move differently from short- and long-term rates. This can impact bonds with intermediate maturities more heavily than others and is often associated with specific economic or market signals.

2. Impact of Yield Curve Shifts on Bond Portfolios

• Portfolio Duration and Convexity Sensitivity: The sensitivity of a bond portfolio to yield curve shifts depends on its duration and convexity. A portfolio with higher duration is more sensitive to parallel shifts, while convexity helps measure how much price sensitivity will change with larger interest rate movements.
• Differential Impact on Bonds by Maturity: Yield curve shifts affect bonds differently based on their maturities. For example, in a steepening yield curve scenario, long-term bonds typically see larger yield changes than short-term bonds, leading to greater price impacts.
• Return Variability Across Bond Types: Bond portfolios with different maturities (short, intermediate, long) and bond types (fixed-rate, floating-rate, inflation-linked) will react differently to shifts. A flattening yield curve, for example, tends to favor shorter-duration bonds, while a steepening curve may benefit longer-duration bonds if investors expect future rate declines.

3. Strategies for Managing Yield Curve Risk

• Barbell and Bullet Strategies: These strategies allocate investments at different points on the yield curve to optimize returns based on yield curve expectations:
  • Barbell Strategy: Involves holding bonds at both the short and long ends of the curve, with fewer holdings in the intermediate range. This can benefit from changes in both ends of the yield curve, such as steepening or flattening.
  • Bullet Strategy: Concentrates investments around a particular maturity, often in the intermediate range, aiming to capitalize on specific yield curve movements that affect these maturities more directly.
• Yield Curve Positioning Based on Economic Outlook: Investors may position their portfolios to benefit from expected yield curve shifts based on economic forecasts. For example, in an environment of expected steepening, long-term bonds may be favored, whereas a flattening curve may lead investors to shorten duration.

Interest Rate Risk Management Strategies

Interest rate risk management is essential for protecting bond portfolios from adverse impacts due to fluctuating interest rates. Several strategies allow investors to manage, mitigate, or hedge interest rate risk effectively. Each approach aligns with specific portfolio objectives, market outlooks, and risk tolerance levels.

1. Duration Matching and Immunization

• Duration Matching: By aligning the portfolio’s duration with a liability’s duration, investors can minimize the impact of interest rate changes on the portfolio’s value relative to the liability. This is particularly useful for institutional investors with fixed obligations, such as pension funds or insurance companies, as it helps ensure that asset values align with liabilities even when interest rates shift.
• Immunization: Immunization extends the concept of duration matching by creating a portfolio that is insensitive to small interest rate changes. It uses a combination of bonds and/or strategies to match the duration and convexity of assets and liabilities, creating a balanced response to rate fluctuations. Immunization works well for portfolios with defined future cash flow needs, as it aims to lock in a specific return.

2. Hedging with Derivatives

• Interest Rate Swaps: In an interest rate swap, one party exchanges a fixed rate for a floating rate (or vice versa) to reduce exposure to interest rate fluctuations. Fixed-rate borrowers can swap to a floating rate to hedge against declining rates, while floating-rate borrowers can secure a fixed rate to hedge against rising rates.
• Futures and Forwards: Investors can use interest rate futures or forward contracts to lock in future rates or hedge against anticipated rate changes. Futures contracts on Treasury bonds or Eurodollar futures are commonly used to manage interest rate exposure.
• Options (Caps, Floors, and Swaptions): Options provide flexible hedging solutions:
  • Caps and Floors: Caps limit the maximum interest rate (for floating-rate investments), while floors provide a minimum rate. These are useful for portfolios exposed to rate volatility.
  • Swaptions: Swaptions give investors the right (but not the obligation) to enter into an interest rate swap in the future, allowing them to respond to anticipated rate movements without committing immediately.

3. Barbell, Ladder, and Bullet Strategies

• Barbell Strategy: This approach involves investing in bonds at both the short and long ends of the maturity spectrum, with fewer intermediate bonds. A barbell strategy allows flexibility, as short-term bonds provide liquidity, while long-term bonds capture higher yields. It can benefit from both rising and falling rates, depending on adjustments in portfolio positioning.
• Ladder Strategy: In a ladder strategy, bonds are distributed evenly across multiple maturities. As bonds mature, they are reinvested at prevailing rates, creating a continuous reinvestment process that helps mitigate interest rate risk over time. This strategy balances risk by spreading exposure across maturities, making it less vulnerable to interest rate shifts.
• Bullet Strategy: This strategy focuses investments around a single maturity point. It is suitable for portfolios with specific future cash flow needs, such as liabilities due at a certain time. Bullet strategies are sensitive to yield curve shifts, especially if concentrated in intermediate durations, making them more effective in stable or predictable interest rate environments.

4. Active Portfolio Management Based on Yield Curve Positioning

• Anticipating Yield Curve Shifts: Investors can position their portfolios to benefit from expected yield curve movements, such as steepening, flattening, or parallel shifts. Active management strategies often involve adjusting the portfolio’s duration, selecting bonds with specific maturities, or even switching between fixed and floating-rate instruments.
• Sector and Quality Allocation Adjustments: Active managers may shift allocations between high-quality government bonds, corporate bonds, or other fixed-income sectors to capitalize on yield curve expectations. For example, they might increase exposure to shorter-term corporate bonds in a flattening yield curve environment.

The Importance of Interest Rate Risk Management Strategies

By using these strategies, investors can protect their portfolios from unexpected interest rate fluctuations, aligning risk exposure with their financial goals and market outlook. For CFA candidates, mastering these approaches is critical, as they form the basis for making strategic investment decisions, optimizing bond portfolio performance, and understanding complex hedging and immunization techniques

Examples

Example 1

A bond with a 10-year maturity and a 5% coupon rate is priced at $1,000. If interest rates rise to 6%, the bond’s price falls to $920. The investor now faces a capital loss due to the interest rate increase. This example demonstrates the inverse relationship between interest rates and bond prices: as rates go up, bond prices decrease, leading to a negative return on the investment.

Example 2

An investor holds a portfolio with a duration of 8 years. If interest rates increase by 1%, the portfolio’s value is expected to decline by approximately 8%. This is because duration estimates the price sensitivity of a bond to interest rate changes. Longer-duration portfolios are more sensitive to interest rate fluctuations, which can amplify both gains and losses based on rate movements.

Example 3

A pension fund with a future liability of $10 million aims to immunize its portfolio against interest rate risk. By matching the duration of its assets with the duration of its liabilities, the fund ensures that changes in interest rates will have a balanced effect on both assets and liabilities. This immunization strategy minimizes the fund’s exposure to interest rate risk, helping it meet its future obligations regardless of interest rate movements.

Example 4

An investor uses an interest rate swap to convert their floating-rate debt into fixed-rate debt. Initially paying LIBOR plus 2% on a $1 million loan, they enter into a swap to pay a fixed rate of 3.5% instead. This swap hedges against rising interest rates, which would increase their floating payments, by locking in a fixed payment structure. If rates rise, the investor benefits by avoiding higher costs; if rates fall, they miss out on potential savings but maintain predictable payments.

Example 5

A bond portfolio manager expects a steepening yield curve and employs a barbell strategy, investing in short-term bonds for flexibility and long-term bonds for higher yields. As long-term rates rise more than short-term rates, the value of the long-term bonds declines, but the short-term bonds are less affected. This diversified approach allows the manager to benefit from the yield curve shift, balancing risk and return according to their market outlook

Practice Questions

Question 1

What is the primary relationship between interest rates and bond prices?

A) Bond prices increase as interest rates increase.
B) Bond prices decrease as interest rates increase.
C) Bond prices remain unaffected by interest rate changes.
D) Bond prices increase only when the bond is close to maturity.

Answer: B) Bond prices decrease as interest rates increase.

Explanation: There is an inverse relationship between bond prices and interest rates. When interest rates increase, the present value of a bond’s future cash flows declines, leading to a decrease in the bond’s price. Conversely, if interest rates fall, bond prices rise. This relationship is fundamental to understanding interest rate risk in bond investing.

Question 2

Which of the following best describes the purpose of duration in interest rate risk management?

A) To estimate the bond’s yield over its lifetime.
B) To measure a bond’s sensitivity to interest rate changes.
C) To determine the default risk of the bond.
D) To assess the bond’s liquidity in the market.

Answer: B) To measure a bond’s sensitivity to interest rate changes.

Explanation: Duration measures how sensitive a bond’s price is to changes in interest rates. A higher duration indicates greater sensitivity, meaning the bond’s price will fluctuate more with interest rate changes. Duration is a key tool in managing and assessing interest rate risk in fixed-income portfolios.

Question 3

If a portfolio manager expects a steepening of the yield curve, which strategy is most likely to benefit the portfolio?

A) Ladder strategy with evenly distributed maturities.
B) Barbell strategy, investing in both short-term and long-term bonds.
C) Bullet strategy, concentrating investments around a single maturity date.
D) Holding only short-term bonds to minimize interest rate risk.

Answer: B) Barbell strategy, investing in both short-term and long-term bonds.

Explanation: A barbell strategy involves investing in both short-term and long-term bonds, which can benefit from a steepening yield curve, as short-term bonds provide flexibility while long-term bonds capture higher yields. This strategy allows the portfolio to be positioned for expected changes in the yield curve shape, offering potential returns in a steepening environment.