Pricing and Valuation of Futures Contracts are fundamental concepts in derivatives that enable investors to determine fair market value and manage risk. The pricing of futures contracts relies on the cost of carry model, which adjusts the spot price based on financing costs, storage costs, income yields, and convenience yields. Understanding these elements is crucial for analyzing market conditions like contango and backwardation, where futures prices differ from spot prices. Additionally, mark-to-market valuation and no-arbitrage principles ensure futures prices align with real-time market dynamics, supporting effective investment strategies.

Free CFA Practice Test

Learning Objectives

In studying “Pricing and Valuation of Futures Contracts” for the CFA exam, you should learn to understand the cost of carry model, including financing, storage, income yield, and convenience yield factors, to calculate futures prices accurately. Analyze market conditions such as contango and backwardation and evaluate their impact on futures pricing. Explore the no-arbitrage principle in identifying and exploiting arbitrage opportunities. Familiarize yourself with mark-to-market valuation, the daily settlement process, and how these affect margin requirements and contract value adjustments. Apply these concepts to assess fair value and interpret pricing dynamics in futures market scenarios.

1. Pricing of Futures Contracts

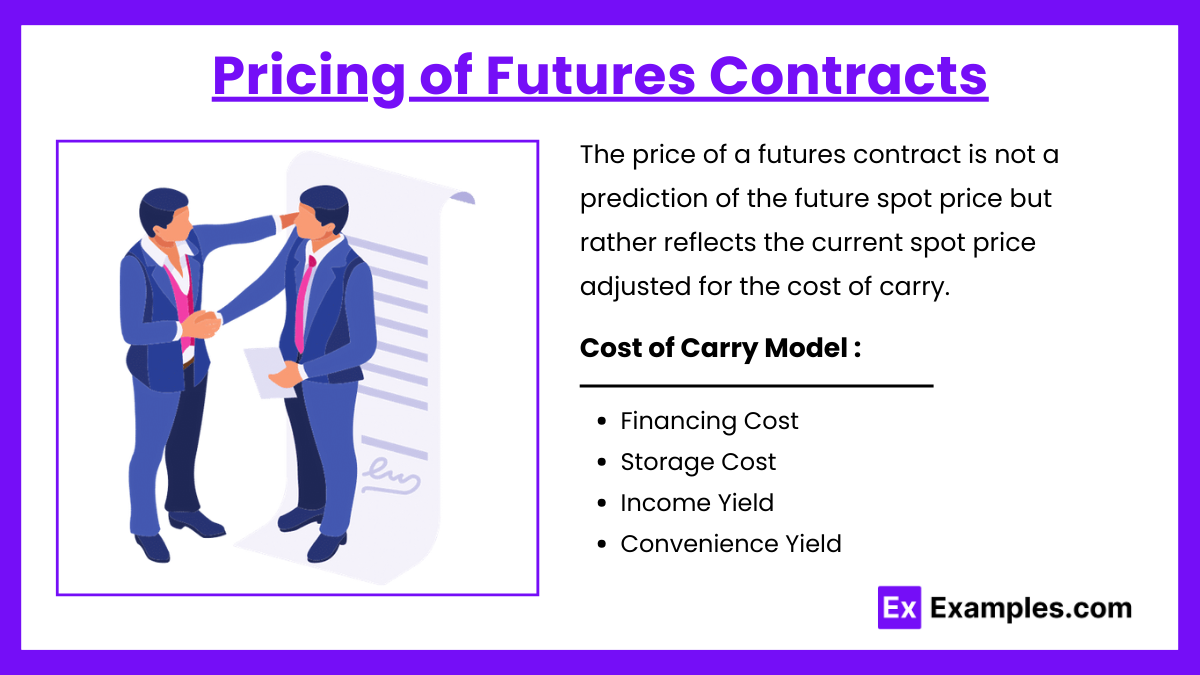
The price of a futures contract is not a prediction of the future spot price but rather reflects the current spot price adjusted for the cost of carry. The formula for calculating the fair futures price is derived from this concept.

Cost of Carry Model

The cost of carry model is the foundation for pricing futures. It includes:

Financing Cost: The cost of borrowing funds to purchase the underlying asset, typically represented by the risk-free rate.
Storage Cost: Costs associated with storing physical commodities like oil or metals.
Income Yield: Cash flows generated by the asset, such as dividends for stocks or interest for bonds, which reduce the cost of carry.
Convenience Yield: A benefit unique to commodities, representing the value of holding the physical asset due to potential shortages or production needs.

The cost of carry model for futures pricing is represented by the formula:

Futures Price (F) = Spot Price (S) ×e^(r+s−y) × T

Where:

S = Current spot price of the underlying asset
r = Risk-free interest rate (cost of financing)
s = Storage cost as a percentage of the spot price
y = Income yield as a percentage of the spot price (e.g., dividends)
T = Time to contract expiration (in years)

Key Implications:

If s + r > y, the futures price will be higher than the spot price, indicating contango (upward sloping futures curve).
If y > r + s, the futures price will be lower than the spot price, indicating backwardation (downward sloping futures curve).

Example Calculation:

Suppose the spot price of a commodity is 1,000 doollars, with a risk-free rate of 5%, no storage costs, and an income yield of 1%. For a futures contract expiring in 1 year, the fair futures price would be:

F = 1000 × e^{(0.05−0.01)×1} = 1000 × e^0.04 ≈ 1040.81.

This calculation shows the futures price after factoring in the cost of carry elements.

2. No-Arbitrage Condition in Futures Pricing

The no-arbitrage principle ensures that the futures price does not deviate significantly from its fair value derived from the spot price and the cost of carry. If the futures price were too high or too low, arbitrageurs could exploit this discrepancy to earn a risk-free profit, which would quickly bring the price back into alignment.

Arbitrage Example:

If the futures price of a commodity is higher than its no-arbitrage price:

An arbitrageur could buy the commodity in the spot market.
They could simultaneously sell a futures contract at the inflated price.
They would then hold the commodity and deliver it when the futures contract expires, locking in a risk-free profit as the prices converge.

3. Valuation of Futures Contracts

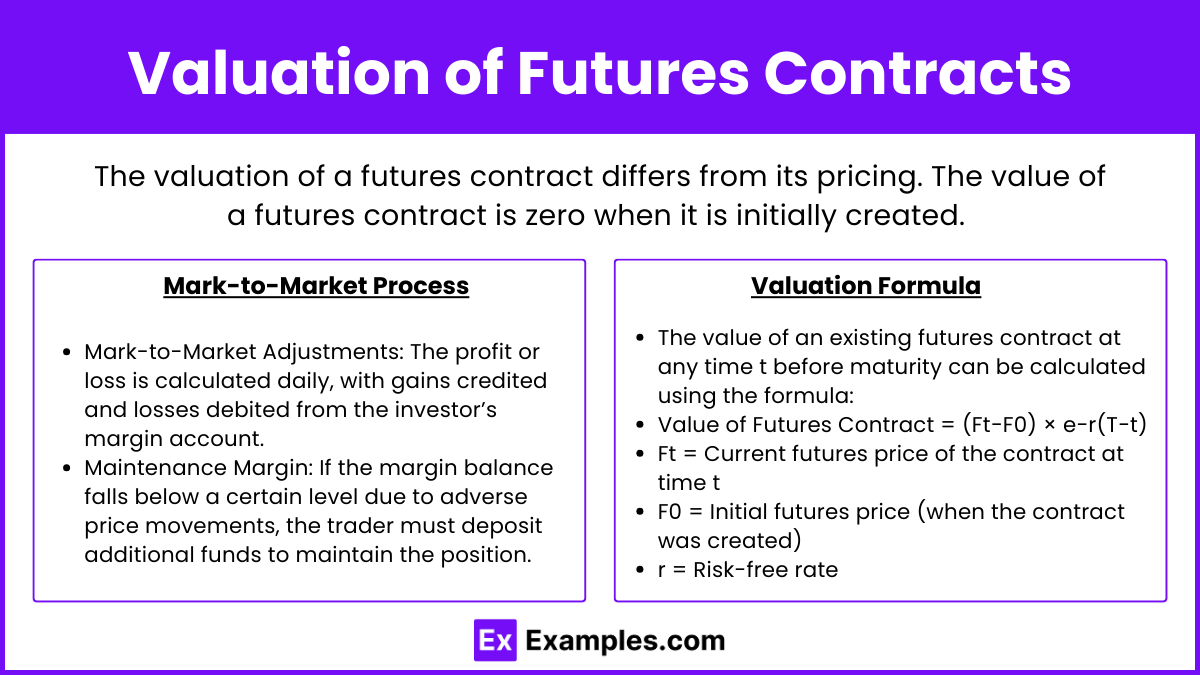
The valuation of a futures contract differs from its pricing. The value of a futures contract is zero when it is initially created. However, as market conditions change, the value of the futures contract to a holder may become positive or negative.

Mark-to-Market Process:

In exchange-traded futures markets, positions are marked-to-market daily, meaning that gains or losses are settled each day based on the contract’s current market price. This daily adjustment affects the margin account, ensuring that the futures contract reflects any price changes in the underlying asset.

Mark-to-Market Adjustments: The profit or loss is calculated daily, with gains credited and losses debited from the investor’s margin account.
Maintenance Margin: If the margin balance falls below a certain level due to adverse price movements, the trader must deposit additional funds to maintain the position.

Valuation Formula:

The value of an existing futures contract at any time t before maturity can be calculated using the formula:

Value of Futures Contract = (F_t−F₀) × e^−r(T−t)

Where:

F_t = Current futures price of the contract at time t
F₀ = Initial futures price (when the contract was created)
r = Risk-free rate
T = Maturity date of the contract
t = Current time

This formula helps determine the unrealized profit or loss on the futures contract at a given point in time before expiration.

4. Practical Applications

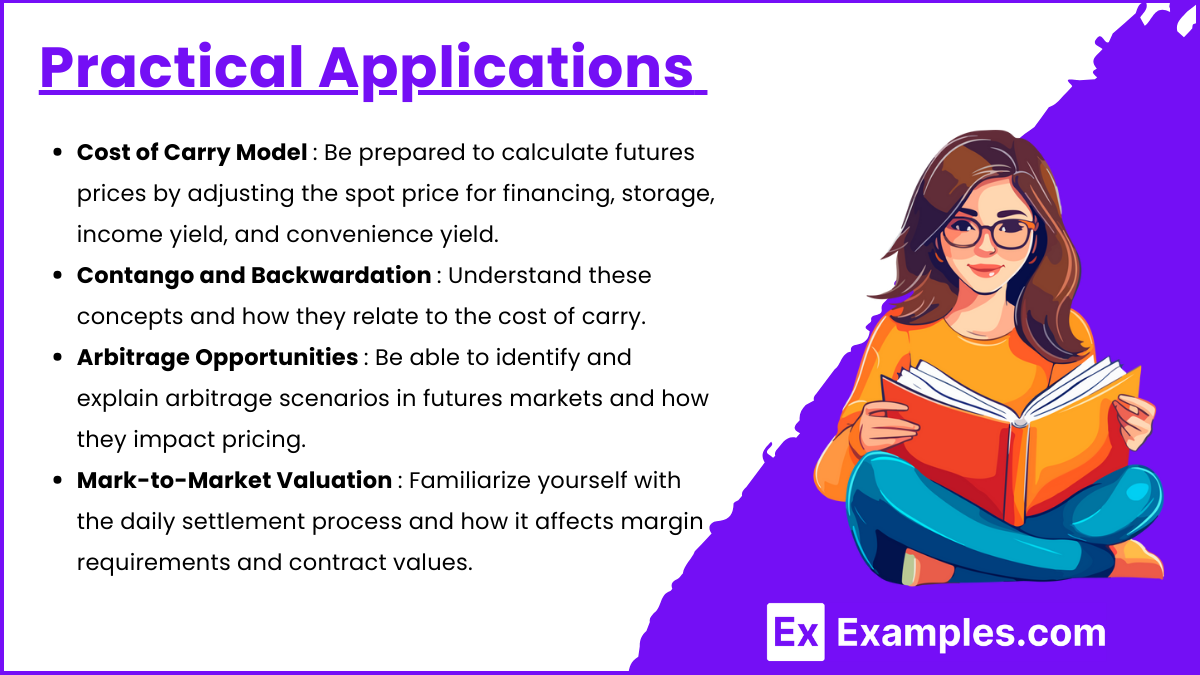
Cost of Carry Model: Be prepared to calculate futures prices by adjusting the spot price for financing, storage, income yield, and convenience yield.
Contango and Backwardation: Understand these concepts and how they relate to the cost of carry.
Arbitrage Opportunities: Be able to identify and explain arbitrage scenarios in futures markets and how they impact pricing.
Mark-to-Market Valuation: Familiarize yourself with the daily settlement process and how it affects margin requirements and contract values.
Interest Rate Parity and Currency Futures: Familiarize yourself with the interest rate parity model to calculate fair futures prices for currency contracts and recognize arbitrage opportunities when parity conditions are violated.
Hedging with Futures: Be able to explain and calculate how futures contracts are used to hedge against price movements in underlying assets.

Examples

Example 1: Cost of Carry in Commodity Futures

Consider a wheat futures contract with a current spot price of $500 per ton. The risk-free rate is 4% annually, storage costs amount to 1% of the spot price, and there are no cash flows or convenience yields. Using the cost of carry model, the futures price for a one-year contract would be calculated as:

F = 500 × e^(0.04+0.01) × 1 ≈ 525.63

This price reflects the cost of financing and storage over the year. Understanding this cost adjustment is essential for calculating fair futures prices, especially for commodities with significant storage requirements.

Example 2: Contango and Backwardation in Oil Markets

Suppose the spot price of oil is 60 dollars per barrel, and the one-year futures price is 65 dollars. This upward sloping futures curve is in contango, where the futures price exceeds the spot price. This often happens due to high storage costs or expectations of higher prices in the future. Conversely, in backwardation, futures prices are lower than the spot price, often driven by a high convenience yield for holding the physical asset, such as when immediate availability is valuable. Mastery of these concepts helps in understanding futures pricing dynamics and market conditions.

Example 3: Interest Rate Parity in Currency Futures

Assume the spot exchange rate for USD/EUR is 1.10, the U.S. risk-free interest rate is 3%, and the European rate is 1%. The one-year futures price can be calculated using interest rate parity:

F = 1.10 × e^{(0.03−0.01)} ≈ 1.1222

This fair value aligns with the interest rate differential, maintaining no-arbitrage conditions. If the futures price deviates from 1.1222, arbitrageurs could exploit the difference by borrowing in one currency, converting to the other, and investing at the respective interest rate, forcing prices back into alignment.

Example 4: Arbitrage in Stock Index Futures

Assume the S&P 500 index is at 4,000, with an annual dividend yield of 2% and a risk-free rate of 5%. The theoretical price for a one-year S&P 500 futures contract would be calculated by factoring in the cost of carry:

F = 4000 × e^{(0.05−0.02)×1} ≈ 4121.87

If the futures price trades above this level, arbitrageurs could short the futures, buy the underlying stocks, and benefit from the arbitrage. This practice aligns futures prices with their theoretical values, highlighting the importance of arbitrage in futures pricing.

Example 5: Mark-to-Market Valuation in Gold Futures

Suppose an investor holds a gold futures contract initially priced at1,800 dollars per ounce. After a month, the market price of the futures contract rises to 1,850 dollars. This $50 gain is credited to the investor’s margin account as part of the mark-to-market process, where gains or losses are realized daily. This daily settlement mitigates counterparty risk and ensures that futures prices reflect real-time market values. Understanding the mark-to-market process is crucial for managing margin requirements and evaluating the current value of a futures position.

Practice Questions

Question 1

The current spot price of a commodity is $200. The risk-free interest rate is 5% per year, the storage cost is 1% per year, and the asset has no income yield. According to the cost of carry model, what should be the futures price for a one-year contract?

A. $205
B. $206.10
C. $210
D. $211.10

Answer: B. $206.10

Explanation: Using the cost of carry model, the futures price can be calculated by adjusting the spot price for the financing and storage costs over one year. The formula for the futures price is:

F = S × e^(r+s−y) × T

where:

S = 200
r = 0.05 (5% risk-free rate)
s = 0.01 (1% storage cost)
y = 0 (no income yield)
T = 1

Calculating the futures price:

F = 200 × e_{^{(0.05+0.01)×1}} ≈ 200 × e^0.06 ≈ 200 × 1.0618 = 206.10

Thus, the correct answer is B.

Question 2

If the spot price of a commodity is 50 dollars, the futures price for a one-year contract is 52 dollars, and the risk-free rate is 4%, which of the following statements is correct about the market condition?

A. The futures market is in backwardation.
B. The futures market is in contango.
C. There is an arbitrage opportunity to short the futures contract.
D. The cost of carry is negative.

Answer: B. The futures market is in contango.

Explanation: Contango is a market condition where the futures price is higher than the spot price. In this question, the futures price of 52 dollars is higher than the spot price of 50 dollars, suggesting a contango situation. Contango typically occurs when the cost of carry (including factors like storage and financing costs) is positive, leading the futures price to exceed the spot price. There is no evidence of an arbitrage opportunity here, as no information is given about the fair futures price relative to cost of carry; the question simply indicates a higher futures price. Therefore, the correct answer is B.

Question 3

An investor holds a long position in a futures contract for gold, initially priced at 1,800 dollars. By the end of the day, the futures price has dropped to 1,750 dollars. Which of the following describes the impact of the mark-to-market process on the investor’s margin account?

A. The investor’s margin account will be credited $50.
B. The investor’s margin account will be debited $50.
C. The investor’s margin account will be debited $1,750.
D. There is no impact on the investor’s margin account.

Answer: B. The investor’s margin account will be debited $50.

Explanation: The mark-to-market process adjusts the value of the investor’s position daily, based on changes in the futures price. Since the futures price has dropped from $1,800 to $1,750, the investor incurs a loss of $50 per unit. This loss is debited from the investor’s margin account to reflect the current market value of the futures contract. This process ensures that the contract reflects real-time price movements and maintains the account balance to cover potential losses. Thus, the correct answer is B.