Pricing and Valuation of Interest Rate and Other Swaps are essential concepts in finance, as swaps are widely used for risk management, hedging, and enhancing portfolio returns. Understanding the pricing involves setting a fair fixed rate at inception, balancing the present value of expected cash flows from the fixed and floating legs, and using the no-arbitrage principle. Valuation over time depends on market changes, such as interest rate shifts, exchange rate movements, and commodity price fluctuations, which impact swap values. Mastery of these concepts is crucial for informed financial decision-making.

Free CFA Practice Test

Learning Objectives

In studying “Pricing and Valuation of Interest Rate and Other Swaps” for the CFA exam, you should learn to understand the mechanics of swap structures, including interest rate, currency, and commodity swaps. Analyze how the fixed and floating legs are valued using present value calculations and the no-arbitrage principle. Evaluate the impact of changes in market interest rates, currency exchange rates, and commodity prices on swap values. Additionally, explore how these swaps are used for hedging and managing risk, and apply these concepts to accurately assess swap pricing, valuation, and potential arbitrage opportunities in financial markets.

Swaps are derivatives that involve the exchange of cash flows between two parties based on an underlying asset or reference rate. The most common types of swaps are interest rate swaps, currency swaps, and commodity swaps. The pricing and valuation of swaps depend on the present value of expected future cash flows and the no-arbitrage condition, which ensures that swaps are fairly priced to eliminate arbitrage opportunities.

Interest Rate Swaps

An interest rate swap is an agreement between two parties to exchange cash flows based on different interest rate structures, typically a fixed rate for a floating rate. These swaps are used by companies and financial institutions to manage interest rate risk, converting fixed-rate debt to floating, or vice versa.

Key Concepts in Pricing Interest Rate Swaps

Fixed Rate Determination:
- The fixed rate in an interest rate swap (also called the swap rate) is set such that the present value of the fixed-rate cash flows equals the present value of the floating-rate cash flows at inception. This creates a no-arbitrage condition, meaning there is no profit or loss when the swap is initiated.
- The fixed rate is often derived from the yield curve of interest rates, particularly focusing on the rates for instruments with maturities corresponding to the swap’s payment dates.
Floating Rate:
- The floating rate in an interest rate swap typically resets periodically (e.g., every 3 or 6 months) and is commonly tied to a benchmark rate, like LIBOR or SOFR (Secured Overnight Financing Rate).
- Because the floating rate varies over time, the value of the swap can fluctuate as market interest rates change.

Pricing Formula for Interest Rate Swaps

The fixed rate for the swap can be calculated using the following formula:Fixed Rate=∑PV Factors of Fixed Leg1−PV of Floating Leg

Where:

PV of Floating Leg = Present value of the floating-rate cash flows, initially set to 1 at inception.
PV Factors of Fixed Leg = Discount factors based on the yield curve for each payment date.

Example:

Suppose a company wants to enter a one-year interest rate swap with quarterly payments. The current 3-month, 6-month, 9-month, and 12-month rates are used to calculate the discount factors for each period, which are then applied to the fixed rate calculation. The resulting fixed rate ensures no arbitrage, making the swap’s initial value zero

Valuation of Interest Rate Swaps

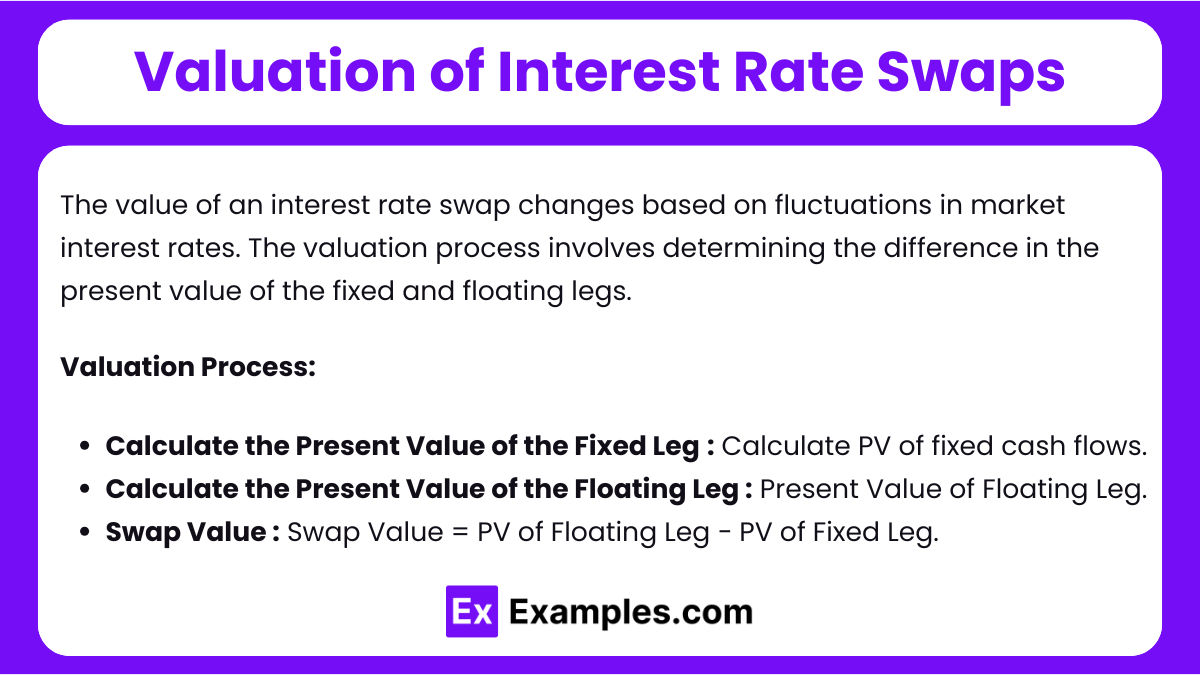
The value of an interest rate swap changes based on fluctuations in market interest rates. The valuation process involves determining the difference in the present value of the fixed and floating legs.

Valuation Process:

Calculate the Present Value of the Fixed Leg: Discount each fixed cash flow at the current market discount rates.
Calculate the Present Value of the Floating Leg: Since the floating rate resets to the current market rate at each payment date, the floating leg is typically valued based on the next payment.
Swap Value: The swap’s value to the fixed-rate payer (or floating-rate receiver) is:

Swap Value = PV of Floating Leg−PV of Fixed Leg

If the result is positive, the fixed-rate payer benefits; if negative, the floating-rate payer benefits. This approach helps evaluate the market value of the swap at any point in time.

Currency Swaps

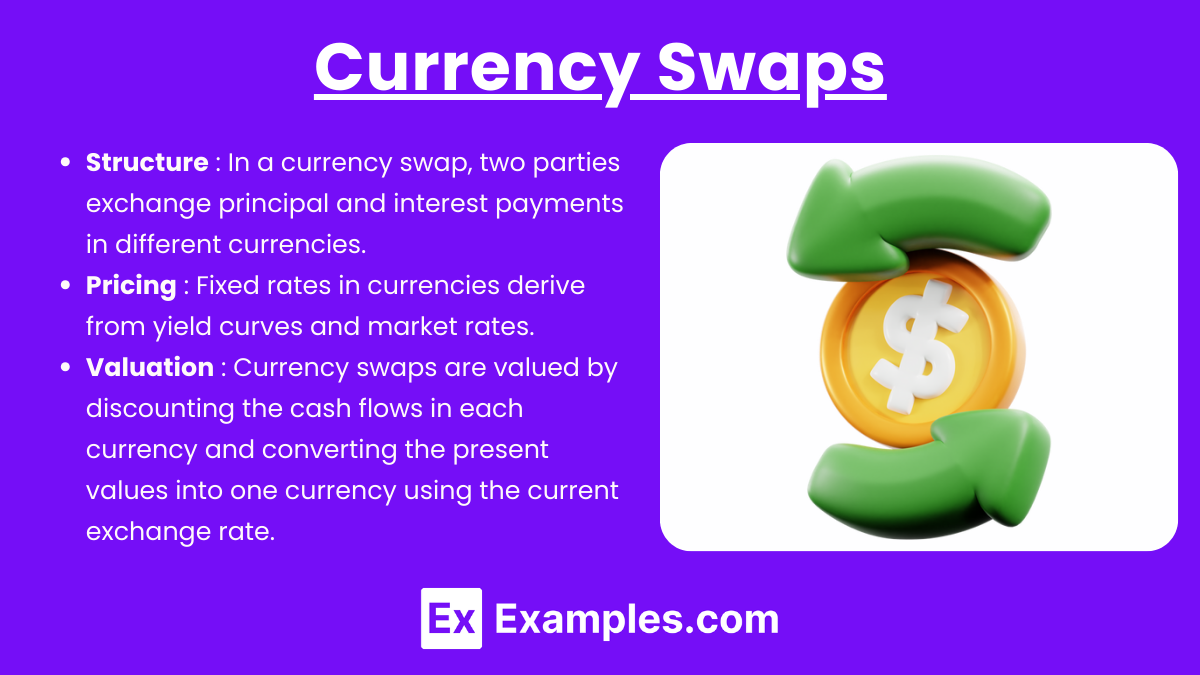
Structure: In a currency swap, two parties exchange principal and interest payments in different currencies. Currency swaps are often used to hedge foreign exchange risk in international investments or loans.
Pricing: The fixed rates in each currency are determined based on the respective yield curves, with the initial exchange rate set at the market rate.
Valuation: Currency swaps are valued by discounting the cash flows in each currency and converting the present values into one currency using the current exchange rate.

Commodity Swaps

Structure: Commodity swaps involve exchanging cash flows based on the price of a commodity, such as oil or natural gas. One party might pay a fixed price for the commodity, while the other pays a floating price based on market prices.
Pricing: The fixed price in a commodity swap is based on forward prices for the commodity, adjusted for the cost of carry.
Valuation: As market prices fluctuate, the value of the swap changes, with the fixed payer or floating payer benefiting depending on price movements in the commodity.

Examples

Example 1: Interest Rate Swap with Fixed and Floating Legs

Suppose a company enters a 5-year interest rate swap, agreeing to pay a 3% fixed rate while receiving a floating rate based on 6-month LIBOR. At inception, the swap rate of 3% is set to make the present value of the fixed and floating legs equal, ensuring a zero value under the no-arbitrage condition. Over time, as LIBOR rates fluctuate, the value of the swap may shift, with the fixed-rate payer benefiting if LIBOR falls and the floating-rate receiver benefiting if LIBOR rises.

Example 2: Valuation of a Currency Swap

Consider a company with dollar-denominated debt that wishes to switch its cash flows to euros due to increased business in Europe. It enters a currency swap, agreeing to exchange USD payments for EUR payments. The pricing of this swap depends on the yield curves in both currencies, with initial principal exchanges based on the spot exchange rate. To value the swap later, the present value of both currency legs is calculated using current market interest rates and the updated exchange rate, which determines gains or losses for each party based on currency fluctuations.

Example 3: Commodity Swap on Oil Prices

An airline company enters a commodity swap to hedge against rising oil prices. In this swap, the airline agrees to pay a fixed price per barrel of oil over the next year while the counterparty pays the floating market price of oil. The fixed rate is set based on the forward curve of oil prices. If market oil prices rise, the airline benefits from the swap; if they fall, the counterparty benefits. The swap’s value fluctuates based on the difference between the fixed and market prices over time.

Example 4: Valuation of an Interest Rate Swap Mid-Contract

A bank enters a 3-year interest rate swap with quarterly payments, paying a fixed rate of 4% and receiving 3-month LIBOR. After one year, with two years remaining, interest rates have risen, making LIBOR higher than the fixed rate. To value the swap, the present value of the remaining fixed payments is compared to the present value of expected floating payments (discounted at current rates). The bank benefits from a positive swap value, as it can receive more from the floating leg due to the rate increase.

Example 5: Zero-Coupon Swap Valuation

A financial institution enters a zero-coupon interest rate swap, where it pays a lump-sum fixed amount at maturity and receives floating rate payments semi-annually. The fixed payment is calculated by discounting the fixed interest rate over the swap’s term. If interest rates rise after inception, the value of the swap increases for the fixed-rate payer, as the present value of the fixed payment falls, whereas the floating payments, tied to higher rates, yield more over time. This swap type is valuable in scenarios where parties prefer lump-sum cash flows over periodic payments.

Practice Questions

Question 1

A company enters a 3-year interest rate swap where it pays a fixed rate of 4% annually and receives a floating rate based on 1-year LIBOR. The notional principal is $1,000,000. After one year, interest rates have risen, and the current 1-year LIBOR is now 5%. Which of the following is true about the value of the swap to the fixed-rate payer?

A. The swap has no value because it is a derivative instrument.
B. The value of the swap is positive for the fixed-rate payer.
C. The value of the swap is negative for the fixed-rate payer.
D. The swap value cannot be determined without knowing future LIBOR rates.

Answer: B. The value of the swap is positive for the fixed-rate payer.

Explanation: In an interest rate swap, the fixed-rate payer benefits when interest rates rise, as they are paying a fixed rate of 4% while receiving a floating rate that is now higher (5%). This increases the value of the swap to the fixed-rate payer since the present value of the expected floating-rate payments they receive is now greater than the value of the fixed payments they must make. Therefore, the correct answer is B.

Question 2

In a currency swap, an investor agrees to exchange 1,000,000 USD for 900,000 EUR at inception and will make annual interest payments in each currency. If the USD interest rate is 3% and the EUR interest rate is 2%, which factor is most important in determining the swap’s value after inception?

A. The credit risk of the counterparty.
B. The prevailing spot exchange rate between USD and EUR.
C. The initial interest rate differential.
D. The notional principal amounts.

Answer: B. The prevailing spot exchange rate between USD and EUR.

Explanation: In a currency swap, the swap’s value after inception is heavily influenced by the prevailing spot exchange rate between the two currencies. Since each party will continue to make interest payments in the currency in which they initially received principal, fluctuations in the exchange rate will impact the value of these cash flows. If, for instance, the USD appreciates relative to the EUR, the USD payments become more valuable in EUR terms, potentially increasing the swap’s value for the USD receiver. Thus, the correct answer is B.

Question 3

A financial institution holds a commodity swap where it pays a fixed price for oil and receives the floating market price of oil. If oil prices are expected to rise significantly in the future, what is the most likely effect on the value of the swap to the fixed-price payer?

A. The value of the swap will increase for the fixed-price payer.
B. The value of the swap will decrease for the fixed-price payer.
C. The swap value will remain unchanged as prices change.
D. The value of the swap will depend on the oil’s convenience yield.

Answer: A. The value of the swap will increase for the fixed-price payer.

Explanation: When oil prices are expected to rise, the floating leg of the swap (based on the market price of oil) becomes more valuable. Since the fixed-price payer will receive these higher floating payments, the value of the swap increases for them. As oil prices increase, the fixed-price payer effectively benefits from paying a lower, fixed cost while receiving payments tied to a higher floating price, enhancing the swap’s value. Therefore, the correct answer is A.