Preparing for the CFA Exam requires a strong grasp of “Valuation & Analysis of Bonds with Embedded Options,” a crucial topic within fixed income analysis. This concept addresses how embedded options, such as call and put features, impact the value and yield of bonds. Understanding these options is essential for assessing the risks and potential returns associated with such bonds. By analyzing how market interest rates and volatility affect the option-adjusted yield, candidates can better evaluate the bond’s price sensitivity. Mastery in this area enables candidates to navigate complex financial instruments, implement strategies to hedge interest rate risk, and enhance investment decisions. This advanced knowledge contributes to achieving a high CFA score.

Free CFA Practice Test

Learning Objectives

In studying “Valuation & Analysis of Bonds with Embedded Options” for the CFA, you should learn to understand the critical principles of bonds with embedded options, such as call or put features. This involves assessing how these options affect bond pricing, yield, and volatility, as well as the bond’s sensitivity to interest rate changes. Understanding embedded options allows you to predict how bonds may respond to interest rate fluctuations and credit events, helping to assess reinvestment risk and prepayment risks. Mastery in this area enables you to apply option-adjusted spreads (OAS) in valuation, refine duration and convexity calculations, and make informed decisions in risk management and portfolio optimization.

Key Concepts of Valuation & Analysis of Bonds with Embedded Options

Understanding the valuation and analysis of bonds with embedded options is crucial for assessing these unique financial instruments. Here’s a breakdown of the key concepts involved:

1. Bond Characteristics with Embedded Options

Definition: Bonds with embedded options contain features that allow either the issuer or the bondholder to take specific actions, like calling or putting the bond.
Types of Options: Common embedded options include call options (issuer can redeem early), put options (holder can sell back to issuer), and convertible options (convert bonds into stock).
Impact on Yield: These options affect the bond’s yield and market behavior, making valuation complex.

2. Option-Adjusted Spread (OAS)

Purpose: OAS adjusts for the embedded option by separating it from the bond’s interest rate spread over the benchmark rate, thus isolating credit risk and market risk.
OAS Analysis: Investors use OAS to compare bonds with similar risk levels; a higher OAS generally indicates a better return relative to the risk after factoring in the option’s impact.

3. Valuation Models for Embedded Options

Binomial Tree Model: A widely used method that models the bond’s possible price paths at each point in time, considering the likelihood of option exercise.
Monte Carlo Simulation: Uses repeated random sampling to determine the bond’s value by simulating various interest rate paths and option scenarios.
Dynamic Interest Rate Models: Such models, like the Black-Scholes model, are used to price the option embedded within the bond, accounting for fluctuating interest rates.

4. Interest Rate Volatility Impact

Influence on Bond Prices: Higher interest rate volatility increases the likelihood of option exercise (e.g., call option when rates fall).
Effect on Embedded Option Value: The value of an embedded call option rises with volatility (increasing call risk), whereas a put option’s value also increases with volatility, offering downside protection for investors.

5. Yield Curve and Pricing Sensitivity

Yield Curve Dynamics: Bond prices with embedded options are highly sensitive to changes in the yield curve. For example, a steepening curve can impact call or put probabilities.
Duration and Convexity Adjustments: Bonds with embedded options have lower effective durations and convexities because of the embedded option, leading to non-linear price behavior relative to interest rate changes.

Techniques of Valuation & Analysis of Bonds with Embedded Options

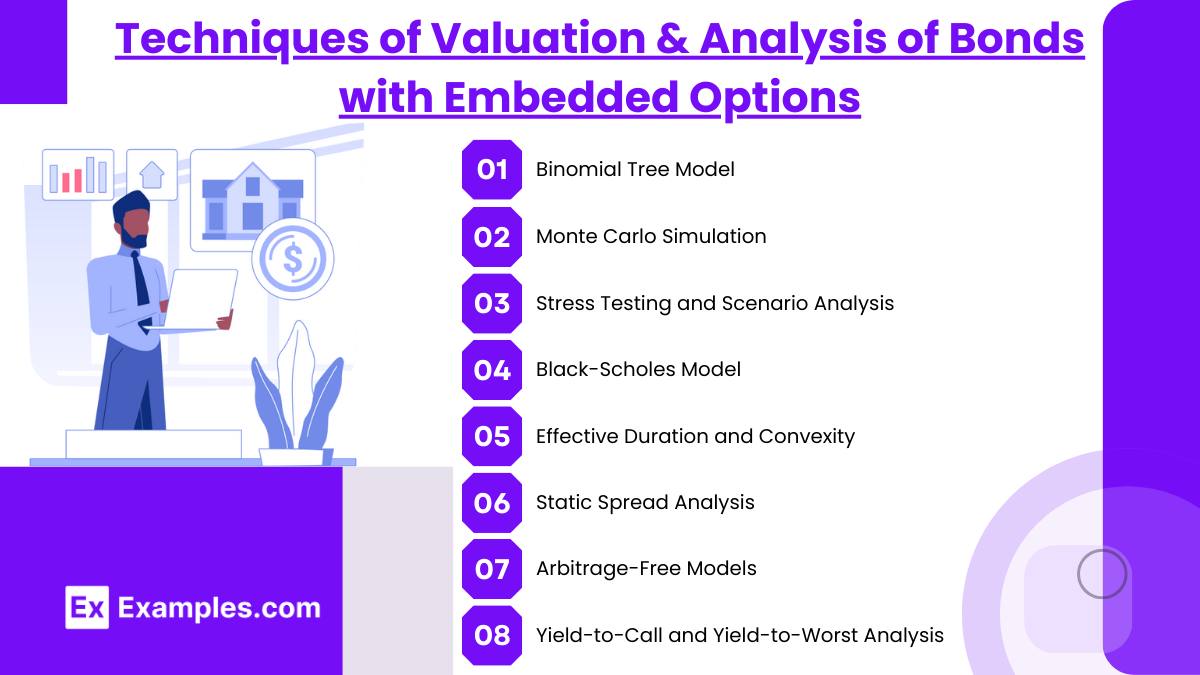
Valuation and analysis of bonds with embedded options require specialized techniques to account for the complex cash flow patterns and option features that may influence the bond’s value. Here are some essential techniques commonly used for these bonds:

1. Binomial Tree Model

Overview: This model uses a binomial lattice to depict potential future interest rate paths, which are essential when valuing bonds with embedded options.
Application: Binomial trees help model the call or put features by valuing the bond across different potential future interest rates.
Advantages: It offers a straightforward, visual method to assess the impact of various interest rates on the embedded option value.

2. Monte Carlo Simulation

Overview: Monte Carlo simulations generate a wide range of random interest rate scenarios to assess potential bond values.
Application: Particularly useful for complex embedded options like those in mortgage-backed securities or convertible bonds.
Advantages: Effective for bonds with multiple embedded options and interest rate paths that don’t fit conventional models.

3. Stress Testing and Scenario Analysis

Overview: Techniques to evaluate the impact of extreme market conditions on financial instruments.
Application: Used in bond valuation and risk management to assess effects of various economic scenarios on bond performance.
Advantages: Enhance risk insight, improve decision-making, increase portfolio resilience, and support regulatory compliance.

4. Black-Scholes Model (for Convertible Bonds)

Overview: The Black-Scholes model is often adapted for valuing the equity option component in convertible bonds.
Application: Used when convertible bonds have embedded options that allow conversion to equity.
Advantages: Helps in calculating the equity option value by modeling the underlying asset price and volatility.

5. Effective Duration and Convexity

Overview: Effective duration and convexity measure the bond’s sensitivity to interest rate changes, taking the embedded options into account.
Application: Allows for adjusting interest rate sensitivity calculations to reflect the presence of callable or putable features.
Advantages: Provides insights into how the bond’s price might change with different interest rates, which is crucial for bonds with embedded options.

6. Static Spread Analysis

Overview: This method assesses the constant spread over a benchmark yield curve that matches the bond’s market price.
Application: Effective in determining a bond’s yield relative to a risk-free yield curve.
Advantages: Gives a straightforward method for yield comparison, which can be informative in assessing relative risk for bonds with embedded options.

7. Arbitrage-Free Models (Ho-Lee, Hull-White Models)

Overview: These are no-arbitrage models that adjust for interest rate volatilities and embedded options to value bonds more accurately.
Application: Useful for valuing callable and putable bonds by assuming an interest rate process consistent with the market.
Advantages: Creates a realistic picture of valuation without assuming arbitrage opportunities, reflecting real-world pricing more closely.

8. Yield-to-Call and Yield-to-Worst Analysis

Overview: Yield-to-call calculates the return if the bond is called at the earliest opportunity, and yield-to-worst gives the lowest possible yield.
Application: Especially relevant for callable bonds, where the call feature might shorten the bond’s life.
Advantages: Helps investors understand the potential downside of an early call and make more informed yield comparisons.

Risk Factors of Valuation & Analysis of Bonds with Embedded Options

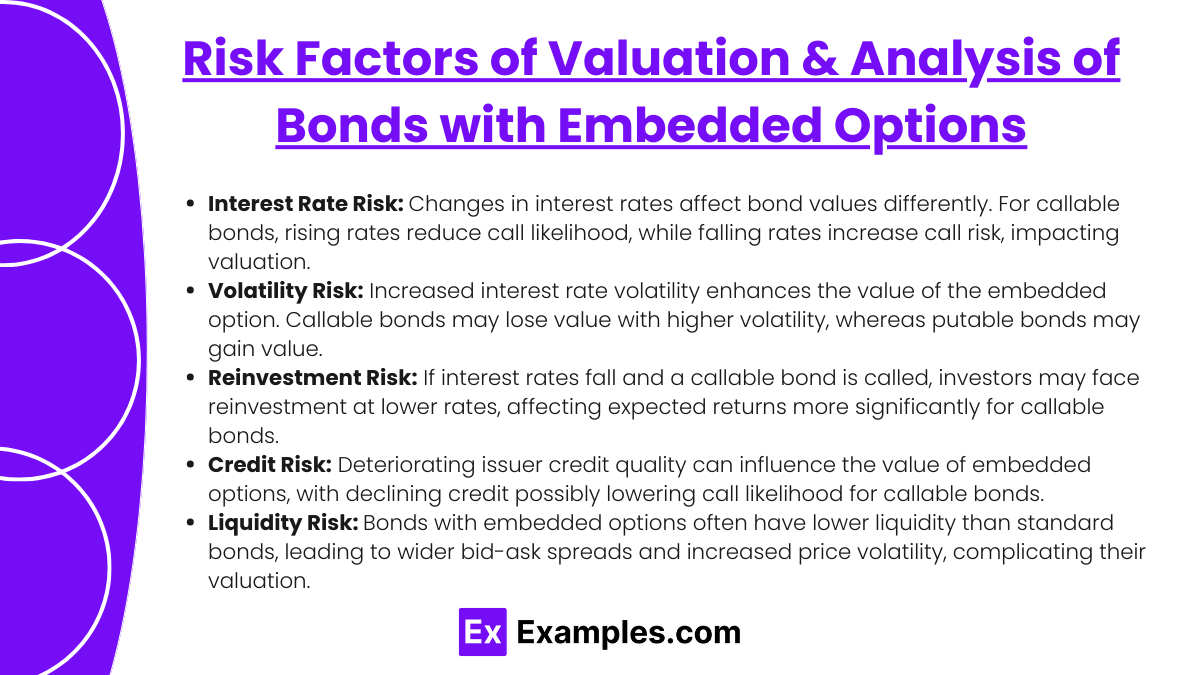
Interest Rate Risk: Changes in interest rates affect the value of bonds, but this impact is more complex for bonds with embedded options. For callable bonds, rising rates may decrease the likelihood of a call, while falling rates increase call risk, affecting the bond’s value.
Volatility Risk: Higher interest rate volatility increases the value of the option embedded in the bond, impacting both callable and putable bonds. Callable bonds may decrease in value with increased volatility, while putable bonds may increase in value.
Reinvestment Risk: For callable bonds, if interest rates drop and the bond is called, investors may have to reinvest at lower rates. This risk impacts expected returns and is more pronounced for bonds with call options.
Credit Risk: Changes in the issuer’s creditworthiness can alter the perceived value of embedded options. For callable bonds, a decline in credit quality may reduce the likelihood of a call, impacting the bond’s overall valuation.
Liquidity Risk: Bonds with embedded options may have lower liquidity than plain-vanilla bonds. Lower liquidity can lead to wider bid-ask spreads and greater price volatility, complicating the valuation process.

Examples

Example 1. Portfolio Optimization for Institutional Investors

When managing large portfolios, institutional investors may analyze bonds with embedded options to optimize their bond selection. By evaluating the callable or putable features within bonds, portfolio managers can better estimate the bond’s effective duration and interest rate sensitivity. This insight allows them to make informed decisions about including such bonds in a diversified portfolio, balancing risk and return based on current market trends and interest rate expectations.

Example 2. Risk Management in Fixed Income

Risk managers often rely on bond valuation with embedded options to identify and mitigate interest rate risk. Bonds with options, such as callable bonds, have a unique risk profile that fluctuates with interest rates. Understanding these dynamics allows risk managers to create hedging strategies that protect the portfolio from adverse price movements. By analyzing option-adjusted spreads (OAS) and other metrics, they can anticipate potential risks and adjust their positions accordingly.

Example 3. Corporate Finance and Debt Structuring

Corporations issuing debt may choose bonds with embedded options as a flexible financing tool. For example, a company might issue callable bonds to capitalize on future refinancing opportunities if interest rates drop. By analyzing the embedded options’ value, financial officers can structure the debt to minimize costs while retaining control over future financing decisions. This approach is beneficial in capital-intensive industries, where flexibility in debt management is essential for operational resilience.

Example 4. Valuation for Investment Analysis

For investors aiming to evaluate complex fixed-income securities, understanding bonds with embedded options is crucial. By calculating option-adjusted duration and yield, investors can assess whether the potential returns justify the risks associated with early repayment or other embedded options. This analysis enables investors to make informed choices based on the bond’s true risk-reward profile, helping them identify undervalued or overvalued securities in the market.

Example 5. Regulatory Compliance and Reporting

Financial institutions, particularly banks, need to account for the embedded options within their bond portfolios to comply with regulatory standards like Basel III. These regulations require banks to maintain sufficient capital to cover risks associated with their assets. Valuing and analyzing bonds with embedded options helps institutions determine the appropriate capital reserves, ensuring they meet regulatory requirements while maintaining profitability.

Practice Questions

Question 1

Which of the following statements is true about the valuation of callable bonds?

A) Callable bonds are more valuable to investors when interest rates rise.
B) Callable bonds tend to have a lower yield compared to non-callable bonds.
C) Callable bonds give the issuer the right to repurchase the bond before maturity.
D) Callable bonds are less affected by interest rate changes than non-callable bonds.

Answer: C) Callable bonds give the issuer the right to repurchase the bond before maturity.

Explanation: Callable bonds contain an embedded option that allows the issuer to “call” or repurchase the bond before its maturity, typically if interest rates decline. This option is advantageous to the issuer because it allows them to refinance the debt at a lower interest rate if conditions improve. However, callable bonds generally carry higher yields to compensate investors for this risk of early redemption. Statement (A) is incorrect because rising interest rates make the call option less valuable; the issuer is more likely to call the bond when interest rates drop, not rise. Statement (B) is incorrect because callable bonds often have higher yields than non-callable bonds due to the additional risk investors face. Finally, statement (D) is also incorrect because callable bonds are, in fact, more sensitive to interest rate changes, particularly when rates fall, as this increases the likelihood of the issuer calling the bond.

Question 2

What is the primary reason investors use option-adjusted spread (OAS) when evaluating bonds with embedded options?

A) To assess the bond’s credit risk
B) To measure the interest rate risk of the bond
C) To evaluate the impact of the embedded options on the bond’s yield
D) To determine the bond’s default probability

Answer: C) To evaluate the impact of the embedded options on the bond’s yield

Explanation: Option-adjusted spread (OAS) is a critical metric used in the valuation of bonds with embedded options, like callable or putable bonds. OAS adjusts the bond’s yield spread over a benchmark rate to reflect the impact of the embedded options. By considering the value of these options, investors can understand the true yield of the bond, accounting for the potential impact of the call or put features. This adjusted spread gives a clearer picture of the bond’s return relative to its risk profile, enabling better comparisons between bonds with and without embedded options. Option (A) is incorrect because OAS is not primarily used to assess credit risk, though credit risk may impact overall yield spreads. Option (B) is incorrect because while OAS can inform interest rate sensitivity, it specifically isolates the effect of options on yield. Option (D) is incorrect because OAS does not directly measure default probability.

Question 3

Which of the following best describes the relationship between interest rates and the likelihood of an issuer calling a bond with an embedded call option?

A) The likelihood increases as interest rates increase.
B) The likelihood decreases as interest rates decrease.
C) The likelihood increases as interest rates decrease.
D) The likelihood remains unaffected by changes in interest rates.

Answer: C) The likelihood increases as interest rates decrease.

Explanation: When interest rates decrease, it becomes more favorable for the issuer to call a bond with an embedded call option. By calling the bond, the issuer can refinance the debt at a lower interest rate, which reduces their cost of borrowing. This characteristic is particularly important for investors to consider, as a callable bond may be repurchased by the issuer at a time when its market value is high due to lower interest rates. Options (A) and (B) are incorrect because higher interest rates make it less attractive for the issuer to call the bond, and option (D) is incorrect because the call likelihood is very much influenced by interest rate changes.