As we can see, the status of our economy is drastically changing, so we must work hard to sustain the daily needs of our family. For us to operate efficiently, we need to know that our work environment is safe, right? A business must value the safety of its employees to avoid spending hospitalization costs among others. Statista revealed that $10 billion was spent on workplace injuries back in 2019. In order to avoid such expenses, you need to observe safety measures for authorities to ensure that your workplace is safe and void of any hazards. In this article, you will learn about safety observation, its importance, and how to conduct a safety observation for the benefit of your employees.
[bb_toc content=”][/bb_toc]
12+ Safety Observation Examples
1. Safety Observation Policy
2. Job Safety Observation
3. Safety Observation Program
4. Safety Observation Report Format
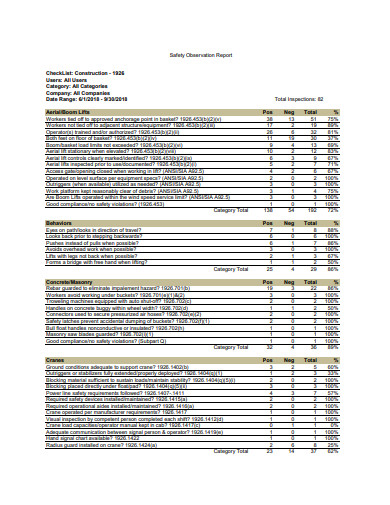
5. Safety Observation Report Sample
6. Sample Safety Observation Report
7. Safety Observation Report in PDF
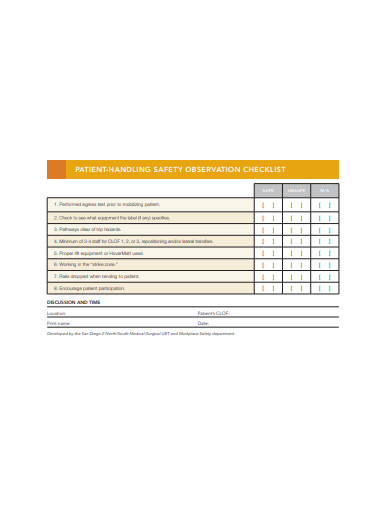
8. Safety Observation Checklist
9. Safety Observation Checklist Format
10. Safety Observation Form
11. Safety Observation Example
12. Safety Observation Audit
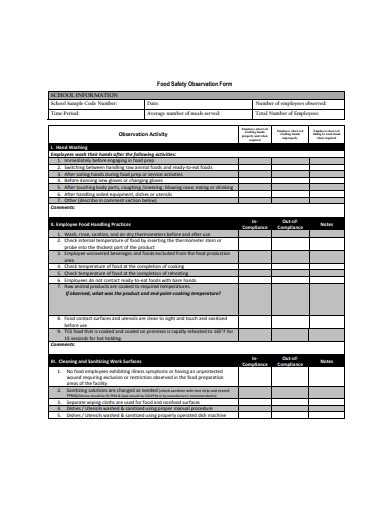
13. Food Safety Observation Form
What Is a Safety Observation?
A safety observation is a procedure in locating dangerous and unsafe surroundings in an environment. It must operate in places with a high number of people going every day, such as classrooms, health facilities, and workplace environment. Safety officers must also be lenient when they do this type of procedure since they are assessing the safety of the people. The International Labor Organization revealed that 2.3 million workers experience work-related accidents. The process of a safety observation must be done carefully to avoid the number of people experiencing accidents and to prevent dangerous situations from happening.
Importance Of a Safety Observation
A safety observation is essential because it is a form of risk management that keeps the safety of individuals in check. It also monitors how individuals behave in their work environment and determine whether such behavior is a risk factor for unsafe conditions. It helps in eliminating the risk of potential accidents from happening and engages individuals to practice safe work implementations. Both authorities and individuals must value the importance of being safe in a work environment.
How To Conduct a Safety Observation
For authorities to properly perform a safety observation, here are some steps and guidelines to follow:
1. Focus
To conduct safety observation, you will need great focus. Remember, you are evaluating the safety of an environment to erase individuals worry if he or she is safe in a work environment. A significant focus would require a calm and relaxed mind. So make sure not to overwhelm yourself when you conduct a safety observation.
2. Check Everything
You must check every corner of an environment when you do a safety observation. Especially if the place you are working on is full of secret compartments and passageways such as classrooms and bank offices. You mustn’t forget to check the hidden parts of a specific environment since this is where most accidents begin.
3. Communicate
Don’t forget to communicate with other authorities since this type of procedure is teamwork. Make sure that you let them know about your observations so that they can give you suggestions on what you should do about it. Communicating can be a significant advantage since it makes the procedure of a safety observation much more manageable.
4. Raise Questions
In a safety observation, it is essential to ask questions about the things that you see in the environment. For example, if you found long cracks in a stairway of a building, you can ask the owner how the cracks appeared so you can write down information that will be needed in how these cracks need to be repaired. You will know important details by asking questions regarding the state of the safety observation.
5. Report
After the safety observation is completed, you must report your overall findings to your direct supervisor. The report will be the basis of how one should improve the operations and conditions of a particular area. For example, the safety observation report stated that some employees are careless in handling production machinery. That report can result in conducting training assessments for employees so that they can manage the machinery in a much better manner.
FAQs
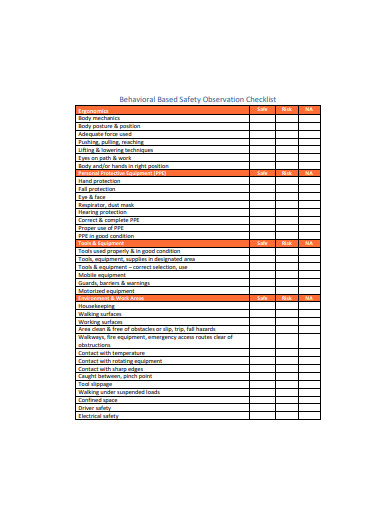
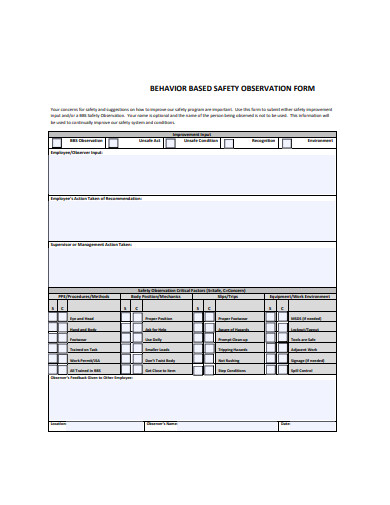
What is a behavior-based safety observation?
A behavior-based safety observation is a type of inspection conducted for employees. It is how their behavior is assessed in a workplace, whether if they have safe or unsafe behavior.
How do I know that the safety observation was conducted well?
You will know when a safety observation is conducted properly when the final report contains information that you didn’t know of. It means that the safety officers thoroughly checked your workplace, and some necessary improvements are needed to be made.
What are some hazardous objects that I can find in my workplace?
Hazardous items depend on what type of work environment you’re working on. For example, if you are working in a kitchen, then the dangerous things you can find are knives, blowtorches, and industrial ovens.
Do I need to provide a safety observation card to my employees?
Yes, a safety observation card would be needed for them to take note of some safety precautions. It will help in determining the unknown dangers of the workplace. Aside from this, you can also provide a safety checklist for your employees.
We must value the safety of our employees as they are an essential asset of a business. We have to make sure the safety observations must be done regularly since some events are unpredictable. One must know the importance of a safety observation program since it is how the safety of a place is evaluated. Being safe is a form of security every employee must feel because it can affect how they perform in the workplace.