Preparing for the CFA Exam requires a solid grasp of yield-based bond convexity and its impact on portfolio properties. Understanding convexity, which measures the sensitivity of bond prices to interest rate changes, is essential for managing bond portfolios effectively. Mastery of this concept aids in assessing risk and optimizing investment performance

Free CFA Pratice Test

Learning Objective

In studying “Yield-Based Bond Convexity and Portfolio Properties” for the CFA Exam, you should gain a clear understanding of convexity as a measure of the curvature in the relationship between bond prices and interest rate changes. Learn how to calculate convexity and assess its impact on bond portfolio sensitivity to interest rate fluctuations. Analyze the implications of convexity on portfolio duration, risk management, and investment strategies, focusing on how convexity adjustments enhance bond portfolio optimization. Additionally, develop the ability to apply convexity concepts in real-world portfolio scenarios, aiming to improve yield management and reduce interest rate risk in investment portfolios.

Understanding Bond Convexity

Bond convexity is a measure of the non-linear relationship between a bond’s price and changes in interest rates. It expands upon the concept of duration, which is a linear measure of bond price sensitivity to small interest rate changes. However, duration alone doesn’t capture how the sensitivity of bond prices shifts as interest rate changes grow larger. Convexity addresses this limitation by accounting for the curvature in the price-yield curve.

When interest rates change, bonds with higher convexity will experience larger price changes compared to bonds with lower convexity. Convexity is particularly useful when evaluating bonds with large price-yield fluctuations, as it helps investors better understand potential price movements for different rate scenarios. Positive convexity indicates that as interest rates fall, bond prices will increase at an accelerating rate, while as rates rise, prices will decrease at a decelerating rate. Bonds with embedded options, like callable bonds, can exhibit negative convexity, meaning they may decline in price faster than other bonds when rates rise.

In sum, bond convexity offers a refined perspective on interest rate sensitivity, making it a crucial tool for portfolio managers to manage interest rate risk more accurately and create balanced portfolios that optimize for both duration and convexity

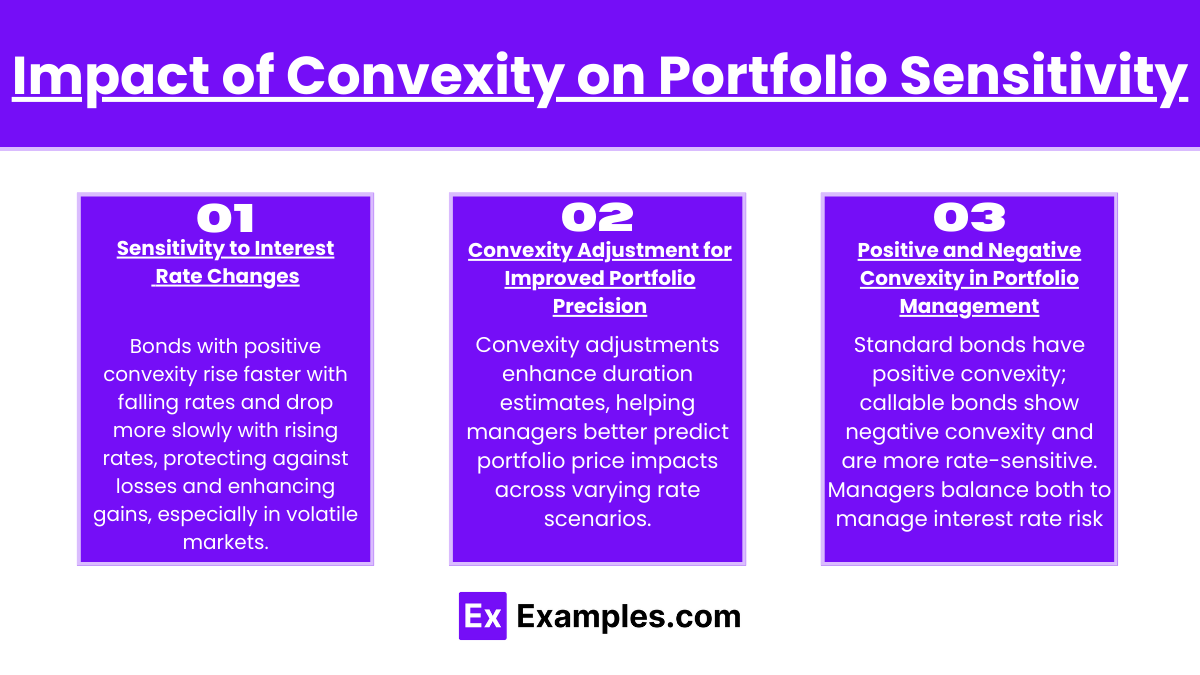
Impact of Convexity on Portfolio Sensitivity

Convexity significantly affects a bond portfolio’s sensitivity to changes in interest rates. While duration measures the approximate linear price response of bonds to small rate changes, convexity enhances this analysis by addressing how price sensitivity itself varies as interest rates fluctuate. This added insight is critical in accurately predicting bond price movements for larger interest rate shifts.

Sensitivity to Interest Rate Changes

For bonds with positive convexity, as interest rates decrease, prices increase at an accelerating rate, and as rates rise, prices decrease at a slower, decelerating rate. This asymmetry is beneficial to bondholders because it offers a buffer against rising rates, limiting potential losses, and amplifies gains when rates fall. In portfolios, bonds with high convexity tend to perform better in volatile interest rate environments due to their ability to absorb rate fluctuations more effectively.

Convexity Adjustment for Improved Portfolio Precision

Convexity adjustments refine duration-based estimates, particularly for portfolios containing bonds with varied maturities and features. When applied, these adjustments help portfolio managers more precisely estimate the overall price impact on a portfolio for different interest rate scenarios. For instance, a portfolio with bonds exhibiting higher convexity would be less sensitive to rate hikes than one relying solely on duration for rate impact estimates.

Positive and Negative Convexity in Portfolio Management

Positive convexity is typically seen in standard bonds, such as Treasuries, while negative convexity is common in bonds with embedded options, like callable bonds or mortgage-backed securities. Portfolios containing negative convexity instruments might be more vulnerable to rising interest rates, as these bonds can lose value more quickly when rates increase. Portfolio managers often adjust the balance of positive and negative convexity bonds based on their market outlook, using convexity as a means to control interest rate risk

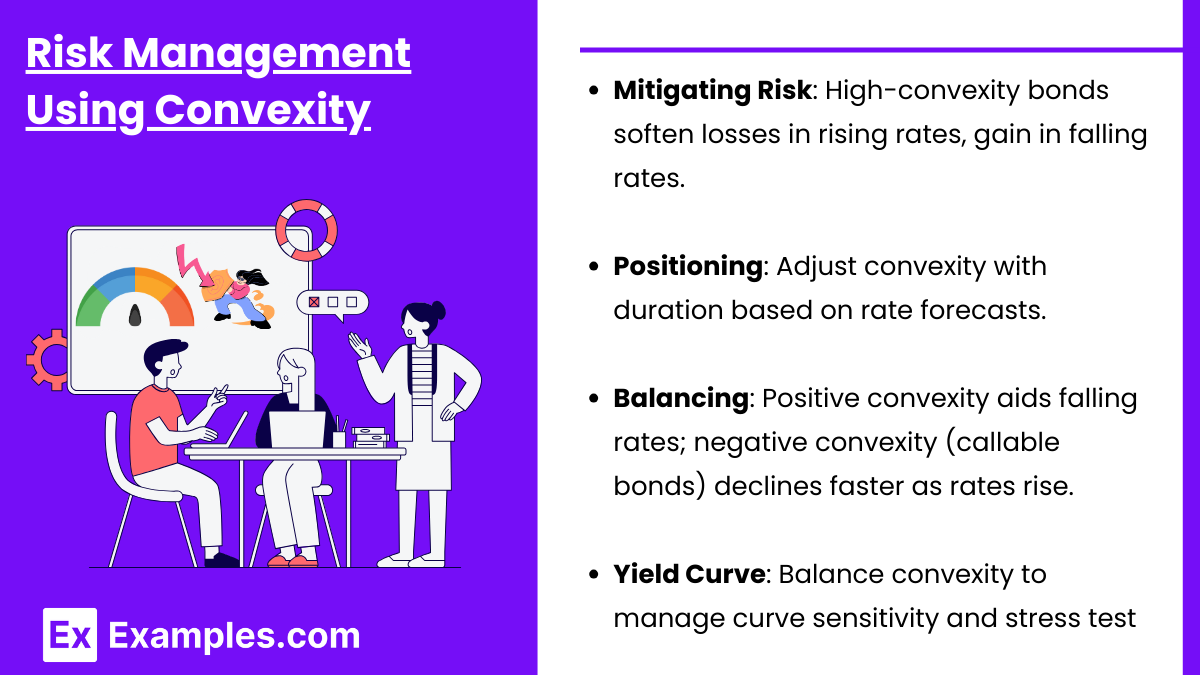
Risk Management Using Convexity

Convexity is a powerful tool in risk management for bond portfolios, as it enhances traditional duration analysis by more accurately accounting for interest rate volatility. By considering convexity, portfolio managers can better anticipate how bond prices will respond to significant rate shifts, which is essential for managing interest rate risk in dynamic market conditions.

Mitigating Interest Rate Risk

Convexity helps to mitigate interest rate risk by providing a clearer picture of a bond’s price volatility. Bonds with higher convexity are less sensitive to adverse interest rate changes. When interest rates rise, these bonds decline in price at a slower rate than those with lower convexity, offering some protection against losses. Conversely, if interest rates fall, high-convexity bonds benefit from an accelerated price increase, which enhances portfolio returns. This asymmetric response can make portfolios with high convexity more resilient in volatile interest rate environments.

Convexity Strategies for Portfolio Positioning

Portfolio managers use convexity strategies to adjust portfolio sensitivity based on market forecasts. In a low-interest-rate environment where rates are expected to rise, managers might reduce portfolio convexity by including bonds with shorter durations or lower convexity to minimize price impact from potential rate increases. In contrast, if rates are likely to decline, managers may increase portfolio convexity by adding long-duration or high-convexity bonds to capitalize on the accelerated price gains.

Balancing Positive and Negative Convexity

Bonds with positive convexity, such as typical government and corporate bonds, respond more favorably to falling rates, whereas bonds with negative convexity, such as callable bonds or mortgage-backed securities, can exhibit accelerated price declines when rates rise. Managing the mix of positive and negative convexity instruments allows portfolio managers to align the portfolio’s interest rate response with the desired risk profile. For instance, in rising rate environments, reducing exposure to negative convexity bonds can prevent excessive losses.

Using Convexity in Yield Curve and Risk Management

Convexity also plays a role in managing exposure to yield curve shifts, especially when the curve steepens or flattens. By balancing convexity across various maturities, portfolio managers can reduce sensitivity to yield curve changes, ensuring that the portfolio maintains stability across different market conditions. Additionally, convexity aids in stress testing scenarios, helping managers assess the potential impact of extreme rate movements on portfolio value

Application of Convexity in Portfolio Optimization

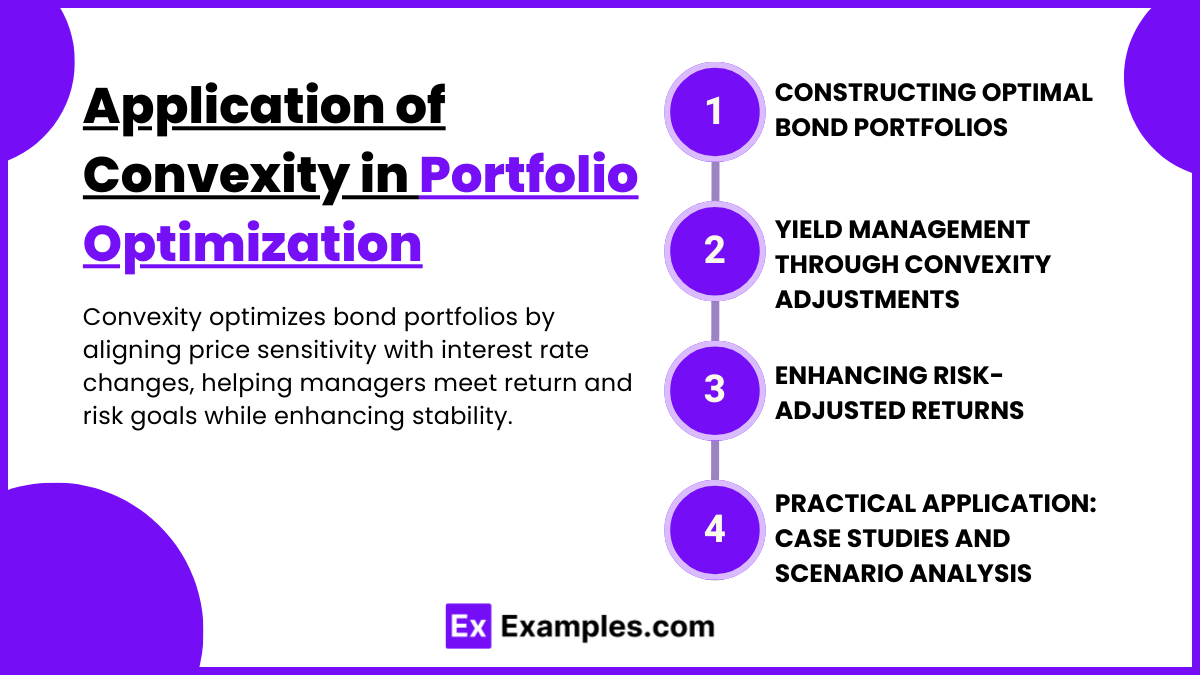
Convexity is essential for optimizing bond portfolios, as it allows portfolio managers to tailor interest rate sensitivity to meet specific return and risk objectives. By incorporating convexity into portfolio construction, managers achieve a more precise alignment of bond price movements with anticipated interest rate changes, enhancing overall portfolio stability and performance.

Constructing Optimal Bond Portfolios

When building a bond portfolio, managers consider convexity alongside duration to create a balanced and diversified mix of bonds. Bonds with higher convexity, while generally offering lower yields, provide greater protection against interest rate volatility. Conversely, low-convexity bonds can offer higher yields but are more vulnerable to interest rate shifts. An optimal portfolio often includes a combination of bonds with varying convexities, balancing stability with yield to match the investor’s risk tolerance and market outlook.

Yield Management Through Convexity Adjustments

Convexity adjustments allow managers to optimize portfolio yield in response to interest rate forecasts. For example, if interest rates are expected to decrease, managers may increase portfolio convexity by adding long-duration, high-convexity bonds to benefit from potential price gains. Alternatively, in a rising-rate environment, reducing convexity helps stabilize portfolio returns by limiting the impact of price declines. This strategic approach to adjusting convexity supports better yield management, providing flexibility to adapt to changing interest rates while protecting the portfolio’s income-generating capacity.

Enhancing Risk-Adjusted Returns

By optimizing for convexity, portfolio managers enhance risk-adjusted returns, aligning the portfolio with desired performance targets. A well-balanced portfolio, where convexity complements duration, allows for more controlled responses to interest rate fluctuations. This results in a portfolio that captures upward price movements in falling-rate environments while cushioning against losses when rates rise. In turn, improved risk-adjusted returns can attract investors seeking stability and growth, especially in uncertain markets.

Practical Application: Case Studies and Scenario Analysis

In real-world scenarios, portfolio managers use convexity to stress-test portfolios and anticipate responses to rate shocks. For instance, scenario analysis can reveal how portfolios with high convexity might outperform in periods of declining rates, while portfolios with low convexity maintain greater stability in rising-rate environments. This application of convexity in practical case studies and simulations allows managers to refine portfolio strategies, test the impact of rate changes, and make informed adjustments to meet investment goals

Examples

Example 1

A portfolio manager holds a mix of high-convexity government bonds and lower-convexity corporate bonds. As interest rates begin to fall, the government bonds with higher convexity experience a faster price appreciation, boosting the portfolio’s overall return. The corporate bonds provide steady income but less price increase, demonstrating how convexity affects different bond types.

Example 2

An investment firm anticipates a volatile interest rate environment and shifts its bond portfolio to include more bonds with positive convexity, such as long-term Treasuries. This move aims to benefit from price gains if rates decline while limiting losses if rates increase slightly. By increasing portfolio convexity, the firm seeks to reduce risk and enhance potential returns.

Example 3

A bond investor holds callable bonds with negative convexity. As interest rates rise, the prices of these callable bonds drop more quickly than similar bonds without call features. The negative convexity of callable bonds amplifies price declines in rising-rate environments, showing the impact of convexity type on bond price behavior.

Example 4

A financial analyst uses convexity adjustments to compare two bond portfolios with identical durations but different convexities. The portfolio with higher convexity shows lower sensitivity to interest rate hikes and more significant gains when rates fall. This analysis helps the analyst choose the portfolio better suited to the client’s risk tolerance.

Example 5

A portfolio manager conducts a scenario analysis to assess the impact of a sudden 2% drop in interest rates on a bond portfolio. Bonds with high convexity in the portfolio are projected to gain value significantly, while those with low convexity see minimal price change. This insight guides the manager in optimizing the portfolio’s convexity to align with future rate expectations

Practice Question

Question 1

Which of the following statements best describes the impact of positive convexity on a bond’s price sensitivity to interest rate changes?

A. A bond with positive convexity will lose value at an accelerating rate as interest rates rise.
B. Positive convexity means a bond’s price increases at a slower rate when interest rates fall.
C. A bond with positive convexity will experience larger price increases when interest rates fall and smaller price declines when rates rise.
D. Positive convexity results in greater sensitivity to changes in credit quality than interest rates.

Answer: C. A bond with positive convexity will experience larger price increases when interest rates fall and smaller price declines when rates rise.
Explanation: Positive convexity indicates that as interest rates fall, bond prices increase at an accelerating rate, while when rates rise, bond prices decrease at a decelerating rate. This asymmetric response benefits bondholders by providing larger price gains in falling-rate environments and smaller losses in rising-rate environments.

Question 2

In a bond portfolio, how does adding high-convexity bonds affect the portfolio’s sensitivity to interest rate changes?

A. It makes the portfolio more sensitive to interest rate declines and less sensitive to rate increases.
B. It decreases the portfolio’s overall duration.
C. It makes the portfolio equally sensitive to both increases and decreases in interest rates.
D. It has no effect on the portfolio’s interest rate sensitivity.

Answer: A. It makes the portfolio more sensitive to interest rate declines and less sensitive to rate increases
Explanation: High-convexity bonds increase a portfolio’s sensitivity to interest rate declines while reducing its sensitivity to rate increases. This is because positive convexity causes prices to rise faster when rates fall and decline slower when rates rise, creating an asymmetric response that mitigates the impact of rising rates on the portfolio.

Question 3

Which type of bond is likely to exhibit negative convexity?

A. Long-term government bonds
B. Callable corporate bonds
C. Zero-coupon bonds
D. Convertible bonds

Answer: B. Callable corporate bonds
Explanation: Callable bonds often exhibit negative convexity because, as interest rates decrease, the likelihood of the issuer calling the bond increases. This limits the bond’s price appreciation, leading to negative convexity. Other types of bonds, like zero-coupon bonds or non-callable government bonds, generally have positive convexity