Yield-Based Bond Duration Measures and Properties

Understanding yield-based bond duration measures and their properties is essential for CFA Exam success. Mastery of concepts like Macaulay duration, modified duration, and effective duration provides insights into interest rate sensitivity and bond price volatility. This knowledge is critical for effective risk management, bond valuation, and informed investment decisions in fixed-income portfolios.

Learning Objective

In studying “Yield-Based Bond Duration Measures and Properties” for the CFA Exam, you should aim to understand the concepts and calculations of various duration measures, including Macaulay, modified, and effective duration. Examine how these metrics quantify a bond’s sensitivity to interest rate changes, and analyze their roles in managing interest rate risk. Evaluate the properties of these measures under different market conditions, including convexity and yield curve shifts. Additionally, explore how duration applies to portfolio management, asset-liability matching, and risk assessment, applying your understanding to interpret and evaluate fixed-income strategies effectively in CFA practice scenarios.

Understanding Duration Measures: Macaulay, Modified, and Effective Duration

1. Macaulay Duration

• Definition: Macaulay Duration is the weighted average time until a bond’s cash flows (coupons and principal) are received. This measure helps in understanding how long it takes for an investor to recover the bond’s price.
• Calculation: Macaulay Duration is calculated by discounting each cash flow by the bond’s yield, then weighting it by the time period in which it occurs. The result is then divided by the bond’s price.
• Usage: While Macaulay Duration is more theoretical, it’s often used as a benchmark for assessing other duration measures. In practical terms, it’s useful for investors focused on the timing of cash flows.

2. Modified Duration

• Definition: Modified Duration takes Macaulay Duration a step further by directly measuring a bond’s price sensitivity to changes in interest rates. Specifically, it approximates the percentage change in bond price for a 1% change in yield.
• Calculation: Modified Duration is derived from Macaulay Duration by dividing it by (1 + yield per period). It shows how the bond’s price would likely move with small changes in yield.
• Importance: Modified Duration is widely used in bond pricing and portfolio management because it provides a quick estimate of interest rate risk. The higher the Modified Duration, the more sensitive a bond is to interest rate changes.

3. Effective Duration

• Definition: Effective Duration is particularly useful for bonds with embedded options (e.g., callable or putable bonds). Unlike Macaulay and Modified Duration, Effective Duration considers potential changes in cash flows due to shifts in interest rates.
• Calculation: Effective Duration is calculated by assessing how the bond price changes with small, parallel shifts in the yield curve. It captures the impact of changing yields on expected cash flows, especially relevant for bonds where early call or put options may be exercised.
• Application: Effective Duration is the preferred measure for bonds with embedded options because it accounts for the non-linear relationship between interest rates and bond prices. It provides a more accurate measure of interest rate risk in scenarios where future cash flows aren’t fixed

Properties and Applications of Duration in Interest Rate Risk Management

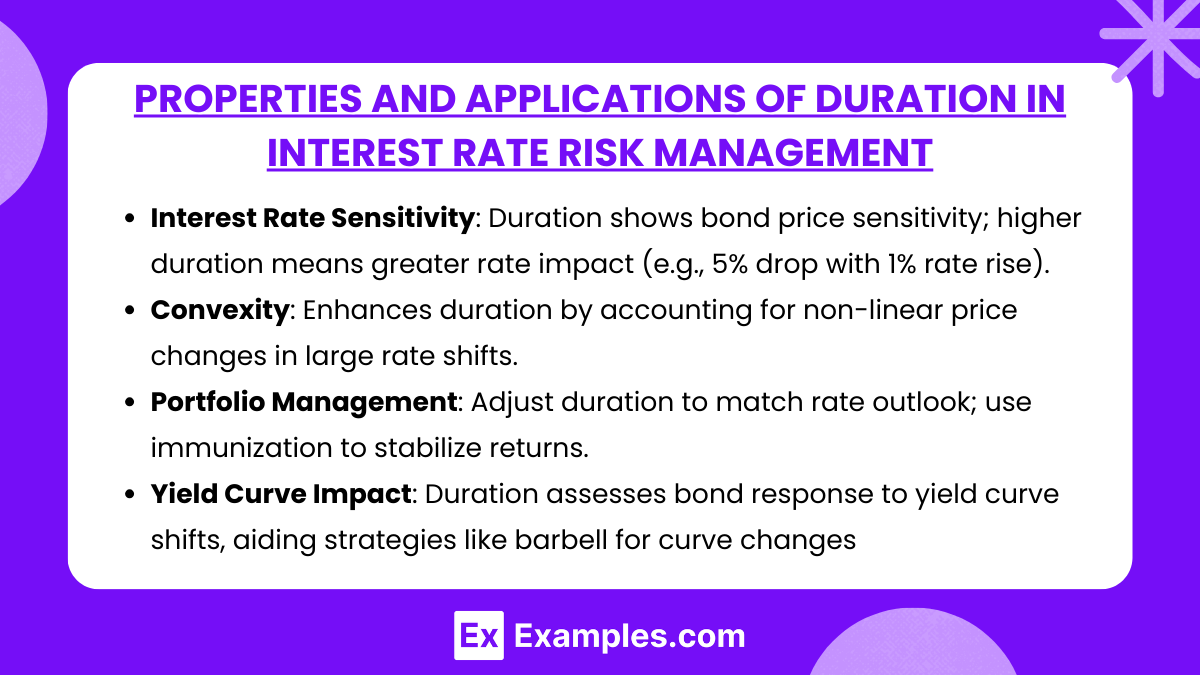
1. Interest Rate Sensitivity and Duration

• Property of Duration: Duration is a measure of the bond’s sensitivity to changes in interest rates, indicating how much the bond’s price is expected to change for a given change in interest rates.
• Application: By using duration, investors can gauge the level of interest rate risk in a bond or portfolio. Bonds with higher durations are more sensitive to interest rate changes, while those with lower durations are less sensitive.
• Example: A bond with a duration of 5 means its price is expected to decrease by approximately 5% if interest rates rise by 1%. This helps investors anticipate and manage potential losses in different interest rate scenarios.

2. Convexity and Duration Adjustment

• Property of Convexity: Duration provides a linear approximation of price sensitivity; however, bond prices react non-linearly to large changes in interest rates. Convexity is a secondary measure that accounts for this curvature in the price-yield relationship.
• Application: When managing portfolios, investors use both duration and convexity together to better approximate bond price changes for larger interest rate movements. Convexity adjustment improves accuracy, especially for longer-duration bonds or in volatile interest rate environments.
• Example: Adding convexity to duration calculations helps in assessing how the price of a bond will change if rates move up or down significantly, reducing estimation errors.

3. Application of Duration in Portfolio Management

• Balancing Risk and Return: Portfolio managers use duration to match investment strategies to interest rate expectations. By adjusting the average duration of a bond portfolio, they can align risk profiles with market forecasts.
• Duration Matching for Immunization: One application of duration is immunization, where portfolio duration is matched to the investment horizon to minimize the impact of interest rate changes on returns.
• Example: A portfolio manager expecting rising rates might shorten portfolio duration to limit price sensitivity, preserving capital, while an investor expecting rate cuts might increase duration to benefit from potential price appreciation.

4. Yield Curve Analysis and Duration

• Property of Yield Curve Impact: Duration is useful in assessing how bond prices are affected by movements along the yield curve, such as parallel shifts or non-parallel changes.
• Application: Investors analyze how yield curve changes impact bonds of different maturities. By understanding these dynamics, they can structure bond portfolios to capitalize on expected yield curve movements.
• Example: For a portfolio with a barbell structure (short and long maturities), duration analysis helps predict the impact of a steepening yield curve on the portfolio’s value. Duration helps in planning strategies for expected yield curve flattening, steepening, or twisting.

Yield Curve and Duration: Parallel and Non-Parallel Shifts

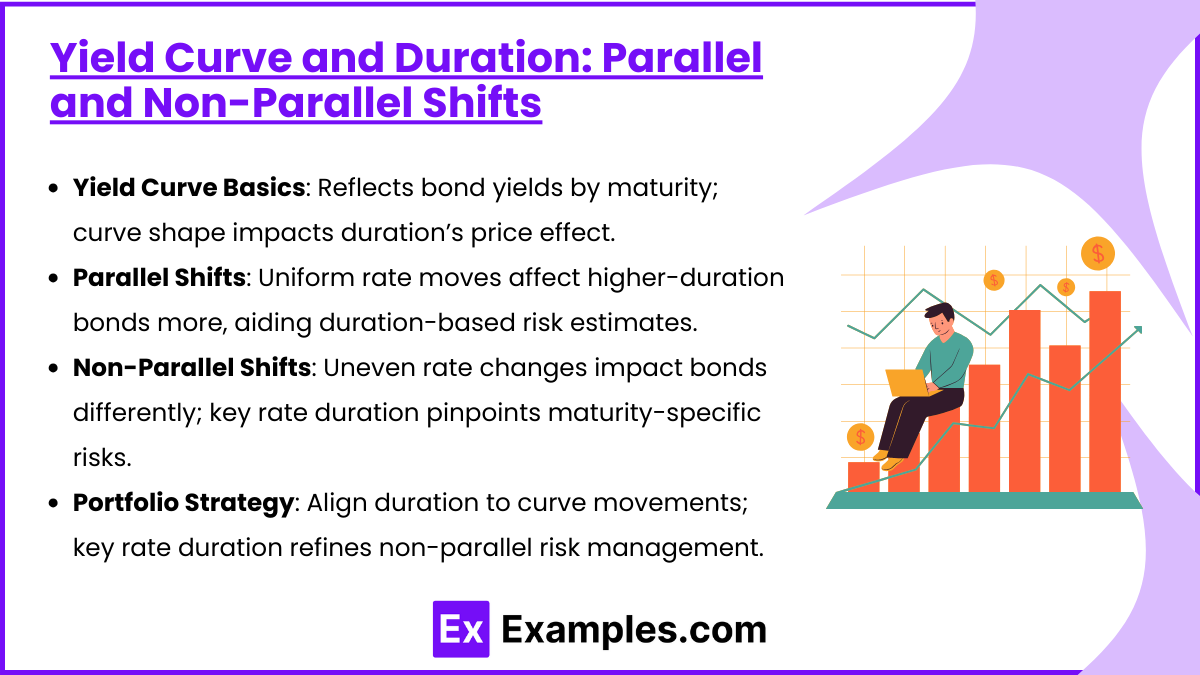
1. Understanding the Yield Curve

• Yield Curve Basics: The yield curve represents the relationship between bond yields and maturities, showing the market’s interest rate expectations. A typical yield curve can be upward sloping, flat, or inverted based on economic conditions.
• Importance for Duration: Duration measures a bond’s sensitivity to changes in yield, but the shape and movement of the yield curve directly influence how duration impacts a bond’s price.

2. Parallel Shifts in the Yield Curve

• Definition of Parallel Shift: A parallel shift occurs when interest rates across all maturities rise or fall by the same amount. This means the entire yield curve moves up or down uniformly.
• Impact on Duration and Bond Prices: In a parallel shift, bonds with higher duration are more sensitive to interest rate changes, experiencing more significant price changes. Duration calculations assume parallel shifts, so they are most accurate in this scenario.
• Application in Risk Management: For bond portfolios, parallel shifts help in estimating the impact of a uniform rate change across maturities. If rates increase by 1% across the board, a portfolio with a 5-year average duration would see a 5% decrease in value.

3. Non-Parallel Shifts in the Yield Curve

• Definition of Non-Parallel Shift: Non-parallel shifts happen when rates change unevenly across maturities. This can include:
  • Steepening: Long-term rates increase more than short-term rates.
  • Flattening: Short-term rates rise or fall more than long-term rates.
  • Twisting: Rates at different points in the curve move in opposite directions.
• Impact on Duration and Bond Prices: Non-parallel shifts create more complex price impacts. Bonds with different durations may react differently based on which part of the curve is affected. Long-duration bonds are more sensitive to long-term rate changes, while short-duration bonds are impacted more by short-term rate shifts.
• Application in Portfolio Management: Non-parallel shifts make duration management more challenging, as traditional duration doesn’t capture the effect of twists and turns in the yield curve. Portfolio managers use key rate durations (sensitivity to changes at specific maturities) to assess risk more accurately in these cases.

4. Practical Use in Portfolio Strategy

• Duration Positioning Based on Curve Shape: Anticipating yield curve movements is key to positioning duration effectively. A portfolio expecting a steepening yield curve might concentrate on shorter-duration bonds to minimize losses, while one anticipating a flattening curve might extend duration to capture long-term yield drops.
• Key Rate Duration Analysis: To manage non-parallel risk effectively, portfolio managers analyze how specific maturities affect overall portfolio sensitivity. Key rate durations allow them to estimate the impact of non-parallel shifts, fine-tuning bond holdings to adapt to anticipated yield curve changes.

Duration in Asset-Liability Management (ALM)

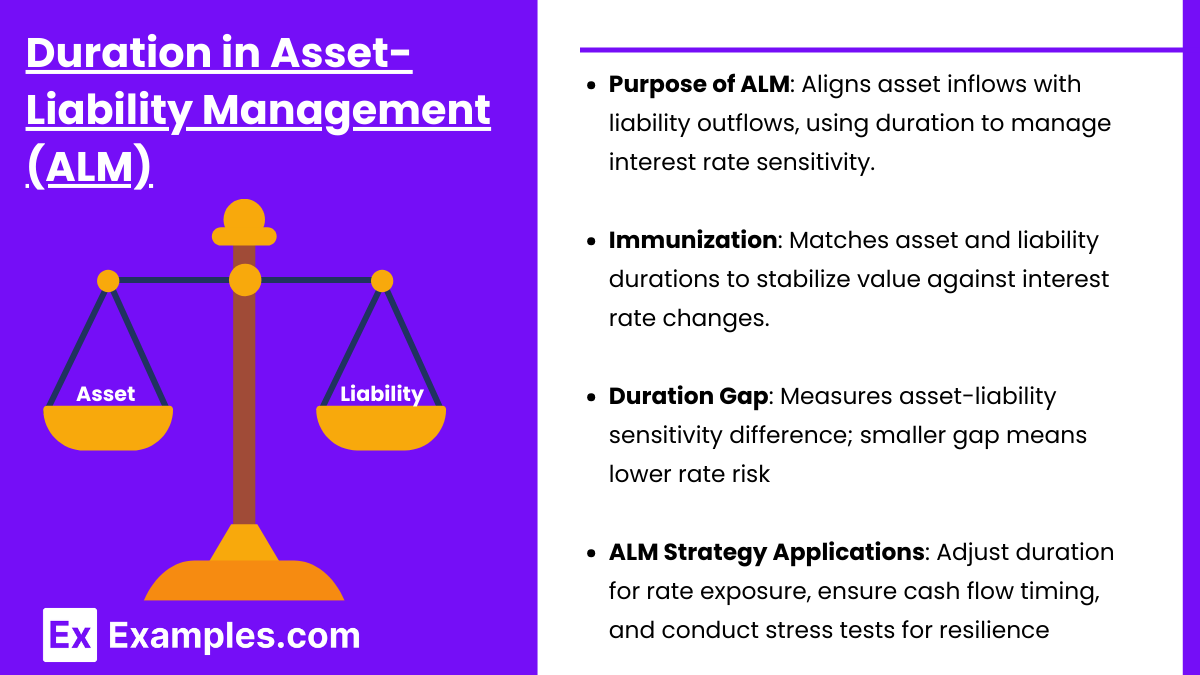
1. Purpose of Asset-Liability Management (ALM)

• Definition of ALM: Asset-Liability Management is a strategy employed by financial institutions to manage the risks arising from differences in the maturities and cash flows of assets and liabilities. It aims to balance the timing of cash inflows from assets with the cash outflows required to meet liabilities.
• Role of Duration in ALM: Duration serves as a measure of interest rate sensitivity for both assets and liabilities, allowing institutions to estimate how changes in interest rates impact their balance sheets. By aligning the durations of assets and liabilities, institutions can better manage their exposure to interest rate fluctuations.

2. Duration Matching for Liability Immunization

• Concept of Immunization: Immunization is an ALM strategy in which an institution matches the duration of its assets to that of its liabilities. This approach minimizes the impact of interest rate changes on the net present value of the balance sheet.
• Application in Managing Interest Rate Risk: When the duration of assets and liabilities is matched, any gains or losses from changes in interest rates are offset, stabilizing the institution’s net worth. This is especially important for entities with fixed liabilities, such as pension funds, where future liabilities are known, and they must secure assets that will cover these obligations.
• Example: If a pension fund has a 10-year liability, it would attempt to hold a portfolio of assets with a 10-year duration. This duration match would help ensure that even if interest rates change, the fund can meet its obligations without needing additional capital.

3. Duration Gap Analysis

• Duration Gap: The duration gap is the difference between the duration of an institution’s assets and liabilities. A positive duration gap indicates that assets have a longer duration than liabilities, while a negative gap means liabilities are more sensitive to interest rates.
• Managing the Duration Gap: An institution can assess its exposure to interest rate risk by analyzing the duration gap. A smaller gap implies lower interest rate risk, while a larger gap means that the institution may be exposed to significant shifts in market value if rates move unexpectedly.
• Example: For a bank with short-term deposits (liabilities) and long-term loans (assets), the duration gap would be positive. This situation exposes the bank to potential losses if interest rates rise, as the value of its long-term assets would decrease more than its short-term liabilities.

4. Applications of Duration in ALM Strategies

• Adjusting Portfolio Duration: By adjusting the duration of their asset portfolio, institutions can manage interest rate exposure. For example, reducing asset duration can help mitigate risk if a rise in interest rates is expected.
• Liquidity and Funding Stability: Duration helps ensure that cash flows from assets are timed to meet liability obligations, enhancing liquidity. This stability is crucial for institutions with predictable liability streams, such as insurance companies needing to pay policy claims.
• Stress Testing and Scenario Analysis: Institutions use duration as a tool in stress testing, simulating various interest rate scenarios to understand their impact on the asset-liability balance. This analysis helps in preparing for adverse market conditions and optimizing capital allocation

Examples

Example 1: Calculating Macaulay Duration

An investor holds a 10-year bond with a 5% annual coupon rate. To calculate the Macaulay Duration, they discount each cash flow (coupon payments and principal repayment) by the bond’s yield (say, 6%) and multiply each discounted cash flow by the time period in which it is received. By summing these weighted cash flows and dividing by the bond’s price, the investor finds the Macaulay Duration to be approximately 8.2 years. This figure tells the investor that, on average, they will recover the bond’s purchase price in about 8.2 years.

Example 2: Using Modified Duration to Measure Price Sensitivity

A portfolio manager holds a bond with a Modified Duration of 7. This means that for every 1% change in interest rates, the bond’s price is expected to change by approximately 7%. If interest rates increase by 1%, the bond’s price will decrease by about 7%, making Modified Duration a helpful tool for estimating price risk due to small interest rate changes.

Example 3: Applying Effective Duration for Callable Bonds

Consider a callable bond with an effective duration of 4.5, compared to an otherwise similar non-callable bond with a duration of 7. Because the bond is callable, its effective duration is lower, reflecting the issuer’s ability to call the bond if interest rates fall. This lower effective duration indicates that the bond is less sensitive to interest rate changes than a similar bond without the call option, as expected cash flows may change if the bond is called early.

Example 4: Impact of Yield Curve Shifts on Duration

A bank’s fixed-income portfolio includes both short- and long-duration bonds. In a parallel yield curve shift, all interest rates rise by 1%, leading to proportional decreases in bond prices across maturities. However, in a non-parallel shift where long-term rates increase more than short-term rates (steepening yield curve), the long-duration bonds will see greater price declines than the shorter-duration bonds. This scenario demonstrates how different yield curve movements impact bond duration sensitivity.

Example 5: Immunization Strategy with Duration Matching

An insurance company has a liability due in 5 years and matches it with a bond portfolio that also has a duration of 5. By aligning the asset duration with the liability duration, the company minimizes interest rate risk and ensures it can meet its obligation regardless of minor interest rate changes. If interest rates rise or fall, the value of both the assets and liabilities will shift similarly, protecting the company’s net position.

Practice Questions

Question 1

Which of the following statements best describes the Macaulay Duration of a bond?

A) It is the time-weighted average of a bond’s cash flows, measured in years.
B) It represents the percentage change in bond price for a 1% change in yield.
C) It is used primarily for bonds with embedded options to measure interest rate sensitivity.
D) It measures the convexity of a bond’s price-yield relationship.

Answer: A) It is the time-weighted average of a bond’s cash flows, measured in years

Explanation: Macaulay Duration is the weighted average time, measured in years, until a bond’s cash flows are received, which helps investors understand the time it takes to recover the bond’s purchase price. Option B describes Modified Duration, Option C refers to Effective Duration, and Option D describes Convexity.

Question 2

Which of the following bond types is best evaluated using Effective Duration?

A) Zero-coupon bonds
B) Fixed-rate bonds without embedded options
C) Callable bonds
D) Inflation-linked bonds

Answer: C) Callable bonds

Explanation: Effective Duration is used for bonds with embedded options, such as callable or putable bonds, as it considers the potential change in cash flows due to shifts in interest rates. For zero-coupon or fixed-rate bonds without options, Modified Duration is more appropriate.

Question 3

If a bond has a Modified Duration of 6, what would be the approximate percentage price change if interest rates decrease by 0.5%?

A) 3% increase
B) 6% decrease
C) 0.5% increase
D) 1.5% increase

Answer: A) 3% increase

Explanation: Modified Duration estimates the percentage change in bond price for a given change in yield. With a Modified Duration of 6, a 0.5% decrease in interest rates would lead to an approximate 3% increase in bond price (6 x 0.5% = 3%).