A+B Whole Square

The formula (𝑎+𝑏)², commonly known as the square of a binomial, is a foundational concept in algebra that simplifies to 𝑎²+2𝑎𝑏+𝑏². This expression plays a crucial role in various mathematical calculations, including those involving rational and irrational numbers, integers, and more complex algebraic structures. In contexts ranging from elementary algebra to more advanced applications like statistics and the least squares method, this identity helps in expanding equations and simplifying computations. Understanding how to apply and manipulate this formula is essential for solving quadratic equations, optimizing numerical methods, and analyzing data variability in statistical models.

What is (a+b) Whole Square Formula?

The expression (𝑎+𝑏)² is a fundamental algebraic identity known as the square and square root of a binomial. It represents the squared result of adding two numbers or expressions together. This formula is essential in various mathematical disciplines and simplifies many algebraic processes.

Formula Expansion

(𝑎+𝑏)² = 𝑎²+2𝑎𝑏+𝑏²

Breakdown of the Formula

• a²: The square of the first term.
• 2𝑎𝑏: Twice the product of the first and second terms.
• 𝑏²: The square of the second term.

Illustration Through Distribution

The expanded form can be derived using the distributive property (also known as the FOIL method for binomials):

1. First: Multiply the first term in each binomial: 𝑎×𝑎 = 𝑎².
2. Outer: Multiply the outer terms: 𝑎×𝑏 = 𝑎𝑏.
3. Inner: Multiply the inner terms: 𝑏×𝑎 = 𝑏𝑎.
4. Last: Multiply the last term in each binomial: 𝑏×𝑏 = 𝑏².

Combining these, you get 𝑎2+𝑎𝑏+𝑏𝑎+𝑏², which simplifies to 𝑎²+2𝑎𝑏+𝑏² because 𝑎𝑏 and 𝑏𝑎 are equivalent.

Geometric Proof of a + b Whole Square Formula

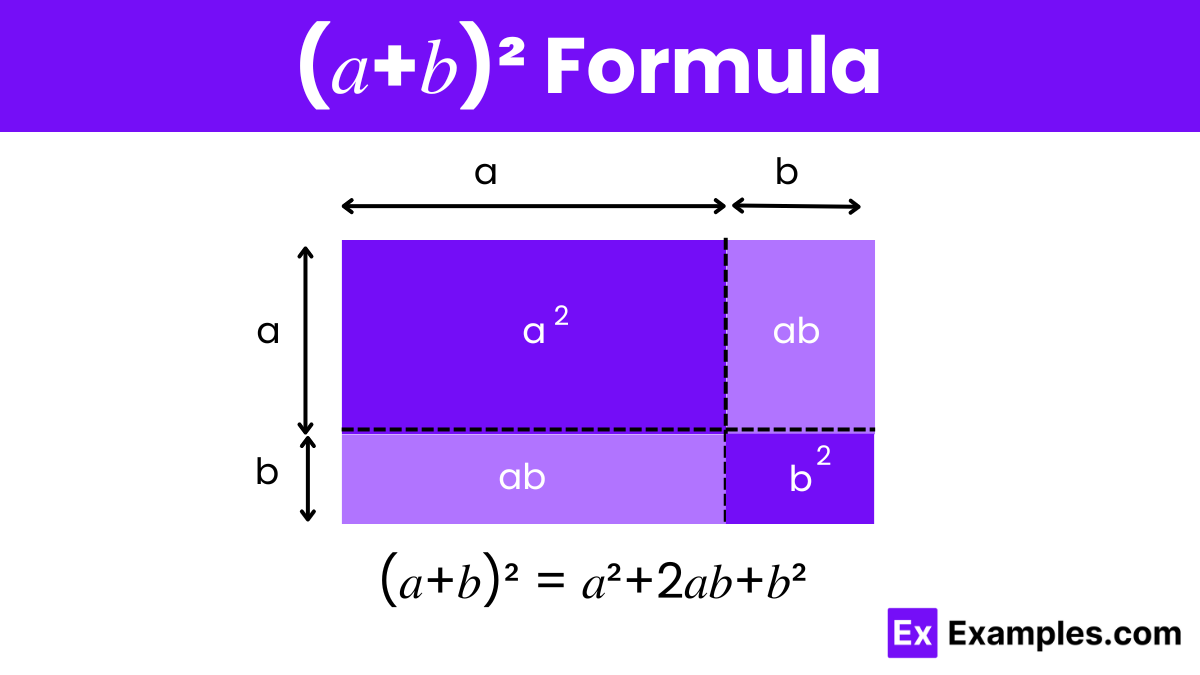
A geometric proof of the formula (𝑎+𝑏)² = 𝑎²+2𝑎𝑏+𝑏² effectively demonstrates how algebra and geometry can work together to confirm mathematical truths. This proof uses a simple geometric construction involving a square and rectangles. Here’s a step-by-step explanation of the proof:

Geometric Construction

1. Construct a Large Square: Draw a large square with each side measuring (𝑎+𝑏). This is the total area we want to express in terms of 𝑎 and 𝑏.
2. Divide the Square: Draw a line parallel to the sides of the square to split it into two rectangles and two smaller squares:
   • One side of each smaller square will measure 𝑎, and the other side will measure 𝑏.
   • You end up with one square measuring 𝑎×𝑎 (or 𝑎²), one square measuring 𝑏×𝑏 (or 𝑏²), and two rectangles, each measuring 𝑎×𝑏 (since the sides of the rectangles are the sides 𝑎 and 𝑏 of the two squares).

Demonstrating the Area

1. Area of the Large Square: The total area of the large square by its definition is (𝑎+𝑏)².
2. Area of the Components Inside the Large Square:

• The area of the smaller square with side 𝑎 is 𝑎².
• The area of the smaller square with side 𝑏 is 𝑏².
• The area of each rectangle is 𝑎𝑏. Since there are two such rectangles, their combined area is 2𝑎𝑏.

Summing the Areas

Add up the areas of the smaller squares and rectangles inside the large square: 𝑎²+2𝑎𝑏+𝑏²

Conclusion

Since both the area of the large square and the sum of the areas of the squares and rectangles inside it represent the same total area, you can equate them: (𝑎+𝑏)² = 𝑎²+2𝑎𝑏+𝑏²

This geometric proof not only confirms the algebraic identity (𝑎+𝑏)² = 𝑎²+2𝑎𝑏+𝑏² but also provides a visual and intuitive understanding of why the formula works. The proof is particularly useful in educational settings, where visual learning aids can help deepen understanding and retention of algebraic concepts.

Examples on (a+b) Whole Square Formula

Example 1: Simple Numerical Expansion

Problem: Calculate (3+5)² using the (𝑎+𝑏)² formula.

Solution:

Identify 𝑎 and 𝑏:

Let 𝑎 = 3 and 𝑏 = 5.

Apply the (𝑎+𝑏)² formula: (𝑎+𝑏)² = 𝑎²+2𝑎𝑏+𝑏²

(3+5)2 = 3²+2(3)(5)+5² = 9+30+25 = 64

Conclusion: (3+5)² calculates to 64, confirming the formula’s correctness as 8² also equals 64.

Example 2: Algebraic Expression Expansion

Problem: Expand and simplify the expression (𝑥+4)².

Solution:

Identify 𝑎 and 𝑏:

Let 𝑎 = 𝑥 and 𝑏 = 4.

Apply the (𝑎+𝑏)² formula: (𝑎+𝑏)² = 𝑎²+2𝑎𝑏+𝑏²

(𝑥+4)² = 𝑥²+2(𝑥)(4)+4² = 𝑥²+8𝑥+16

Conclusion: The expanded form of (𝑥+4)² is 𝑥²+8𝑥+16, illustrating how the formula simplifies algebraic binomial squaring.

Example 3: Application in Geometry

Problem: If the side length of a square is increased by 2 cm, and the original side length is 6 cm, find the area of the new square.

Solution:

1. Original side length (𝑎): 6 cm
2. Increase (𝑏): 2 cm
3. New side length: 𝑎+𝑏 = 6+2 = 8cm
4. Calculate the area using (𝑎+𝑏)²: (6+2)² = 6²+2(6)(2)+2² = 36+24+4 = 64 cm²

Conclusion: The area of the new square with side length 8 cm is 64 cm², easily calculated using the (𝑎+𝑏)² formula.

FAQs

What is the formula (𝑎+𝑏)² used for in mathematics?

The formula (𝑎+𝑏)² = 𝑎²+2𝑎𝑏+𝑏² is primarily used to expand and simplify expressions where two terms are squared together. It is a fundamental algebraic identity that helps in solving quadratic equations, simplifying algebraic expressions, and calculating areas in geometric contexts.

Can the (𝑎+𝑏)² formula be used with any type of number?

Yes, the (𝑎+𝑏)² formula is versatile and can be applied to any numbers—integers, decimals, fractions, and even irrational numbers—as long as they adhere to basic algebraic rules.

How does understanding the (𝑎+𝑏)² formula benefit students in their academic pursuits?

Mastering the (𝑎+𝑏)² formula equips students with the skills to handle complex algebraic operations, enhances their problem-solving capabilities, and lays a solid foundation for advanced mathematical studies, including calculus and beyond. It also aids in standardized tests that often include algebraic manipulations.