Composite Numbers
Composite Numbers
Composite Numbers with our comprehensive guide, designed to make mathematics engaging and accessible for educators and students. This resource unravels the complexity of composite numbers, offering clear definitions, vivid examples, and practical applications. By bridging the gap between abstract concepts and tangible understanding, we aim to enhance mathematical literacy, foster curiosity, and empower learners to explore the vast landscape of numbers with confidence.
What are Composite Numbers?
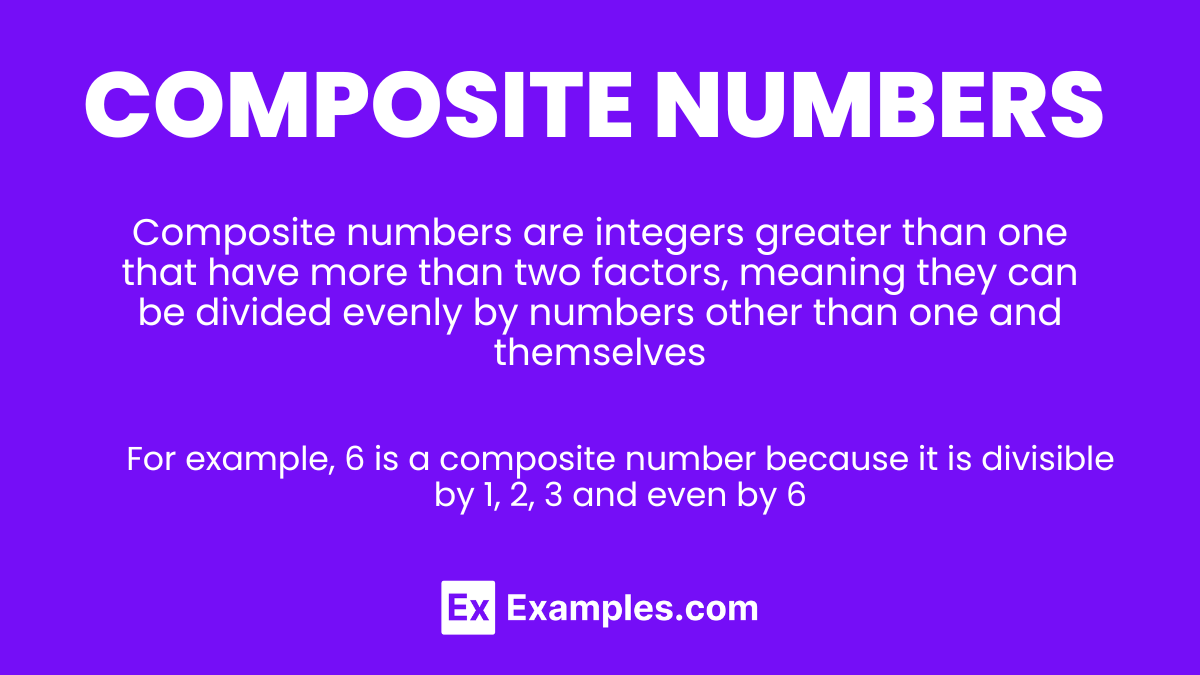
Composite numbers are integers greater than one that have more than two factors, meaning they can be divided evenly by numbers other than one and themselves. Unlike prime numbers, which are only divisible by one and themselves, composite numbers can be broken down into smaller factors. This concept is crucial in the study of mathematics, offering a foundation for understanding divisibility, factorization, and the fundamental theorem of arithmetic.
What is the Best Example of a Composite Number?
A prime example of a composite number is 4. It is greater than one and has three factors: 1, 2, and 4. Unlike a prime number, which only has two distinct factors, 4 can be evenly divided by 2, showcasing its composite nature. This illustrates the basic principle of composite numbers — they are the building blocks of mathematics, enabling the exploration of more complex concepts such as factorization and the study of number properties.
Composite Numbers Examples
| Composite Numbers | Factors |
|---|---|
| 6 | 1, 2, 3, 6 |
| 14 | 1, 2, 7, 14 |
| 25 | 1, 5, 25 |
| 30 | 1, 2, 3, 5, 6, 10, 15, 30 |
| 52 | 1, 2, 4, 13, 26, 52 |
| 35 | 1, 5, 7, 35 |
| 45 | 1, 3, 5, 9, 15, 45 |
| 63 | 1, 3, 7, 9, 21, 63 |
| 72 | 1, 2, 3, 4, 6, 8, 9, 12, 18, 24, 36, 72 |
| 81 | 1, 3, 9, 27, 81 |
Composite numbers are integers that have at least one divisor other than 1 and themselves. They play a significant role in various mathematical concepts and applications. Understanding composite numbers helps students grasp the basics of number theory, factorization, and divisibility rules, enhancing their problem-solving skills.
Examples:
- Number 6:
- Explanation: 6 is divisible by 1, 2, 3, and 6. Its ability to be divided by 2 and 3 in addition to 1 and itself classifies it as a composite number.
- Number 12:
- Explanation: 12 can be divided by 1, 2, 3, 4, 6, and 12. The presence of multiple divisors highlights its composite nature, making it a fundamental example in teaching divisibility.
- Number 15:
- Explanation: 15 is divisible by 1, 3, 5, and 15. It demonstrates the concept of composite numbers through its divisibility by numbers other than just 1 and itself.
- Number 21:
- Explanation: 21 has divisors of 1, 3, 7, and 21. This example is particularly useful in explaining how prime factorization works for composite numbers.
- Number 100:
- Explanation: 100 is divisible by 1, 2, 4, 5, 10, 20, 25, 50, and 100. Its wide range of divisors serves as an excellent example to discuss factors and multiples.
Properties of Composite Numbers
The properties of composite numbers are crucial for understanding their behavior within the number system. These properties assist in distinguishing composite numbers from primes and play a vital role in various mathematical operations and problem-solving.
Properties:
- Divisibility by More Than Two Numbers:
- Explanation: Composite numbers are defined by their divisibility by at least one integer besides 1 and themselves, illustrating the core property that sets them apart from prime numbers.
- Presence in Even Numbers:
- Explanation: With the exception of 2, all even numbers are composite because they are divisible by 2, showcasing a simple method for identifying many composite numbers.
- Factorization:
- Explanation: Composite numbers can be expressed as the product of smaller numbers, other than 1 and the number itself, highlighting their role in understanding factor trees and prime factorization.
- Square Numbers:
- Explanation: Many composite numbers are perfect squares (e.g., 4, 9, 16), showing that numbers multiplied by themselves result in composite outcomes.
- Multiples of Primes:
- Explanation: Every composite number can be broken down into prime factors, demonstrating that they are essentially multiples of prime numbers, which is key in studying the fundamental theorem of arithmetic.
List of Composite Numbers
Composite Numbers Chart
Composite Numbers 1 to 200
4, 6, 8, 9, 10, 12, 14, 15, 16, 18, 20, 21, 22, 24, 25, 26, 27, 28, 30, 32, 33, 34, 35, 36, 38, 39, 40, 42, 44, 45, 46, 48, 49, 50, 51, 52, 54, 55, 56, 57, 58, 60, 62, 63, 64, 65, 66, 68, 69, 70, 72, 74, 75, 76, 77, 78, 80, 81, 82, 84, 85, 86, 87, 88, 90, 91, 92, 93, 94, 95, 96, 98, 99, 100, 102, 104, 105, 106, 108, 110, 111, 112, 114, 115, 116, 117, 118, 119, 120, 121, 122, 123, 124, 125, 126, 128, 129, 130, 132, 133, 134, 135, 136, 138, 140, 141, 142, 143, 144, 145, 146, 147, 148, 150, 152, 154, 155, 156, 157, 158, 159, 160, 162, 164, 165, 166, 168, 170, 172, 174, 175, 176, 177, 178, 180, 182, 184, 185, 186, 187, 188, 189, 190, 192, 194, 195, 196, and 198.
How to Find the Composite Number?
Discovering composite numbers involves identifying integers greater than one that are divisible by at least one other number besides one and themselves. This process is essential for strengthening mathematical understanding and analytical skills. By employing divisibility tests, learners can efficiently distinguish composite numbers from primes, enhancing their ability to navigate through various mathematical challenges. This knowledge forms the backbone of arithmetic and number theory, serving as a crucial step in the exploration of factors, multiples, and the structure of numbers.
Examples:
- Number 6:
- Explanation: 6 is divisible by 1, 2, 3, and 6. Its divisibility by numbers other than 1 and itself (2 and 3) classifies it as a composite number.
- Number 15:
- Explanation: 15 has factors 1, 3, 5, and 15. The presence of factors 3 and 5, in addition to 1 and 15, confirms its status as a composite number.
- Number 21:
- Explanation: Recognized as composite because it is divisible by 1, 3, 7, and 21. The factors 3 and 7 demonstrate its composite nature.
- Number 28:
- Explanation: 28 is divisible by 1, 2, 4, 7, 14, and 28. Its multiple factors beyond 1 and itself make it a clear example of a composite number.
- Number 45:
- Explanation: With factors including 1, 3, 5, 9, 15, and 45, 45’s divisibility by several numbers verifies it as composite.
Types of Composite Numbers
Composite numbers are categorized based on their factors and the uniqueness of these factors. Understanding the different types enriches mathematical discussions and problem-solving strategies, offering a nuanced view of number theory. This segmentation helps in identifying patterns and applying mathematical concepts more effectively, particularly in factorization and the study of prime factorials.
Examples:
- Square Numbers:
- Explanation: Composite numbers like 16, whose square root is an integer (4), fall into this category. It’s composite because it has more factors than just 1 and itself.
- Rectangular Numbers:
- Explanation: Consider 8, which can be arranged in a rectangle but not a square. Its factors (1, 2, 4, 8) make it a composite rectangular number.
- Cubic Numbers:
- Explanation: Numbers like 8, which is 2^3, demonstrate cubic composites, showcasing three-dimensional factorization properties.
- Even Composite Numbers:
- Explanation: Numbers such as 10 are even and composite because they have even divisibility and multiple factors (1, 2, 5, 10).
- Odd Composite Numbers:
- Explanation: Numbers like 9, divisible by 1, 3, and 9, highlight odd composites, distinguished by their odd number of factors beyond the solitary and the number itself.
Smallest Composite Number
The smallest composite number is 4. It’s the first integer greater than 1 that is not prime, as it can be divided evenly by 1, 2, and itself. Understanding this concept is pivotal for students beginning to explore the realm of number theory, providing a clear starting point for identifying and classifying numbers based on their factors. This foundational knowledge is essential for grasping more complex mathematical concepts.
Examples:
- Number 4:
- Explanation: 4 is the smallest composite number because it has three factors: 1, 2, and 4. This makes it the first composite number students encounter.
- Comparison with 2 and 3:
- Explanation: Unlike 4, the numbers 2 and 3 are prime because they can only be divided by 1 and themselves, highlighting the defining characteristic of composite numbers.
- Factorization of 4:
- Explanation: 4 can be factorized as 2 x 2, demonstrating its composite nature through its multiple factors.
- Use in Learning:
- Explanation: Recognizing 4 as the smallest composite number helps students understand the transition from prime to composite numbers in the number sequence.
- Mathematical Significance:
- Explanation: The concept of the smallest composite number introduces the idea of divisibility beyond prime numbers, a key concept in mathematics.
Difference Between Prime and Composite Numbers
Understanding the difference between prime and composite numbers is crucial for students to navigate through basic arithmetic and number theory. Prime numbers have exactly two distinct positive divisors: 1 and themselves. In contrast, composite numbers have more than two divisors, making them divisible by at least one other number besides 1 and themselves.
| Feature | Prime Numbers | Composite Numbers |
|---|---|---|
| Definition | Numbers greater than 1 that have exactly two factors: 1 and themselves. | Numbers greater than 1 that have more than two factors. |
| Examples | 2, 3, 5, 7, 11 | 4, 6, 8, 9, 10 |
| Divisibility | Can only be divided evenly by 1 and the number itself. | Can be divided evenly by at least one other number besides 1 and itself. |
| Factor Count | Exactly two distinct factors. | More than two distinct factors. |
| Factorization | Cannot be broken down into other prime numbers. | Can be broken down into smaller prime numbers. |
| First Instance | 2 (the only even prime number) | 4 (the smallest composite number) |
| Nature | Building blocks of all numbers. | Made up of prime numbers multiplied together. |
Prime Factorization of Composite Numbers
| Composite Number | Prime Factorization |
|---|---|
| 4 | 2 x 2 |
| 6 | 2 x 3 |
| 8 | 2 x 2 x 2 |
| 9 | 3 x 3 |
| 10 | 2 x 5 |
| 12 | 2 x 2 x 3 |
| 15 | 3 x 5 |
| 18 | 2 x 3 x 3 |
| 20 | 2 x 2 x 5 |
| 24 | 2 x 2 x 2 x 3 |
| 25 | 5 x 5 |
| 30 | 2 x 3 x 5 |
| 36 | 2 x 2 x 3 x 3 |
| 40 | 2 x 2 x 2 x 5 |
| 45 | 3 x 3 x 5 |
| 50 | 2 x 5 x 5 |
| 60 | 2 x 2 x 3 x 5 |
| 72 | 2 x 2 x 2 x 3 x 3 |
| 80 | 2 x 2 x 2 x 2 x 5 |
| 90 | 2 x 3 x 3 x 5 |
| 100 | 2 x 2 x 5 x 5 |
Prime factorization of composite numbers involves breaking down a composite number into a set of prime numbers that, when multiplied together, give the original number. This process is fundamental in mathematics, enabling the simplification of fractions, finding the greatest common divisors, and solving a variety of problem types.
Examples:
- Number 12:
- Explanation: The prime factorization of 12 is 2 x 2 x 3, demonstrating how composite numbers can be expressed as products of prime numbers.
- Number 18:
- Explanation: 18 can be broken down into 2 x 3 x 3, showing the decomposition of a composite number into its prime components.
- Number 20:
- Explanation: The prime factorization of 20 is 2 x 2 x 5, illustrating the use of prime factorization in identifying the prime building blocks.
- Number 30:
- Explanation: 30 is factorized as 2 x 3 x 5, highlighting how different prime numbers can combine to form a composite number.
- Number 100:
- Explanation: 100’s prime factorization is 2 x 2 x 5 x 5, showcasing a more complex example of breaking down a composite number into prime factors.
Solved Problems on Composite Numbers
Problem 1: Identifying a Composite Number
Question: Is 15 a composite number?
Solution: Yes, 15 is a composite number because it has more than two divisors. It can be divided evenly by 1, 3, 5, and 15.
Problem 2: Finding the Smallest Composite Number
Question: What is the smallest composite number?
Solution: The smallest composite number is 4. It’s the first number after 1 that is not a prime number, with divisors being 1, 2, and 4.
Problem 3: Prime Factorization of a Composite Number
Question: What is the prime factorization of 18?
Solution: The prime factorization of 18 is 2 x 3 x 3. This shows that 18 can be divided into prime numbers 2 and 3, where 3 is used twice.
Problem 4: Verifying a Composite Number
Question: Verify if 22 is a composite number and provide its prime factorization.
Solution: Yes, 22 is a composite number because it has divisors other than 1 and itself. Its prime factorization is 2 x 11, indicating it can be divided by 2 and 11.
Problem 5: Composite Number Between a Range
Question: Find a composite number between 10 and 20.
Solution: One composite number between 10 and 20 is 12. It’s composite because it can be divided by 1, 2, 3, 4, 6, and 12. Another example is 15, divisible by 1, 3, 5, and 15.
Composite numbers play a crucial role in the foundation of mathematics, bridging the gap between basic arithmetic and complex number theory. Understanding and working with composite numbers not only enriches students’ mathematical skills but also fosters critical thinking through factorization and divisibility concepts. This article aimed to demystify composite numbers, making them accessible and engaging for both teachers and students in their mathematical journey.
Share :
