What is the cube of 2?
4
6
8
10
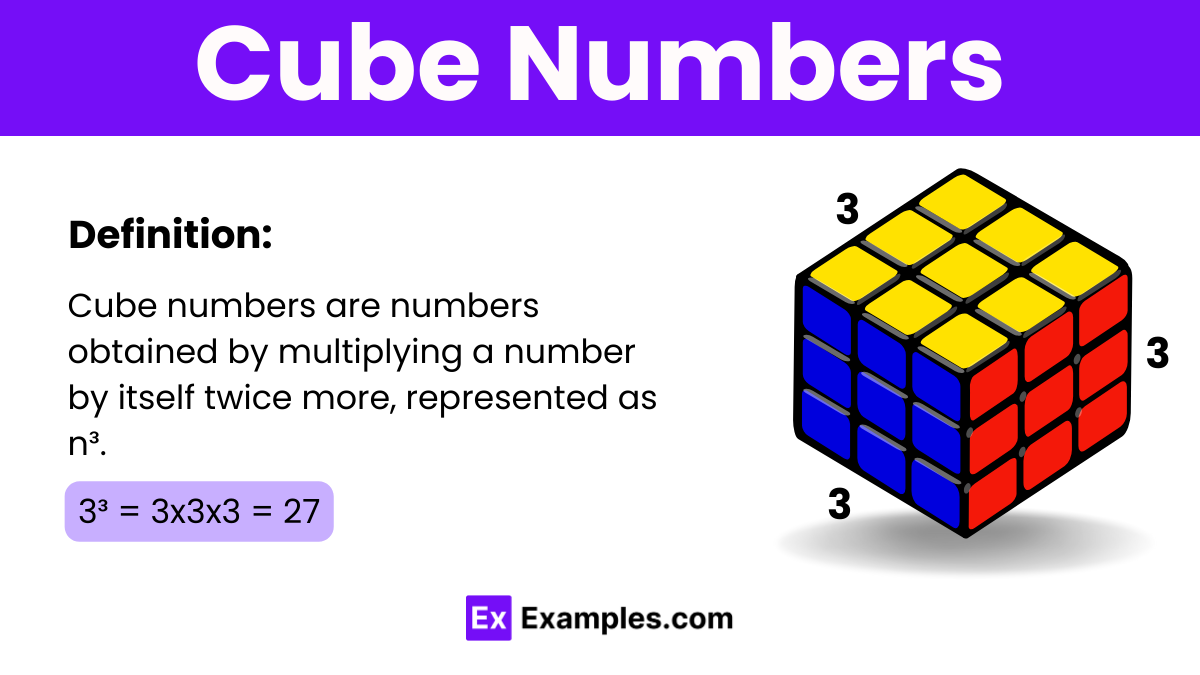
Cube numbers are special numbers that you get when you multiply a number by itself twice. It’s like taking a number and using it three times in a multiplication. For example, if we think about building a cube out of blocks, the total number of blocks used gives us a cube number. This concept is not only fun but also very useful, especially when we talk about shapes and spaces in geometry.
In geometry, to find out how much space is inside a cube, you multiply the side lengths together. But since all sides of a cube are the same, you just take the length of one side and multiply it by itself three times. This is written as a3, where a is the side length. So, the space inside a cube is a special kind of number called a cube number.
ubing a number means multiplying that number by itself twice. It’s a simple process that involves taking any given number and using it in a multiplication equation three times. Here’s how you do it:
So, when you cube the number 4, you get 64. This is represented mathematically as 4³ or 4×4×4 =64.
To cube a number is to use it in a multiplication three times, leading to results like 2³ or 2×2×2 =8 and 3³ or 3×3×3 =27. It’s a handy math tool, especially in geometry and when working with volumes.
Cubing a fraction involves raising it to the power of three, just like you would cube a whole number. This process multiplies the fraction by itself twice more, affecting both the numerator (the top number) and the denominator (the bottom number). The result is a new fraction that represents the original fraction cubed. Here’s how it works in detail:
Let’s cube the fraction ¾.
Cubing a negative number means multiplying the negative number by itself three times. When you do this with a negative number, the result is always a negative number. This happens because multiplying two negative numbers together gives you a positive result, but then multiplying that positive result by another negative number turns it back into a negative.
Let’s cube -3:
So, the cube of -3 is -27.

| Number | Cubed | Cube Number | Number | Cubed | Cube Number |
|---|---|---|---|---|---|
| 1 | 1³ | 1 | 26 | 26³ | 17,576 |
| 2 | 2³ | 8 | 27 | 27³ | 19,683 |
| 3 | 3³ | 27 | 28 | 28³ | 21,952 |
| 4 | 4³ | 64 | 29 | 29³ | 24,389 |
| 5 | 5³ | 125 | 30 | 30³ | 27,000 |
| 6 | 6³ | 216 | 31 | 31³ | 29,791 |
| 7 | 7³ | 343 | 32 | 32³ | 32,768 |
| 8 | 8³ | 512 | 33 | 33³ | 35,937 |
| 9 | 9³ | 729 | 34 | 34³ | 39,304 |
| 10 | 10³ | 1,000 | 35 | 35³ | 42,875 |
| 11 | 11³ | 1,331 | 36 | 36³ | 46,656 |
| 12 | 12³ | 1,728 | 37 | 37³ | 50,653 |
| 13 | 13³ | 2,197 | 38 | 38³ | 54,872 |
| 14 | 14³ | 2,744 | 39 | 39³ | 59,319 |
| 15 | 15³ | 3,375 | 40 | 40³ | 64,000 |
| 16 | 16³ | 4,096 | 41 | 41³ | 68,921 |
| 17 | 17³ | 4,913 | 42 | 42³ | 74,088 |
| 18 | 18³ | 5,832 | 43 | 43³ | 79,507 |
| 19 | 19³ | 6,859 | 44 | 44³ | 85,184 |
| 20 | 20³ | 8,000 | 45 | 45³ | 91,125 |
| 21 | 21³ | 9,261 | 46 | 46³ | 97,336 |
| 22 | 22³ | 10,648 | 47 | 47³ | 103,823 |
| 23 | 23³ | 12,167 | 48 | 48³ | 110,592 |
| 24 | 24³ | 13,824 | 49 | 49³ | 117,649 |
| 25 | 25³ | 15,625 | 50 | 50³ | 125,000 |
Cube numbers get their name because they help us figure out how much space is inside a 3D shape called a cube, where all sides are the same size. To find a cube’s volume, you multiply the length of one side by itself twice, which is also known as “cubing.”
Since all sides of a cube are equal, finding the volume is easy – just cube the length of one side!
For instance, if a cube’s side is 2 inches long, its volume is 8 cubic inches (because 2 x 2 x 2 = 8). Similarly, if a cube’s volume is 27 cubic inches, each side is 3 inches long (since 3 x 3 x 3 = 27).
Imagine pictures that show these examples of cube numbers to understand better.
Cube numbers, or cubed numbers, are an integral part of mathematical learning, offering a foundational understanding that connects to various areas of mathematics. By delving into how cube numbers relate to other areas, students can appreciate the interconnectedness of mathematical concepts and enhance their problem-solving skills.
Cube numbers play a crucial role in algebra, especially in understanding polynomials and equations. They help in the comprehension of exponentiation, where numbers are raised to powers, a fundamental concept in algebraic expressions and functions. For example, solving cubic equations, which include terms raised to the third power, directly involves cube numbers. This knowledge is also pivotal in exploring patterns and sequences, including geometric sequences where each term is a certain multiple of the previous one.
In geometry, cube numbers are essential in calculating the volume of cubic shapes. Understanding the volume of a cube, where all sides are equal, directly applies the concept of cubing a number. This extends to more complex geometric problems involving cuboids or other three-dimensional shapes where dimensions are multiplied to find volume, reinforcing spatial reasoning and the practical application of cube numbers.
Cube numbers intersect with number theory in the study of perfect cubes and their properties. Investigating patterns within cube numbers, such as the sum of consecutive odd numbers forming a cube, enriches students’ understanding of number properties and relationships. Furthermore, number theory explores the uniqueness of cube numbers, including problems related to Fermat’s Last Theorem, which involves cubes among other powers.
In calculus, cube numbers find their application in understanding the behavior of functions, especially when dealing with cubic functions. Derivatives and integrals involving cubic functions or powers demonstrate how changes in volume can be modeled and analyzed, providing a real-world application of cube numbers in measuring rates of change and accumulation.
Cube numbers can also be seen in probability and statistics, albeit indirectly. For example, when calculating the volume of spaces or understanding geometric probabilities, cube numbers and their principles may be applied. They also appear in statistical formulas that involve cubic computations or models that describe three-dimensional space.
Beyond the classroom, cube numbers are utilized in various real-world contexts, such as physics, engineering, and computer science. For instance, in physics, understanding the concept of density involves cubing units of length to calculate volume, which is then used to determine the mass of an object per unit volume. In computer science, algorithms that involve three-dimensional data processing may use cube numbers to optimize computations related to volume or spatial configurations.
| Aspect | Cube Numbers | Square Numbers |
|---|---|---|
| Definition | Cube numbers are the result of a number multiplied by itself twice more (n x n x n). | Square numbers are the result of a number multiplied by itself (n x n). |
| Notation | Expressed as n³. | Expressed as n². |
| Geometric Representation | Correspond to the volume of a cube (three-dimensional). | Correspond to the area of a square (two-dimensional). |
| Example | 8 is a cube number because 2 x 2 x 2 = 8. | 4 is a square number because 2 x 2 = 4. |
| Sequence | Starts as 1, 8, 27, 64, 125 (for the numbers 1, 2, 3, 4, 5 cubed respectively). | Starts as 1, 4, 9, 16, 25 (for the numbers 1, 2, 3, 4, 5 squared respectively). |
| Impact of Negative Numbers | Cubing a negative number results in a negative cube number (-2 x -2 x -2 = -8). | Squaring a negative number results in a positive square number (-2 x -2 = 4). |
| Visual Example | A cube with sides of length n. Each side’s length is raised to the power of three to calculate volume. | A square with sides of length n. Each side’s length is squared to calculate area. |

Cube numbers are special numbers that you get when you multiply a number by itself twice. It’s like taking a number and using it three times in a multiplication. For example, if we think about building a cube out of blocks, the total number of blocks used gives us a cube number. This concept is not only fun but also very useful, especially when we talk about shapes and spaces in geometry.
Cube numbers are numbers you get when you multiply a number by itself twice more, making it three times in total. It’s like saying a number is used three times in a multiplication. For example, if you take 2 and multiply it by itself twice (2 x 2 x 2), you end up with 8, which is a cube number. This process is also called “cubing” a number, and the result, such as 8 in this case, is referred to as “two cubed.” Cube numbers can be positive or negative because multiplying three negatives or three positives together follows the same rule.
2³ = 2 × 2 × 2 = 8
3³ = 3 × 3 × 3 = 27
4³ = 4 × 4 × 4 = 64
5³ = 5 × 5 × 5 = 125
In geometry, to find out how much space is inside a cube, you multiply the side lengths together. But since all sides of a cube are the same, you just take the length of one side and multiply it by itself three times. This is written as a3, where a is the side length. So, the space inside a cube is a special kind of number called a cube number.
ubing a number means multiplying that number by itself twice. It’s a simple process that involves taking any given number and using it in a multiplication equation three times. Here’s how you do it:
Pick a Number: Start with any number you want to cube. Let’s say you choose the number 4.
Multiply It by Itself: Take that number and multiply it by itself: 4 x 4 = 16.
Multiply One More Time: Now, take the result and multiply it by the original number again: 16 x 4 = 64.
So, when you cube the number 4, you get 64. This is represented mathematically as 4³ or 4×4×4 =64.
To cube a number is to use it in a multiplication three times, leading to results like 2³ or 2×2×2 =8 and 3³ or 3×3×3 =27. It’s a handy math tool, especially in geometry and when working with volumes.
Cubing a fraction involves raising it to the power of three, just like you would cube a whole number. This process multiplies the fraction by itself twice more, affecting both the numerator (the top number) and the denominator (the bottom number). The result is a new fraction that represents the original fraction cubed. Here’s how it works in detail:
Identify the Fraction: Start with your fraction, which has a numerator and a denominator, like ½.
Multiply the Numerator by Itself Three Times: Take the numerator and multiply it by itself twice more. For example, if your fraction is ½, multiply 1 (numerator) by itself three times (1 x 1 x 1), which equals 1.
Multiply the Denominator by Itself Three Times: Similarly, take the denominator and multiply it by itself twice more. Using ½ as an example, multiply 2 (denominator) by itself three times (2 x 2 x 2), which equals 8.
Combine the Results: The result of the numerator’s multiplication becomes the new numerator, and the result of the denominator’s multiplication becomes the new denominator. Therefore, cubing ½ results in 1/8.
Let’s cube the fraction ¾.
Cubing the numerator: 3 x 3 x 3 = 27.
Cubing the denominator: 4 x 4 x 4 = 64.
Combining the results gives you the fraction 27/64, which is the cube of ¾.
Cubing a negative number means multiplying the negative number by itself three times. When you do this with a negative number, the result is always a negative number. This happens because multiplying two negative numbers together gives you a positive result, but then multiplying that positive result by another negative number turns it back into a negative.
First Step: Multiply the negative number by itself once. (-n) × (-n) = positive result.
Second Step: Multiply that positive result by the negative number again. Positive result × (-n) = negative result.
Let’s cube -3:
First, -3 × -3 = 9 (since a negative times a negative gives a positive).
Then, 9 × -3 = -27.
So, the cube of -3 is -27.
Number | Cubed | Cube Number | Number | Cubed | Cube Number |
|---|---|---|---|---|---|
1 | 1³ | 1 | 26 | 26³ | 17,576 |
2 | 2³ | 8 | 27 | 27³ | 19,683 |
3 | 3³ | 27 | 28 | 28³ | 21,952 |
4 | 4³ | 64 | 29 | 29³ | 24,389 |
5 | 5³ | 125 | 30 | 30³ | 27,000 |
6 | 6³ | 216 | 31 | 31³ | 29,791 |
7 | 7³ | 343 | 32 | 32³ | 32,768 |
8 | 8³ | 512 | 33 | 33³ | 35,937 |
9 | 9³ | 729 | 34 | 34³ | 39,304 |
10 | 10³ | 1,000 | 35 | 35³ | 42,875 |
11 | 11³ | 1,331 | 36 | 36³ | 46,656 |
12 | 12³ | 1,728 | 37 | 37³ | 50,653 |
13 | 13³ | 2,197 | 38 | 38³ | 54,872 |
14 | 14³ | 2,744 | 39 | 39³ | 59,319 |
15 | 15³ | 3,375 | 40 | 40³ | 64,000 |
16 | 16³ | 4,096 | 41 | 41³ | 68,921 |
17 | 17³ | 4,913 | 42 | 42³ | 74,088 |
18 | 18³ | 5,832 | 43 | 43³ | 79,507 |
19 | 19³ | 6,859 | 44 | 44³ | 85,184 |
20 | 20³ | 8,000 | 45 | 45³ | 91,125 |
21 | 21³ | 9,261 | 46 | 46³ | 97,336 |
22 | 22³ | 10,648 | 47 | 47³ | 103,823 |
23 | 23³ | 12,167 | 48 | 48³ | 110,592 |
24 | 24³ | 13,824 | 49 | 49³ | 117,649 |
25 | 25³ | 15,625 | 50 | 50³ | 125,000 |
Cube numbers get their name because they help us figure out how much space is inside a 3D shape called a cube, where all sides are the same size. To find a cube’s volume, you multiply the length of one side by itself twice, which is also known as “cubing.”
Since all sides of a cube are equal, finding the volume is easy – just cube the length of one side!
For instance, if a cube’s side is 2 inches long, its volume is 8 cubic inches (because 2 x 2 x 2 = 8). Similarly, if a cube’s volume is 27 cubic inches, each side is 3 inches long (since 3 x 3 x 3 = 27).
Imagine pictures that show these examples of cube numbers to understand better.
Cube numbers are usually introduced to students in 4th to 5th grade.
The concept helps students understand the process of multiplying a number by itself twice, which is essential for grasping volume concepts.
The exact timing of introduction can vary depending on the curriculum and the educational approach adopted by schools or regions.
Learning cube numbers enhances spatial awareness, as students learn about volume and geometry.
It also improves numerical fluency, as students practice computing and recognizing cube numbers.
Introducing cube numbers prepares students for algebra, familiarizing them with the concept of numbers raised to powers.
Students develop problem-solving skills by applying the concept of cubing to mathematical and real-world problems.
Cube numbers, or cubed numbers, are an integral part of mathematical learning, offering a foundational understanding that connects to various areas of mathematics. By delving into how cube numbers relate to other areas, students can appreciate the interconnectedness of mathematical concepts and enhance their problem-solving skills.
Cube numbers play a crucial role in algebra, especially in understanding polynomials and equations. They help in the comprehension of exponentiation, where numbers are raised to powers, a fundamental concept in algebraic expressions and functions. For example, solving cubic equations, which include terms raised to the third power, directly involves cube numbers. This knowledge is also pivotal in exploring patterns and sequences, including geometric sequences where each term is a certain multiple of the previous one.
In geometry, cube numbers are essential in calculating the volume of cubic shapes. Understanding the volume of a cube, where all sides are equal, directly applies the concept of cubing a number. This extends to more complex geometric problems involving cuboids or other three-dimensional shapes where dimensions are multiplied to find volume, reinforcing spatial reasoning and the practical application of cube numbers.
Cube numbers intersect with number theory in the study of perfect cubes and their properties. Investigating patterns within cube numbers, such as the sum of consecutive odd numbers forming a cube, enriches students’ understanding of number properties and relationships. Furthermore, number theory explores the uniqueness of cube numbers, including problems related to Fermat’s Last Theorem, which involves cubes among other powers.
In calculus, cube numbers find their application in understanding the behavior of functions, especially when dealing with cubic functions. Derivatives and integrals involving cubic functions or powers demonstrate how changes in volume can be modeled and analyzed, providing a real-world application of cube numbers in measuring rates of change and accumulation.
Cube numbers can also be seen in probability and statistics, albeit indirectly. For example, when calculating the volume of spaces or understanding geometric probabilities, cube numbers and their principles may be applied. They also appear in statistical formulas that involve cubic computations or models that describe three-dimensional space.
Beyond the classroom, cube numbers are utilized in various real-world contexts, such as physics, engineering, and computer science. For instance, in physics, understanding the concept of density involves cubing units of length to calculate volume, which is then used to determine the mass of an object per unit volume. In computer science, algorithms that involve three-dimensional data processing may use cube numbers to optimize computations related to volume or spatial configurations.
Aspect | Cube Numbers | Square Numbers |
|---|---|---|
Definition | Cube numbers are the result of a number multiplied by itself twice more (n x n x n). | Square numbers are the result of a number multiplied by itself (n x n). |
Notation | Expressed as n³. | Expressed as n². |
Geometric Representation | Correspond to the volume of a cube (three-dimensional). | Correspond to the area of a square (two-dimensional). |
Example | 8 is a cube number because 2 x 2 x 2 = 8. | 4 is a square number because 2 x 2 = 4. |
Sequence | Starts as 1, 8, 27, 64, 125 (for the numbers 1, 2, 3, 4, 5 cubed respectively). | Starts as 1, 4, 9, 16, 25 (for the numbers 1, 2, 3, 4, 5 squared respectively). |
Impact of Negative Numbers | Cubing a negative number results in a negative cube number (-2 x -2 x -2 = -8). | Squaring a negative number results in a positive square number (-2 x -2 = 4). |
Visual Example | A cube with sides of length n. Each side’s length is raised to the power of three to calculate volume. | A square with sides of length n. Each side’s length is squared to calculate area. |
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
What is the cube of 2?
4
6
8
10
What is 3³?
27
30
33
36
What is the result of 5³?
100
115
120
125
Calculate 10³.
900
950
1000
1050
What is 21³?
9200
9261
9300
9400
Calculate the cube of 6.
200
210
216
220
Find the cube of 8.
500
512
520
530
What is the cube of 15?
3300
3375
3400
3450
What is the cube of 5 using repeated multiplication?
115
120
125
130
Find the cube of 3 using a shortcut method.
24
26
27
29
Before you leave, take our quick quiz to enhance your learning!

