Derivative of Cos Square x (Cos^2x)

The derivative of cos⁡²(𝑥) is a fundamental concept in calculus, intertwining with various branches of mathematics. Involving algebra and the power rule, the differentiation process utilizes the chain rule to handle the square of the cosine function, symbolically integral to both rational and irrational numbers. This derivative calculation lays the groundwork for more complex equations in fields such as statistics and numerical methods, including the least squares method. Integer operations and the square and square roots also play a role in understanding the behavior of this trigonometric function across different numerical systems.

What is Derivative of Cos Square x?

The derivative of cos²(𝑥), where the function is squared, is calculated using the chain rule resulting in −2cos(x)sin(x). This expression can also be represented as −sin(2𝑥), demonstrating the application of trigonometric identities in calculus.

Derivative of Cos²x Formula

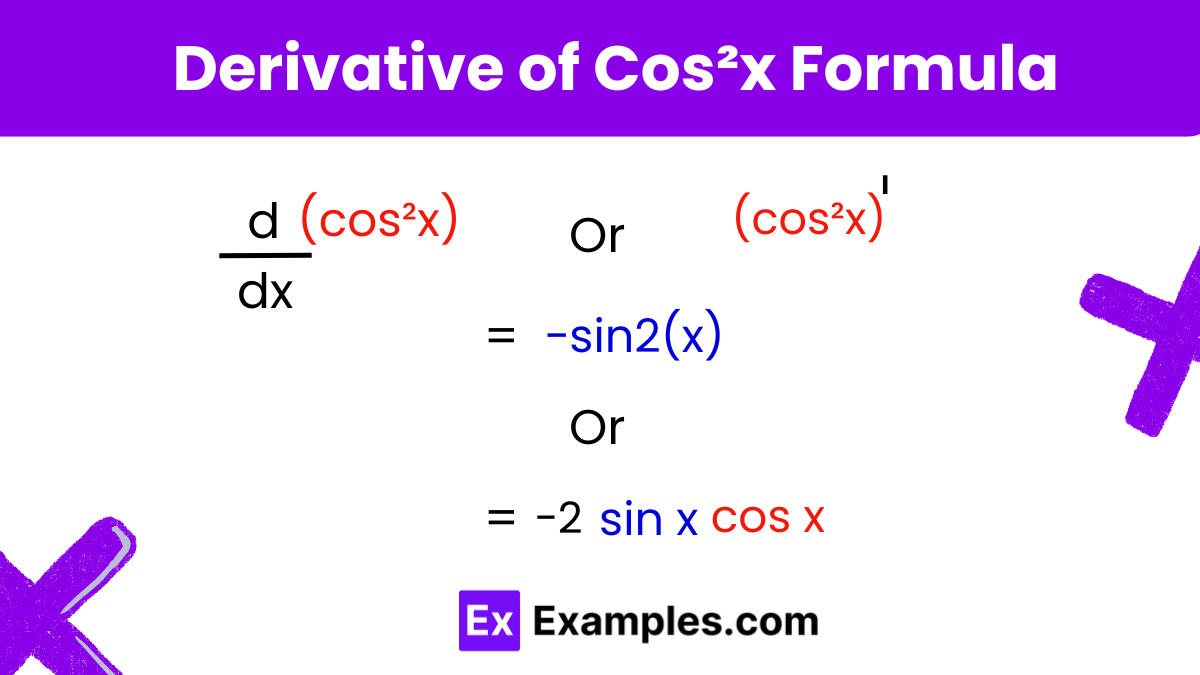
The derivative of cos²(𝑥) is given by the formula:

d/dx(cos²(x)) = −2cos(x)sin(x)

This can also be expressed using the double angle identity for sine:

d/dx(cos²(x)) = −sin(2x)

Derivative of Cos Square x Using the Chain Rule

1. Identify the Outer and Inner Functions:

• Outer function: 𝑢² where 𝑢 is the inner function.
• Inner function: 𝑢 = cos⁡(𝑥).

2. Differentiate the Outer Function:

• Derivative of 𝑢² with respect to 𝑢 is 2𝑢.

3. Differentiate the Inner Function:

• Derivative of cos⁡(𝑥) with respect to 𝑥 is −sin⁡(𝑥).

4. Apply the Chain Rule:

Multiply the derivative of the outer function by the derivative of the inner function:

d//dx(cos²(x)) = −2(cos(x)).(-sin(x))

5. Simplify the Expression:

• This simplifies to −2cos⁡(𝑥)sin⁡(𝑥).

Final Expression

d/dx(cos²(x)) = −2cos(x)sin(x)

Derivative of Cos²x Using the First Principle

To find the derivative of cos⁡²(𝑥) using the first principles of calculus, also known as the definition of the derivative, follow these step-by-step instructions:

Derivative Using First Principle

Formula for Derivative Using First Principle

f'(x) = lim​ₕ→₀f(x+h)−f(x)​/h

Apply to cos²2(𝑥):

Function Definition:

𝑓(𝑥) = cos²(𝑥)

Function Value at 𝑥+ℎ:

𝑓(𝑥+ℎ) = cos²(𝑥+ℎ)

Difference Quotient:

cos⁡²(𝑥+ℎ)−cos⁡2(𝑥)/ℎ

Simplify Using Trigonometric Identities:

Use the cosine addition formula: cos⁡(𝑥+ℎ) = cos⁡(𝑥)cos⁡(ℎ)−sin⁡(𝑥)sin⁡(ℎ)

Then apply cos²(𝑥+ℎ) = (cos⁡(𝑥)cos⁡(ℎ)−sin⁡(𝑥)sin⁡(ℎ))²

Expand and Simplify:

Due to complexity, this involves expanding (cos⁡(𝑥)cos⁡(ℎ)−sin⁡(𝑥)sin⁡(ℎ))² and then simplifying under the assumption that ℎ is very small, so cos⁡(ℎ) = 1 and sin⁡(ℎ) = ℎ.

Apply Limits:

Simplify the expanded expression and apply the limit ℎ→0. The terms involving ℎ will approach zero, leaving terms that contribute to the derivative.

Computation

Continuing the computation:

lim​ₕ→₀(cos⁡(𝑥)cos⁡(ℎ)−sin⁡(𝑥)sin⁡(ℎ))²−cos⁡²(𝑥)/ℎ

Expanding and substituting cos⁡(ℎ) = 1 and sin⁡(ℎ) = ℎ:

lim​ₕ→₀(cos⁡(𝑥)⋅1−sin⁡(𝑥)⋅ℎ)²−cos⁡²(𝑥)/ℎ

=lim​ₕ→₀(cos⁡²(𝑥)−2cos⁡(𝑥)sin⁡(𝑥)ℎ+sin⁡²(𝑥)ℎ²)−cos²𝑥)/ℎ

=lim​ₕ→₀−2cos⁡(𝑥)sin⁡(𝑥)ℎ+sin⁡²(𝑥)ℎ²/ℎ

=lim​ₕ→₀(−2cos⁡(𝑥)sin⁡(𝑥)+sin²(𝑥)ℎ)

=−2cos⁡(𝑥)sin⁡(𝑥)

Final Result

𝑑/𝑑𝑥(cos⁡²(𝑥)) = −2cos⁡(𝑥)sin⁡(𝑥)

Derivative of Cos Square x Using Product Rule

To find the derivative of cos⁡²(x) using the product rule, consider cos⁡²(𝑥) as cos⁡(𝑥)⋅cos⁡(𝑥). The product rule states that if 𝑢 and 𝑣 are functions of 𝑥, then the derivative of their product 𝑢𝑣 is given by 𝑢𝑣′+𝑣𝑢′.

Applying the Product Rule to cos²(x)

Step 1: Define the Functions

• Let 𝑢(𝑥) = cos⁡(𝑥) and 𝑣(𝑥) = cos(x).

Step 2: Differentiate Each Function

• The derivative of 𝑢u with respect to 𝑥 is 𝑢′(𝑥) = −sin⁡(𝑥).
• Similarly, 𝑣′(𝑥) = −sin⁡(𝑥).

Step 3: Apply the Product Rule

• Plug the derivatives into the product rule formula:(cos⁡(𝑥)⋅cos⁡(𝑥))′ = cos⁡(𝑥)(−sin⁡(𝑥))+(−sin⁡(𝑥))cos⁡(𝑥)
• Simplify the expression:−sin⁡(𝑥)cos⁡(𝑥)−sin⁡(𝑥)cos⁡(𝑥) = −2sin⁡(𝑥)cos⁡(𝑥)

Final Expression

𝑑/𝑑𝑥(cos⁡²(𝑥)) = −2cos⁡(𝑥)sin⁡(𝑥)

Derivative of Cos Square x Examples

Example 1

Calculate 𝑑/𝑑𝑥[cos⁡⁡²(𝑥)] when 𝑥 = 𝜋/4​.

• Substituting 𝑥 = 𝜋/4 into the derivative, we use −sin⁡(2𝑥): −sin⁡(2×𝜋/4)=−sin⁡(𝜋/2)=−1
• Thus, the derivative at 𝑥 = 𝜋/4 is −1.

Example 2

Evaluate the rate of change of cos⁡²(𝑥) at 𝑥 = 𝜋.

• Using the derivative formula −sin⁡(2𝑥): −sin⁡(2×𝜋)=−sin⁡(2𝜋)=0
• Therefore, the derivative at 𝑥 = 𝜋 is 0, indicating no rate of change at this point.

Example 2

Find the derivative of cos²(𝑥) at 𝑥 = 3𝜋/2​.

• Substituting 𝑥 = 3𝜋/2​ into the derivative formula, we get: −sin⁡(2×3𝜋/2) = −sin⁡(3𝜋) = 0
• Again, the derivative is 0, showing no rate of change at 𝑥 = 3𝜋/2​.

FAQs

Why do we use the double angle identity in finding the derivative of cos²(x)?

The double angle identity (sin⁡(2𝑥)=2sin⁡(𝑥)cos⁡(𝑥) is used to simplify the expression derived from the chain rule. By recognizing that −2cos⁡(𝑥)sin⁡(𝑥) can be rewritten as −sin⁡(2𝑥), the derivative not only becomes easier to compute but also more concise, facilitating further calculations and integrations.

How is the derivative of cos⁡²(x) used in practical applications?

The derivative of cos⁡⁡²(x) is particularly useful in fields such as physics and engineering, where it helps analyze phenomena that involve oscillations and wave patterns, such as sound waves and light waves. It allows researchers and professionals to determine the rate of change of these waves at any given point, which is crucial for designing systems that operate efficiently under wave-based mechanisms.

Is there a general method to find derivatives of squared trigonometric functions?

Yes, a general method to find derivatives of squared trigonometric functions involves using the chain rule. First, take the derivative of the trigonometric function itself, then multiply it by the derivative of the outer function, which in cases of squared functions is 2 times the original function. Applying this method systematically will yield the derivative for any squared trigonometric function.