Divisibility Rule of 13

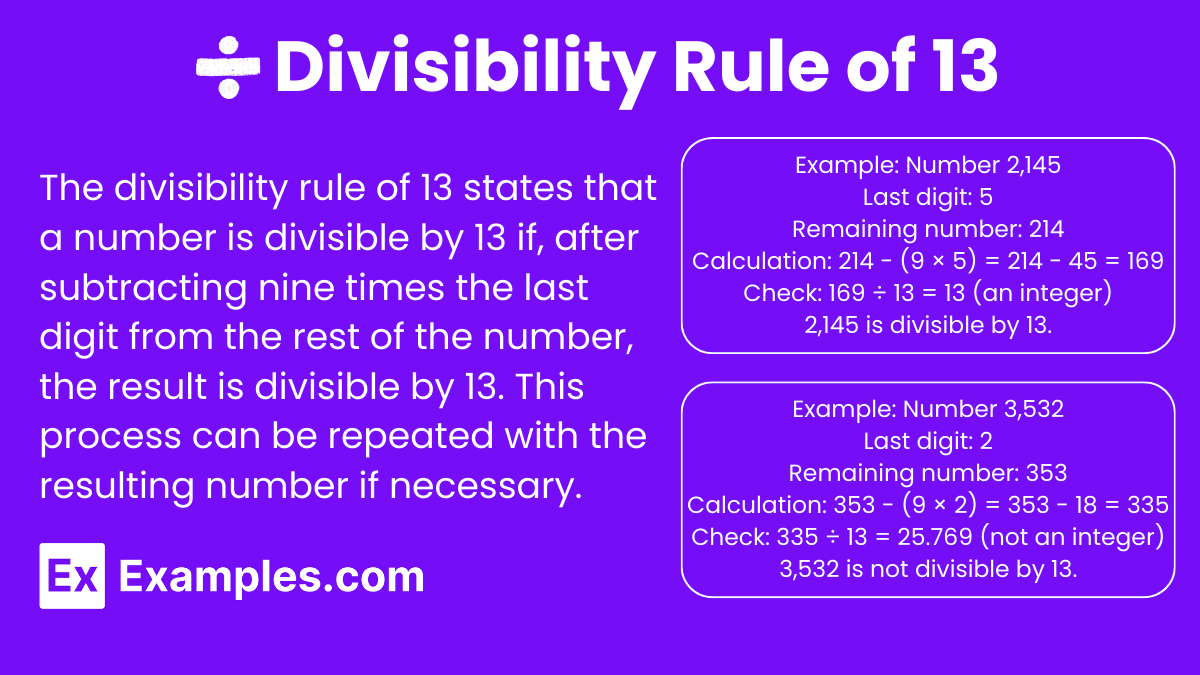
The divisibility rule of 13 is a method to determine if a number is divisible by 13 without performing the division. To check, subtract nine times the last digit from the rest of the number; if the result is divisible by 13, then the original number is too. Understanding divisibility rules is crucial in mathematics, especially in topics like rational and irrational numbers, algebra, integers, addition, subtraction, multiplication, and division. These concepts form the foundation for solving more complex problems and improving numerical fluency.

Download Proof of the Divisibility Rule of 13 in PDF

What is the Divisibility Rule of 13?

To determine if a number is divisible by 13, take the last digit of the number, multiply it by 9, and subtract this product from the rest of the number. If the result is divisible by 13, then the original number is also divisible by 13.

Proof of the Divisibility Rule of 13

Download Proof of the Divisibility Rule of 13 in PDF

Step 1: Consider a Number

Start with a number, for example, 728.

Step 2: Separate the Last Digit

Isolate the last digit. Here, the last digit is 8.

Step 3: Multiply the Last Digit by 9

Multiply the isolated last digit by 9. For 8, we get: 8×9 = 728

Step 4: Subtract This Product from the Rest of the Number

Subtract the result from the remaining part of the original number. Remove the last digit and subtract the product: 72−72 = 0.

Step 5: Check the Result for Divisibility by 13

Check if the resulting number is divisible by 13. In this case, 0 is divisible by 13.

Step 6: Conclusion for This Example

Since the resulting number (0) is divisible by 13, the original number (728) is also divisible by 13.

Step 7: Generalize the Rule

To generalize, if the number is n, separate the last digit d, then the remaining part m. Calculate: m−9d

Step 8: Verify with Divisibility by 13

If the result from step 7 is divisible by 13, then the original number n is divisible by 13. This completes the proof of the divisibility rule of 13.

Divisibility Rule of 13 and 14

Divisibility Rule of 13

To determine if a number is divisible by 13, follow these steps:

• Separate the Last Digit: Take the last digit of the number.
• Multiply by 9: Multiply this last digit by 9.
• Subtract from Remaining Number: Subtract the result from the rest of the number.
• Check for Divisibility: If the resulting number is divisible by 13, then the original number is divisible by 13.

Example:

Consider the number 351:

1. Last digit: 1
2. Multiply by 9: 1×9 = 9
3. Subtract from the rest of the number: 35−9 = 26
4. Check divisibility: 26 is divisible by 13 (26 ÷ 13 = 2).

Thus, 351 is divisible by 13.

Divisibility Rule of 14

To determine if a number is divisible by 14, follow these steps:

1. Divisibility by 2: Check if the number is even. If it is not even, it is not divisible by 14.
2. Divisibility by 7: Check if the number is divisible by 7.

Example:

Consider the number 98:

Check Divisibility by 2: 98 is even.

Check Divisibility by 7:

• Double the last digit and subtract it from the rest of the number: 9−2×8=9−16=−7.
• Since -7 is divisible by 7, 98 is also divisible by 7.

Since 98 is divisible by both 2 and 7, it is divisible by 14.

Divisibility Test of 13 and 17

Divisibility Test of 13

• Remove the last digit of the number.
• Multiply the last digit by 9.
• Subtract this product from the remaining truncated number.
• If the result is divisible by 13, then the original number is also divisible by 13.

Example: To check if 2,366 is divisible by 13:

• Remove the last digit (6), leaving 236.
• Multiply the last digit by 9: 6 × 9 = 54.
• Subtract 54 from 236: 236 – 54 = 182.
• Check if 182 is divisible by 13: 182 ÷ 13 = 14.

Since 182 is divisible by 13, 2,366 is also divisible by 13.

Divisibility Test of 17

• Remove the last digit of the number.
• Multiply the last digit by 5.
• Subtract this product from the remaining truncated number.
• If the result is divisible by 17, then the original number is also divisible by 17.

Example: To check if 3,246 is divisible by 17:

• Remove the last digit (6), leaving 324.
• Multiply the last digit by 5: 6 × 5 = 30.
• Subtract 30 from 324: 324 – 30 = 294.
• Check if 294 is divisible by 17: 294 ÷ 17 = 17.29 (not a whole number).

Since 294 is not divisible by 17, 3,246 is also not divisible by 17.

Divisibility Rule of 13 Examples

Example 1: Checking 2,366

• Remove the last digit: The number becomes 236.
• Multiply the last digit by 9: 6×9 = 54.
• Subtract this product from the truncated number: 236−54 = 182.
• Check if the result is divisible by 13: 182÷13 = 14.

Since 182 is divisible by 13, 2,366 is also divisible by 13.

Example 2: Checking 4,713

• Remove the last digit: The number becomes 471.
• Multiply the last digit by 9: 3×9 = 27.
• Subtract this product from the truncated number: 471−27 = 444.
• Check if the result is divisible by 13: 444÷13 = 34.15.

Since 444 is not divisible by 13, 4,713 is not divisible by 13.

Example 3: Checking 1,092

• Remove the last digit: The number becomes 109.
• Multiply the last digit by 9: 2×9 = 18.
• Subtract this product from the truncated number: 109−18 = 91.
• Check if the result is divisible by 13: 91÷13 = 7.

Since 91 is divisible by 13, 1,092 is also divisible by 13.

Example 4: Checking 5,101

• Remove the last digit: The number becomes 510.
• Multiply the last digit by 9: 1×9 = 9.
• Subtract this product from the truncated number: 510−9 = 501.
• Check if the result is divisible by 13: 501÷13 = 38.54.

Since 501 is not divisible by 13, 5,101 is not divisible by 13.

Example 5: Checking 3,859

• Remove the last digit: The number becomes 385.
• Multiply the last digit by 9: 9×9 = 81.
• Subtract this product from the truncated number: 385−81 = 304.
• Check if the result is divisible by 13: 304÷13 = 23.38.

Since 304 is not divisible by 13, 3,859 is not divisible by 13.

FAQs