Which of the following numbers is divisible by 9?
123
124
125
126
of 10
Divisibility Rule of 9 – Examples, Proof, Methods, What is Divisibility Rule of 9
Divisibility Rule of 9 – Examples, Proof, Methods, What is Divisibility Rule of 9
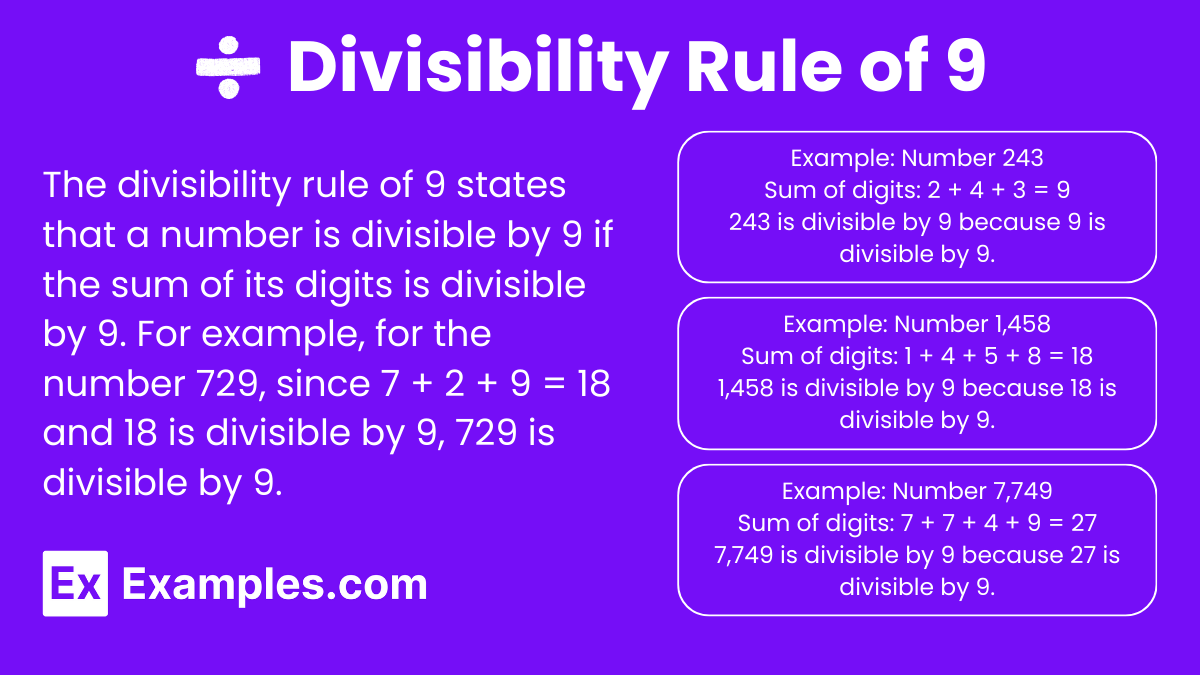
The divisibility rule of 9 is a handy mathematical tool used to determine if a number is divisible by 9 without performing division. To apply this rule, sum the digits of the number in question. If the resulting sum is divisible by 9, then the original number is also divisible by 9. This concept is essential in various branches of mathematics, including rational and irrational numbers, algebra, integers, and operations like addition, subtraction, multiplication, and division. Understanding these rules simplifies complex calculations and aids in number theory analysis.
https://images.examples.com/wp-content/uploads/2024/05/Proof-of-Divisibility-Rule-of-9.png
What is the Divisibility Rule of 9?
Proof of the Divisibility Rule of 9
https://images.examples.com/wp-content/uploads/2024/05/Proof-of-Divisibility-Rule-of-9.png
Let’s prove the divisibility rule of 9 using the number 5724 as an example.
- Express the Number: 5724 = 5×1000+7×100+2×10+4×1
- Rewrite Each Term: 5724 = 5×(999+1)+7×(99+1)+2×(9+1)+4×1
- Distribute Each Multiplication: = (5×999+5×1)+(7×99+7×1)+(2×9+2×1)+4×1
- Group the Multiples of 9: = (5×999+7×99+2×9)+(5×1+7×1+2×1+4×1)
- Simplify the Grouped Terms: = (5×999+7×99+2×9)+(5+7+2+4)
- Identify the Sum of the Digits: = (5×999+7×99+2×9)+18
- Recognize Multiples of 9: The terms 5×9995×999, 7×997×99, and 2×92×9 are all multiples of 9, and thus divisible by 9.
- Determine Divisibility by 9: The sum of the digits 5+7+2+4 = 18, which is divisible by 9. Therefore, the original number 5724 is divisible by 9.
Divisibility rule of 3 and 9
Divisibility Rule of 3
The divisibility rule of 3 states that a number is divisible by 3 if the sum of its digits is divisible by 3. Here’s how you can check:
- Add the Digits: Sum the digits of the number.
- Check the Sum: Determine if this sum is divisible by 3.
- Conclusion: If the sum is divisible by 3, then the original number is also divisible by 3.
Example:
- For the number 123, sum the digits: 1 + 2 + 3 = 6.
- Since 6 is divisible by 3, 123 is also divisible by 3.
Divisibility Rule of 9
The divisibility rule of 9 states that a number is divisible by 9 if the sum of its digits is divisible by 9. Here’s how you can check:
- Add the Digits: Sum the digits of the number.
- Check the Sum: Determine if this sum is divisible by 9.
- Conclusion: If the sum is divisible by 9, then the original number is also divisible by 9.
Example:
- For the number 729, sum the digits: 7 + 2 + 9 = 18.
- Since 18 is divisible by 9, 729 is also divisible by 9.
Divisibility Rule of 9 and 11
Divisibility Rule of 9
The divisibility rule of 9 states that a number is divisible by 9 if the sum of its digits is divisible by 9. Here’s the step-by-step process to check:
- Add the Digits: Sum all the digits of the number.
- Check the Sum: Determine if the resulting sum is divisible by 9.
- Conclusion: If the sum is divisible by 9, then the original number is also divisible by 9.
Example:
- For the number 567, sum the digits: 5 + 6 + 7 = 18.
- Since 18 is divisible by 9, 567 is also divisible by 9.
Divisibility Rule of 11
The divisibility rule of 11 states that a number is divisible by 11 if the difference between the sum of the digits in odd positions and the sum of the digits in even positions is either 0 or divisible by 11. Here’s how to check:
- Identify the Positions: Identify the positions of each digit (from right to left, the rightmost digit is in position 1).
- Sum the Odd-Position Digits: Add the digits in the odd positions.
- Sum the Even-Position Digits: Add the digits in the even positions.
- Find the Difference: Calculate the absolute difference between the sums obtained in steps 2 and 3.
- Conclusion: If the difference is 0 or divisible by 11, then the original number is also divisible by 11.
Example:
- For the number 121, sum the digits in odd positions (1st and 3rd): 1 + 1 = 2.
- Sum the digits in even positions (2nd): 2.
- Find the difference: |2 – 2| = 0.
- Since the difference is 0, 121 is divisible by 11.
Divisibility Rule of 9 Examples
Example 1: 729
- Sum the Digits: 7 + 2 + 9 = 18
- Check the Sum: 18 is divisible by 9
- Conclusion: 729 is divisible by 9
Example 2: 4563
- Sum the Digits: 4 + 5 + 6 + 3 = 18
- Check the Sum: 18 is divisible by 9
- Conclusion: 4563 is divisible by 9
Example 3: 81
- Sum the Digits: 8 + 1 = 9
- Check the Sum: 9 is divisible by 9
- Conclusion: 81 is divisible by 9
Example 4: 9999
- Sum the Digits: 9 + 9 + 9 + 9 = 36
- Check the Sum: 36 is divisible by 9
- Conclusion: 9999 is divisible by 9
Example 5: 2025
- Sum the Digits: 2 + 0 + 2 + 5 = 9
- Check the Sum: 9 is divisible by 9
- Conclusion: 2025 is divisible by 9
FAQs
How can I quickly check if a number is divisible by 9?
To quickly check if a number is divisible by 9, add up all the digits of the number. If the sum is divisible by 9, then the original number is also divisible by 9.
Can you provide an example of the divisibility rule of 9?
Sure! For the number 729, sum the digits: 7 + 2 + 9 = 18. Since 18 is divisible by 9, the number 729 is also divisible by 9.
Is there a shortcut to determining divisibility by 9 for large numbers?
Yes, the shortcut is to add the digits of the large number repeatedly until you get a single digit. If this single digit is 9, then the original number is divisible by 9.
Does the divisibility rule of 9 apply to negative numbers?
Yes, the divisibility rule of 9 applies to negative numbers. Simply sum the absolute values of the digits, and if the sum is divisible by 9, then the negative number is also divisible by 9.
Can the divisibility rule of 9 be used in algebraic expressions?
Yes, the rule can be used in algebraic expressions where the coefficients sum to a number that is divisible by 9.
How does the divisibility rule of 9 help in simplifying mathematical calculations?
The rule helps in quickly determining factors of numbers and can be used to simplify large arithmetic problems by breaking them into smaller, more manageable parts.
Is the divisibility rule of 9 related to the rule of 3?
Yes, both rules are similar in that they involve summing the digits of a number. However, for 9, the sum must be divisible by 9, while for 3, the sum must be divisible by 3.
What are some real-world applications of the divisibility rule of 9?
The rule is useful in number theory, cryptography, coding theory, and error detection in identification numbers like bank account numbers and credit card numbers.
Can the divisibility rule of 9 be extended to other bases besides 10?
Yes, the rule can be extended to other bases. In base-N, a number is divisible by N−1 if the sum of its digits in that base is divisible by N−1. For example, in base 8, a number is divisible by 7 if the sum of its digits is divisible by 7.
Share :
Practice Test
Which number is not divisible by 9?
441
333
513
555
of 10
What is the sum of the digits of 198?
18
19
20
21
of 10
Which number is divisible by 9?
3456
2345
1245
5432
of 10
What is the sum of the digits of 891?
18
9
12
15
of 10
Which of these numbers is not divisible by 9?
180
999
162
252
of 10
How do you determine if a number is divisible by 9?
The number must end in 0 or 5
The sum of its digits must be divisible by 9
The number must be even
The number must end in 9
of 10
Which of the following numbers is divisible by 9?
1234
987
5678
2345
of 10
Which of these numbers is divisible by 9?
150
216
345
678
of 10
What is the sum of the digits of 324?
9
10
11
12
of 10