What is the value of in the equation 2x + 5 = 15?
3
5
10
15


An equation is a mathematical statement that asserts the equality of two expressions. It consists of two parts, separated by an equal sign (=), which indicate that the values or results on both sides of the sign are equivalent. For example, the equation 3 + 2 = 5 states that the sum of 3 and 2 is equal to 5.
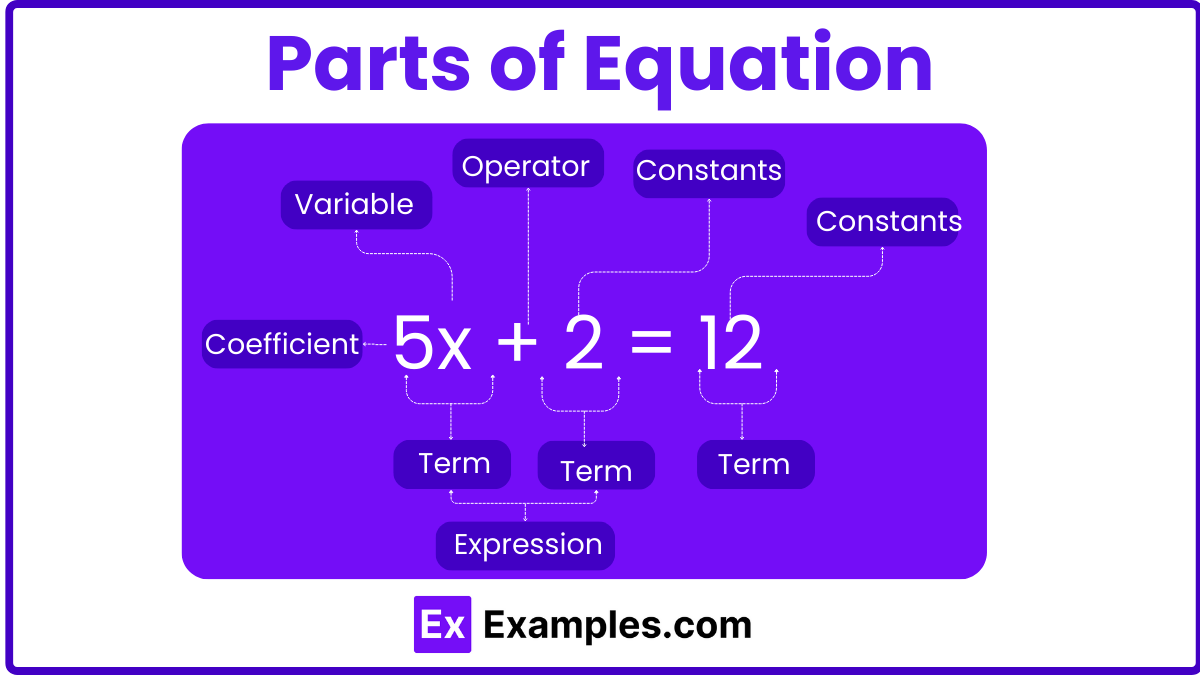
An equation is a mathematical statement that asserts the equality of two expressions. It consists of various parts:
3x + 5 = 8, the number 3 is a coefficient of x.2x + 4 = 10, x is a variable.+), subtraction (-), multiplication (*), and division (/).2x + 4 = 10.2x and 4 are terms in the equation 2x + 4 = 10.2x + 4 = 10 is an expression.=): Indicates the equality between the two expressions on either side of it.Equations can range from simple arithmetic forms, like 5 + 10 = 15, which have no variables, to complex algebraic forms involving variables and multiple terms. An algebraic equation includes at least one variable, like in the equation 2x + 4 = 10
Solving an equation involves finding the value or values that satisfy the equation, making both sides equal. The approach depends on the type of equation. Here are general steps for solving an equation:
3x + 2x = 10, combine the terms to get 5x = 10.5x = 10 by 5 to get x = 2.3x + 2x = 10, substitute x = 2 to see if 3(2) + 2(2) = 10.Equations can be classified into various types based on their structure, degree, and the nature of their variables. Here are some key types of equations:
ax + b = 0 where a and b are constants, and x is a variable. They graph as straight lines and have a degree of one.ax² + bx + c = 0 where a, b, and c are constants, and x is a variable. They graph as parabolas and have a degree of two.aⁿxⁿ + aⁿ⁻¹xⁿ⁻¹ + ... + a¹x + a⁰ = 0 where n is the degree of the polynomial, and each term includes a constant coefficient a and a power of x.aˣ bˣ = c. Solving these often involves logarithmic functions.log_b(x) = y, and can be rewritten into exponential form for easier manipulation.(x+1)/(x-1) = 3.sin(x) = 0.5. Solutions often require knowledge of inverse trigonometric functions or identities.dy/dx = 3x. They describe the relationship between a function and its rate of change, often modeling physical or natural phenomena.x² + y² =>, <, >=, or <= to compare two expressions.A linear equation is a mathematical statement that represents a straight-line relationship between variables. It typically has the form:
𝑦=𝑚𝑥+𝑐
Or, in a more general form:
𝑎𝑥+𝑏𝑦=𝑐
where m represents the slope of the line, c is the y-intercept, and a, b, and c are constants. Here are some key points about linear equations:
m (or -a/b in the general form) defines the slope, indicating how much y changes for each unit change in x.c represents the y-intercept, the point where the line intersects the y-axis.3x + 2y = 6, you can rearrange to solve for y in terms of x or vice versa.A quadratic equation is a type of polynomial equation that has a degree of two, meaning it involves a variable raised to the power of two. The general form of a quadratic equation is:
𝑎𝑥²+𝑏𝑥+𝑐=0
where a, b, and c are constants, and a is not zero. Here are some key characteristics of quadratic equations:
a.𝑥=−𝑏±𝑏²−4𝑎𝑐²𝑎
This formula calculates the roots based on the values of a, b, and c.
A cubic equation is a type of polynomial equation of degree three, meaning it involves a variable raised to the power of three. The general form of a cubic equation is:
𝑎𝑥³+𝑏𝑥²+𝑐𝑥+𝑑=0
where a, b, c, and d are constants, and a is not zero. Here are some key characteristics of cubic equations:
These will give you an idea of the meaning of an equation in math.
| Equation | Is it an equation? | |
|---|---|---|
| 1 | y = 9x – 5 | Yes |
| 2 | y + x²- 8 | No, because there is no ‘equal to’ symbol. |
| 3 | 9 + 2 = 12 - 1 | Yes |
| Aspect | Equation | Expression |
|---|---|---|
| Definition | A mathematical statement that asserts the equality of two expressions, connected by an equal sign (=). | A combination of numbers, variables, and operators that represent a value, but do not assert equality. |
| Components | Consists of two expressions or terms separated by an equal sign (=). | Consists of numbers, variables, and operators without an equal sign. |
| Example | 3x + 2 = 8 | 3x + 2 |
| Purpose | Establishes a relationship between two expressions or values, showing they are equivalent. | Represents a value or combination of values that can be evaluated or simplified. |
| Solving | Can be solved by finding the values of variables that make both sides equal. | Can be simplified by performing arithmetic or algebraic operations. |
| Use | Used in mathematical problems, modeling relationships, and real-world applications to find unknown values. | Often used to create equations or to simplify and evaluate values. |
Equations are a fundamental part of mathematics and have numerous applications across various fields. Here are some key points to consider:
Simplify the equation and solve for 𝑥: 5𝑥−7=3𝑥+5
Answer:
Subtract 3𝑥 from both sides:
2𝑥−7=5
Add 7 to both sides:
2𝑥=12
Divide by 2:
𝑥=6
Solve the following quadratic equation by factoring:
𝑥²−7𝑥+12=0
Answer:
Factor the equation:
(𝑥−3)(𝑥−4)=0
Set each factor equal to zero:
𝑥−3=0 ⇒ 𝑥=3
𝑥−4=0 ⇒ 𝑥=4
Thus, the solutions are:
𝑥=3 or 𝑥=4
Simplify and solve the following equation for 𝑦: 3(𝑦−2)=2𝑦+6
Answer:
Distribute 3:
3𝑦−6=2𝑦+6
Subtract 2𝑦 from both sides:
𝑦−6=6
Add 6 to both sides:
𝑦=12
Solve the equation for 𝑥:
2(𝑥+4)=3𝑥+10
Answer:
Distribute 2:
2𝑥+8=3𝑥+10
Subtract 2𝑥 from both sides:
8=𝑥+10
Subtract 10 from both sides:
−2=𝑥
Thus, 𝑥=−2
Solve for 𝑥: 4𝑥−2(3𝑥−4)=6
Answer:
Distribute the -2:
4𝑥−6𝑥+8=6
Combine like terms:
−2𝑥+8=6
Subtract 8 from both sides:
−2𝑥=−2
Divide by -2:
𝑥=1
An equation is a mathematical statement that asserts equality between two expressions. For example, 3x + 5 = 14 is an equation where both sides are balanced, and solving it involves finding the value of x that makes this equality true.
An equation is a mathematical statement that declares the equality of two expressions, connected by an equal sign (=). It consists of terms, variables, constants, and operators, allowing it to describe mathematical relationships and form the basis for solving various problems.
An equation in math is a formal statement asserting that two expressions are equal. It can range from simple arithmetic equations to complex algebraic forms. Equations play a vital role in modeling relationships, representing mathematical functions, and solving real-world problems.
A simple equation is an equation with straightforward expressions and limited variables or constants, such as 2x + 3 = 7. Solving it involves basic algebraic manipulation to isolate and determine the value of the variable, making both sides equal.
The simplest type of equation is an arithmetic equation, which involves only numbers and arithmetic operators. An example is 5 + 10 = 15, where both sides consist solely of numerical values, and no variables or complex algebraic forms are present

An equation is a mathematical statement that asserts the equality of two expressions. It consists of two parts, separated by an equal sign (=), which indicate that the values or results on both sides of the sign are equivalent. For example, the equation 3 + 2 = 5 states that the sum of 3 and 2 is equal to 5.
An equation is a mathematical statement that asserts the equality of two expressions. It consists of various parts:
Coefficients: Numerical factors that multiply variables in an equation. For instance, in 3x + 5 = 8, the number 3 is a coefficient of x.
Variables: Symbols, usually letters, representing unknown values or quantities that can change. In the equation 2x + 4 = 10, x is a variable.
Operators: Symbols that define the mathematical operations performed on the equation’s terms. Common operators include addition (+), subtraction (-), multiplication (*), and division (/).
Constants: Fixed values that do not change, such as the numbers 4 and 10 in 2x + 4 = 10.
Terms: Individual elements in an equation, which can include variables, constants, or products of these. For example, 2x and 4 are terms in the equation 2x + 4 = 10.
Expressions: Combinations of terms linked by operators. The left side of the equation 2x + 4 = 10 is an expression.
Equal Sign (=): Indicates the equality between the two expressions on either side of it.
Equations can range from simple arithmetic forms, like 5 + 10 = 15, which have no variables, to complex algebraic forms involving variables and multiple terms. An algebraic equation includes at least one variable, like in the equation 2x + 4 = 10
Solving an equation involves finding the value or values that satisfy the equation, making both sides equal. The approach depends on the type of equation. Here are general steps for solving an equation:
Identify the type of equation: Recognizing the type (e.g., linear, quadratic, exponential) helps determine the appropriate solving method.
Simplify both sides: Combine like terms and simplify each side as much as possible. For example, in the equation 3x + 2x = 10, combine the terms to get 5x = 10.
Isolate the variable: The goal is to get the variable on one side of the equation and constants on the other. This may involve performing arithmetic operations like addition, subtraction, multiplication, or division on both sides. For instance, divide both sides of 5x = 10 by 5 to get x = 2.
Check your solution: Substitute the value back into the original equation to ensure both sides are equal. For example, in 3x + 2x = 10, substitute x = 2 to see if 3(2) + 2(2) = 10.
Solve more complex equations: For quadratic equations, you might factorize, complete the square, or use the quadratic formula. For exponential equations, logarithmic functions can be used.
Consider multiple solutions: Some equations, especially quadratic or higher-degree equations, may have more than one solution. Check all potential solutions to ensure they satisfy the original equation.
Equations can be classified into various types based on their structure, degree, and the nature of their variables. Here are some key types of equations:
Linear Equations: These are equations of the form ax + b = 0 where a and b are constants, and x is a variable. They graph as straight lines and have a degree of one.
Quadratic Equations: These equations are of the form ax² + bx + c = 0 where a, b, and c are constants, and x is a variable. They graph as parabolas and have a degree of two.
Polynomial Equations: These have the general form aⁿxⁿ + aⁿ⁻¹xⁿ⁻¹ + ... + a¹x + a⁰ = 0 where n is the degree of the polynomial, and each term includes a constant coefficient a and a power of x.
Exponential Equations: In these equations, the variable appears as an exponent, such as in aˣ bˣ = c. Solving these often involves logarithmic functions.
Logarithmic Equations: These involve logarithmic functions, such as log_b(x) = y, and can be rewritten into exponential form for easier manipulation.
Rational Equations: These include rational expressions, such as fractions with polynomials in the numerator and denominator. An example is (x+1)/(x-1) = 3.
Trigonometric Equations: These involve trigonometric functions like sine, cosine, or tangent, such as sin(x) = 0.5. Solutions often require knowledge of inverse trigonometric functions or identities.
Differential Equations: These involve derivatives, such as dy/dx = 3x. They describe the relationship between a function and its rate of change, often modeling physical or natural phenomena.
Diophantine Equations: These are equations that seek integer solutions, often used in number theory. An example is x² + y² =
Inequalities: While not equations in the strict sense, these involve inequality signs like >, <, >=, or <= to compare two expressions.
A linear equation is a mathematical statement that represents a straight-line relationship between variables. It typically has the form:
𝑦=𝑚𝑥+𝑐
Or, in a more general form:
𝑎𝑥+𝑏𝑦=𝑐
where m represents the slope of the line, c is the y-intercept, and a, b, and c are constants. Here are some key points about linear equations:
Slope: The coefficient m (or -a/b in the general form) defines the slope, indicating how much y changes for each unit change in x.
Y-Intercept: The constant c represents the y-intercept, the point where the line intersects the y-axis.
Graph: A linear equation graphs as a straight line on a Cartesian coordinate system, with each point on the line representing a solution to the equation.
Solving: Linear equations can be solved algebraically by isolating the variable of interest. For instance, in 3x + 2y = 6, you can rearrange to solve for y in terms of x or vice versa.
Systems: Multiple linear equations can form a system of linear equations, which can be solved simultaneously to find a point of intersection (if any).
A quadratic equation is a type of polynomial equation that has a degree of two, meaning it involves a variable raised to the power of two. The general form of a quadratic equation is:
𝑎𝑥²+𝑏𝑥+𝑐=0
where a, b, and c are constants, and a is not zero. Here are some key characteristics of quadratic equations:
Graph: A quadratic equation graphs as a parabola on a Cartesian coordinate system. The parabola can open upwards or downwards depending on the sign of the coefficient a.
Roots: Quadratic equations typically have two solutions or roots, which can be real or complex numbers. These roots correspond to the points where the graph intersects the x-axis.
Factoring: Some quadratic equations can be solved by factoring the expression into two binomial terms, such as transforming 𝑎𝑥²+𝑏𝑥+𝑐 into (𝑚𝑥+𝑛)(𝑝𝑥+𝑞).
Quadratic Formula: For equations that cannot be factored easily, the quadratic formula provides a solution:
𝑥=−𝑏±𝑏²−4𝑎𝑐²𝑎
This formula calculates the roots based on the values of a, b, and c.
Completing the Square: Another method to solve quadratic equations involves rearranging the equation into a perfect square trinomial, which simplifies the equation into a square of a binomial.
Vertex: The vertex of the parabola is a significant point, representing the minimum or maximum value of the quadratic function, depending on whether the parabola opens upwards or downwards.
A cubic equation is a type of polynomial equation of degree three, meaning it involves a variable raised to the power of three. The general form of a cubic equation is:
𝑎𝑥³+𝑏𝑥²+𝑐𝑥+𝑑=0
where a, b, c, and d are constants, and a is not zero. Here are some key characteristics of cubic equations:
Graph: A cubic equation graphs as a curve that can exhibit up to two turning points. The nature of the curve depends on the coefficients of the equation, and it can take various shapes, including having one or three real roots.
Roots: A cubic equation can have one or three real roots, or one real root and a pair of complex conjugate roots. The nature and number of roots depend on the discriminant of the equation and the values of its coefficients.
Factoring: Some cubic equations can be factored into a product of simpler polynomials, such as a linear term and a quadratic term, or three linear terms. Factoring can simplify the process of finding roots.
Cardano’s Method: For general cubic equations, Cardano’s method provides an algebraic solution. This method involves a series of transformations and substitutions to simplify the equation into a form that allows for a straightforward solution.
Applications: Cubic equations have various applications in mathematics and other fields. In calculus, they can describe functions with multiple turning points. In physics, they model real-world phenomena such as the behavior of polynomials in thermodynamics or kinematic equations.
These will give you an idea of the meaning of an equation in math.
Equation | Is it an equation? | |
|---|---|---|
1 | y = 9x – 5 | Yes |
2 | y + x²- 8 | No, because there is no ‘equal to’ symbol. |
3 |
|
|
Aspect | Equation | Expression |
|---|---|---|
Definition | A mathematical statement that asserts the equality of two expressions, connected by an equal sign ( | A combination of numbers, variables, and operators that represent a value, but do not assert equality. |
Components | Consists of two expressions or terms separated by an equal sign ( | Consists of numbers, variables, and operators without an equal sign. |
Example |
|
|
Purpose | Establishes a relationship between two expressions or values, showing they are equivalent. | Represents a value or combination of values that can be evaluated or simplified. |
Solving | Can be solved by finding the values of variables that make both sides equal. | Can be simplified by performing arithmetic or algebraic operations. |
Use | Used in mathematical problems, modeling relationships, and real-world applications to find unknown values. | Often used to create equations or to simplify and evaluate values. |
Equations are a fundamental part of mathematics and have numerous applications across various fields. Here are some key points to consider:
Defining Equality: Equations assert that two expressions are equal, creating a balanced relationship between variables, constants, and terms.
Types: Equations come in various types, including linear, quadratic, cubic, polynomial, exponential, logarithmic, rational, trigonometric, and differential equations. Each type has unique properties and applications.
Graphical Representation: Many equations can be visualized graphically, such as linear equations forming straight lines, quadratic equations creating parabolas, and cubic equations producing curves with multiple turning points.
Solving Methods: Different equations require different solving techniques, such as algebraic manipulation, factoring, completing the square, the quadratic formula, Cardano’s method, or logarithmic transformation.
Applications: Equations are used in various fields, including physics, engineering, economics, and computer science. They model relationships, such as distance-time-speed in physics, profit functions in economics, and algorithms in computer science.
Systems of Equations: Multiple equations can form systems, which can be solved simultaneously to find common solutions. This is particularly useful in modeling complex relationships between variables.
Real and Complex Solutions: Depending on the type of equation, solutions can be real or complex numbers. For example, quadratic equations can have one, two, or no real solutions, with complex solutions arising from a negative discriminant.
Symbolic and Numerical Solutions: Equations can be solved symbolically to yield general solutions or numerically for specific values. Symbolic solutions provide general forms, while numerical solutions give specific answers.
Manipulation: Equations can be manipulated algebraically by performing operations on both sides, such as addition, subtraction, multiplication, and division, to simplify or solve them.
Role in Education: Equations play a crucial role in mathematics education, helping students develop problem-solving skills and analytical thinking. Understanding different types of equations and their solutions is essential for advancing in mathematics and related fields.
Simplify the equation and solve for 𝑥: 5𝑥−7=3𝑥+5
Answer:
Subtract 3𝑥 from both sides:
2𝑥−7=5
Add 7 to both sides:
2𝑥=12
Divide by 2:
𝑥=6
Solve the following quadratic equation by factoring:
𝑥²−7𝑥+12=0
Answer:
Factor the equation:
(𝑥−3)(𝑥−4)=0
Set each factor equal to zero:
𝑥−3=0 ⇒ 𝑥=3
𝑥−4=0 ⇒ 𝑥=4
Thus, the solutions are:
𝑥=3 or 𝑥=4
Simplify and solve the following equation for 𝑦: 3(𝑦−2)=2𝑦+6
Answer:
Distribute 3:
3𝑦−6=2𝑦+6
Subtract 2𝑦 from both sides:
𝑦−6=6
Add 6 to both sides:
𝑦=12
Solve the equation for 𝑥:
2(𝑥+4)=3𝑥+10
Answer:
Distribute 2:
2𝑥+8=3𝑥+10
Subtract 2𝑥 from both sides:
8=𝑥+10
Subtract 10 from both sides:
−2=𝑥
Thus, 𝑥=−2
Solve for 𝑥: 4𝑥−2(3𝑥−4)=6
Answer:
Distribute the -2:
4𝑥−6𝑥+8=6
Combine like terms:
−2𝑥+8=6
Subtract 8 from both sides:
−2𝑥=−2
Divide by -2:
𝑥=1
An equation is a mathematical statement that asserts equality between two expressions. For example, 3x + 5 = 14 is an equation where both sides are balanced, and solving it involves finding the value of x that makes this equality true.
An equation is a mathematical statement that declares the equality of two expressions, connected by an equal sign (=). It consists of terms, variables, constants, and operators, allowing it to describe mathematical relationships and form the basis for solving various problems.
An equation in math is a formal statement asserting that two expressions are equal. It can range from simple arithmetic equations to complex algebraic forms. Equations play a vital role in modeling relationships, representing mathematical functions, and solving real-world problems.
A simple equation is an equation with straightforward expressions and limited variables or constants, such as 2x + 3 = 7. Solving it involves basic algebraic manipulation to isolate and determine the value of the variable, making both sides equal.
The simplest type of equation is an arithmetic equation, which involves only numbers and arithmetic operators. An example is 5 + 10 = 15, where both sides consist solely of numerical values, and no variables or complex algebraic forms are present
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
What is the value of x in the equation 2x + 5 = 15?
3
5
10
15
Solve the equation 4(x−2)=12. What is x?
1
3
4
5
If 3x−7=2x+8, find x.
15
-15
1
7
What is the solution to 5(x+3)=2x+21?
-3
0
3
6
Solve for y in the equation 7y−3=4y+12.
-15
15
5
10
If 6−x=2x+1, find x.
-5
5
7
-7
Find x if 8x+4=20.
2
4
6
8
Solve the equation 2(x−1)=x+4. What is x?
3
5
6
7
What is x in the equation 3x4=9?
12
15
18
27
Solve 2x+7=3x−5. What is x?
-12
12
6
-6
Before you leave, take our quick quiz to enhance your learning!

