What is 2^3 \times 2^2 ?
2^5
2^6
2^4
2^3

Exponents are a fundamental concept in mathematics used to express repeated multiplication of the same number. An exponent tells us how many times to multiply a base number by itself. This operation is often called “raising to a power.”

Exponents are mathematical expressions that simplify the process of multiplying a number by itself multiple times. Understanding different types of exponents is crucial for handling complex calculations effectively. Here’s a look at the various types of exponents and their specific characteristics:
Exponents are a key element in algebra that facilitate the manipulation and simplification of expressions involving powers of numbers or variables. Understanding the properties of exponents is essential for efficient problem-solving. Here’s a comprehensive guide to the main properties:
Handling exponents effectively is key to solving algebraic expressions and equations efficiently.
Problem: Calculate 2³
Solution: 2³=2×2×2=8
Answer: 8
Problem: Evaluate 5⁻².
Solution: 5⁻²=1/5²=1/25
Answer: 1/25
Problem: Simplify 16¹/².
Solution: 16¹/²=16=4.
Answer: 4
Problem: Simplify (3²)×(3³).
Solution: (3²)×(3³)=3²⁺³=3⁵=243
Answer: 243
Problem: Simplify 2⁸/2³ Solution: 2⁸/2³=2⁸⁻³=2⁵ Answer: 32
Problem: Calculate (4²)³.
Solution: (4²)³=4²×3=46=4096
Answer: 4096
Problem: What is 7⁰?
Solution: Any non-zero number raised to the power of zero equals 1.
Answer: 1
The seven rules of exponents are: product of powers, quotient of powers, power of a power, power of a product, power of a quotient, zero exponent, and negative exponent. These rules simplify expressions and solve equations involving powers.
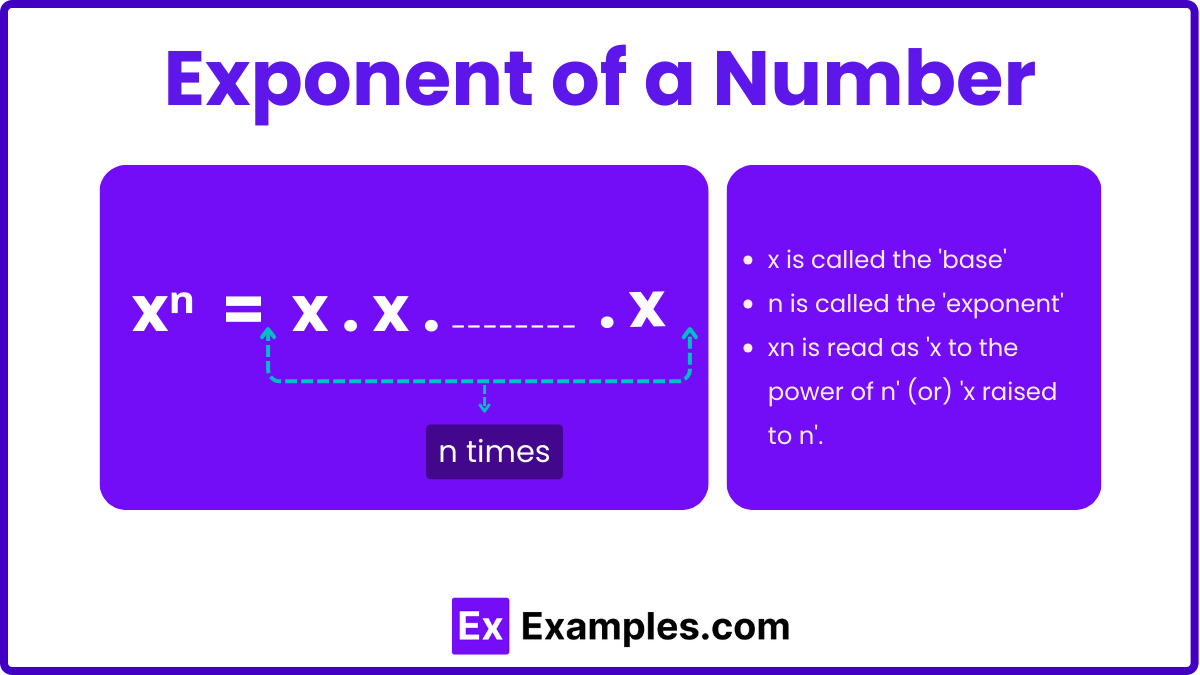
To calculate an exponent, multiply the base (the number) by itself as many times as indicated by the exponent. For example, 3⁴ means multiplying 3 by itself four times: 3×3×3×3=81
Exponents can be explained to children as a shortcut for multiplication. Tell them it shows how many times to use the number in a multiplication. For example, 5 means multiplying 5 by itself three times: 5×5×5
Exponents are a way to express repeated multiplication. Instead of writing a number many times, you can use an exponent to tell how many times to multiply a number by itself. They simplify numbers and calculations, especially with large values.
Two raised to the power of 16 is 216, which equals 65,536. It is the result of multiplying 2 by itself 16 times.
Two raised to the power of 9 is 29, which equals 512. This results from multiplying 2 by itself nine times.

Exponents are a fundamental concept in mathematics used to express repeated multiplication of the same number. An exponent tells us how many times to multiply a base number by itself. This operation is often called “raising to a power.”

Exponents are mathematical expressions that simplify the process of multiplying a number by itself multiple times. Understanding different types of exponents is crucial for handling complex calculations effectively. Here’s a look at the various types of exponents and their specific characteristics:
Description: A positive exponent tells how many times to multiply the base number by itself.
Example: 5³ means 5×5×5=125
Description: Any non-zero base raised to the power of zero equals one.
Example: 7⁰=1
Description: A negative exponent indicates the reciprocal of the base raised to the corresponding positive exponent.
Example: 2⁻³means 1/2³=1/8
Description: A fractional exponent, where the numerator is a power and the denominator is a root, simplifies to taking the root of the base raised to the power of the numerator.
Example: 16¹/² represents the square root of 16, which is 4.
Description: Similar to fractional exponents, rational exponents represent powers and roots but with potentially more complex fractions.
Example: 8²/³ means the cube root of 8 squared, which is 4.
Description: These exponents are represented by irrational numbers and often arise in advanced mathematics and physics.
Example: 𝑒𝜋, where 𝑒 is Euler’s number and 𝜋 is pi.
Description: Complex exponents involve a complex number in the exponent and are used in higher-level mathematics, particularly in the study of waves and oscillations.
Example: 𝑒ᶦπ equals −1, according to Euler’s formula.
Exponents are a key element in algebra that facilitate the manipulation and simplification of expressions involving powers of numbers or variables. Understanding the properties of exponents is essential for efficient problem-solving. Here’s a comprehensive guide to the main properties:
Rule: When multiplying powers with the same base, add their exponents.
Formula: 𝑎ᵐ×𝑎ⁿ=𝑎ᵐ⁺ⁿ
Example: 𝑥²×𝑥³=𝑥²⁺³=𝑥⁵
Rule: When dividing powers with the same base, subtract the exponent of the denominator from the exponent of the numerator.
Formula: 𝑎ᵐ/𝑎ⁿ=𝑎ᵐ⁻ⁿ
Example: 𝑥⁵/𝑥²=𝑥⁵⁻³=𝑥³
Rule: When raising a power to another power, multiply the exponents.
Formula: (𝑎ᵐ)ⁿ=𝑎ᵐˣⁿ
Example: (𝑥²)³=𝑥²ˣ³=𝑥⁶
Rule: When raising a product to a power, raise each factor of the product to the power.
Formula: (𝑎𝑏)ⁿ=𝑎ⁿ×𝑏ⁿ
Example: (2𝑥)³=2³ . x³ =8𝑥³
Rule: When raising a quotient to a power, raise both the numerator and the denominator to the power.
Formula: (𝑎/𝑏)ⁿ=𝑎ⁿ/𝑏ⁿ
Example: (𝑥𝑦)²=𝑥²/𝑦²
Rule: Any non-zero base raised to the power of zero equals one.
Formula: 𝑎⁰=1 (where 𝑎≠0)
Example: 5⁰=1
Rule: A negative exponent represents the reciprocal of the base raised to the absolute value of the exponent.
Formula: 𝑎⁻ⁿ=1/𝑎ⁿ (where 𝑎≠0)
Example:
2⁻³=1/2³=1/8
Handling exponents effectively is key to solving algebraic expressions and equations efficiently.
If a fraction has a negative exponent, then we take the reciprocal of the fraction to make the exponent positive, i.e., (a/b)-m = (b/a)m.
Decimal exponents can be solved by first converting the decimal into fraction form, i.e., 20.5 can be written as 21/2

Problem: Calculate 2³
Solution: 2³=2×2×2=8
Answer: 8
Problem: Evaluate 5⁻².
Solution: 5⁻²=1/5²=1/25
Answer: 1/25
Problem: Simplify 16¹/².
Solution: 16¹/²=16=4.
Answer: 4
Problem: Simplify (3²)×(3³).
Solution: (3²)×(3³)=3²⁺³=3⁵=243
Answer: 243
Problem: Simplify 2⁸/2³ Solution: 2⁸/2³=2⁸⁻³=2⁵ Answer: 32
Problem: Calculate (4²)³.
Solution: (4²)³=4²×3=46=4096
Answer: 4096
Problem: What is 7⁰?
Solution: Any non-zero number raised to the power of zero equals 1.
Answer: 1
The seven rules of exponents are: product of powers, quotient of powers, power of a power, power of a product, power of a quotient, zero exponent, and negative exponent. These rules simplify expressions and solve equations involving powers.
To calculate an exponent, multiply the base (the number) by itself as many times as indicated by the exponent. For example, 3⁴ means multiplying 3 by itself four times: 3×3×3×3=81
Exponents can be explained to children as a shortcut for multiplication. Tell them it shows how many times to use the number in a multiplication. For example, 5 means multiplying 5 by itself three times: 5×5×5
Exponents are a way to express repeated multiplication. Instead of writing a number many times, you can use an exponent to tell how many times to multiply a number by itself. They simplify numbers and calculations, especially with large values.
Two raised to the power of 16 is 216, which equals 65,536. It is the result of multiplying 2 by itself 16 times.
Two raised to the power of 9 is 29, which equals 512. This results from multiplying 2 by itself nine times.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
What is 2^3 \times 2^2 ?
2^5
2^6
2^4
2^3
Simplify (3^4)^2 .
3^8
3^6
3^4
3^2
What is 5^0 ?
0
1
2
4
Evaluate \frac{7^5}{7^2} .
7^7
7^6
7^2
7^3
Simplify 2^{-3} .
\frac{1}{2^3}
2^3
-2^3
\frac{1}{2}
Which of the following is equivalent to (x^3)^4 ?
x^7
x^{12}
x^{16}
x^4
What is \left(\frac{2}{5}\right)^3 ?
\frac{6}{15}
\frac{8}{15}
\frac{1}{2^3}
\frac{8}{125}
What is the value of 10^2 - 10^1 ?
70
80
90
100
Simplify \frac{(2x^3)^2}{4x^2} .
\frac{4x^6}{4x^2} = x^4
\frac{4x^6}{4x^4} = x^2
\frac{4x^6}{x^2} = 4x^4
\frac{x^6}{4x^2} = \frac{x^4}{4}
If a = 2 and b = 3 , what is a^b \times b^a ?
48
49
50
51
Before you leave, take our quick quiz to enhance your learning!

