What are the factors of 24?
1, 2, 4, 6, 8, 12, 24
1, 2, 3, 4, 6, 12, 24
1, 2, 4, 6, 10, 12, 24
1, 2, 5, 6, 8, 12, 24

The factors of 24 is an essential math skill that helps in various arithmetic operations. Factors are numbers that can be evenly divided into another number without leaving a remainder. For 24, these include both positive and negative numbers. This guide will explore the factors of 24, how to find them, and their significance. Whether you’re a student tackling homework or a math enthusiast brushing up on your skills, knowing the factors of 24 and how to determine them is fundamental. Read on to learn about prime factorization, common factor pairs, and more.
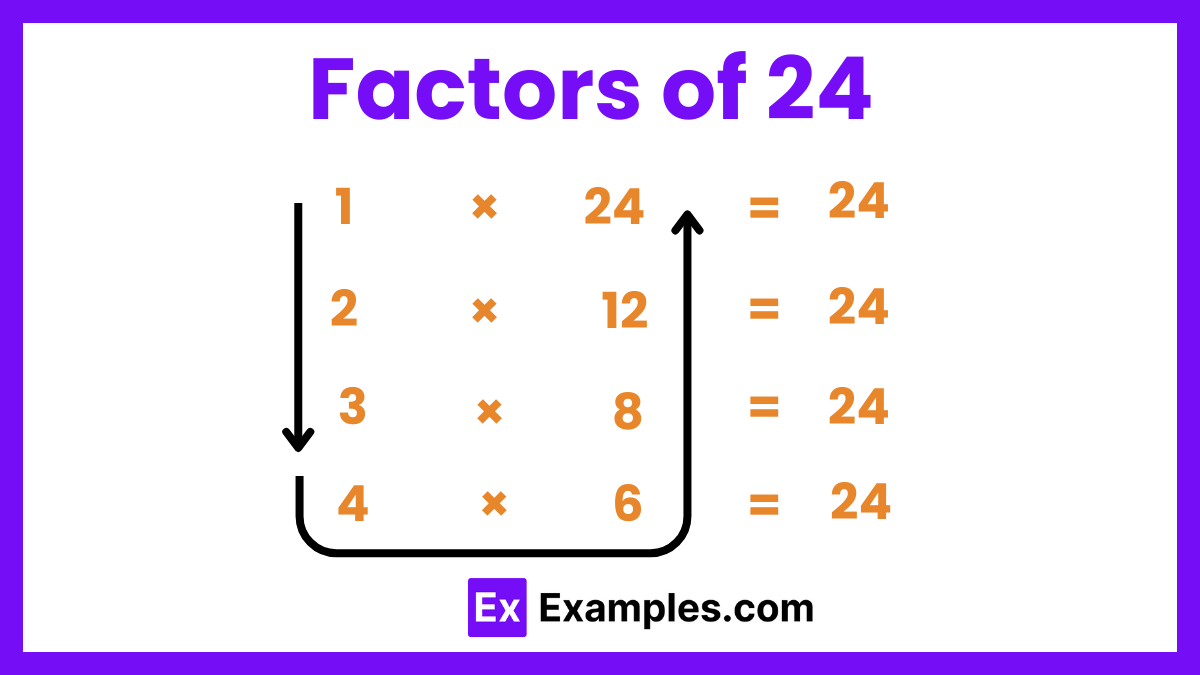
The factors of 24 are the numbers that divide 24 without leaving a remainder. These include 1, 2, 3, 4, 6, 8, 12, and 24. Additionally, each of these numbers can be paired with another factor to multiply and result in 24, such as (1, 24), (2, 12), (3, 8), and (4, 6). Understanding these factors helps in various mathematical applications, from simplifying fractions to solving complex equations.
Calculating the prime factors of 24 involves breaking down the number into the prime numbers that multiply together to result in 24. Prime factors are the building blocks of a number, consisting only of prime numbers, which are numbers greater than 1 that have no positive divisors other than 1 and themselves. Here’s how to find the prime factors of 24:
Understanding the factors of 24 can simplify many mathematical tasks, from basic arithmetic to more complex problem-solving. Here are some helpful tips for working with the factors of 24:
To verify, list the factors and multiply each pair to ensure they result in 24. Check for completeness by confirming no factors are missed.
To find the sum, add all the factors of 24: 1 + 2 + 3 + 4 + 6 + 8 + 12 + 24 = 60.
To simplify a fraction, find the common factors of the numerator and denominator. For example, 24/36 simplifies to 2/3 by dividing both by their GCF, which is 12.
To find pairs of numbers that multiply together to give 24, we look for factor pairs. Here are the pairs of two factors that equal 24:
(1, 24): 1 × 24 = 24, (2, 12): 2 × 12 = 24, (3, 8): 3 × 8 = 24, (4, 6): 4 × 6 = 24, (-1, -24): -1 × -24 = 24, (-2, -12): -2 × -12 = 24, (-3, -8): -3 × -8 = 24, (-4, -6): -4 × -6 = 24.
Factors of 24 help find the LCM with another number by identifying the highest power of each prime factor involved. For example, the LCM of 24 and 18 (prime factors 2³×3 and 2×3²) is 2³×3²=72
The prime factors of 24 are 2 and 3. The prime factorization of 24 is 23×3.

The factors of 24 is an essential math skill that helps in various arithmetic operations. Factors are numbers that can be evenly divided into another number without leaving a remainder. For 24, these include both positive and negative numbers. This guide will explore the factors of 24, how to find them, and their significance. Whether you’re a student tackling homework or a math enthusiast brushing up on your skills, knowing the factors of 24 and how to determine them is fundamental. Read on to learn about prime factorization, common factor pairs, and more.

The factors of 24 are the numbers that divide 24 without leaving a remainder. These include 1, 2, 3, 4, 6, 8, 12, and 24. Additionally, each of these numbers can be paired with another factor to multiply and result in 24, such as (1, 24), (2, 12), (3, 8), and (4, 6). Understanding these factors helps in various mathematical applications, from simplifying fractions to solving complex equations.
(1, 24): Multiplying 1 by 24 gives 24. This is the simplest factor pair, demonstrating that 24 can be divided by 1 and remain itself.
(2, 12): Multiplying 2 by 12 results in 24. This shows that 24 can be divided into two groups of 12 or twelve groups of 2.
(3, 8): Multiplying 3 by 8 equals 24. This pair is useful in understanding how 24 can be broken down into smaller, yet still whole, components.
(4, 6): Multiplying 4 by 6 produces 24. This factor pair is significant in various mathematical problems and real-life applications.
(-1, -24): Multiplying -1 by -24 also results in 24. Negative factor pairs are important in algebra and equations involving negative numbers.
(-2, -12): Multiplying -2 by -12 gives 24. This pair highlights that the product of two negative numbers is positive.
(-3, -8): Multiplying -3 by -8 equals 24. Negative factor pairs help in understanding the properties of multiplication with negative integers.
(-4, -6): Multiplying -4 by -6 results in 24. Recognizing these pairs is crucial for solving equations with negative values.
Calculating the prime factors of 24 involves breaking down the number into the prime numbers that multiply together to result in 24. Prime factors are the building blocks of a number, consisting only of prime numbers, which are numbers greater than 1 that have no positive divisors other than 1 and themselves. Here’s how to find the prime factors of 24:
Begin with 2, the smallest prime number. Check if 24 is divisible by 2. Since 24 is even, it is divisible by 2.
Divide 24 by 2, which gives 12. So, 2 is a prime factor, and 24 can be written as 2 × 12.
Divide 12 by 2 again, resulting in 6. Now, 24 can be written as 2 × 2 × 6.
Divide 6 by 2, giving 3. Now, 24 can be written as 2 × 2 × 2 × 3.
The quotient 3 is a prime number. Thus, the factorization process is complete.
Problem: List all the positive factors of 24.
Solution: The positive factors of 24 are 1, 2, 3, 4, 6, 8, 12, and 24. These numbers divide 24 without leaving a remainder.
Problem: Find all the factor pairs of 24.
Solution: The factor pairs of 24 are:
(1, 24): 1 × 24 = 24
(2, 12): 2 × 12 = 24
(3, 8): 3 × 8 = 24
(4, 6): 4 × 6 = 24
Problem: Determine the prime factorization of 24.
Solution: The prime factorization of 24 involves breaking it down into prime factors:
24 ÷ 2 = 12
12 ÷ 2 = 6
6 ÷ 2 = 3
3 is a prime number So, the prime factorization of 24 is 23×3.
Problem: Simplify the fraction 24/36 using the factors of 24.
Solution: First, find the greatest common factor (GCF) of 24 and 36. The factors of 24 are 1, 2, 3, 4, 6, 8, 12, and 24. The factors of 36 are 1, 2, 3, 4, 6, 9, 12, 18, and 36. The GCF is 12.
24÷12/36÷12=2/3 So, 24/36 simplifies to 2/3.
Problem: If you have 24 apples and want to divide them equally among 3 friends, how many apples will each friend get?
Solution: To find out how many apples each friend will get, divide 24 by 3.
24 ÷ 3 = 8 Each friend will get 8 apples.
Understanding the factors of 24 can simplify many mathematical tasks, from basic arithmetic to more complex problem-solving. Here are some helpful tips for working with the factors of 24:
Start with the smallest factor: Always begin by identifying the smallest prime factor, which for 24 is 2.
Use division to find factors: Divide 24 by its smaller factors to find pairs that multiply to give 24.
For example, 24 ÷ 2 = 12, so 2 and 12 are factors.
Check for completeness: Ensure that you’ve found all factor pairs by multiplying them back to see if they result in 24.
Prime factorization: Break 24 down into its prime factors for a clearer understanding. The prime factorization of 24 is 23×3.
Utilize factor pairs: Recognize and use factor pairs for quick calculations and simplifications, such as (1, 24), (2, 12), (3, 8), and (4, 6).
Negative factors: Remember that negative numbers can also be factors. For example, -1 and -24 are negative factor pairs of 24 because their product is also 24.
Visual aids: Use factor trees or lists to visualize and organize the factors of 24, making it easier to identify all possible factors.
To verify, list the factors and multiply each pair to ensure they result in 24. Check for completeness by confirming no factors are missed.
To find the sum, add all the factors of 24: 1 + 2 + 3 + 4 + 6 + 8 + 12 + 24 = 60.
To simplify a fraction, find the common factors of the numerator and denominator. For example, 24/36 simplifies to 2/3 by dividing both by their GCF, which is 12.
To find pairs of numbers that multiply together to give 24, we look for factor pairs. Here are the pairs of two factors that equal 24:
(1, 24): 1 × 24 = 24, (2, 12): 2 × 12 = 24, (3, 8): 3 × 8 = 24, (4, 6): 4 × 6 = 24, (-1, -24): -1 × -24 = 24, (-2, -12): -2 × -12 = 24, (-3, -8): -3 × -8 = 24, (-4, -6): -4 × -6 = 24.
Factors of 24 help find the LCM with another number by identifying the highest power of each prime factor involved. For example, the LCM of 24 and 18 (prime factors 2³×3 and 2×3²) is 2³×3²=72
The prime factors of 24 are 2 and 3. The prime factorization of 24 is 23×3.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
What are the factors of 24?
1, 2, 4, 6, 8, 12, 24
1, 2, 3, 4, 6, 12, 24
1, 2, 4, 6, 10, 12, 24
1, 2, 5, 6, 8, 12, 24
Which of the following is not a factor of 24?
5
8
12
15
What is the sum of all factors of 24?
36
42
50
60
What is the smallest factor of 24?
1
2
3
4
What is the largest factor of 24?
6
8
12
24
Which two factors of 24 multiply to give 12?
2 and 6
3 and 4
4 and 6
2 and 8
Which factor of 24 is also a prime number?
1
2
4
8
If you divide 24 by 8, what is the quotient?
2
3
4
6
What are the prime factors of 24?
2 and 3
2 and 4
2, 2, 2, and 3
2, 2, 3, and 6
Which of the following numbers is a factor of 24 but not of 12?
6
8
11
15
Before you leave, take our quick quiz to enhance your learning!

