Factors of 25, Prime Factors of 25, How to Calculate, Tips

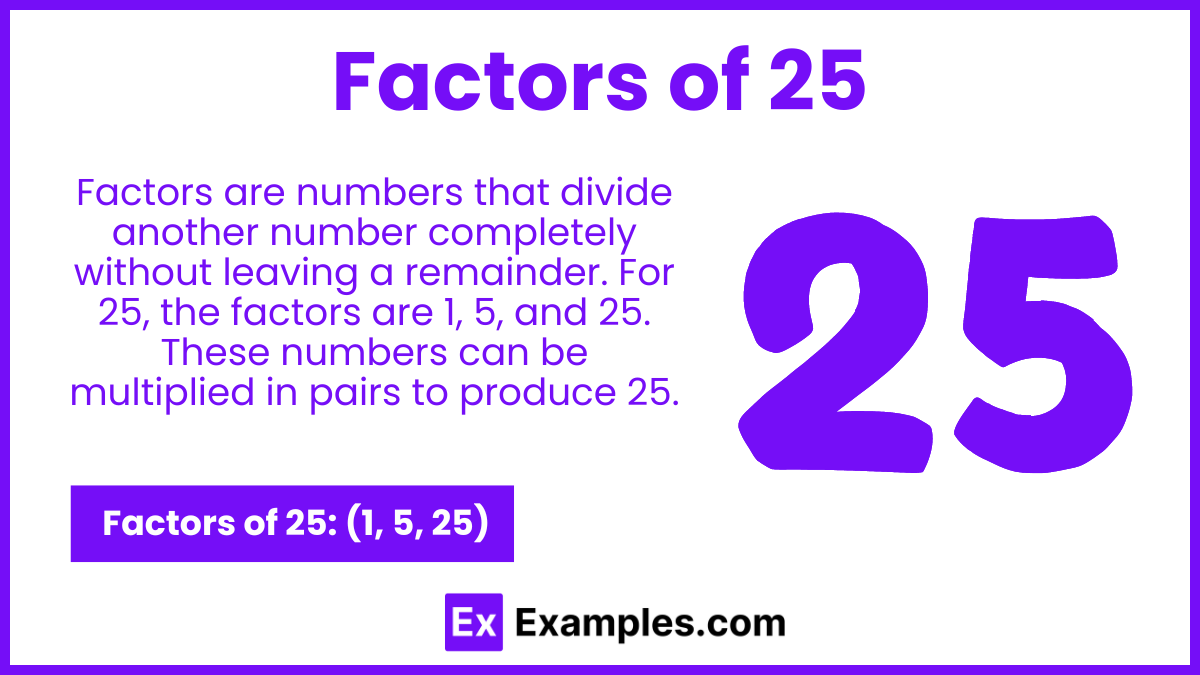
The factors of 25 is essential for mastering basic arithmetic and number theory. Factors are numbers that divide evenly into another number without leaving a remainder. For 25, these include both positive and negative numbers. As a perfect square, 25 has unique properties and factor pairs. This guide will explore all the factors of 25, how to identify them, and their significance. Whether you’re a student working on math problems or someone interested in numerical properties, knowing the factors of 25 can simplify various calculations and enhance your mathematical understanding. Read on to learn more about the factors of 25.

What are the Factors of 25?

The factors of 25 are the numbers that divide 25 without leaving a remainder. These factors are 1, 5, and 25. Since 25 is a perfect square, it has a repeated factor of 5, as 5 × 5 equals 25. Additionally, 25 has negative factors: -1, -5, and -25, because the product of two negative factors also gives a positive result. Understanding these factors is crucial for simplifying mathematical expressions and solving equations involving the number 25.

Factors Pairs of 25

• (1, 25): Multiplying 1 by 25 gives 25. This shows that 1 and 25 are factor pairs.
• (5, 5): Multiplying 5 by 5 equals 25. This demonstrates that 25 is a perfect square.
• (-1, -25): Multiplying -1 by -25 also results in 25. This pair shows that the product of two negative factors is positive.
• (-5, -5): Multiplying -5 by -5 equals 25. This further confirms the perfect square property with negative factors.

How to Calculate Prime Factors of 25

Calculating the prime factors of a number involves breaking it down into its prime components. Prime factors are prime numbers that, when multiplied together, give the original number. Here’s how to determine the prime factors of 25:

Step 1: Identify the smallest prime number.

• Begin with the smallest prime number, which is 2. Check if 25 is divisible by 2. Since 25 is odd, it is not divisible by 2.

Step 2: Move to the next smallest prime number.

• Check if 25 is divisible by 3. The sum of the digits (2 + 5 = 7) is not divisible by 3, so 25 is not divisible by 3.

Step 3: Check divisibility by the next prime number.

• Check if 25 is divisible by 5. Since 25 ends in 5, it is divisible by 5. Divide 25 by 5, which gives 5. Therefore, 5 is a prime factor of 25.

Step 4: Continue dividing by the same prime number.

• Divide 5 by 5 again, which results in 1. This confirms that 25 can be expressed as 5 × 5.

Factors of 25: Examples

Example 1: Identifying Factors

• Problem: List all the positive factors of 25.
• Solution: The positive factors of 25 are 1, 5, and 25. These numbers divide 25 without leaving a remainder.

Example 2: Finding Factor Pairs

• Problem: Find all the factor pairs of 25.
• Solution: The factor pairs of 25 are:
• (1, 25): 1 × 25 = 25
• (5, 5): 5 × 5 = 25

Example 3: Using Prime Factorization

• Problem: Determine the prime factorization of 25.
• Solution: The prime factorization of 25 involves breaking it down into prime factors:
• 25 ÷ 5 = 5
• 5 is a prime number, so the factorization process stops here. Therefore, the prime factorization of 25 is 5².

Example 4: Simplifying Fractions

• Problem: Simplify the fraction 50/25 using the factors of 25.
• Solution: Since 25 is a factor of 50, divide both the numerator and denominator by 25:
• 50÷25/25÷25=2/1 So, 50/25​ simplifies to 2.

Example 5: Solving Word Problems

• Problem: If you have 25 apples and want to distribute them equally among 5 friends, how many apples will each friend get?
• Solution: To find out how many apples each friend will get, divide 25 by 5:
• 25 ÷ 5 = 5 Each friend will get 5 apples.

Factors of 25 : Tips

The factors of 25 can simplify many mathematical tasks, from basic arithmetic to solving complex problems. Here are some helpful tips for working with the factors of 25:

1. Since 25 is a perfect square, remember that one of its factor pairs is the same number multiplied by itself, which is 5 × 5.
2. Knowing that the prime factorization of 25 is 5² helps in breaking down the number into its prime components quickly.
3. Use the factors of 25 to simplify fractions. For example, 50/25​ simplifies to 2 by dividing both the numerator and the denominator by 25.
4. List factor pairs to solve problems efficiently. For 25, the factor pairs are (1, 25) and (5, 5), which can be useful in various calculations and distributions.
5. To determine if a number is divisible by 25, check if it ends in 00 or 25. This simple rule can save time in identifying divisibility.
6. When solving equations involving 25, consider using its factors to simplify the expressions or find common multiples.
7. Use visual aids like factor trees to understand the breakdown of 25 into its prime factors, making it easier to comprehend and apply.
8. In word problems, use the factors of 25 to distribute quantities evenly or to find equal groups, ensuring accurate solutions.
9. Recognize that the factors of 25 are limited due to its prime factorization, making calculations straightforward and less complex.
10. Use knowledge of factors and prime factorization of 25 to solve higher-level math problems, such as those involving least common multiples or greatest common divisors.

Is 25 a composite number?

Yes, 25 is a composite number because it has factors other than 1 and itself, specifically 5.

What is the prime factorization of 25?

The prime factorization of 25 is 5², which means 25 can be expressed as 5 × 5.

What is the sum of all the factors of 25?

A12: The sum of all the factors of 25 is 1 + 5 + 25 = 31.

How do factors of 25 relate to divisibility rules?

For a number to be divisible by 25, it must end in 00 or 25. This helps in quickly determining if a number is divisible by 25.

What is the difference between factors and multiples of 25?

Factors of 25 are numbers that divide 25 without leaving a remainder (1, 5, 25). Multiples of 25 are numbers that 25 can divide into without a remainder (25, 50, 75, etc.).

How do factors of 25 assist in least common denominators (LCD) for fractions?

When finding the LCD for fractions with denominators including 25, knowing its factors helps determine the smallest common multiple, facilitating the addition or subtraction of fractions. For example, the LCD of 1/25 and 1/30​ is 150.