Factors of 27, Prime Factors of 27, How to Calculate, Tips
The factors of a number is essential in mathematics, as it helps in simplifying complex problems and finding solutions in various fields like algebra, arithmetic, and number theory. The factors of 27, a perfect cube, play a crucial role in understanding its properties and applications. Factors are numbers that divide a given number exactly without leaving a remainder. For 27, these factors include 1, 3, 9, and 27 itself. Recognizing these factors can aid in breaking down equations, simplifying fractions, and solving problems involving multiples and divisibility. In practical terms, knowing the factors of 27 is useful in fields like engineering, computer science, and everyday problem-solving scenarios where numerical analysis is required.
What are the Factors of 27?
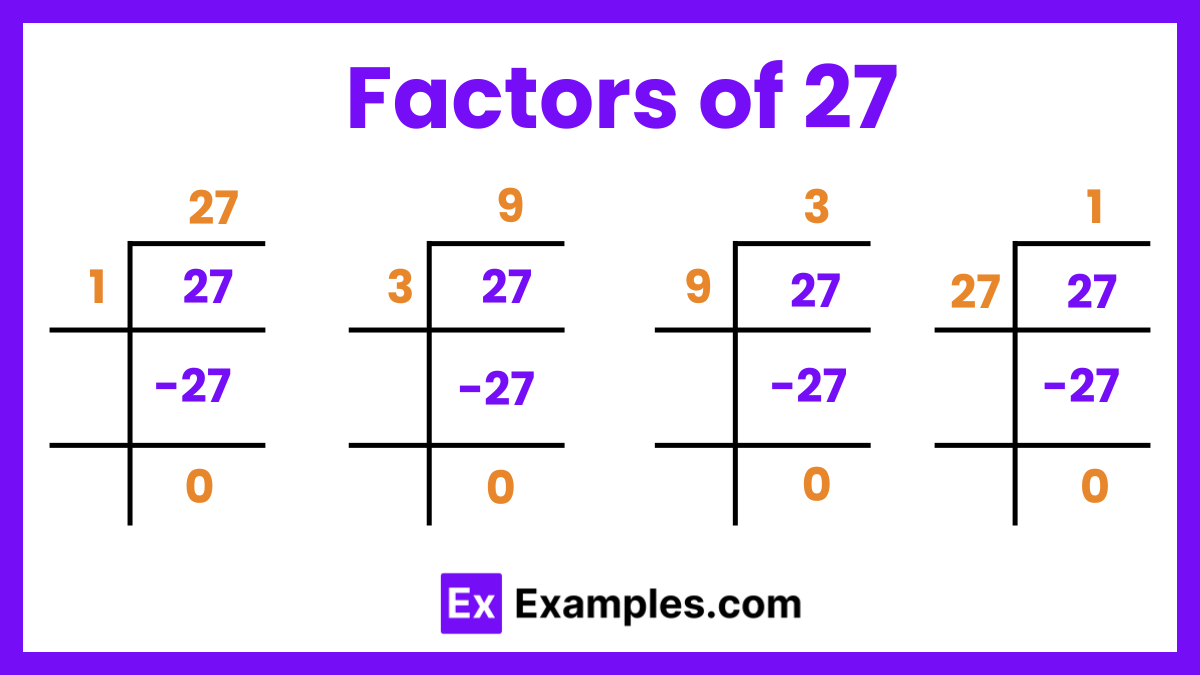
The factors of 27 are the numbers that divide it exactly without leaving a remainder. These factors are 1, 3, 9, and 27. Specifically, 27 can be expressed as 3 multiplied by itself three times (3 × 3 × 3), making it a perfect cube. Understanding these factors helps in simplifying mathematical problems and finding common multiples.
Factors Pairs of 27
The factor pairs of 27 are combinations of two numbers that, when multiplied together, yield 27. These pairs include:
- 1 and 27: Since 1 multiplied by 27 equals 27.
- 3 and 9: Because 3 multiplied by 9 equals 27.
How to Calculate Prime Factors of 27
To find the prime factors of 27, we need to identify the prime numbers that multiply together to result in 27. Prime numbers are those greater than 1 and have no divisors other than 1 and themselves. The process involves dividing 27 by the smallest prime number and continuing until we reach a prime number. Here’s a step-by-step guide:
Step 1: Start with the smallest prime number
- The smallest prime number is 2. Check if 27 is divisible by 2. Since 27 is an odd number, it is not divisible by 2.
Step 2: Move to the next smallest prime number
- The next smallest prime number is 3. Check if 27 is divisible by 3. Since 27 divided by 3 equals 9, write down 3 as a prime factor.
Step 3: Continue dividing by 3
- Now, take the quotient from the previous step, which is 9. Check if 9 is divisible by 3. Since 9 divided by 3 equals 3, write down 3 as another prime factor.
Step 4: Repeat the division
- Take the quotient from the previous step, which is 3. Check if 3 is divisible by 3. Since 3 divided by 3 equals 1, write down 3 as the final prime factor.
Step 5: Complete the factorization
- Once you reach 1, you have all the prime factors. For 27, the prime factors are 3, 3, and 3.
In conclusion, the prime factorization of 27 is 3 × 3 × 3, or 33.
Factors of 27: Examples
Example 1: Listing Factors
Problem: List all the factors of 27.
Solution: The factors of 27 are: 1, 3, 9, and 27.
Example 2: Checking if a Number is a Factor
Problem: Is 6 a factor of 27?
Solution: Divide 27 by 6. Since 27 ÷ 6 = 4.5 (not an integer), 6 is not a factor of 27.
Example 3: Sum of Factors
Problem: What is the sum of all factors of 27?
Solution: Sum the factors: 1 + 3 + 9 + 27 = 40.
Example 4: Product of Factor Pairs
Problem: Verify the product of factor pairs equals 27.
Solution: Factor pairs are (1, 27) and (3, 9). 1 × 27 = 27 3 × 9 = 27.
Example 5: Greatest Common Factor (GCF)
Problem: Find the GCF of 27 and 15.
Solution: Factors of 27: 1, 3, 9, 27.
Factors of 15: 1, 3, 5, 15. Common factors: 1, 3.
The greatest common factor is 3.
Factors of 27: Tips
When working with numbers, understanding their factors is essential. Factors of a number are integers that divide the number exactly without leaving a remainder. Here are some important points and tips about the factors of 27:
- The factors of 27 are the numbers that divide 27 exactly without leaving any remainder.
- 27 is a composite number, meaning it has more factors than just 1 and itself.
- The prime factorization of 27 is 3 × 3 × 3, which can also be written as 3333.
- This shows that 3 is a prime factor of 27, and it appears three times in its factorization.
- The complete list of factors of 27 includes: 1, 3, 9, and 27.
- To find the factors of 27, start by dividing 27 by the smallest prime number, which is 3.
- Since 27 ÷ 3 = 9, 3 is a factor. Then, divide 9 by 3 again to get 3, which is also a factor.
- The final division, 3 ÷ 3 = 1, confirms that 1 is a factor.
- You can verify the factors by multiplying them: 1 × 27 = 27 and 3 × 9 = 27.
- Understanding factors is useful in simplifying fractions, finding common denominators, and solving problems involving divisibility.
Are the factors of 27 only positive numbers?
Factors of 27 include both positive and negative numbers. The positive factors are 1, 3, 9, and 27, while the negative factors are -1, -3, -9, and -27.
Is 27 a perfect square?
No, 27 is not a perfect square. A perfect square is a number that has an integer as its square root, and the square root of 27 is not an integer.
What is the greatest common factor (GCF) of 27 and 36?
The greatest common factor of 27 and 36 is 9. This is the largest number that can divide both 27 and 36 without leaving a remainder.
Do the factors of 27 include zero?
No, the factors of 27 do not include zero. Zero is not considered a factor of any number because division by zero is undefined.
How can the factors of 27 be represented on a number line?
On a number line, the factors of 27 can be marked at positions corresponding to 1, 3, 9, and 27. This helps visualize the intervals that divide 27 evenly.
How can factors of 27 be applied in data analysis?
In data analysis, factors of 27 can be used to group data points, create equal intervals for histograms, or simplify statistical calculations by reducing numbers to their factor components. This makes it easier to handle large datasets and perform accurate analyses.
Factors of 27, Prime Factors of 27, How to Calculate, Tips

The factors of a number is essential in mathematics, as it helps in simplifying complex problems and finding solutions in various fields like algebra, arithmetic, and number theory. The factors of 27, a perfect cube, play a crucial role in understanding its properties and applications. Factors are numbers that divide a given number exactly without leaving a remainder. For 27, these factors include 1, 3, 9, and 27 itself. Recognizing these factors can aid in breaking down equations, simplifying fractions, and solving problems involving multiples and divisibility. In practical terms, knowing the factors of 27 is useful in fields like engineering, computer science, and everyday problem-solving scenarios where numerical analysis is required.
What are the Factors of 27?

The factors of 27 are the numbers that divide it exactly without leaving a remainder. These factors are 1, 3, 9, and 27. Specifically, 27 can be expressed as 3 multiplied by itself three times (3 × 3 × 3), making it a perfect cube. Understanding these factors helps in simplifying mathematical problems and finding common multiples.
Factors Pairs of 27
The factor pairs of 27 are combinations of two numbers that, when multiplied together, yield 27. These pairs include:
1 and 27: Since 1 multiplied by 27 equals 27.
3 and 9: Because 3 multiplied by 9 equals 27.
How to Calculate Prime Factors of 27
To find the prime factors of 27, we need to identify the prime numbers that multiply together to result in 27. Prime numbers are those greater than 1 and have no divisors other than 1 and themselves. The process involves dividing 27 by the smallest prime number and continuing until we reach a prime number. Here’s a step-by-step guide:
Step 1: Start with the smallest prime number
The smallest prime number is 2. Check if 27 is divisible by 2. Since 27 is an odd number, it is not divisible by 2.
Step 2: Move to the next smallest prime number
The next smallest prime number is 3. Check if 27 is divisible by 3. Since 27 divided by 3 equals 9, write down 3 as a prime factor.
Step 3: Continue dividing by 3
Now, take the quotient from the previous step, which is 9. Check if 9 is divisible by 3. Since 9 divided by 3 equals 3, write down 3 as another prime factor.
Step 4: Repeat the division
Take the quotient from the previous step, which is 3. Check if 3 is divisible by 3. Since 3 divided by 3 equals 1, write down 3 as the final prime factor.
Step 5: Complete the factorization
Once you reach 1, you have all the prime factors. For 27, the prime factors are 3, 3, and 3.
In conclusion, the prime factorization of 27 is 3 × 3 × 3, or 33.
Factors of 27: Examples
Example 1: Listing Factors
Problem: List all the factors of 27.
Solution: The factors of 27 are: 1, 3, 9, and 27.
Example 2: Checking if a Number is a Factor
Problem: Is 6 a factor of 27?
Solution: Divide 27 by 6. Since 27 ÷ 6 = 4.5 (not an integer), 6 is not a factor of 27.
Example 3: Sum of Factors
Problem: What is the sum of all factors of 27?
Solution: Sum the factors: 1 + 3 + 9 + 27 = 40.
Example 4: Product of Factor Pairs
Problem: Verify the product of factor pairs equals 27.
Solution: Factor pairs are (1, 27) and (3, 9). 1 × 27 = 27 3 × 9 = 27.
Example 5: Greatest Common Factor (GCF)
Problem: Find the GCF of 27 and 15.
Solution: Factors of 27: 1, 3, 9, 27.
Factors of 15: 1, 3, 5, 15. Common factors: 1, 3.
The greatest common factor is 3.
Factors of 27: Tips
When working with numbers, understanding their factors is essential. Factors of a number are integers that divide the number exactly without leaving a remainder. Here are some important points and tips about the factors of 27:
The factors of 27 are the numbers that divide 27 exactly without leaving any remainder.
27 is a composite number, meaning it has more factors than just 1 and itself.
The prime factorization of 27 is 3 × 3 × 3, which can also be written as 3333.
This shows that 3 is a prime factor of 27, and it appears three times in its factorization.
The complete list of factors of 27 includes: 1, 3, 9, and 27.
To find the factors of 27, start by dividing 27 by the smallest prime number, which is 3.
Since 27 ÷ 3 = 9, 3 is a factor. Then, divide 9 by 3 again to get 3, which is also a factor.
The final division, 3 ÷ 3 = 1, confirms that 1 is a factor.
You can verify the factors by multiplying them: 1 × 27 = 27 and 3 × 9 = 27.
Understanding factors is useful in simplifying fractions, finding common denominators, and solving problems involving divisibility.
Are the factors of 27 only positive numbers?
Factors of 27 include both positive and negative numbers. The positive factors are 1, 3, 9, and 27, while the negative factors are -1, -3, -9, and -27.
Is 27 a perfect square?
No, 27 is not a perfect square. A perfect square is a number that has an integer as its square root, and the square root of 27 is not an integer.
What is the greatest common factor (GCF) of 27 and 36?
The greatest common factor of 27 and 36 is 9. This is the largest number that can divide both 27 and 36 without leaving a remainder.
Do the factors of 27 include zero?
No, the factors of 27 do not include zero. Zero is not considered a factor of any number because division by zero is undefined.
How can the factors of 27 be represented on a number line?
On a number line, the factors of 27 can be marked at positions corresponding to 1, 3, 9, and 27. This helps visualize the intervals that divide 27 evenly.
How can factors of 27 be applied in data analysis?
In data analysis, factors of 27 can be used to group data points, create equal intervals for histograms, or simplify statistical calculations by reducing numbers to their factor components. This makes it easier to handle large datasets and perform accurate analyses.

