What is the fraction equivalent to 0.75?
3/4
1/2
2/3
1/4

Did you know that 25 percent can be listed as 1/4 or one-fourth? That is because of the transverse nature that both percentages and fractions share. Fractions are so deeply ingrained in our everyday life, that a lot of things use fractions to denote quantity.
A fraction is one of the valid forms of a quotient, the byproduct of the division between two numbers. The fraction has multiple uses outside of mathematics due to its shorthand form. Another common way to express fractions is through the usage of percentages or percent values.
The multiplication and division of fractions are very confusing yet important things a person should know or be familiar with. There are two primary methods of multiplying with fractions, cross multiplication is used you are searching for a missing value in an equation. While straight multiplication is used if you are just searching for the product between two fractions. If you are still confused about fractions, the operations of fractions, or the format of fractions you may use any of the fraction templates, samples, and examples listed above.
Begin by selecting a method based on the answer you are searching for. If you are looking for a missing number in a solution that is in the form of a fraction, then you may use and apply cross multiplication. But if you are searching for the product of two fractions then you will multiply as is.
After you have selected your method, you must check if both fraction’s denominator and the numerator can be simplified, which is the act of dividing both the denominator and the numerator with a common denominator. For example, if the fraction is 6/12 then you can simplify this fraction to 1/2 as 6 is the common denominator of both the values in the fraction.
When you have finished simplifying both the denominator and the numerator, then you will multiply both the numerator of the two fractions. For example, if we were to multiply 3/4 and 6/5, then the product will be 18/20. Just note that the product in fraction form should be simplified to its most simple form, in the case of the example 18/20 should be simplified to 9/10.
If you are looking for a missing value then you must cross multiply the fraction. To cross multiply you will need to multiply the first fraction’s denominator with the other fraction’s numerator, and then multiply the first fraction’s numerator with the other fraction’s denominator. To put it simply if x/y is to be cross multiplied with z/a, the resulting product should be xz = ya. For example, if we are looking at the value of x in the equation of 6/x = 7/8, the resulting product of the cross multiplication will be 7x = (8)(6) or 7x = 48.
A quotient can be written as a fraction or a decimal, which often creates confusion between both terms. A fraction represents the ratio between both numbers one acting as a numerator and the other as a denominator. Fractions often come in the form of X/Y (Where X is the numerator and Y is the denominator), which are vague representations of the quotient but are easier to read. While a decimal is the accurate representation of the quotient which can also be used to represent the values of infinite numbers. (e.g. the quotient of ten divided by three)
Fractions are best used when you don’t want to waste energy writing down or typing out the decimal value of a quotient. This is because fractions act as short-form versions of decimals, and has more real-life applications when compared to decimals. Unless stated otherwise, most of the time it is best to use fractions to represent the quotient between two or more numbers.
There are plenty of real-life examples and usages of fractions that we can see notice in our everyday lives. One of the most common usages of fractions can be found in the directions of various recipes, this is because fractions are more commonly used in measurements than decimals. Another common usage of fractions is the notation of progress found in any project because factions can also be expressed through percentages.
Fractions present themselves as one of the most important forms of a quotient. That is because of the various real-life applications of fractions that will make our lives more efficient and easy. In conclusion, we must know what fractions are as they can affect the overall efficiency and understanding of our everyday lives.
Did you know that 25 percent can be listed as 1/4 or one-fourth? That is because of the transverse nature that both percentages and fractions share. Fractions are so deeply ingrained in our everyday life, that a lot of things use fractions to denote quantity.

lrt.ednet.ns.ca
Details
File Format
Size: 34 KB

taxsuperandyou.gov.au
Details
File Format
Size: 46 KB
nflcacademy.com
Details
File Format
Size: 32 KB
uen.org
Details
File Format
Size: 30 KB
cpb-us-e1.wpmucdn.com
Details
File Format
Size: 16 KB

opsu.edu
Details
File Format
Size: 18 KB

ivyhawnschool.org
Details
File Format
Size: 41 KB

intel.com
Details
File Format
Size: 23 KB
pdst.ie
Details
File Format
Size: 46 KB

edugains.ca
Details
File Format
Size: 82 KB

doe.virginia.gov
Details
File Format
Size: 66 KB

moey.gov.jm
Details
File Format
Size: 70 KB

csx.com
Details
File Format
Size: 102 KB

sbq.org.br
Details
File Format
Size: 113 KB
lsco.edu
Details
File Format
Size: 93 KB

intensiveintervention.org
Details
File Format
Size: 45 KB

students.flinders.edu.au
Details
File Format
Size: 63 KB

mav.delegateconnect.co
Details
File Format
Size: 91 KB

activemath.com
Details
File Format
Size: 42 KB
webcontent.indianhills.edu
Details
File Format
Size: 31 KB

amt.copernicus.org
Details
File Format
Size: 50 KB
personal.maths.surrey.ac.uk
Details
File Format
Size: 20 KB
ece.uccs.edu
Details
File Format
Size: 44 KB

math.purdue.edu
Details
File Format
Size: 33 KB

westerville.k12.oh.us
Details
File Format
Size: 85 KB
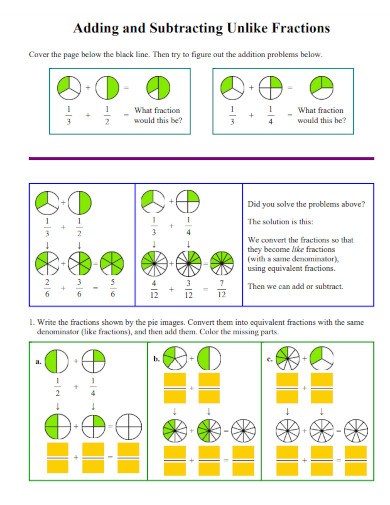
k5learning.com
Details
File Format
Size: 71 KB
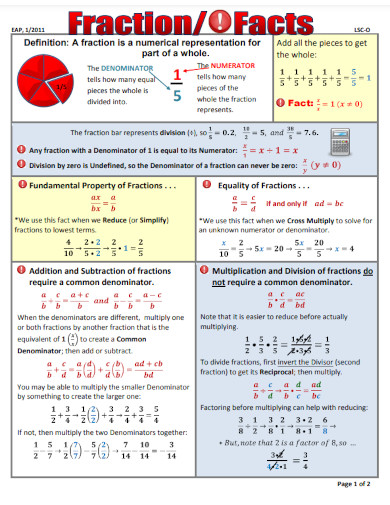
A fraction is one of the valid forms of a quotient, the byproduct of the division between two numbers. The fraction has multiple uses outside of mathematics due to its shorthand form. Another common way to express fractions is through the usage of percentages or percent values.
The multiplication and division of fractions are very confusing yet important things a person should know or be familiar with. There are two primary methods of multiplying with fractions, cross multiplication is used you are searching for a missing value in an equation. While straight multiplication is used if you are just searching for the product between two fractions. If you are still confused about fractions, the operations of fractions, or the format of fractions you may use any of the fraction templates, samples, and examples listed above.
Begin by selecting a method based on the answer you are searching for. If you are looking for a missing number in a solution that is in the form of a fraction, then you may use and apply cross multiplication. But if you are searching for the product of two fractions then you will multiply as is.
After you have selected your method, you must check if both fraction’s denominator and the numerator can be simplified, which is the act of dividing both the denominator and the numerator with a common denominator. For example, if the fraction is 6/12 then you can simplify this fraction to 1/2 as 6 is the common denominator of both the values in the fraction.
When you have finished simplifying both the denominator and the numerator, then you will multiply both the numerator of the two fractions. For example, if we were to multiply 3/4 and 6/5, then the product will be 18/20. Just note that the product in fraction form should be simplified to its most simple form, in the case of the example 18/20 should be simplified to 9/10.
If you are looking for a missing value then you must cross multiply the fraction. To cross multiply you will need to multiply the first fraction’s denominator with the other fraction’s numerator, and then multiply the first fraction’s numerator with the other fraction’s denominator. To put it simply if x/y is to be cross multiplied with z/a, the resulting product should be xz = ya. For example, if we are looking at the value of x in the equation of 6/x = 7/8, the resulting product of the cross multiplication will be 7x = (8)(6) or 7x = 48.
A quotient can be written as a fraction or a decimal, which often creates confusion between both terms. A fraction represents the ratio between both numbers one acting as a numerator and the other as a denominator. Fractions often come in the form of X/Y (Where X is the numerator and Y is the denominator), which are vague representations of the quotient but are easier to read. While a decimal is the accurate representation of the quotient which can also be used to represent the values of infinite numbers. (e.g. the quotient of ten divided by three)
Fractions are best used when you don’t want to waste energy writing down or typing out the decimal value of a quotient. This is because fractions act as short-form versions of decimals, and has more real-life applications when compared to decimals. Unless stated otherwise, most of the time it is best to use fractions to represent the quotient between two or more numbers.
There are plenty of real-life examples and usages of fractions that we can see notice in our everyday lives. One of the most common usages of fractions can be found in the directions of various recipes, this is because fractions are more commonly used in measurements than decimals. Another common usage of fractions is the notation of progress found in any project because factions can also be expressed through percentages.
Fractions present themselves as one of the most important forms of a quotient. That is because of the various real-life applications of fractions that will make our lives more efficient and easy. In conclusion, we must know what fractions are as they can affect the overall efficiency and understanding of our everyday lives.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
What is the fraction equivalent to 0.75?
3/4
1/2
2/3
1/4
Which of the following fractions is in simplest form?
6/9
15/20
8/10
5/7
What is the result of adding 14 and 24?
38
12
58
14
What is 56 minus 13?
38
23
34
14
What is 35 divided by 15?
31
13
35
53
What is the equivalent fraction of 812?
46
23
12
35
Which of the following fractions is the largest?
16
27
38
15
If ab=35, what is ab×5?
31
155
153
53
What is 34 multiplied by 23?
612
12
13
23
What is 915 simplified?
35
25
13
34
Before you leave, take our quick quiz to enhance your learning!

