GCF of 12 and 18
GCF of 12 and 18
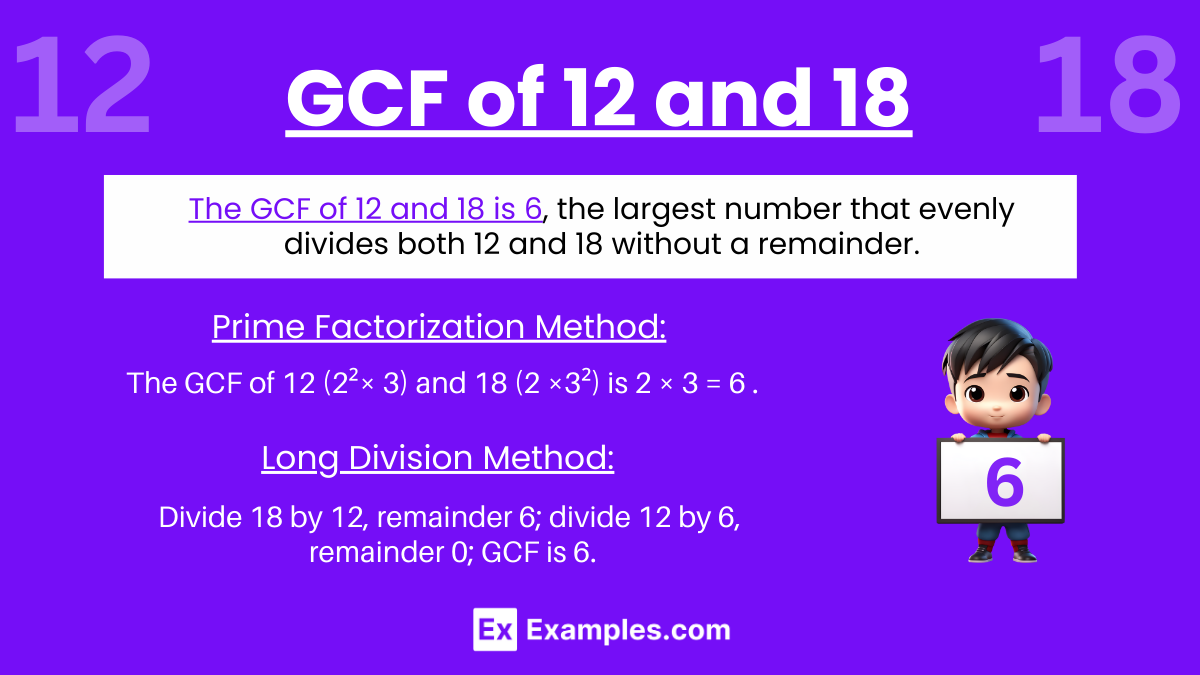
The greatest common factor (GCF) of 12 and 18 is 6. To find the GCF, you can identify the common factors of both numbers and select the greatest one. For 12, the factors are 1, 2, 3, 4, 6, and 12. For 18, the factors are 1, 2, 3, 6, 9, and 18. The greatest common factor among them is 6, as it’s the largest number that divides both 12 and 18 without leaving a remainder. This means that 6 is the largest number that can evenly divide both 12 and 18, making it their greatest common factor.
GCF of 12 and 18
GCF of 12 and 18 is 6.
The GCF of 12 and 18 is 6. By identifying their common factors (1, 2, 3, and 6), we find 6 as the largest number that divides both without remainder. This value aids in simplifying fractions and other mathematical calculations involving these numbers.
Methods to Find GCF of 12 and 18
- Prime Factorization Method
- Long Division Method
- Listing Common Factors
GCF of 12 and 18 by Prime Factorization Method
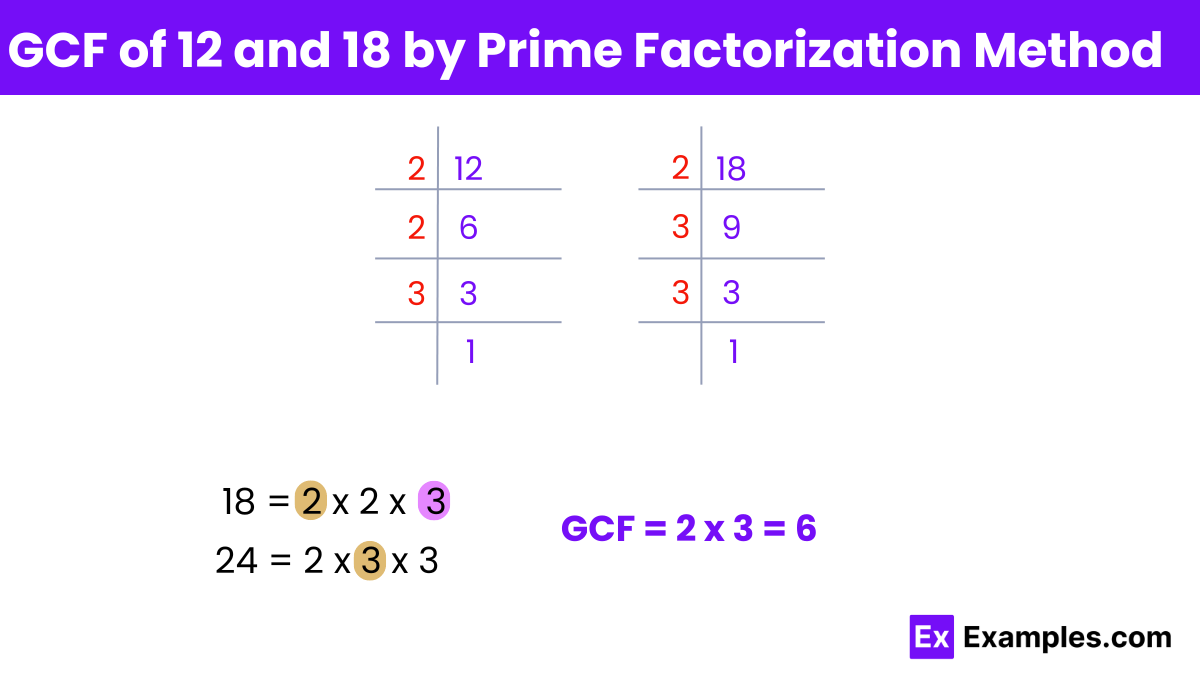
Step 1: Prime factorize each number.
- Prime factorization of 12: 12 = 2 × 2 × 3
- Prime factorization of 18: 18 = 2 × 3 × 3
Step 2: Identify common prime factors.
- The common prime factors are 2 and 3.
Step 3: Multiply the common prime factors.
2 × 3 = 6
So, the GCF of 12 and 18 is 6.
GCF of 12 and 18 by Long Division Method.
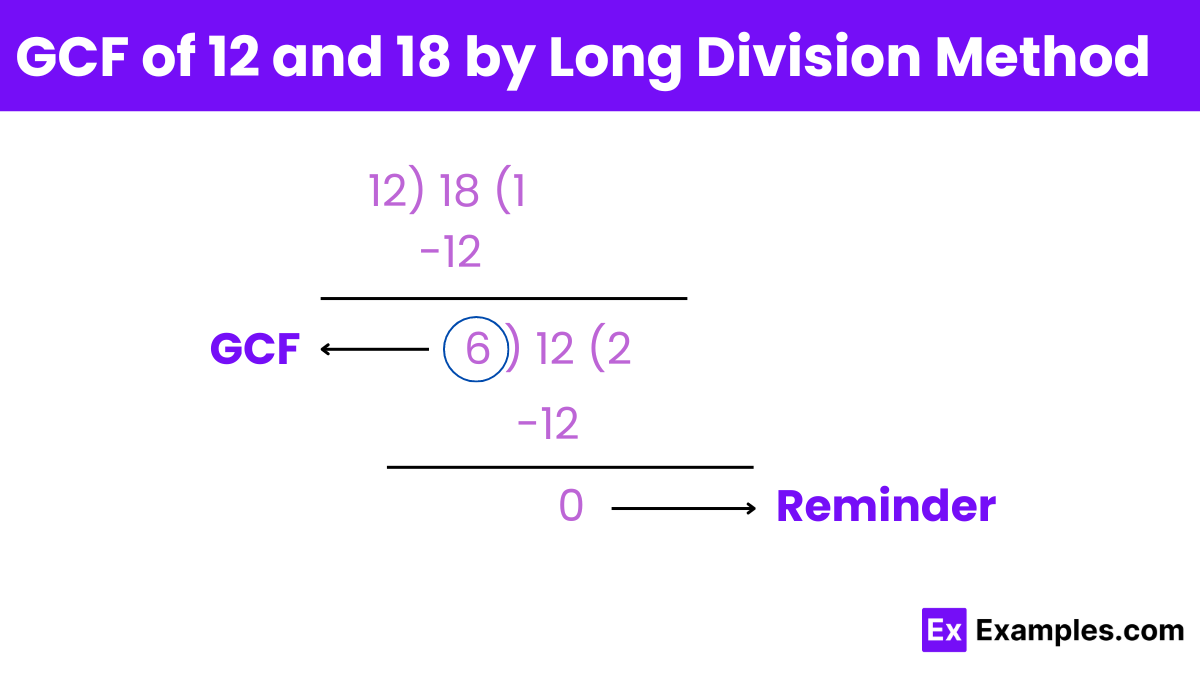
Step 1: We divide 18 (the larger number) by 12 (the smaller number). This yields a quotient of 1 and a remainder of 6.
Step 2: As the remainder (6) is not 0, we continue by dividing the divisor from the previous step (12) by the remainder (6). This results in a quotient of 2 and no remainder.
Step 3: Since the remainder is now 0, we stop. The divisor from the last division, which is 6, represents the GCF of 12 and 18.
Thus, the GCF of 12 and 18 is 6.
GCF of 12 and 18 by Listing Common Factors
- Factors of 12: 1, 2, 3, 4, 6, 12
- Factors of 18: 1, 2, 3, 6, 9, 18
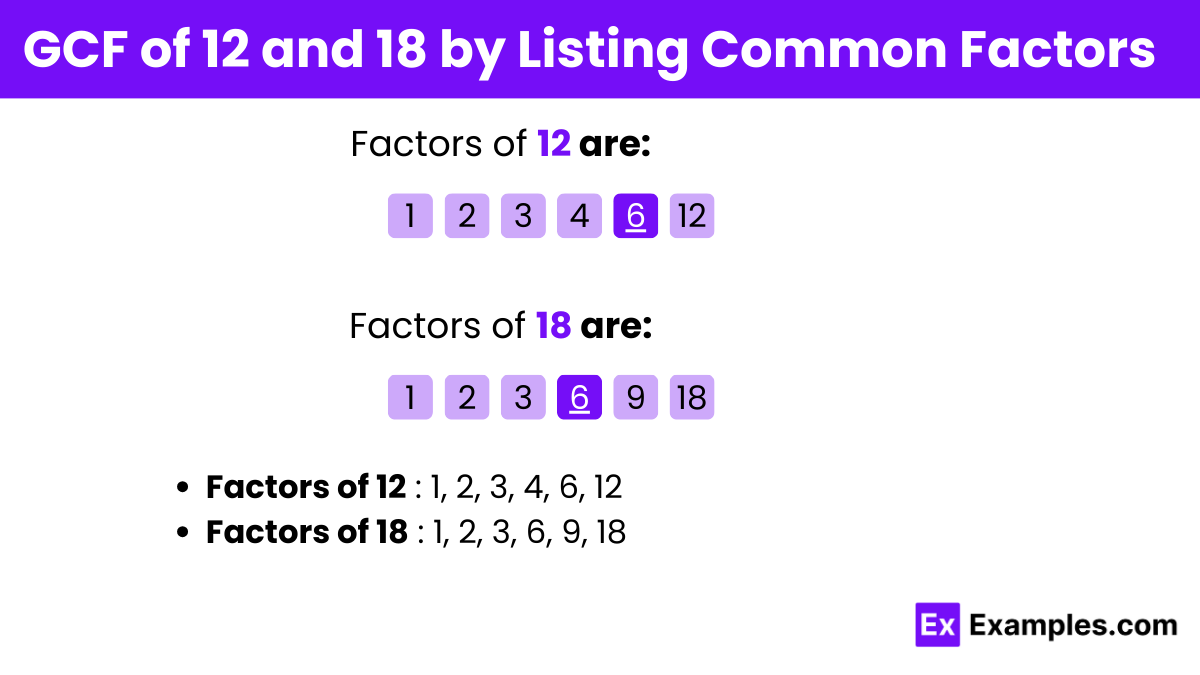
To find the greatest common factor (GCF) of 12 and 18 by listing common factors, identify numbers that divide both 12 and 18, yielding 1, 2, 3, and 6. The largest common factor, 6, is the GCF.
How is the GCF calculated?
The GCF is calculated by identifying the largest number that divides evenly into two or more numbers.
Why is finding the GCF important?
Finding the GCF is important for simplifying fractions, finding common denominators, and solving various mathematical problems.
How can the GCF be useful in everyday math?
The GCF is useful for simplifying calculations involving fractions, ratios, and proportions.
What is the greatest common multiple of 12 and 18?
The greatest common multiple of 12 and 18 is 36, the smallest number divisible by both without remainder.
What is the lowest common factor of 12 and 18?
The lowest common factor of 12 and 18 is 1, as it’s the smallest positive integer that divides both numbers without leaving a remainder.
Share :
