The difference between the GCF of 12 and 30 and 8 is?
2
4
6
8

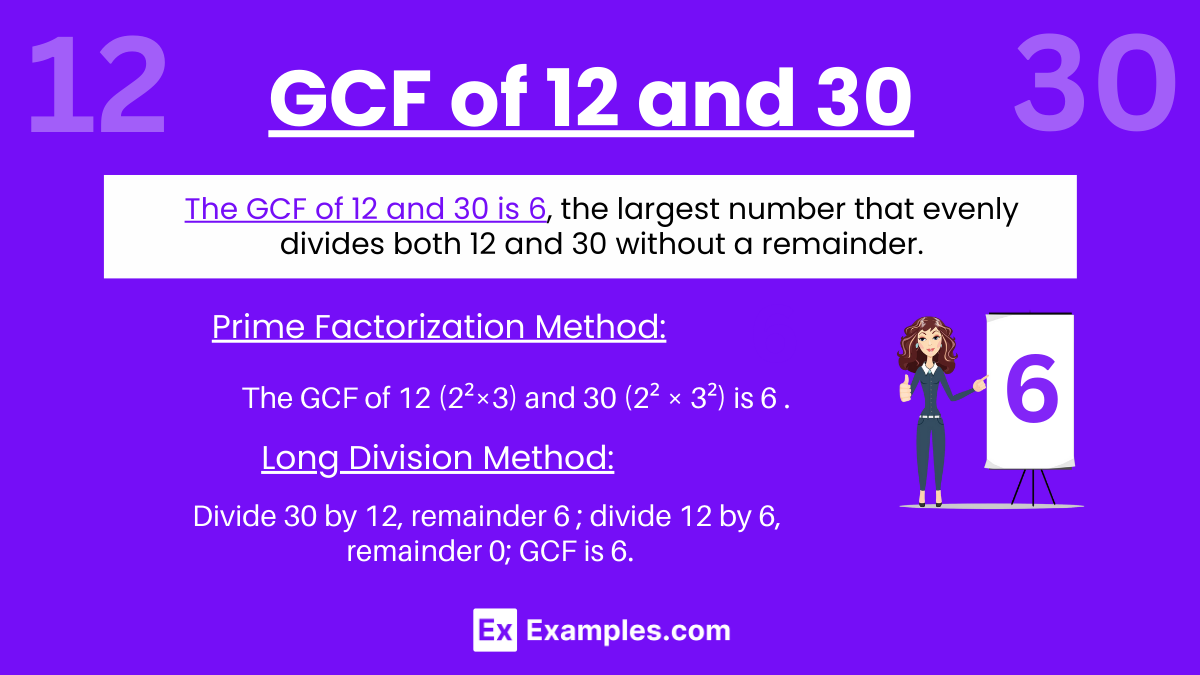
The greatest common factor (GCF) of 12 and 30 is 6. This value is found using various methods such as prime factorization, listing common factors, or employing the Euclidean algorithm. By prime factorizing, 12 breaks down into 2²×3 and 30 into 2×3×5. The common prime factors are 2 and 3, with their lowest powers being 2 and 3 respectively, giving 2¹×3¹=6. Alternatively, listing the factors shows that 12 (1, 2, 3, 4, 6, 12) and 30 (1, 2, 3, 5, 6, 10, 15, 30) share the factors 1, 2, 3, and 6, with 6 being the largest. The Euclidean algorithm, using successive divisions, would also result in finding 6 as the GCF, further confirming it as the largest number that divides both 12 and 30 without leaving a remainder.
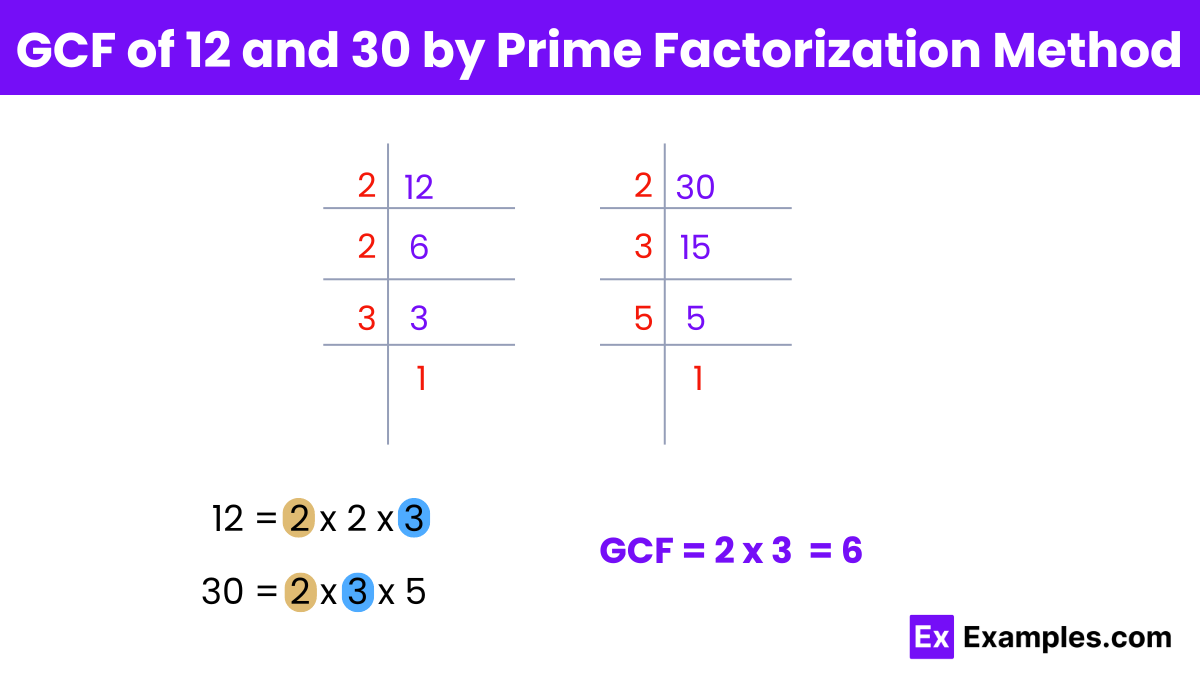
To find the greatest common factor (GCF) of 12 and 30 using the prime factorization method:
Step 1: Prime factorize both numbers:
For 12: 12 =2²×3
For 30: 30 =2 × 3 × 5
Step 2: Identify the common prime factors and their lowest powers:
Both 12 and 30 have the common prime factors of 2 and 3. The lowest power for each common factor is:
2¹ (since the lower of 2² and 2¹ is 2¹)
3¹ (since the lower of 3¹ in both factorizations is 3¹)
Step 3: Multiply the common prime factors with their lowest powers to determine the GCF:
GCF = 2¹ × 3¹ = 2 × 3 = 6
Therefore, the greatest common factor (GCF) of 12 and 30 by prime factorization method is 6.
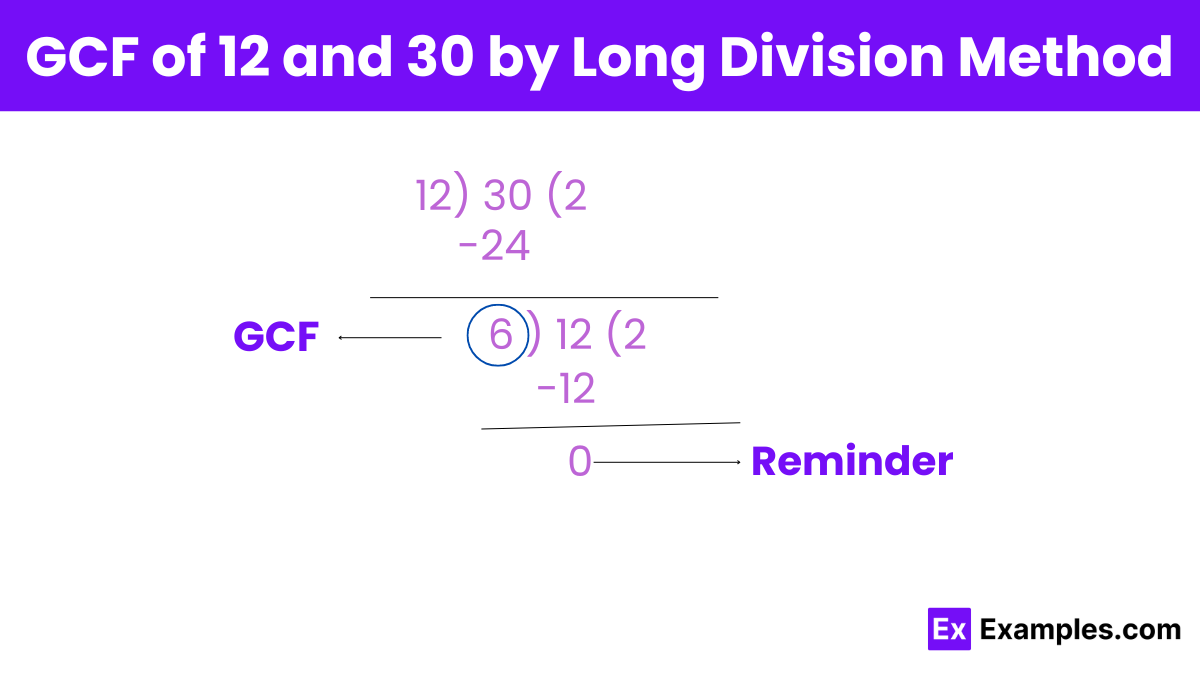
To find the greatest common factor (GCF) of 12 and 30 using the long division method:
Step 1: Start by dividing the larger number (30) by the smaller number (12).
30 ÷ 12 = 2 with a remainder of 6.
Step 2: Then, take the divisor (12) and divide it by the remainder (6).
12 ÷ 6 = 2 with a remainder of 0.
Since the remainder is now 0, the division process stops here.
Step 3: The divisors at this step where the remainder becomes zero is the greatest common factor (GCF).
GCF = 6.
Therefore, the greatest common factor (GCF) of 12 and 30 by the long division method is 6.
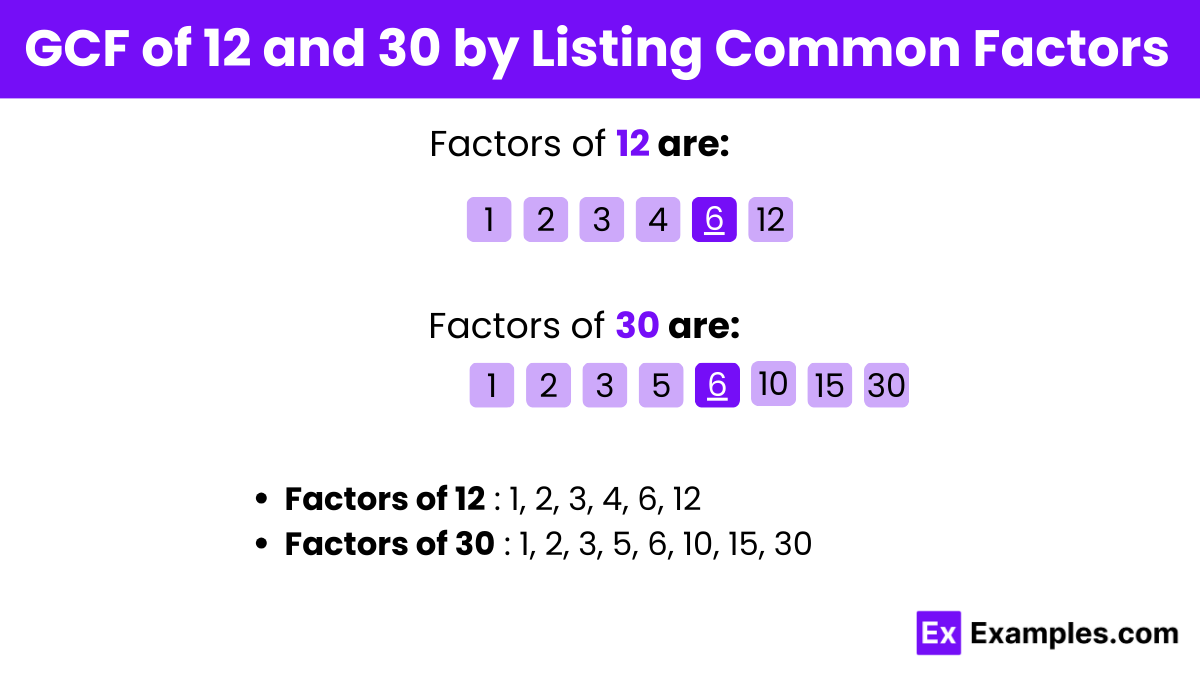
Step 1: List the factors of each number.
Factors of 12: 1, 2, 3, 4, 6, 12
Factors of 30: 1, 2, 3, 5, 6, 10, 15, 30
Step 2: Identify the common factors.
Common factors: 1, 2, 3, 6
Step 3: Determine the greatest common factor. GCF=6. \text{GCF} = 6.GCF=6.
Therefore, the greatest common factor (GCF) of 12 and 30 by listing common factors is 6.
Yes, by prime factorizing 12 and 30 and identifying the lowest common prime factors.
No, the GCF cannot be larger than the smaller of the two numbers involved.
The Euclidean algorithm, often implemented through long division, can efficiently find the GCF.
LCM (Least Common Multiple) is another concept related to GCF.
Frequently in areas like engineering, computing, and when working with proportions.
Yes, using Venn diagrams with prime factor circles can visually represent common factors.

The greatest common factor (GCF) of 12 and 30 is 6. This value is found using various methods such as prime factorization, listing common factors, or employing the Euclidean algorithm. By prime factorizing, 12 breaks down into 2²×3 and 30 into 2×3×5. The common prime factors are 2 and 3, with their lowest powers being 2 and 3 respectively, giving 2¹×3¹=6. Alternatively, listing the factors shows that 12 (1, 2, 3, 4, 6, 12) and 30 (1, 2, 3, 5, 6, 10, 15, 30) share the factors 1, 2, 3, and 6, with 6 being the largest. The Euclidean algorithm, using successive divisions, would also result in finding 6 as the GCF, further confirming it as the largest number that divides both 12 and 30 without leaving a remainder.
To find the greatest common factor (GCF) of 12 and 30 using the prime factorization method:
Step 1: Prime factorize both numbers:
For 12: 12 =2²×3
For 30: 30 =2 × 3 × 5
Step 2: Identify the common prime factors and their lowest powers:
Both 12 and 30 have the common prime factors of 2 and 3. The lowest power for each common factor is:
2¹ (since the lower of 2² and 2¹ is 2¹)
3¹ (since the lower of 3¹ in both factorizations is 3¹)
Step 3: Multiply the common prime factors with their lowest powers to determine the GCF:
GCF = 2¹ × 3¹ = 2 × 3 = 6
Therefore, the greatest common factor (GCF) of 12 and 30 by prime factorization method is 6.
To find the greatest common factor (GCF) of 12 and 30 using the long division method:
Step 1: Start by dividing the larger number (30) by the smaller number (12).
30 ÷ 12 = 2 with a remainder of 6.
Step 2: Then, take the divisor (12) and divide it by the remainder (6).
12 ÷ 6 = 2 with a remainder of 0.
Since the remainder is now 0, the division process stops here.
Step 3: The divisors at this step where the remainder becomes zero is the greatest common factor (GCF).
GCF = 6.
Therefore, the greatest common factor (GCF) of 12 and 30 by the long division method is 6.
Step 1: List the factors of each number.
Factors of 12: 1, 2, 3, 4, 6, 12
Factors of 30: 1, 2, 3, 5, 6, 10, 15, 30
Step 2: Identify the common factors.
Common factors: 1, 2, 3, 6
Step 3: Determine the greatest common factor. GCF=6. \text{GCF} = 6.GCF=6.
Therefore, the greatest common factor (GCF) of 12 and 30 by listing common factors is 6.
Yes, by prime factorizing 12 and 30 and identifying the lowest common prime factors.
No, the GCF cannot be larger than the smaller of the two numbers involved.
The Euclidean algorithm, often implemented through long division, can efficiently find the GCF.
LCM (Least Common Multiple) is another concept related to GCF.
Frequently in areas like engineering, computing, and when working with proportions.
Yes, using Venn diagrams with prime factor circles can visually represent common factors.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
The difference between the GCF of 12 and 30 and 8 is?
2
4
6
8
If you multiply the GCF of 12 and 30 by 4, what is the result?
18
24
36
30
What is the difference between the GCF of 12 and 30 and the number 5?
1
2
3
4
What is the GCF of 12 and 30 when divided by 6?
1
2
3
4
The GCF of 12 and 30 is also a factor of which of the following numbers?
48
60
72
90
Which of the following numbers is not divisible by the GCF of 12 and 30?
18
24
30
40
What is the result of dividing the GCF of 12 and 30 by 3?
1
2
3
4
Which pair of numbers has the same GCF as 12 and 30?
18 and 24
15 and 25
12 and 15
20 and 30
How many factors does the GCF of 12 and 30 have?
2
3
4
6
If you subtract the GCF of 12 and 30 from 30, what is the result?
20
22
24
26
Before you leave, take our quick quiz to enhance your learning!

