What is the GCF of 15 and 30?
5
10
15
30


The Greatest Common Factor (GCF) of 15 and 30 is the largest number that divides both numbers without leaving a remainder. To find the GCF, we can list the factors of each number: for 15, the factors are 1, 3, 5, and 15, while for 30, the factors are 1, 2, 3, 5, 6, 10, 15, and 30. The common factors are 1, 3, 5, and 15. The largest of these common factors is 15. Therefore, the GCF of 15 and 30 is 15, which is useful for simplifying fractions and solving problems involving these numbers.
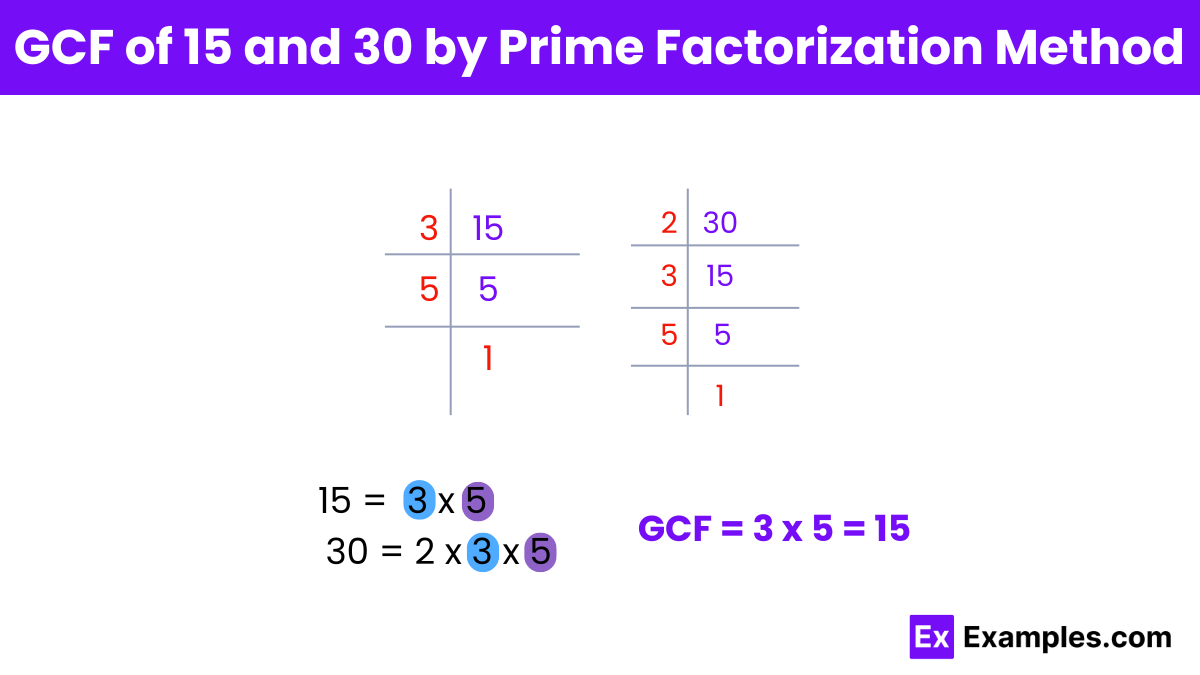
To find the Greatest Common Factor (GCF) of 15 and 30 using the prime factorization method, follow these steps:
Prime Factorization of Each Number:
Prime factors of 15:
15 = 3 × 5
Prime factors of 30:
30 = 2 × 3 × 5
Identify the Common Prime Factors:
The common prime factors are 3 and 5.
Multiply the Common Prime Factors:
GCF = 3 × 5
GCF = 15
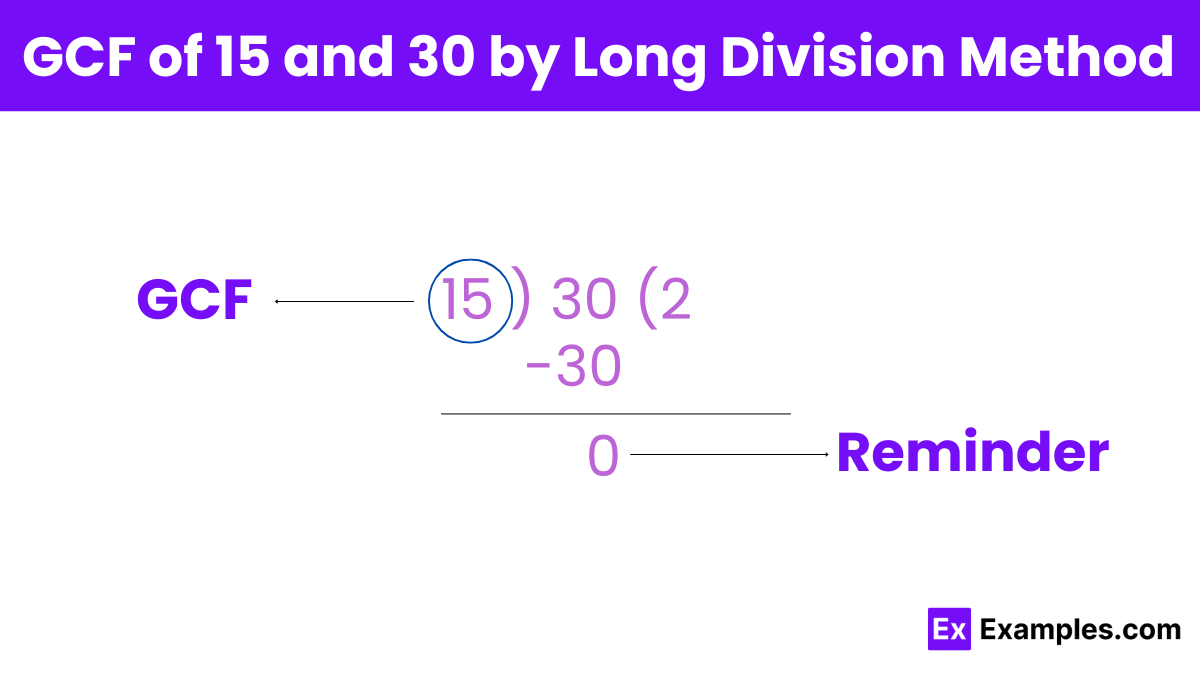
To find the Greatest Common Factor (GCF) of 15 and 30 using the Long Division Method, follow these steps:
Divide the Larger Number by the Smaller Number:
Divide 30 (larger number) by 15 (smaller number).
30 ÷ 15 = 2 remainder 0
Check the Remainder:
When the remainder is 0, the current divisors is the GCF.
The remainder is 0, and the current divisor is 15.
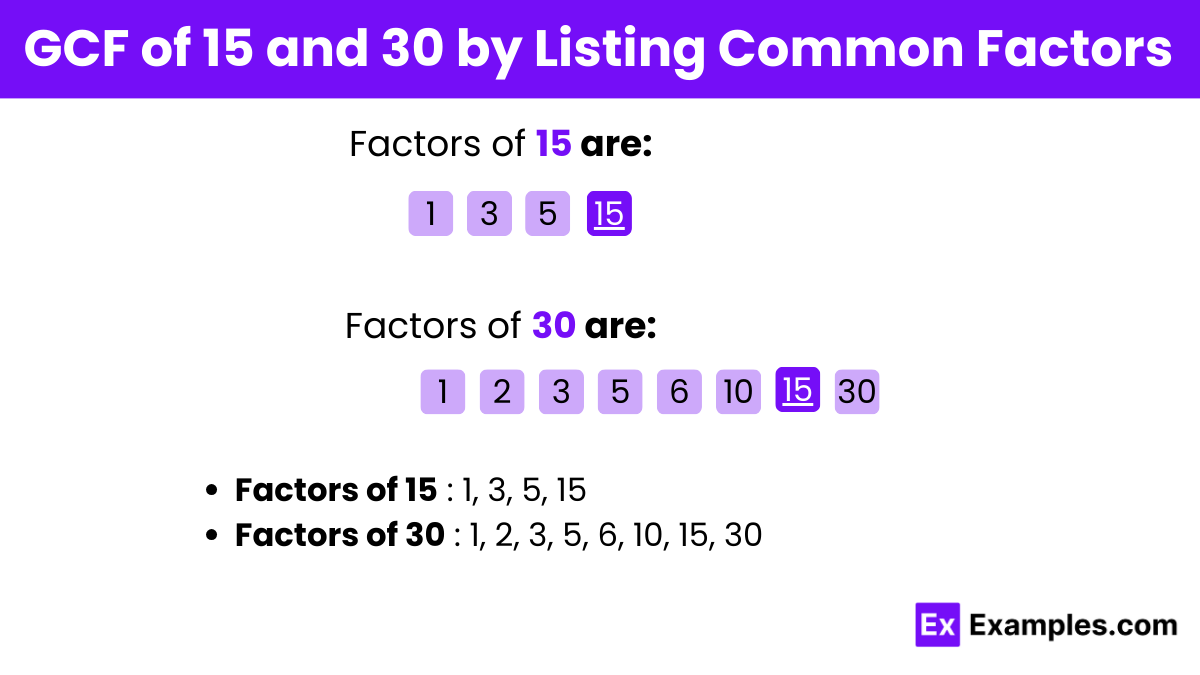
To find the Greatest Common Factor (GCF) of 15 and 30 by listing their common factors, follow these steps:
List the Factors of Each Number:
Factors of 15: 1, 3, 5, 15
Factors of 30: 1, 2, 3, 5, 6, 10, 15, 30
Identify the Common Factors:
The common factors of 15 and 30 are: 1, 3, 5, 15
Find the Greatest Common Factor:
The largest number in the list of common factors is 15.
The GCF is useful for simplifying fractions, solving problems involving ratios, and finding common denominators.
Yes, the Euclidean algorithm involves repeated division. Divide 30 by 15 to get a remainder of 0. The GCF is 15.
The product of the GCF and LCM of two numbers equals the product of the numbers. For 15 and 30: GCF × LCM = 15 × 30.
Yes, the Greatest Common Factor (GCF) and the Highest Common Factor (HCF) are the same, which is 15 for 15 and 30.
The GCF is used to simplify ratios. For example, the ratio 15:30 simplifies to 1:2 by dividing both terms by their GCF, which is 15.
The fastest way is often the Euclidean algorithm, which involves a series of divisions until the remainder is 0.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
What is the GCF of 15 and 30?
5
10
15
30
Which number is a common factor of both 15 and 30?
4
6
10
15
How many factors does the GCF of 15 and 30 have?
2
3
4
5
Which of the following numbers has the same GCF as 15 and 30?
20 and 30
45 and 60
25 and 35
30 and 40
What is the smallest prime factor of the GCF of 15 and 30?
2
3
5
7
The GCF of 15 and 30 is added to 5. What is the result?
20
25
30
35
What is the result when the GCF of 15 and 30 is multiplied by 2?
15
30
45
60
What is the GCF of 15, 30, and 45?
5
10
15
20
What is the sum of the GCF of 15 and 30 and the number 7?
12
15
22
25
The GCF of 15 and 30 is divided by 3. What is the result?
3
5
7
10
Before you leave, take our quick quiz to enhance your learning!

