What is the greatest common factor (GCF) of 18 and 24?
4
6
8
12

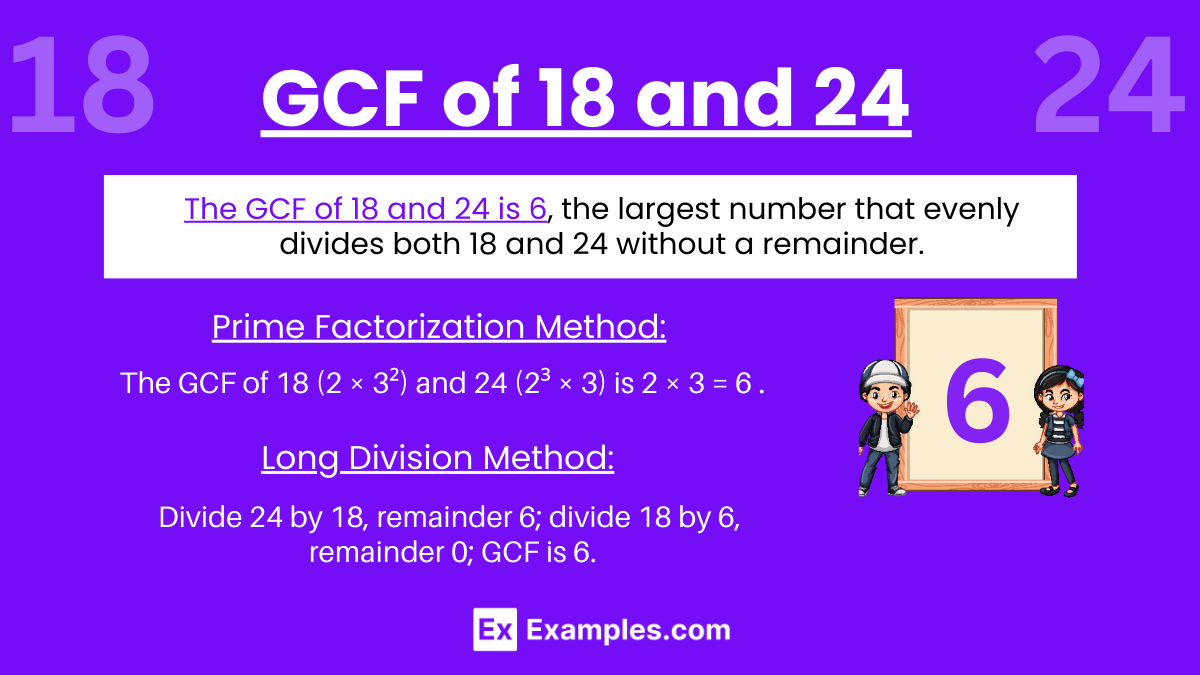
The Greatest Common Factor (GCF) of 18 and 24 is the highest number that can evenly divide both 18 and 24 without leaving a remainder. Understanding the concept of the GCF, also known as the Greatest Common Divisor (GCD), is essential in mathematics, especially in number theory and algebra. The factors of 18 include 1, 2, 3, 6, 9, and 18, while the factors of 24 are 1, 2, 3, 4, 6, 8, 12, and 24. The GCF is a foundational concept that helps simplify fractions, solve equations, and understand relationships between numbers. By using the prime factorization method, we find the common prime factors of 18 (2 × 3 × 3) and 24 (2 × 2 × 2 × 3), identifying the GCF as 6. used: the long division method, the prime factorization method, and the Euclidean algorithm.
The Greatest Common Factor (GCF) of 18 and 24 is 6. The simplest way to find it is by listing the factors of each number. The factors of 18 are 1, 2, 3, 6, 9, and 18; the factors of 24 are 1, 2, 3, 4, 6, 8, 12, and 24. The largest common factor is 6.
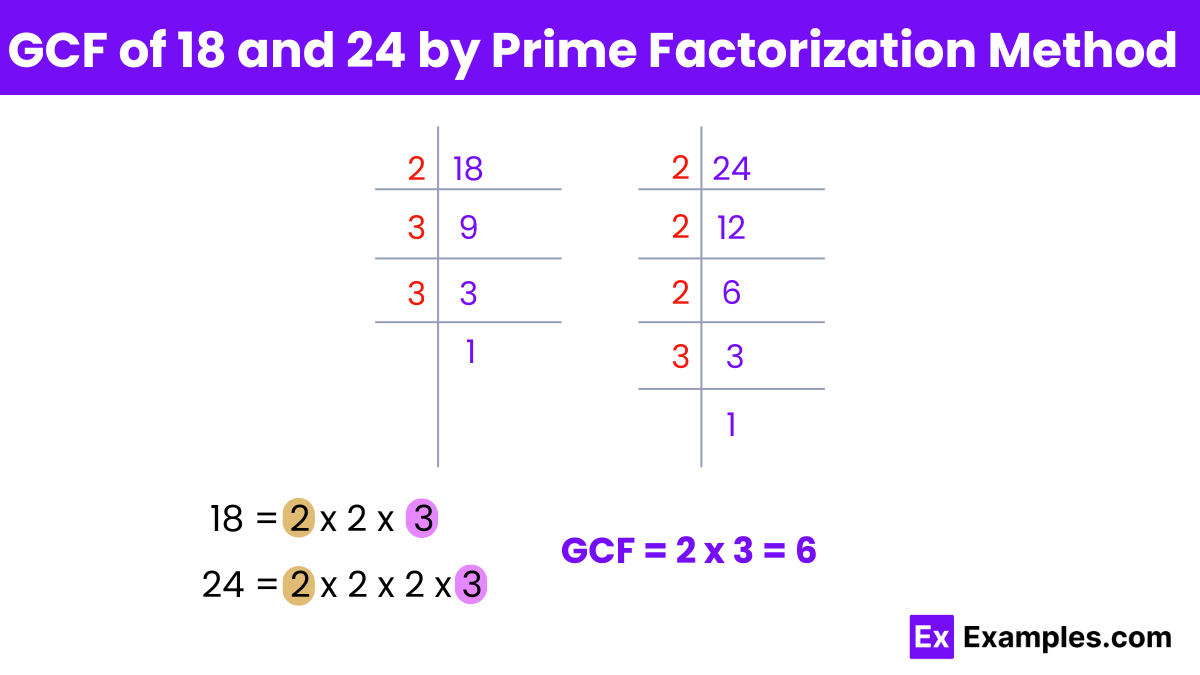
The prime factorization of 18 and 24 is (2 × 3 × 3) and (2 × 2 × 2 × 3) respectively. As visible, 18 and 24 have common prime factors. Hence, the HCF (Highest Common Factor) of 18 and 24 is 2 × 3 = 6.
HCF of 18 and 24 by Long Division:
Steps:
The corresponding divisor (6) is the HCF of 18 and 24.
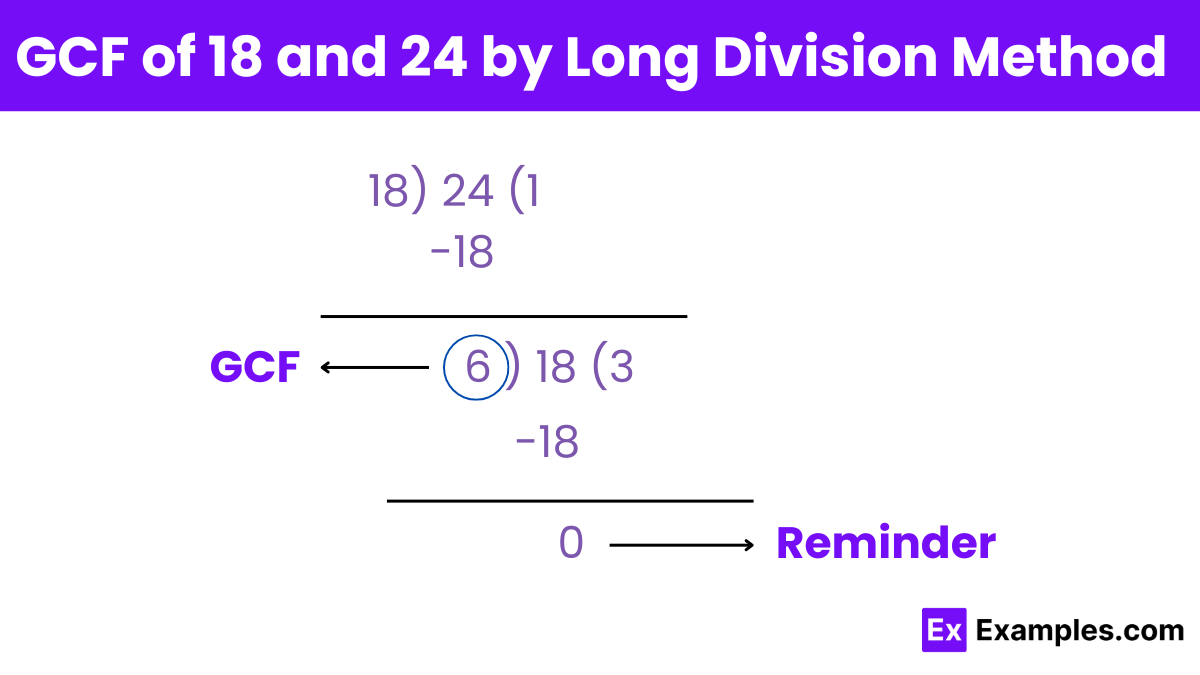
The GCF of 18 and 24 can be found using the long division method by following these steps:
Step 1: Divide 24 (the larger number) by 18 (the smaller number).
24 ÷ 18 = 1 remainder 6
Step 2: Use the divisor (18) from Step 1 and divide it by the remainder (6).
18 ÷ 6 = 3 remainder 0
Step 3: When the remainder becomes 0, the divisor at this step (6) is the GCF. The corresponding divisor, 6, is the GCF of 18 and 24.

To find the GCF (Greatest Common Factor) of 18 and 24 by listing common factors, follow these steps:
List the factors of 18:
Factors of 18: 1, 2, 3, 6, 9, 18
List the factors of 24:
Factors of 24: 1, 2, 3, 4, 6, 8, 12, 24
Identify the common factors:
Common factors of 18 and 24: 1, 2, 3, 6
Determine the greatest common factor:
The greatest common factor among these is 6.
Thus, the GCF of 18 and 24 is 6.
The LCM of 18 and 24 by prime factorization is found by multiplying the highest powers of all prime factors: 2³ ×3 = 72². Thus, LCM = 72.
A factor of both 24 and 18 is a number that evenly divides both without leaving a remainder. Common factors include 1, 2, 3, and 6.
Multiples of 18 include 18, 36, 54, 72, and 90. Multiples of 24 include 24, 48, 72, 96, and 120. Common multiples include 72 and 144.
The GCF is used to simplify fractions, solve problems involving ratios, and find common denominators in algebra.
No, the GCF of 18 and 24 cannot be greater than 6 because 6 is the largest number that can evenly divide both 18 and 24 without leaving a remainder.

The Greatest Common Factor (GCF) of 18 and 24 is the highest number that can evenly divide both 18 and 24 without leaving a remainder. Understanding the concept of the GCF, also known as the Greatest Common Divisor (GCD), is essential in mathematics, especially in number theory and algebra. The factors of 18 include 1, 2, 3, 6, 9, and 18, while the factors of 24 are 1, 2, 3, 4, 6, 8, 12, and 24. The GCF is a foundational concept that helps simplify fractions, solve equations, and understand relationships between numbers. By using the prime factorization method, we find the common prime factors of 18 (2 × 3 × 3) and 24 (2 × 2 × 2 × 3), identifying the GCF as 6. used: the long division method, the prime factorization method, and the Euclidean algorithm.
The Greatest Common Factor (GCF) of 18 and 24 is 6. The simplest way to find it is by listing the factors of each number. The factors of 18 are 1, 2, 3, 6, 9, and 18; the factors of 24 are 1, 2, 3, 4, 6, 8, 12, and 24. The largest common factor is 6.
Prime Factorization Method
Long Division Method
Listing Common Factors
The prime factorization of 18 and 24 is (2 × 3 × 3) and (2 × 2 × 2 × 3) respectively. As visible, 18 and 24 have common prime factors. Hence, the HCF (Highest Common Factor) of 18 and 24 is 2 × 3 = 6.
HCF of 18 and 24 by Long Division:
The HCF of 18 and 24 is the divisor we get when the remainder becomes 0 after repeatedly performing long division.
Steps:
Divide 24 (larger number) by 18 (smaller number).
Since the remainder is not 0, divide the divisor of step 1 (18) by the remainder (6).
Repeat this process until the remainder is 0.
The corresponding divisor (6) is the HCF of 18 and 24.
The GCF of 18 and 24 can be found using the long division method by following these steps:
Step 1: Divide 24 (the larger number) by 18 (the smaller number).
Calculation: 24 ÷ 18 = 1 with a remainder of 6.
24 ÷ 18 = 1 remainder 6
Step 2: Use the divisor (18) from Step 1 and divide it by the remainder (6).
Calculation: 18 ÷ 6 = 3 with a remainder of 0.
18 ÷ 6 = 3 remainder 0
Step 3: When the remainder becomes 0, the divisor at this step (6) is the GCF. The corresponding divisor, 6, is the GCF of 18 and 24.
To find the GCF (Greatest Common Factor) of 18 and 24 by listing common factors, follow these steps:
List the factors of 18:
Factors of 18: 1, 2, 3, 6, 9, 18
List the factors of 24:
Factors of 24: 1, 2, 3, 4, 6, 8, 12, 24
Identify the common factors:
Common factors of 18 and 24: 1, 2, 3, 6
Determine the greatest common factor:
The greatest common factor among these is 6.
Thus, the GCF of 18 and 24 is 6.
The LCM of 18 and 24 by prime factorization is found by multiplying the highest powers of all prime factors: 2³ ×3 = 72². Thus, LCM = 72.
A factor of both 24 and 18 is a number that evenly divides both without leaving a remainder. Common factors include 1, 2, 3, and 6.
Multiples of 18 include 18, 36, 54, 72, and 90. Multiples of 24 include 24, 48, 72, 96, and 120. Common multiples include 72 and 144.
The GCF is used to simplify fractions, solve problems involving ratios, and find common denominators in algebra.
No, the GCF of 18 and 24 cannot be greater than 6 because 6 is the largest number that can evenly divide both 18 and 24 without leaving a remainder.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
What is the greatest common factor (GCF) of 18 and 24?
4
6
8
12
Which of the following is NOT a common factor of 18 and 24?
2
3
6
8
If you list the factors of 18 and 24, which number is the greatest common factor?
3
6
9
12
What is the GCF of 18 and 24 using prime factorization?
2
3
6
12
Which method can be used to find the GCF of 18 and 24?
Listing multiples
Prime factorization
Subtraction
Division
What is the largest number that can exactly divide both 18 and 24?
2
4
6
12
How do you find the GCF of 18 and 24 using the Euclidean algorithm?
Divide 24 by 18
Divide 18 by 24
Subtract 18 from 24
Add 18 and 24
Which of the following pairs has the same GCF as 18 and 24?
20 and 30
36 and 48
14 and 21
22 and 28
If 18 and 24 are both divided by their GCF, what is the result?
1 and 1
2 and 4
3 and 4
3 and 6
What is the GCF of 18 and 24 if both numbers are multiplied by 2?
6
12
24
36
Before you leave, take our quick quiz to enhance your learning!

