What is the GCF of 24 and 36?
6
12
18
24
of 10
GCF of 24 and 36 – How to find in Different Ways
GCF of 24 and 36 – How to find in Different Ways
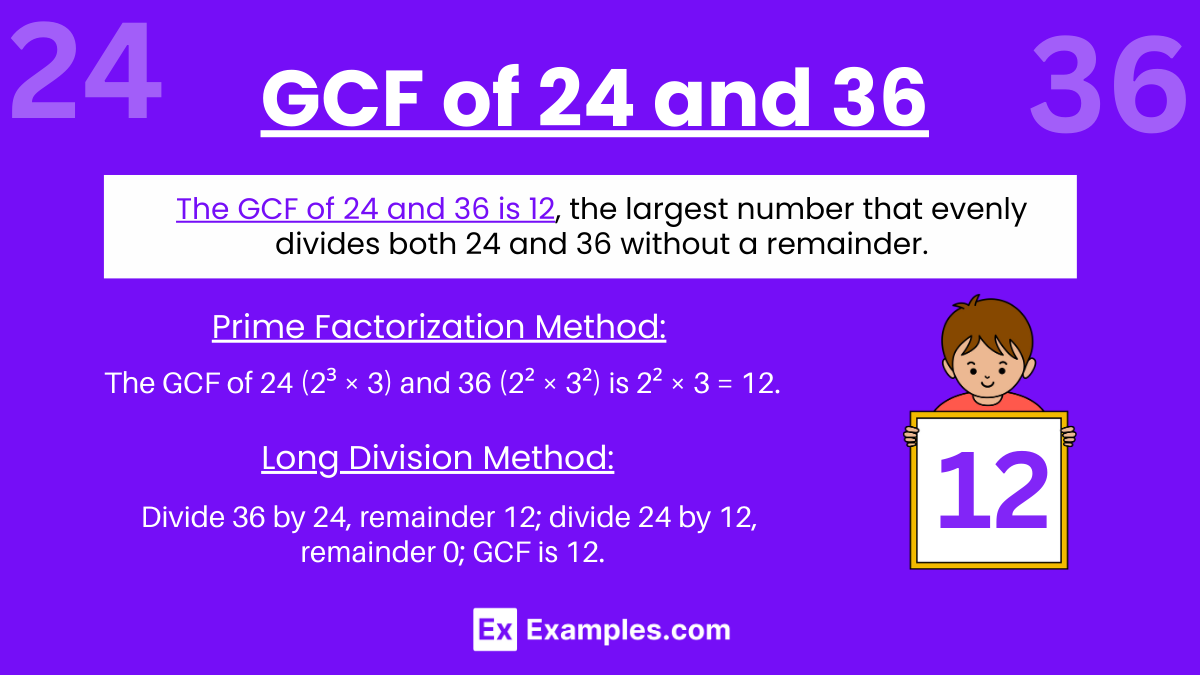
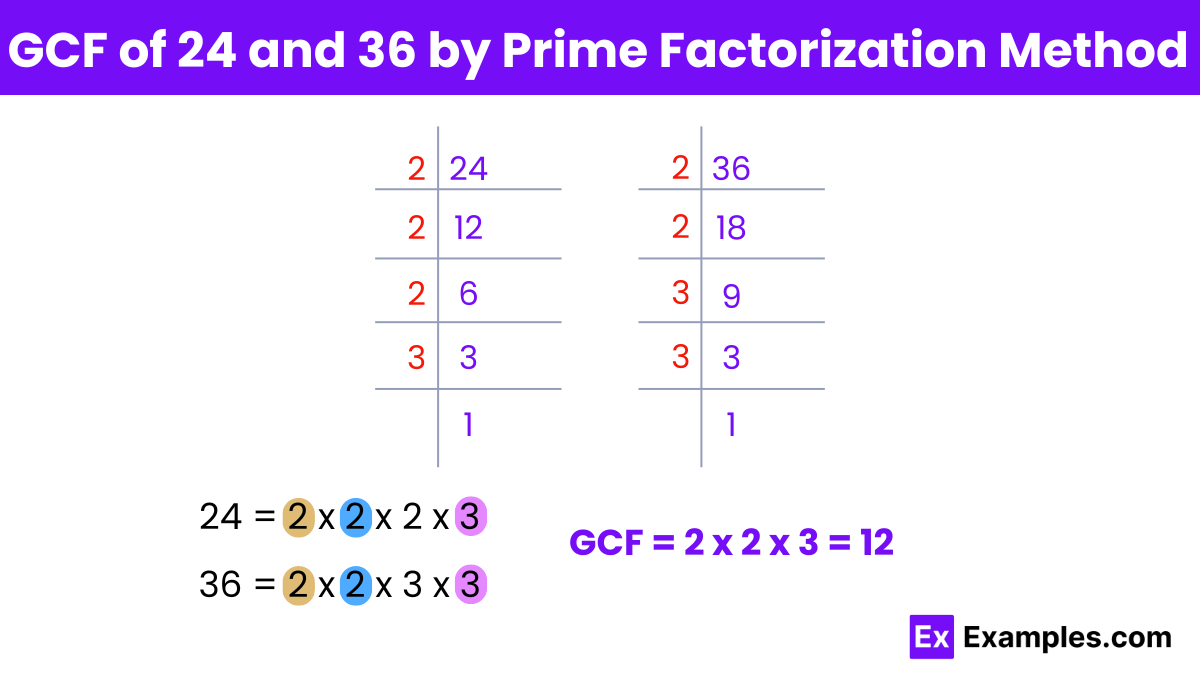
The Greatest Common Factor (GCF) of 24 and 36 is the highest number that can evenly divide both 24 and 36 without leaving a remainder. Understanding the concept of the GCF, also known as the Greatest Common Divisor (GCD), is essential in mathematics, especially in number theory and algebra. The factors of 24 include 1, 2, 3, 4, 6, 8, 12, and 24, while the factors of 36 are 1, 2, 3, 4, 6, 9, 12, 18, and 36. The GCF is a foundational concept that helps simplify fractions, solve equations, and understand relationships between numbers. To find the GCF of 24 and 36, three popular methods are used: the long division method, the prime factorization method, and the Euclidean algorithm.
GCF of 24 and 36
GCF of 24 and 36 is 12.
The factors of 24 are 1, 2, 3, 4, 6, 8, 12, and 24, while the factors of 36 are 1, 2, 3, 4, 6, 9, 12, 18, and 36. The Greatest Common Factor (GCF) of two non-zero integers, 24 and 36, is the largest positive integer, 12, that can divide both 24 and 36 without leaving a remainder.
Methods to Find GCF of 24 and 36
- Prime Factorization Method
- Long Division Method
- Listing Common Factors
GCF of 24 and 36 by Prime Factorization Method
- Prime factorization of 24: 2 × 2 × 2 × 3
- Prime factorization of 36: 2 × 2 × 3 × 3
- Both 24 and 36 have common prime factors: 2 and 3
- The GCF is found by multiplying these common prime factors: 2 × 2 × 3 = 12
- Hence, the GCF of 24 and 36 is 12
GCF of 24 and 36 by Long Division Method
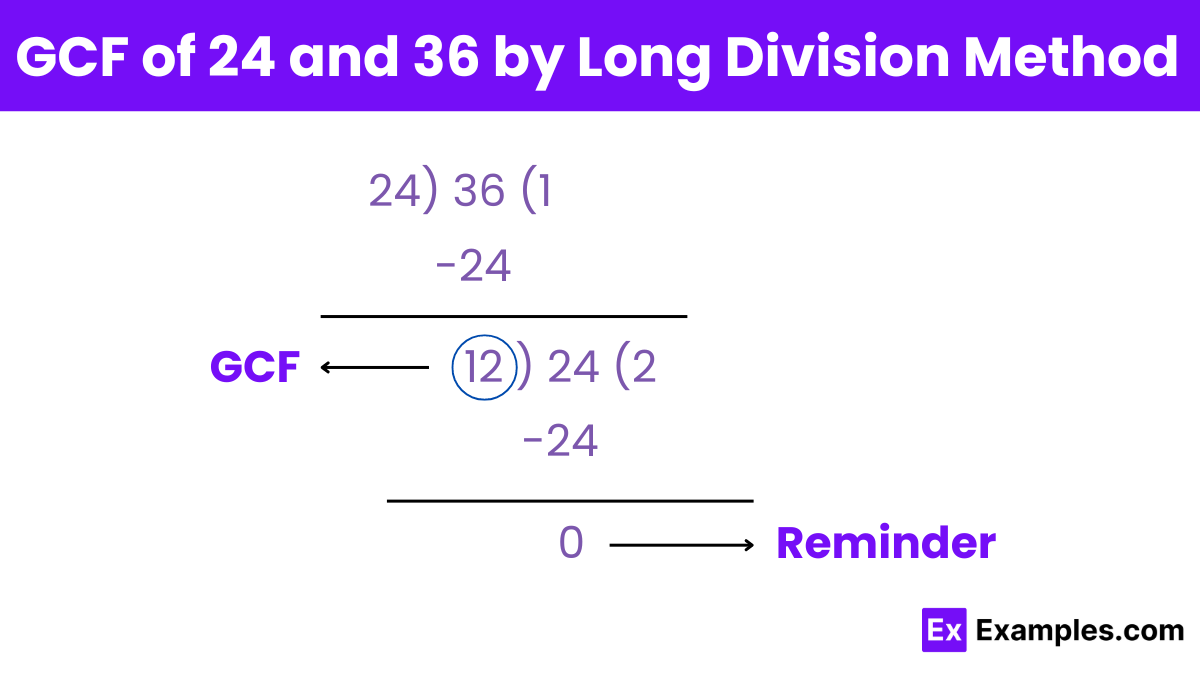
The long division method is a straightforward approach to find the Greatest Common Factor (GCF) of two numbers. Here are the steps to find the GCF of 24 and 36 using this method:
- Divide the Larger Number by the Smaller Number:
- Step 1: Divide 36 (larger number) by 24 (smaller number).
- Result: The quotient is 1, and the remainder is 12.
- 36÷24=136÷24=1 with a remainder of 12.
- Replace the Larger Number with the Smaller Number:
- Step 2: Now, replace the larger number (36) with the smaller number (24), and the smaller number (24) with the remainder from the previous division (12).
- Repeat the Division Process:
- Step 3: Divide 24 by 12.
- Result: The quotient is 2, and the remainder is 0.
- 24÷12=224÷12=2 with a remainder of 0.
- Identify the GCF:
- Step 4: Since the remainder is now 0, the divisor at this stage (12) is the GCF.
- GCF: 12
GCF of 24 and 36 by Listing Common Factors
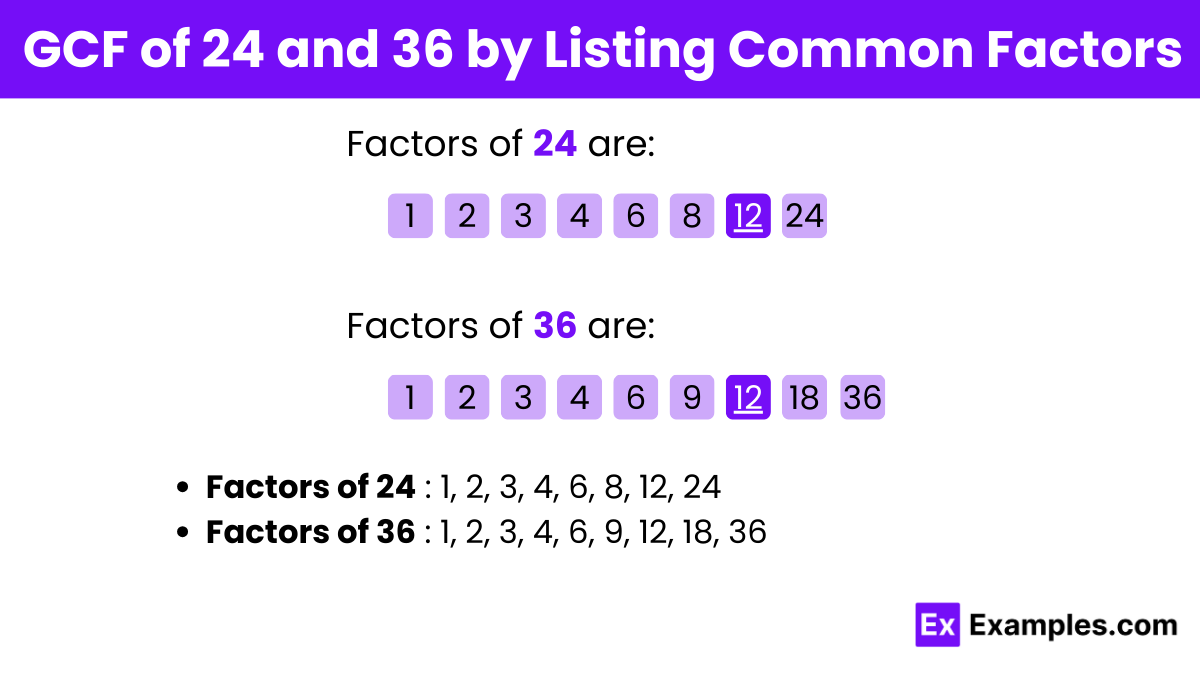
The numbers 24 and 36 share six common factors: 1, 2, 3, 4, 6, and 12. Among these, the largest common factor is 12. Therefore, the greatest common factor (GCF) of 24 and 36 is 12.
Is 24 a factor of 36?
No, 24 is not a factor of 36 because 36 divided by 24 does not result in an integer without a remainder.
What is the lcm of 24 and 36?
The LCM of 24 and 36 is 72, calculated by finding the least common multiple of their prime factorizations or using the formula LCM = (24 × 36) / GCF.
Why is the GCF of 24 and 36 important?
The GCF helps in simplifying fractions and solving problems involving divisibility.
Can you use the Euclidean algorithm to find the GCF of 24 and 36?
Yes, using the Euclidean algorithm, the GCF is found by repeated subtraction or division.
Is the GCF of 24 and 36 the same as the GCD?
Yes, the GCF (Greatest Common Factor) and GCD (Greatest Common Divisor) are the same.
Share :
Practice Test
Which of the following numbers is a factor of both 24 and 36?
8
14
20
22
of 10
If you divide 24 and 36 by their GCF, what do you get?
2 and 3
4 and 6
6 and 9
12 and 18
of 10
What is the result of multiplying the GCF of 24 and 36 by 3?
18
24
36
30
of 10
The product of 24 and 36 divided by their GCF is:
36
48
72
108
of 10
Which pair has a GCF of 12?
18 and 24
24 and 36
30 and 45
20 and 40
of 10
The sum of the factors of the GCF of 24 and 36 is:
18
24
28
32
of 10
Which number is not a factor of the GCF of 24 and 36?
1
2
5
6
of 10
The GCF of 24 and 36 is also a factor of which of the following?
50
60
70
80
of 10
If you add the GCF of 24 and 36 to 15, what is the result?
24
27
33
24
of 10