What is the GCF of 9 and 12?
1
2
3
4

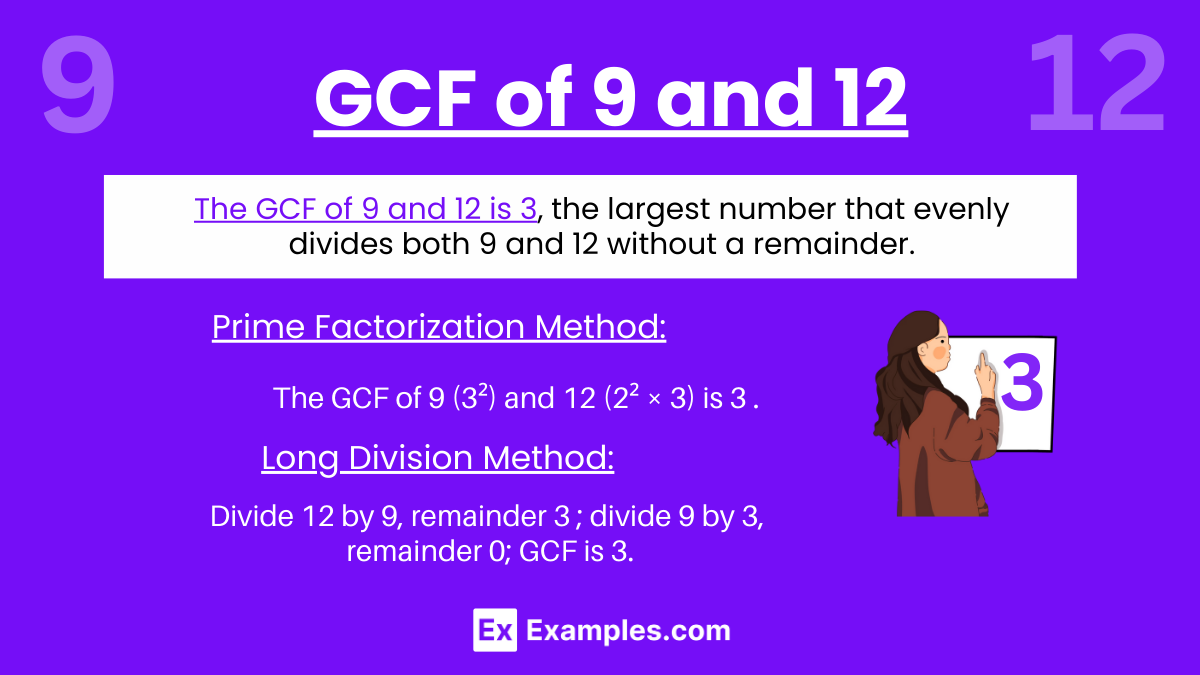
The simplest way to find the greatest common factor (GCF) of 9 and 12 is by listing their factors and identifying the largest numbers common to both lists. The factors of 9 are 1, 3, and 9, while the factors of 12 are 1, 2, 3, 4, 6, and 12. The common factors shared by both numbers are 1 and 3, with 3 being the largest. Therefore, the GCF of 9 and 12 is 3. This method is direct and effective, especially for smaller numbers, allowing for a quick and clear determination of the greatest common divisor.
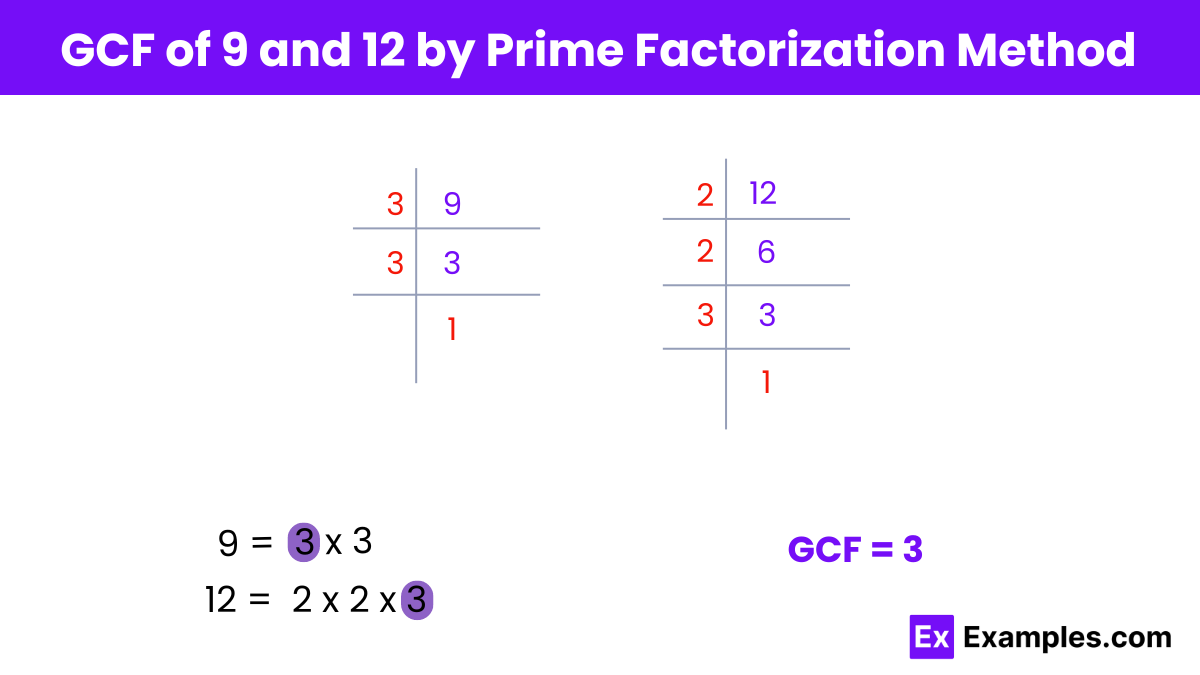
To find the greatest common factor (GCF) of 9 and 12 using the prime factorization method:
Step 1: Prime factorize both numbers:
For 9: 9 = 3²
For 12: 12 = 2² × 3
Step 2: Identify the common prime factors and their lowest powers:
The common prime factor between 9 and 12 is 3. The lowest power of 3 in both factorizations is 3¹.
Step 3: Multiply the common prime factors with their lowest powers to determine the GCF:
GCF = 3¹ = 3
Therefore, the greatest common factor (GCF) of 9 and 12 by prime factorization method is 3.
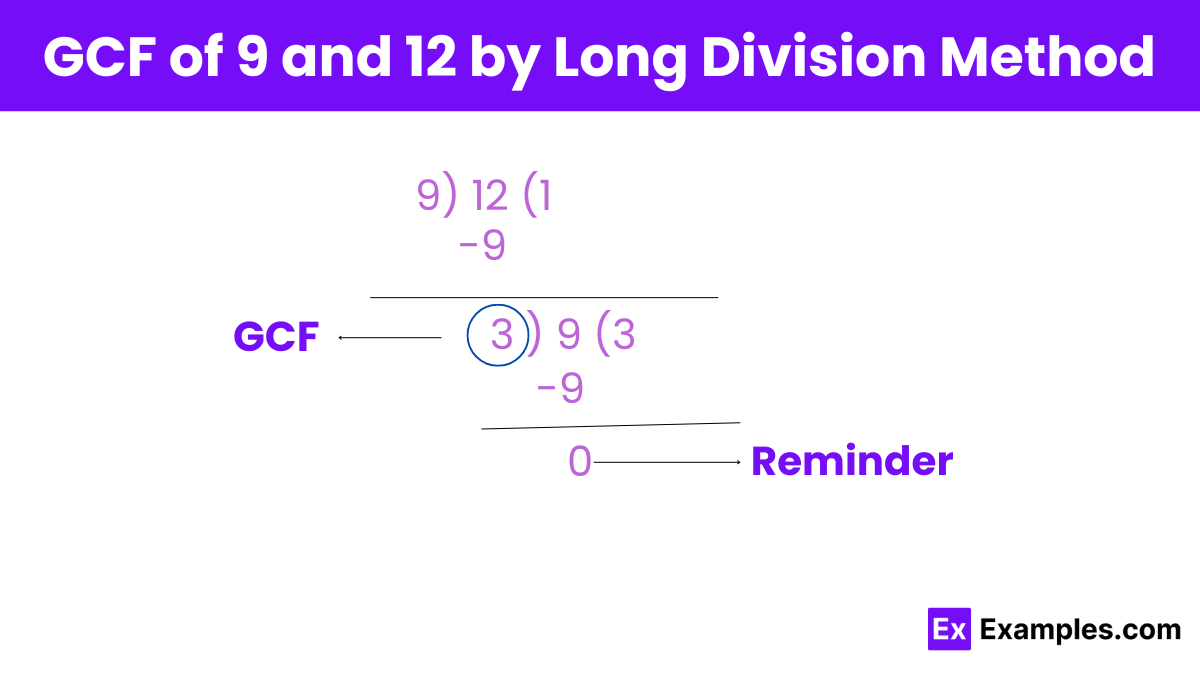
To find the greatest common factor (GCF) of 9 and 12 using the long division method, follow these steps:
Step 1: Start by dividing the larger number (12) by the smaller number (9).
12 ÷ 9 = 1 with a remainder of 3.
Step 2: Then, take the divisor (9) and divide it by the remainder (3).
9 ÷ 3 = 3 with a remainder of 0.
Since the remainder is now 0, the division process stops here.
Step 3: The divisors at this step where the remainder becomes zero is the greatest common factor (GCF).
GCF = 3.
Therefore, the greatest common factor (GCF) of 9 and 12 by the long division method is 3.

To find the greatest common factor (GCF) of 9 and 12 by listing common factors:
Step 1: List the factors of each number.
Factors of 9: 1, 3, 9
Factors of 12: 1, 2, 3, 4, 6, 12
Step 2: Identify the common factors.
Step 3: Determine the greatest common factor.
The highest number in the list of common factors is 3.
It aids in making decisions involving ratios, such as splitting ingredients or dividing tasks equally.
Because both involve finding commonalities between sets of numbers, though they serve different mathematical purposes.
This would be incorrect as 4 is not a factor of 9.
Continually subtract the smaller number from the larger until the numbers equal each other, revealing the GCF.
Listing common factors is typically the quickest method for small numbers.
They have two common factors: 1 and 3.

The simplest way to find the greatest common factor (GCF) of 9 and 12 is by listing their factors and identifying the largest numbers common to both lists. The factors of 9 are 1, 3, and 9, while the factors of 12 are 1, 2, 3, 4, 6, and 12. The common factors shared by both numbers are 1 and 3, with 3 being the largest. Therefore, the GCF of 9 and 12 is 3. This method is direct and effective, especially for smaller numbers, allowing for a quick and clear determination of the greatest common divisor.
To find the greatest common factor (GCF) of 9 and 12 using the prime factorization method:
Step 1: Prime factorize both numbers:
For 9: 9 = 3²
For 12: 12 = 2² × 3
Step 2: Identify the common prime factors and their lowest powers:
The common prime factor between 9 and 12 is 3. The lowest power of 3 in both factorizations is 3¹.
Step 3: Multiply the common prime factors with their lowest powers to determine the GCF:
GCF = 3¹ = 3
Therefore, the greatest common factor (GCF) of 9 and 12 by prime factorization method is 3.
To find the greatest common factor (GCF) of 9 and 12 using the long division method, follow these steps:
Step 1: Start by dividing the larger number (12) by the smaller number (9).
12 ÷ 9 = 1 with a remainder of 3.
Step 2: Then, take the divisor (9) and divide it by the remainder (3).
9 ÷ 3 = 3 with a remainder of 0.
Since the remainder is now 0, the division process stops here.
Step 3: The divisors at this step where the remainder becomes zero is the greatest common factor (GCF).
GCF = 3.
Therefore, the greatest common factor (GCF) of 9 and 12 by the long division method is 3.
To find the greatest common factor (GCF) of 9 and 12 by listing common factors:
Step 1: List the factors of each number.
Factors of 9: 1, 3, 9
Factors of 12: 1, 2, 3, 4, 6, 12
Step 2: Identify the common factors.
Common factors: 1, 3
Step 3: Determine the greatest common factor.
The highest number in the list of common factors is 3.
It aids in making decisions involving ratios, such as splitting ingredients or dividing tasks equally.
Because both involve finding commonalities between sets of numbers, though they serve different mathematical purposes.
This would be incorrect as 4 is not a factor of 9.
Continually subtract the smaller number from the larger until the numbers equal each other, revealing the GCF.
Listing common factors is typically the quickest method for small numbers.
They have two common factors: 1 and 3.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
What is the GCF of 9 and 12?
1
2
3
4
Which method is used to find the GCF of 9 and 12?
Subtraction method
Listing factors
Multiplication method
Prime factorization
Which of the following is a common factor of both 9 and 12?
2
4
5
3
If you divide both 9 and 12 by their GCF, what are the results?
1 and 2
3 and 6
3 and 4
9 and 12
Which number is a multiple of the GCF of 9 and 12?
5
8
9
11
If the GCF of 9 and 12 is 3, which of these numbers can also be a GCF for another pair of numbers?
6
2
4
1
The GCF of 9 and 12 is used in simplifying the fraction 9/12. What is the simplified form?
3/4
3/6
2/3
1/2
What is the product of the GCF of 9 and 12 and 2?
3
6
9
12
If you add the GCF of 9 and 12 to 5, what is the result?
7
8
9
11
How does the GCF of 9 and 12 relate to their least common multiple (LCM)?
The GCF is half of the LCM
The GCF is the LCM
The LCM is the product of the GCF and their quotient
The GCF and LCM are the same
Before you leave, take our quick quiz to enhance your learning!

