What is the square root of 144?
10
12
14
16


The square root of a number reveals the value that, when multiplied by itself, yields the original number. This concept is foundational in mathematics, particularly in algebra and number theory. Square roots can be categorized into rational numbers, which have a finite or repeating decimal expansion, and irrational numbers, which do not terminate or repeat. The process of finding square roots ties into various mathematical disciplines, including statistics through methods like the least squares method, which optimizes the fit of a statistical model. Understanding square and square roots is essential for working with integers and comprehending deeper algebraic functions.
Finding the square root of a number involves determining which number, when squared, produces the original number. This task is relatively straightforward for perfect squares, which are positive integers that can result from squaring an integer. Essentially, perfect squares are numbers that are the squares of integers.
There are four main methods to find the square root of numbers, suitable for different situations:
The Repeated Subtraction Method is a straightforward technique to find the square root of perfect square numbers. This method involves continuously subtracting consecutive odd numbers from the number in question until you reach zero. Here’s how you can apply this method step-by-step:
You subtracted numbers four times. Therefore, the square root of 16 is 4.
Calculating the square root of a number using the prime factorization method involves expressing the number as a product of prime factors and then simplifying by taking the square root. This method is particularly useful for finding the square roots of perfect squares. Here’s a step-by-step guide on how to use this method:
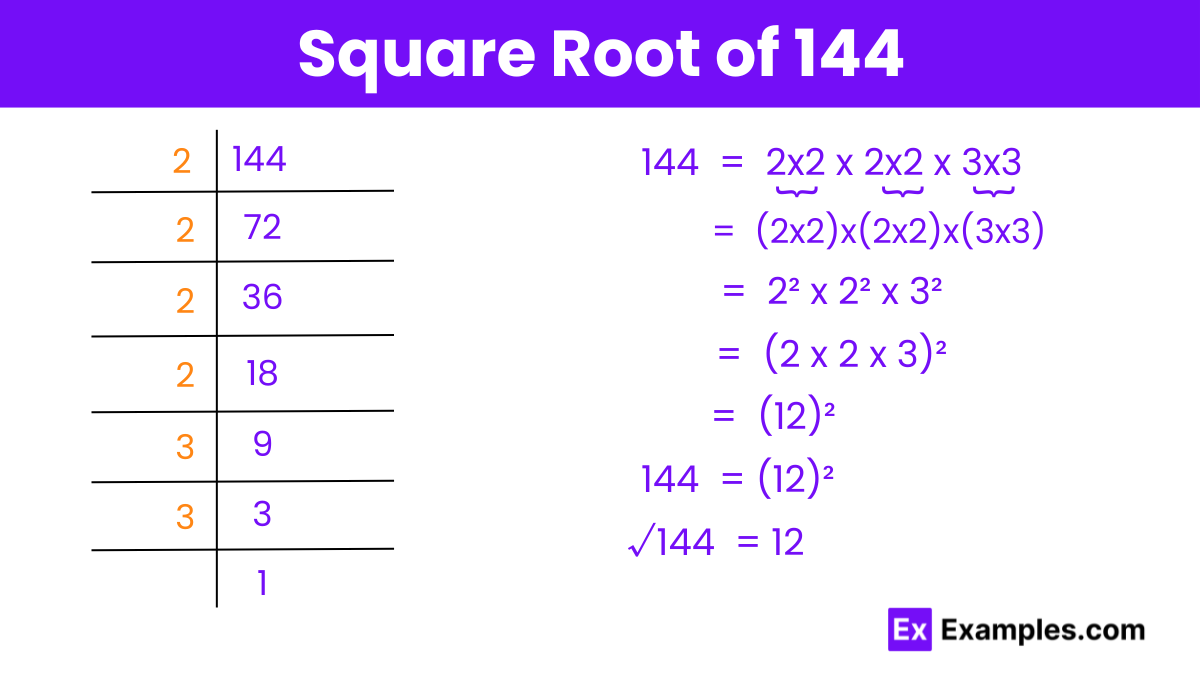
Prime Factorization:
Prime factors are: 2,2,2,2,3,3
Listing and Pairing:
Listed as 24×32.
Pair Extraction and Multiplication:
Therefore, the square root of 144 is 12. This method confirms the number is a perfect square and provides a clear, straightforward calculation of its square root.
The Estimation Method for finding square roots is a practical technique, especially when dealing with non-perfect squares or when a calculator is not available. This method involves using guesses and refining these guesses based on how close the squared guess is to the number in question. Here’s how to apply the Estimation Method step-by-step:
The Long Division Method for calculating square roots is an effective numerical procedure, especially useful for extracting square roots of large numbers or numbers that are not perfect squares. This method, also known as the digit-by-digit calculation of square roots, resembles traditional long division and provides an accurate approximation of square roots. Here’s a detailed step-by-step guide on how to perform this method:
Pair the Digits:
Start from the decimal point (if any) and move towards the left and right, grouping the digits of the number into pairs.
Find the Largest Square:
Identify the largest perfect square less than or equal to the first leftmost pair or single digit of the number.
Initial Division:
Divide the largest grouped number by the square root of the largest perfect square you identified.
Write the result as the first digit of your square root.
Subtract and Bring Down Next Pair:
Subtract the result of the multiplication from the first pair or digit and bring down the next pair of digits.
New Divisor:
Double the current result (which is the part of your ongoing calculation of the square root) and use it as the new divisor.
Find a digit which, when used both as the multiplier and the last digit of the new divisor, gives a product less than or equal to the remainder from the previous step.
Repeat the Process:
Append this digit to your existing divisor and to the result as the next digit of the square root.
Subtract and bring down the next pair of digits, and continue as before.
Continue Until Satisfied:
Continue this process until you have achieved the desired number of decimal places or until the entire number has been processed.
Pair the Digits: 12 and 25 (as 1225).
Largest Square: The largest perfect square less than 12 is 99 (3²).
Initial Division: 12÷3 = 4.
Subtract and Bring Down:
12−9=3, bring down 25 to make 325.
New Divisor: The initial 4 doubled is 8.
Find the Next Digit:
85×5 = 425 (too high), so try 84×4 = 336 (still high), then 83×3 = 249 (just right).
Repeat: The next digit is 3, so the square root so far is 35.
This process shows that the square root of 1225 is 35, as we subtract 249 from 325 and get 76 with no more digits to bring down.
| Number | Square Root |
|---|---|
| 1 | 1 |
| 2 | 1.414 |
| 3 | 1.732 |
| 4 | 2 |
| 5 | 2.236 |
| 6 | 2.449 |
| 7 | 2.646 |
| 8 | 2.828 |
| 9 | 3 |
| 10 | 3.162 |
| 11 | 3.317 |
| 12 | 3.464 |
| 13 | 3.606 |
| 14 | 3.742 |
| 15 | 3.873 |
The square root formula, expressed in terms of exponents, is:
This indicates that finding the square root of 𝑥x is equivalent to raising x to the power of 1/2. This exponentiation approach is foundational in algebra and helps in extending the concept to other types of roots, such as cube roots or fourth roots, which would similarly be expressed as 𝑥¹/³ or 𝑥¹/⁴ respectively.
Identify the Largest Square Factor:
The largest perfect square that factors into 200 is 100 (since 100 = 10²).
Factorize the Number:
Factorize 200 into 200 = 100×2.
Separate the Square Factors:
100 is a perfect square, and 2 is not.
Take Square Roots of Perfect Squares:
The square root of 100 is 10.
Rewrite the Expression:
Combine the square roots: √200= 100×2 = 10√2.
To handle square roots of negative numbers, mathematicians define the imaginary unit, denoted as i, where:
𝑖 = −1
Using the imaginary unit 𝑖, the square root of a negative number can be expressed as:
√−𝑥 = 𝑖√𝑥
where 𝑥 is a positive real number. This formula shows that the square root of a negative number involves taking the square root of the positive counterpart of that number and then multiplying the result by 𝑖.
To find the square root of −16:
√−16 = 𝑖√16 = 4𝑖
The result is 4𝑖, indicating that the square root of −16 is four times the imaginary unit 𝑖.
The introduction of imaginary numbers allows for solutions to equations that no real number can solve, expanding the scope of algebra, calculus, and other branches of mathematics. Complex numbers (comprising both real and imaginary parts, such as 𝑎+𝑏𝑖) are crucial in advanced mathematics and engineering, especially in signal processing, quantum mechanics, and electrical engineering. Imaginary and complex numbers enable a deeper understanding of phenomena that cannot be described using only real numbers.
The square of a number is a fundamental mathematical operation where a number is multiplied by itself. This operation is crucial in various branches of mathematics and its applications, ranging from geometry to algebra and beyond.
The square of a number 𝑥 is the result of multiplying 𝑥 by itself. This is mathematically expressed as:
𝑥² = 𝑥×𝑥
| Aspect | Squares (𝑥²) | Square Roots (√x) |
|---|---|---|
| Definition | The square of a number 𝑥x is 𝑥x multiplied by itself: 𝑥×𝑥. | The square root of a number is a value 𝑦 such that 𝑦×𝑦 = 𝑥. |
| Result | Always non-negative, as squaring any real number yields a positive number or zero. | Can be real (non-negative only for real numbers) or imaginary (if 𝑥 is negative under the real number system). |
| Mathematical Notation | 𝑥² | √𝑥 or 𝑥¹/² |
| Purpose | Used to calculate areas, powers, and in formulas for physical laws. | Used to extract original dimensions, like side length from area, and in solving quadratic equations. |
| Graph Shape | Parabola opening upwards for 𝑦 = 𝑥². | Two branches, one positive and one negative, for 𝑦 = √𝑥 (only the non-negative branch for real numbers). |
| Complex Numbers | Squaring a real number never results in a complex number. | Extracting square roots from negative numbers introduces imaginary numbers (√−1 = 𝑖). |
| Operation Type | Multiplicative operation: multiplying a number by itself. | Inverse operation: finding the number whose square gives the original number. |
Problem: Find the square root of 144.
Steps:
Factorize the Number:
Begin by factorizing 144 into its prime factors.
144 = 2×2×2×2×3×3.
Pair the Factors:
Group the factors into pairs of identical numbers.
Pairs: (2×2),(2×2),(3×3).
Take One from Each Pair:
Take one number from each pair.
You have two pairs of 2s and one pair of 3s, so take two 2s and one 3.
Multiply the Selected Numbers:
Multiply the numbers you’ve taken from each pair.
2×2×3 = 12.
Result:
The square root of 144 is 12.
Conclusion:
Problem: Find the square root of 1225.
Steps:
Pair the Digits from Right to Left:
1225 can be divided into pairs: 12 and 25.
Initial Guess:
Find the largest number whose square is less than or equal to 12. That number is 3, because 3² = 9.
Subtract and Bring Down:
Subtract 9 from 12, which gives 3. Bring down the next pair, making it 325.
Double the First Digit of the Result:
The first digit of the result is 3. Doubling it gives 6. Place it next to a blank space (6_).
Find the Next Digit:
Find the largest digit X that fits the formula 60𝑋+𝑋²≤325. X is 5, because 605+5² = 605+25 = 630 (too high), hence, adjust to 4.
Complete the Operation:
Place 4 next to 6, making it 64.
604+4² = 604+16 = 620, which is less than 325.
Subtract 620 from 325, leaving a remainder.
Refine as Necessary:
The next number would be added as decimals, but for the integer square root, we stop here.
Conclusion:
√1225 = 35.
To find the square root using prime factorization, break the number down into its prime factors, pair identical factors, and then take one factor from each pair. Multiply these factors together to get the square root.
The long division method is used to find the square root of both perfect and non-perfect squares. It is particularly useful for large numbers and provides a precise decimal result if necessary.
Every non-negative real number has a real number square root, either positive or zero. Negative numbers have imaginary square roots, denoted using the imaginary unit 𝑖.
Square roots are used in various fields including physics (calculating speeds, energies), engineering (stress analysis, resonant frequencies), finance (compound interest calculations), and statistics (standard deviation).
To simplify a square root, identify and factor out the largest square factor, take the square root of that factor, and then multiply it by the square root of the remaining number.
The square of a number is the result of multiplying the number by itself, while the square is the operation that reverses squaring, returning the original number that was squared to produce a given number.
Yes, the long division method is especially useful for calculating the square roots of non-perfect squares, providing a way to get an approximate decimal result.
The estimation method can provide a reasonably accurate approximation, especially with successive refinements. However, its accuracy depends on the initial guess and the number of refinement steps performed.

The square root of a number reveals the value that, when multiplied by itself, yields the original number. This concept is foundational in mathematics, particularly in algebra and number theory. Square roots can be categorized into rational numbers, which have a finite or repeating decimal expansion, and irrational numbers, which do not terminate or repeat. The process of finding square roots ties into various mathematical disciplines, including statistics through methods like the least squares method, which optimizes the fit of a statistical model. Understanding square and square roots is essential for working with integers and comprehending deeper algebraic functions.
The square root of a number is a value that, when squared, results in the original number. For example, the square root of 9 is 3 because 3² = 9. This mathematical operation is crucial in various fields such as geometry, where it is used to determine the length of the sides of squares, and in algebra for solving quadratic equations. Understanding square roots also helps in higher math and applications like statistics and physics.
The square root of a number is a value that, when multiplied by itself, produces the original number. It is a fundamental operation in algebra that helps in solving equations and understanding geometric and statistical concepts.
Finding the square root of a number involves determining which number, when squared, produces the original number. This task is relatively straightforward for perfect squares, which are positive integers that can result from squaring an integer. Essentially, perfect squares are numbers that are the squares of integers.
There are four main methods to find the square root of numbers, suitable for different situations:
Repeated Subtraction Method: This involves subtracting successive odd numbers from the number until you reach zero. The number of times you subtract is the square root.
Prime Factorization Method: Factorize the number into its prime factors and pair identical factors. The product of one factor from each pair gives the square root.
Estimation Method: Make an educated guess based on known squares close to the number, then refine your guess based on how close the square of your estimate is to the original number.
Long Division Method: This method is more complex and can be used to find the square root of any number, including non-perfect squares. It involves a process similar to long division.
The Repeated Subtraction Method is a straightforward technique to find the square root of perfect square numbers. This method involves continuously subtracting consecutive odd numbers from the number in question until you reach zero. Here’s how you can apply this method step-by-step:
Start with the Number: Choose the number from which you want to find the square root. Ensure it’s a perfect square to use this method effectively.
Subtract Consecutive Odd Numbers: Begin by subtracting the first odd number (1) from your chosen number, then continue with the next odd number (3), the next after that (5), and so on. Keep a count of how many numbers you subtract.
Continue Until Zero: Keep subtracting consecutive odd numbers until the result is zero. The process must always end at zero for perfect squares.
Count the Subtractions: The number of times you subtracted the odd numbers is the square root of the original number.
Start with 16.
Subtract 1 (16 – 1 = 15)
Subtract 3 (15 – 3 = 12)
Subtract 5 (12 – 5 = 7)
Subtract 7 (7 – 7 = 0)
You subtracted numbers four times. Therefore, the square root of 16 is 4.
Calculating the square root of a number using the prime factorization method involves expressing the number as a product of prime factors and then simplifying by taking the square root. This method is particularly useful for finding the square roots of perfect squares. Here’s a step-by-step guide on how to use this method:
Factorize the Number into Primes: Begin by factorizing the given number into its prime factors. This means breaking the number down repeatedly by dividing it by prime numbers (like 2, 3, 5, 7, etc.) until all the factors are prime.
List the Prime Factors: Write down the list of prime factors. For example, the prime factors of 72 are 2,2,2,3,3.
Pair the Prime Factors: Arrange the prime factors in pairs of identical factors. If a prime factor does not have a pair, it means the number is not a perfect square and its square root will be irrational.
Take One from Each Pair: For each pair of identical factors, take one factor out. For example, from the pairs 2,2 and 3,3 take one ‘2’ and one ‘3’.
Multiply the Extracted Factors: Multiply the factors taken from each pair to get the square root of the original number. Using the example above, 2×3 = 6, so the square root of 72 is 6.
Prime Factorization:
144 ÷ 2 = 72
72 ÷ 2 = 36
36 ÷ 2 = 18
18 ÷ 2 = 9
9 ÷ 3 = 3
3 ÷ 3 = 1
Prime factors are: 2,2,2,2,3,3
Listing and Pairing:
Listed as 24×32.
Pair Extraction and Multiplication:
Pairs: 2,2 and 3,3.
Extract one 2 and one 3: 2×2×3 = 12.
Therefore, the square root of 144 is 12. This method confirms the number is a perfect square and provides a clear, straightforward calculation of its square root.
The Estimation Method for finding square roots is a practical technique, especially when dealing with non-perfect squares or when a calculator is not available. This method involves using guesses and refining these guesses based on how close the squared guess is to the number in question. Here’s how to apply the Estimation Method step-by-step:
Identify Close Squares: Begin by identifying perfect squares that are close to your target number. For example, if you need the square root of 50, recognize that 49 (square of 7) and 64 (square of 8) are nearby perfect squares.
Initial Guess: Choose an initial guess for the square root. This guess should be a number between the square roots of the perfect squares identified. In our example, since 7² = 49 and 8² = 64, an initial guess could be 7.5.
Refine Your Guess: Square your initial guess and compare the result to your target number. If the squared guess is too high, decrease your guess; if it’s too low, increase your guess.
Use Averages for Closer Approximation: To refine your guess further, use the average of your guess and the result of dividing your target number by your guess. This average will be a new, more accurate guess. New Guess = Guess+Target Number/Guess/2.
Repeat as Needed: Repeat the process of refining your guess until you reach a satisfactory level of accuracy. Each iteration should bring you closer to the actual square root.
Close Squares: 49 and 64, with roots 7 and 8.
Initial Guess: Start with 7.5.
Square 7.5: 7.5×7.5 = 56.25.
Refine: 56.25 is close to 50, but a bit high, so try lowering the guess.
Use Averages: New guess = 7.5+50/7.52 = 7.5+6.67/2=7.08.
Square 7.08 and adjust as necessary.
Flexibility: Useful for both perfect and non-perfect squares.
No Tools Needed: Does not require a calculator, making it ideal for mental math or when tools are not available.
The Long Division Method for calculating square roots is an effective numerical procedure, especially useful for extracting square roots of large numbers or numbers that are not perfect squares. This method, also known as the digit-by-digit calculation of square roots, resembles traditional long division and provides an accurate approximation of square roots. Here’s a detailed step-by-step guide on how to perform this method:
Pair the Digits:
Start from the decimal point (if any) and move towards the left and right, grouping the digits of the number into pairs.
Find the Largest Square:
Identify the largest perfect square less than or equal to the first leftmost pair or single digit of the number.
Initial Division:
Divide the largest grouped number by the square root of the largest perfect square you identified.
Write the result as the first digit of your square root.
Subtract and Bring Down Next Pair:
Subtract the result of the multiplication from the first pair or digit and bring down the next pair of digits.
New Divisor:
Double the current result (which is the part of your ongoing calculation of the square root) and use it as the new divisor.
Find a digit which, when used both as the multiplier and the last digit of the new divisor, gives a product less than or equal to the remainder from the previous step.
Repeat the Process:
Append this digit to your existing divisor and to the result as the next digit of the square root.
Subtract and bring down the next pair of digits, and continue as before.
Continue Until Satisfied:
Continue this process until you have achieved the desired number of decimal places or until the entire number has been processed.
Pair the Digits: 12 and 25 (as 1225).
Largest Square: The largest perfect square less than 12 is 99 (3²).
Initial Division: 12÷3 = 4.
Subtract and Bring Down:
12−9=3, bring down 25 to make 325.
New Divisor: The initial 4 doubled is 8.
Find the Next Digit:
85×5 = 425 (too high), so try 84×4 = 336 (still high), then 83×3 = 249 (just right).
Repeat: The next digit is 3, so the square root so far is 35.
This process shows that the square root of 1225 is 35, as we subtract 249 from 325 and get 76 with no more digits to bring down.
Number | Square Root |
|---|---|
1 | 1 |
2 | 1.414 |
3 | 1.732 |
4 | 2 |
5 | 2.236 |
6 | 2.449 |
7 | 2.646 |
8 | 2.828 |
9 | 3 |
10 | 3.162 |
11 | 3.317 |
12 | 3.464 |
13 | 3.606 |
14 | 3.742 |
15 | 3.873 |
The square root formula, expressed in terms of exponents, is:
√x = x¹/²
This indicates that finding the square root of 𝑥x is equivalent to raising x to the power of 1/2. This exponentiation approach is foundational in algebra and helps in extending the concept to other types of roots, such as cube roots or fourth roots, which would similarly be expressed as 𝑥¹/³ or 𝑥¹/⁴ respectively.
Identify the Largest Square Factor:
Look for the largest perfect square that is a factor of the number under the square root.
Factorize the Number:
Break down the number inside the square root into its prime factors, or any factors where one is a perfect square.
Separate the Square Factors:
Separate the factors into those that are perfect squares and those that are not.
Take Square Roots of Perfect Squares:
Take the square root of the perfect square factors. Since they are perfect squares, their square roots will be whole numbers.
Rewrite the Expression:
Multiply the whole numbers obtained from the square roots of the perfect squares by the square root of the remaining factors.
Identify the Largest Square Factor:
The largest perfect square that factors into 200 is 100 (since 100 = 10²).
Factorize the Number:
Factorize 200 into 200 = 100×2.
Separate the Square Factors:
100 is a perfect square, and 2 is not.
Take Square Roots of Perfect Squares:
The square root of 100 is 10.
Rewrite the Expression:
Combine the square roots: √200= 100×2 = 10√2.
To handle square roots of negative numbers, mathematicians define the imaginary unit, denoted as i, where:
𝑖 = −1
Using the imaginary unit 𝑖, the square root of a negative number can be expressed as:
√−𝑥 = 𝑖√𝑥
where 𝑥 is a positive real number. This formula shows that the square root of a negative number involves taking the square root of the positive counterpart of that number and then multiplying the result by 𝑖.
To find the square root of −16:
Recognize that 𝑥 = 16 in this scenario.
Compute the square root of the positive part, which is √16 = 4.
Apply the formula for the square root of a negative number:
√−16 = 𝑖√16 = 4𝑖
The result is 4𝑖, indicating that the square root of −16 is four times the imaginary unit 𝑖.
The introduction of imaginary numbers allows for solutions to equations that no real number can solve, expanding the scope of algebra, calculus, and other branches of mathematics. Complex numbers (comprising both real and imaginary parts, such as 𝑎+𝑏𝑖) are crucial in advanced mathematics and engineering, especially in signal processing, quantum mechanics, and electrical engineering. Imaginary and complex numbers enable a deeper understanding of phenomena that cannot be described using only real numbers.
The square of a number is a fundamental mathematical operation where a number is multiplied by itself. This operation is crucial in various branches of mathematics and its applications, ranging from geometry to algebra and beyond.
The square of a number 𝑥 is the result of multiplying 𝑥 by itself. This is mathematically expressed as:
𝑥² = 𝑥×𝑥
Non-negativity: The square of any real number, whether positive or negative, is always non-negative. For example, (−5)² = 25 and 5² =25.
Identity Element: The square of zero is zero. 0² = 0.
Increasing Function: For any two non-negative numbers 𝑎a and 𝑏b such that 𝑎<𝑏, then 𝑎²<𝑏². This shows that squaring is an increasing function in the non-negative domain.
Symmetry: The square of −𝑥 is the same as the square of 𝑥, i.e., (−𝑥)² = 𝑥². This property reflects the symmetrical nature of the function 𝑓(𝑥) = 𝑥² on the coordinate plane.
Square of 3: 3² = 3×3 = 9
Square of -4: (−4)² = (−4)×(−4) = 16
Square of 10: 10² = 10×10 = 100
Geometry: Calculating area of squares. The area of a square with side length 𝑠 is 𝑠².
Algebra: Solving quadratic equations. The solutions often involve squaring terms.
Statistics: Computing variance and standard deviation. Variance is the average of the squared differences from the Mean.
Physics: Many physical laws involve squaring, such as the law of gravitational force and formulas for kinetic energy (𝐾𝐸 = 12𝑚𝑣²).
Aspect | Squares (𝑥²) | Square Roots (√x) |
|---|---|---|
Definition | The square of a number 𝑥x is 𝑥x multiplied by itself: 𝑥×𝑥. | The square root of a number is a value 𝑦 such that 𝑦×𝑦 = 𝑥. |
Result | Always non-negative, as squaring any real number yields a positive number or zero. | Can be real (non-negative only for real numbers) or imaginary (if 𝑥 is negative under the real number system). |
Mathematical Notation | 𝑥² | √𝑥 or 𝑥¹/² |
Purpose | Used to calculate areas, powers, and in formulas for physical laws. | Used to extract original dimensions, like side length from area, and in solving quadratic equations. |
Graph Shape | Parabola opening upwards for 𝑦 = 𝑥². | Two branches, one positive and one negative, for 𝑦 = √𝑥 (only the non-negative branch for real numbers). |
Complex Numbers | Squaring a real number never results in a complex number. | Extracting square roots from negative numbers introduces imaginary numbers (√−1 = 𝑖). |
Operation Type | Multiplicative operation: multiplying a number by itself. | Inverse operation: finding the number whose square gives the original number. |
Square Root of 22 | Square Root of 23 | |||
Square Root of 31 | Square Root of 33 | |||
Square Root of 38 | Square Root of 39 | |||
Square Root of 43 | ||||
Square Root of 46 | Square Root of 47 | |||
Square Root of 51 | Square Root of 53 | Square Root of 54 | Square Root of 55 | |
Square Root of 57 | Square Root of 58 | Square Root of 59 | ||
Square Root of 62 | Square Root of 63 | Square Root of 65 | ||
Square Root of 66 | Square Root of 67 | Square Root of 68 | ||
Square Root of 71 | Square Root of 73 | Square Root of 74 | ||
Square Root of 76 | Square Root of 77 | Square Root of 78 | Square Root of 79 | |
Square Root of 82 | Square Root of 83 | Square Root of 84 | ||
Square Root of 86 | Square Root of 87 | Square Root of 88 | Square Root of 89 | |
Square Root of 91 | Square Root of 92 | Square Root of 93 | Square Root of 94 | Square Root of 95 |
Square Root of 97 |
Problem: Find the square root of 144.
Steps:
Factorize the Number:
Begin by factorizing 144 into its prime factors.
144 = 2×2×2×2×3×3.
Pair the Factors:
Group the factors into pairs of identical numbers.
Pairs: (2×2),(2×2),(3×3).
Take One from Each Pair:
Take one number from each pair.
You have two pairs of 2s and one pair of 3s, so take two 2s and one 3.
Multiply the Selected Numbers:
Multiply the numbers you’ve taken from each pair.
2×2×3 = 12.
Result:
The square root of 144 is 12.
Conclusion:
√144 = 12.
Problem: Find the square root of 1225.
Steps:
Pair the Digits from Right to Left:
1225 can be divided into pairs: 12 and 25.
Initial Guess:
Find the largest number whose square is less than or equal to 12. That number is 3, because 3² = 9.
Subtract and Bring Down:
Subtract 9 from 12, which gives 3. Bring down the next pair, making it 325.
Double the First Digit of the Result:
The first digit of the result is 3. Doubling it gives 6. Place it next to a blank space (6_).
Find the Next Digit:
Find the largest digit X that fits the formula 60𝑋+𝑋²≤325. X is 5, because 605+5² = 605+25 = 630 (too high), hence, adjust to 4.
Complete the Operation:
Place 4 next to 6, making it 64.
604+4² = 604+16 = 620, which is less than 325.
Subtract 620 from 325, leaving a remainder.
Refine as Necessary:
The next number would be added as decimals, but for the integer square root, we stop here.
Conclusion:
√1225 = 35.
To find the square root using prime factorization, break the number down into its prime factors, pair identical factors, and then take one factor from each pair. Multiply these factors together to get the square root.
The long division method is used to find the square root of both perfect and non-perfect squares. It is particularly useful for large numbers and provides a precise decimal result if necessary.
Every non-negative real number has a real number square root, either positive or zero. Negative numbers have imaginary square roots, denoted using the imaginary unit 𝑖.
Square roots are used in various fields including physics (calculating speeds, energies), engineering (stress analysis, resonant frequencies), finance (compound interest calculations), and statistics (standard deviation).
To simplify a square root, identify and factor out the largest square factor, take the square root of that factor, and then multiply it by the square root of the remaining number.
The square of a number is the result of multiplying the number by itself, while the square is the operation that reverses squaring, returning the original number that was squared to produce a given number.
Yes, the long division method is especially useful for calculating the square roots of non-perfect squares, providing a way to get an approximate decimal result.
The estimation method can provide a reasonably accurate approximation, especially with successive refinements. However, its accuracy depends on the initial guess and the number of refinement steps performed.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
What is the square root of 144?
10
12
14
16
Find the square root of 81.
7
8
9
10
Determine the square root of 64.
6
7
8
9
What is the square root of 225?
14
15
16
17
Find the square root of 81.
8
10
9
11
Which number has a square root of 7?
51
50
49
48
Find the square root of 324.
17
18
19
20
22
23
24
25
Find the square root of 1444.
33
34
35
36
What is the square root of 676?
24
25
26
27
Before you leave, take our quick quiz to enhance your learning!

