Integration of Tan Square x

Integration of tan⁡²(x) involves techniques that encompass a broad array of mathematical concepts. From exploring rational and irrational numbers to delving into the realms of algebra and statistics, the process links various foundational elements like integers and square and square roots. Employing methods such as the least squares method in data fitting, this topic extends beyond simple calculus, bridging the gap between numerical theory and real-world applications. Such integrations often challenge our understanding of numbers and their properties, underscoring the interconnected nature of mathematical disciplines.

What is Integration of Tan Square x?

The integration of $(x)$ can be computed by using a trigonometric identity and substitution. First, recall the identity tan⁡⁡²(𝑥) = sec⁡⁡²(𝑥)−1. Thus, the integral of $(x)$ becomes ∫tan⁡⁡²(𝑥) 𝑑𝑥 = ∫(sec⁡⁡²(𝑥)−1) 𝑑𝑥, which simplifies to tan⁡(𝑥)−𝑥+𝐶, where 𝐶 is the constant of integration.

Integration of Tan Square x Formula

∫ tan⁡²x dx = tan x – x + C

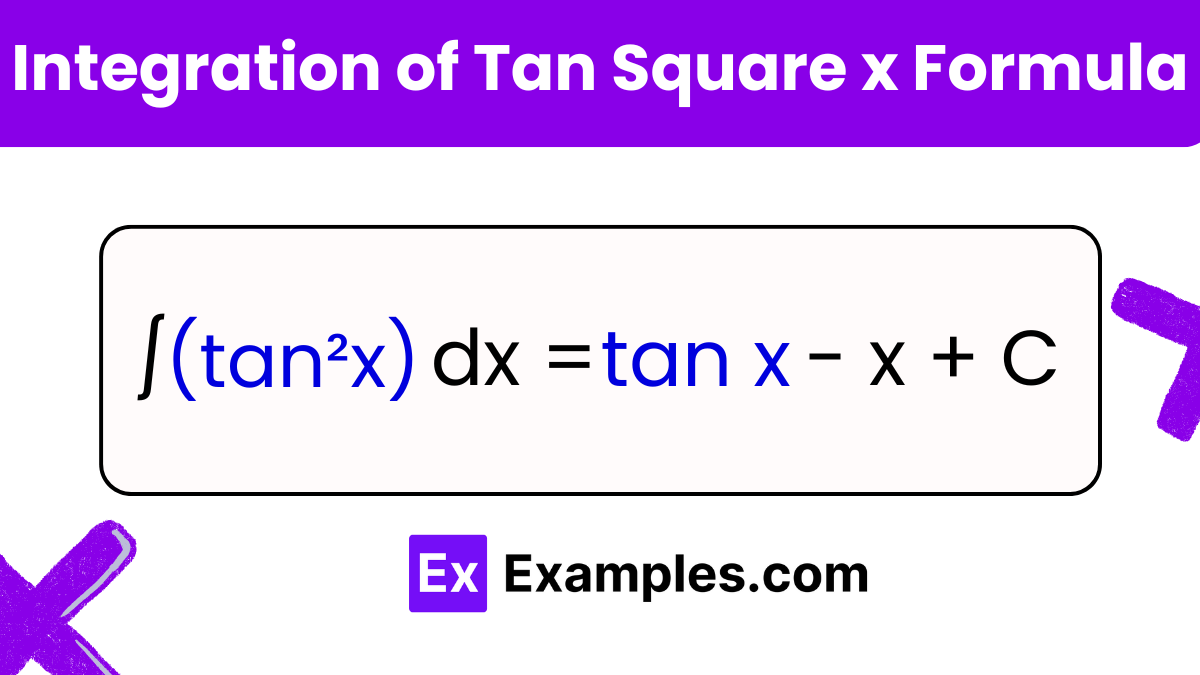
The integral ∫tan⁡⁡²(𝑥) 𝑑𝑥 equals tan⁡(𝑥)−𝑥+𝐶 by utilizing the trigonometric identity tan⁡²(𝑥) = sec⁡²(𝑥)−1. This transformation allows the integration of sec⁡⁡²(x) and −1 separately, simplifying to tan⁡(𝑥)−𝑥, with 𝐶 as the integration constant representing any constant value added to the indefinite integral.

Integration of Tan Square x Proof

To provide a proof for the integral ∫tan⁡²(𝑥) 𝑑𝑥, we start by utilizing a known trigonometric identity and proceed with basic integration techniques. Here’s a detailed step-by-step explanation:

Step 1: Use the Trigonometric Identity

First, we employ the identity for tan⁡²(x):

tan⁡²(𝑥) = sec²(𝑥)−1

Step 2: Express the Integral Using the Identity

Using the identity, we rewrite the integral:

∫tan⁡²(𝑥) 𝑑𝑥 = ∫(sec⁡²(𝑥)−1) 𝑑𝑥

Step 3: Separate the Integral

The integral can now be separated into two simpler integrals:

∫tan⁡²(𝑥) 𝑑𝑥 = ∫sec⁡²(𝑥) 𝑑𝑥−∫1 𝑑𝑥

Step 4: Integrate Each Term

The integral of sec⁡²(x) is straightforward:

∫sec⁡²(𝑥) 𝑑𝑥 = tan⁡(𝑥)

The integral of 1 with respect to 𝑥 is simply 𝑥:

∫1 𝑑𝑥 = 𝑥

Step 5: Combine the Results

Subtracting the integral of 1 from the integral of sec⁡²(x) gives:

∫tan⁡²(𝑥) 𝑑𝑥 = tan⁡(𝑥)−𝑥+𝐶

Where 𝐶 is the constant of integration.

Integration of Tan Square x From 0 to Pi by 4

To find the definite integral of tan⁡⁡²(x) from 0 to 𝜋/4​, we can use the previously established integral formula for tan⁡²(x), which is ∫tan⁡²(𝑥) 𝑑𝑥 = tan⁡(𝑥)−𝑥. Applying the limits, we evaluate:

Integral Setup

∫tan⁡²(𝑥) 𝑑𝑥𝜋/⁴₀ = [tan⁡(𝑥)−𝑥]𝜋/⁴₀​​

Evaluate at the Upper Limit 𝜋/4​

At 𝑥 = 𝜋/4, tan⁡(𝜋/4)=1, so:

tan⁡(𝜋/4)−𝜋/4 = 1−𝜋/4​

Evaluate at the Lower Limit 0

At 𝑥 = 0, tan⁡(0) = 0, so:

tan⁡(0)−0 = 0

Compute the Integral

Subtract the value at the lower limit from the value at the upper limit:

[1−𝜋/4]−[0] = 1−𝜋/4​

Integration of Tan Square x Examples

Example 1: Integration from 𝜋/6 to 𝜋/3

Problem: Compute the integral of tan⁡⁡²(x) from 𝜋/6 to 𝜋/3.

Solution: Using the identity tan⁡⁡⁡²(𝑥) = sec⁡⁡⁡²(𝑥)−1 and the integral formula:

∫tan⁡⁡⁡²(𝑥) 𝑑𝑥 = tan⁡(𝑥)−𝑥

Evaluating from 𝜋/6 to 𝜋/3:

[tan⁡(𝑥)−𝑥]^𝜋/³𝜋/₆ = (tan⁡(𝜋/3)−𝜋/3)−(tan⁡(𝜋/6)−𝜋/6)

Given tan⁡(𝜋/3) = 3​ and tan⁡(𝜋/6) = 1/3, the calculation becomes:

(3−𝜋/3)−(1/3−𝜋/6)

Result: The integral evaluates to a specific numerical value, simplifying the expression by combining terms.

Example 2: Integration over a Full Period of tan⁡⁡²(x)

Problem: Compute the integral of tan⁡⁡²(x) over one full period, from −𝜋/4 to 3𝜋/4.

Solution: Again using the integral formula:

∫tan⁡⁡⁡²(𝑥) 𝑑𝑥 = tan⁡(𝑥)−𝑥

Evaluating from −𝜋/4 = to 3𝜋/4:

[tan⁡(𝑥)−𝑥]³𝜋/⁴−𝜋/₄ = (tan⁡(3𝜋/4)−3𝜋/4)−(tan⁡(−𝜋/4)−(−𝜋/4))

Since tan⁡(3𝜋/4) = −1 and tan⁡(−𝜋/4)= −1, the calculation becomes:

(−1−3𝜋/4)−(−1+𝜋/4)=−𝜋

Result: The result demonstrates the behavior of the tan⁡²(x) function over its periodic interval.

Example 3: Evaluating Zero to 𝜋

Problem: Evaluate ∫𝜋₀tan⁡⁡²(𝑥) 𝑑𝑥.

Solution: Direct computation is challenging due to the undefined nature of tan⁡(𝑥) at 𝜋/2. This integral technically diverges as tan⁡⁡²(x) approaches infinity near 𝑥 = 𝜋/2.

Result: The integral does not converge due to the asymptotic behavior at 𝜋/2.

FAQs

What is the significance of integrating tan⁡⁡⁡²(x) in practical applications?

Integrating tan⁡⁡²(x) is often encountered in physics and engineering, particularly in problems involving angular motion and oscillations where tangent functions model behaviors varying over time.

How does the periodicity of tan⁡(𝑥) affect its square’s integration?

The periodic nature of tan⁡(𝑥) implies that integrals of tan⁡⁡⁡²(x) over its full periods or multiples thereof simplify the evaluation, but care must be taken to avoid points of discontinuity where the tangent function is undefined.

How does the trigonometric identity help in integrating tan⁡²(x)?

By substituting tan⁡²(x) with sec⁡²(𝑥)−1, the integral breaks into simpler terms, sec²(x) and 1, which are straightforward to integrate individually.