Multiples of 3
Multiples of 3
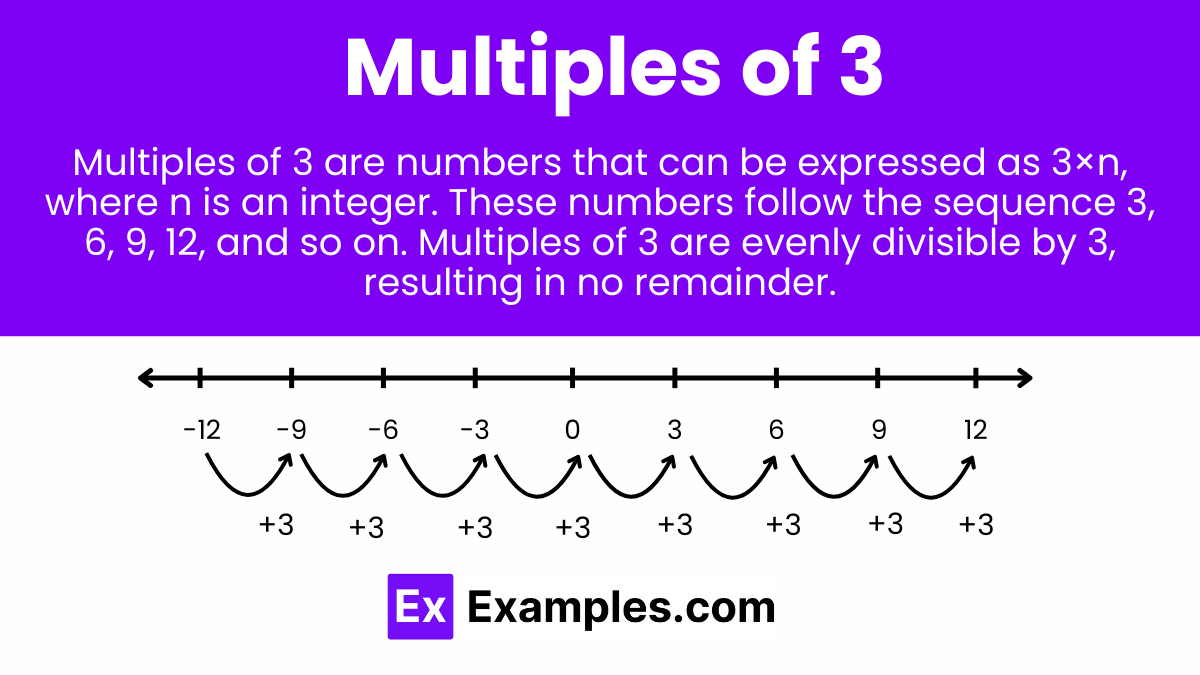
Multiples of 3 are integers that can be obtained by multiplying the number 3 by any whole number. These numbers, such as 3, 6, 9, 12, and so on, are the result of the multiplication process involving 3. The concept of multiples is closely related to factors and divisors; specifically, a multiple of 3 is any number that 3 can evenly divide without leaving a remainder. Understanding multiples helps in various mathematical applications, including finding common multiples and solving problems involving divisibility.
What are Multiples of 3?
Multiples of 3 are numbers that result from multiplying 3 by any integer. They are the sequence 3, 6, 9, 12, 15, and so on, where each number is a product of 3 and another whole number. These numbers are divisible by 3 without leaving a remainder.
For example, 70, 80, 20 and 60 are all multiples of 3, 22 is not a multiple of 3 for the following reasons:
| Number | Calculation | Remainder |
|---|---|---|
| 15 | 15 ÷ 3 = 5 | 0 |
| 27 | 27 ÷ 3 = 9 | 0 |
| 33 | 33 ÷ 3 = 11 | 0 |
| 60 | 60 ÷ 3 = 20 | 0 |
| 22 | 22 ÷ 3 = 7.33 | 1 |
List of First 100 Multiples of 3 with Remainders
| Number | Calculation | Remainder |
|---|---|---|
| 3 | 3÷3 = 1, so 3 is divisible by 3. | 0 |
| 6 | 6÷3 = 2, so 6 is divisible by 3. | 0 |
| 9 | 9÷3 = 3, so 9 is divisible by 3. | 0 |
| 12 | 12÷3 = 4, so 12 is divisible by 3. | 0 |
| 15 | 15÷3 = 5, so 15 is divisible by 3. | 0 |
| 18 | 18÷3 = 6, so 18 is divisible by 3. | 0 |
| 21 | 21÷3 = 7, so 21 is divisible by 3. | 0 |
| 24 | 24÷3 = 8, so 24 is divisible by 3. | 0 |
| 27 | 27÷3 = 9, so 27 is divisible by 3. | 0 |
| 30 | 30÷3 = 10, so 30 is divisible by 3. | 0 |
| 33 | 33÷3 = 11, so 33 is divisible by 3. | 0 |
| 36 | 36÷3 = 12, so 36 is divisible by 3. | 0 |
| 39 | 39÷3 = 13, so 39 is divisible by 3. | 0 |
| 42 | 42÷3 = 14, so 42 is divisible by 3. | 0 |
| 45 | 45÷3 = 15, so 45 is divisible by 3. | 0 |
| 48 | 48÷3 =16, so 48 is divisible by 3. | 0 |
| 51 | 51÷3 = 17, so 51 is divisible by 3. | 0 |
| 54 | 54÷3 = 18, so 54 is divisible by 3. | 0 |
| 57 | 57÷3 = 19, so 57 is divisible by 3. | 0 |
| 60 | 60÷3 = 20, so 60 is divisible by 3. | 0 |
| 63 | 63÷3 = 21, so 63 is divisible by 3. | 0 |
| 66 | 66÷3 = 22, so 66 is divisible by 3. | 0 |
| 69 | 69÷3 = 23, so 69 is divisible by 3. | 0 |
| 72 | 72÷3 = 24, so 72 is divisible by 3. | 0 |
| 75 | 75÷3 = 25, so 75 is divisible by 3. | 0 |
| 78 | 78÷3=26, so 78 is divisible by 3. | 0 |
| 81 | 81÷3 = 27, so 81 is divisible by 3. | 0 |
| 84 | 84÷3 = 28, so 84 is divisible by 3. | 0 |
| 87 | 87÷3 = 29, so 87 is divisible by 3. | 0 |
| 90 | 90÷3 = 30, so 90 is divisible by 3. | 0 |
| 93 | 93÷3 = 31, so 93 is divisible by 3. | 0 |
| 96 | 96÷3 = 32, so 96 is divisible by 3. | 0 |
| 99 | 99÷3 = 33, so 99 is divisible by 3. | 0 |
| 102 | 102÷3 = 34, so 102 is divisible by 3. | 0 |
| 105 | 105÷3 = 35, so 105 is divisible by 3. | 0 |
| 108 | 108÷3 = 36, so 108 is divisible by 3. | 0 |
| 111 | 111÷3 = 37, so 111 is divisible by 3. | 0 |
| 114 | 114÷3 = 38, so 114 is divisible by 3. | 0 |
| 117 | 117÷3 = 39, so 117 is divisible by 3. | 0 |
| 120 | 120÷3 = 40, so 120 is divisible by 3. | 0 |
| 123 | 123÷3 = 41, so 123 is divisible by 3. | 0 |
| 126 | 126÷3 = 42, so 126 is divisible by 3. | 0 |
| 129 | 129÷3 = 43, so 129 is divisible by 3. | 0 |
| 132 | 132÷3 = 44, so 132 is divisible by 3. | 0 |
| 135 | 135÷3 = 45, so 135 is divisible by 3. | 0 |
| 138 | 138÷3 = 46, so 138 is divisible by 3. | 0 |
| 141 | 141÷3 = 47, so 141 is divisible by 3. | 0 |
| 144 | 144÷3 = 48, so 144 is divisible by 3. | 0 |
| 147 | 147÷3 = 49, so 147 is divisible by 3. | 0 |
| 150 | 150÷3 = 50, so 150 is divisible by 3. | 0 |
| 153 | 153÷3 = 51, so 153 is divisible by 3. | 0 |
| 156 | 156÷3 = 52, so 156 is divisible by 3. | 0 |
| 159 | 159÷3 = 53, so 159 is divisible by 3. | 0 |
| 162 | 162÷3 = 54, so 162 is divisible by 3. | 0 |
| 165 | 165÷3 = 55, so165 is divisible by 3. | 0 |
| 168 | 168÷3 = 56, so 168 is divisible by 3. | 0 |
| 171 | 171÷3 = 57, so 171 is divisible by 3. | 0 |
| 174 | 174÷3 = 58, so 174 is divisible by 3. | 0 |
| 177 | 177÷3 = 59, so 177 is divisible by 3. | 0 |
| 180 | 180÷3 = 60, so 180 is divisible by 3. | 0 |
| 183 | 183÷3 = 61, so 183 is divisible by 3. | 0 |
| 186 | 186÷3 = 62, so 186 is divisible by 3. | 0 |
| 189 | 189÷3 = 63, so 189 is divisible by 3. | 0 |
| 192 | 192÷3 = 64, so 192 is divisible by 3. | 0 |
| 195 | 195÷3 = 65, so 1195 is divisible by 3. | 0 |
| 198 | 198÷3 = 66, so 198 is divisible by 3. | 0 |
| 201 | 201÷3 = 67, so 201 is divisible by 3. | 0 |
| 204 | 204÷3 = 68, so 204 is divisible by 3. | 0 |
| 207 | 207÷3 = 69, so 207 is divisible by 3. | 0 |
| 210 | 210÷3 = 70, so 210 is divisible by 3. | 0 |
| 213 | 213÷3 = 71, so 213 is divisible by 3. | 0 |
| 216 | 216÷3 = 72, so 216 is divisible by 3. | 0 |
| 219 | 219÷3 = 73, so219 is divisible by 3. | 0 |
| 222 | 222÷3 = 74, so 222 is divisible by 3. | 0 |
| 225 | 225÷3 = 75, so 225 is divisible by 3. | 0 |
| 228 | 228÷3 = 76, so 228 is divisible by 3. | 0 |
| 231 | 231÷3 = 77, so 231 is divisible by 3. | 0 |
| 234 | 234÷3 = 78, so 234 is divisible by 3. | 0 |
| 237 | 237÷3 = 79, so 237 is divisible by 3. | 0 |
| 240 | 240÷3 = 80, so 240 is divisible by 3. | 0 |
| 243 | 243÷3 = 81, so 243 is divisible by 3. | 0 |
| 246 | 246÷3 = 82, so 246 is divisible by 3. | 0 |
| 249 | 249÷3=83, so 249 is divisible by 3. | 0 |
| 252 | 252÷3 = 84, so 252 is divisible by 3. | 0 |
| 255 | 255÷3 = 85, so 255 is divisible by 3. | 0 |
| 258 | 258÷3 = 86, so 258 is divisible by 3. | 0 |
| 261 | 261÷3 = 87, so 261 is divisible by 3. | 0 |
| 264 | 264÷3 = 88, so 264 is divisible by 3. | 0 |
| 267 | 267÷3 = 89, so 267 is divisible by 3. | 0 |
| 270 | 270÷3 = 90, so 270 is divisible by 3. | 0 |
| 273 | 273÷3 = 91, so 273 is divisible by 3. | 0 |
| 276 | 276÷3 = 92, so 276 is divisible by 3. | 0 |
| 279 | 279÷3 = 93, so 279 is divisible by 3. | 0 |
| 282 | 282÷3 = 94, so 282 is divisible by 3. | 0 |
| 285 | 285÷3 = 95, so 285 is divisible by 3. | 0 |
| 288 | 288÷3 = 96, so 288 is divisible by 3. | 0 |
| 291 | 291÷3 = 97, so 291 is divisible by 3. | 0 |
| 294 | 294÷3 = 98, so 94 is divisible by 3. | 0 |
| 297 | 297÷3 = 99, so 297 is divisible by 3. | 0 |
| 300 | 300÷3 = 100, so 300 is divisible by 3. | 0 |
Read More About Multiples of 3
Important Notes
- Definition: Multiples of a number are obtained by multiplying the number by integers (e.g., multiples of 2 are 2, 4, 6, 8, …).
- Divisibility: A number is a multiple of another if it can be divided by that number without leaving a remainder.
- Sequence: The sequence of multiples is infinite. For instance, multiples of 2 are 2, 4, 6, 8, 10, and so on, continuing indefinitely.
- Applications: Understanding multiples is crucial for solving problems in arithmetic, algebra, and number theory, such as finding the least common multiple (LCM) and greatest common divisor (GCD).
- Pattern Recognition: Recognizing patterns in multiples helps in simplifying calculations and solving mathematical puzzles and problems effectively.
Examples on Multiples of 3
Example 1: Checking if a Number is a Multiple of 3
To determine if 45 is a multiple of 3, divide 45 by 3: 45÷3 = 15 Since the result is an integer with no remainder, 45 is a multiple of 3.
Example 2: Finding Multiples of 3
To find the first five multiples of 3, multiply 3 by the first five integers:
- 3×1 = 3
- 3×2 = 6
- 3×3 = 9
- 3×4 = 12
- 3×5 = 15
Thus, the first five multiples of 3 are 3, 6, 9, 12, and 15.
Example 3: Real-Life Application
Consider you have 27 apples, and you want to distribute them equally into groups of 3: 27÷3 = 9 You will have 9 groups with 3 apples each. This shows that 27 is a multiple of 3.
Example 4: Summing Multiples of 3
Find the sum of the multiples of 3 up to 15:
- Multiples: 3, 6, 9, 12, 15
- Sum: 3+6+9+12+15 = 45
Therefore, the sum of the multiples of 3 up to 15 is 45.
Example 5: Multiples of 3 within a Range
Identify multiples of 3 between 10 and 30:
- Starting from 12 (the first multiple of 3 greater than 10)
- 12, 15, 18, 21, 24, 27, 30
Practical Examples of Multiples of 3
Example 1: Sharing Candies
You have 18 candies and want to divide them equally among 3 friends. Each friend gets: 18÷3 = 6 So, 18 is a multiple of 3.
Example 2: Counting Steps
If you take 3 steps at a time, after 5 times, you will have taken: 3×5 = 15 Therefore, 15 steps is a multiple of 3.
Example 3: Packing Items
You have 27 books to pack into boxes, with 3 books per box. You will need: 27÷3 = 9 This means 27 is a multiple of 3, requiring 9 boxes.
Example 4: Buying Tickets
Concert tickets are sold in packs of 3. If you need 12 tickets, you will buy: 3×4 = 12 So, 12 is a multiple of 3, and you buy 4 packs.
Example 5: Arranging Chairs
You want to arrange 30 chairs in rows of 3. The number of rows will be: 30÷3 = 10 Thus, 30 is a multiple of 3, and you will have 10 rows.
FAQs
What is a multiple of 3?
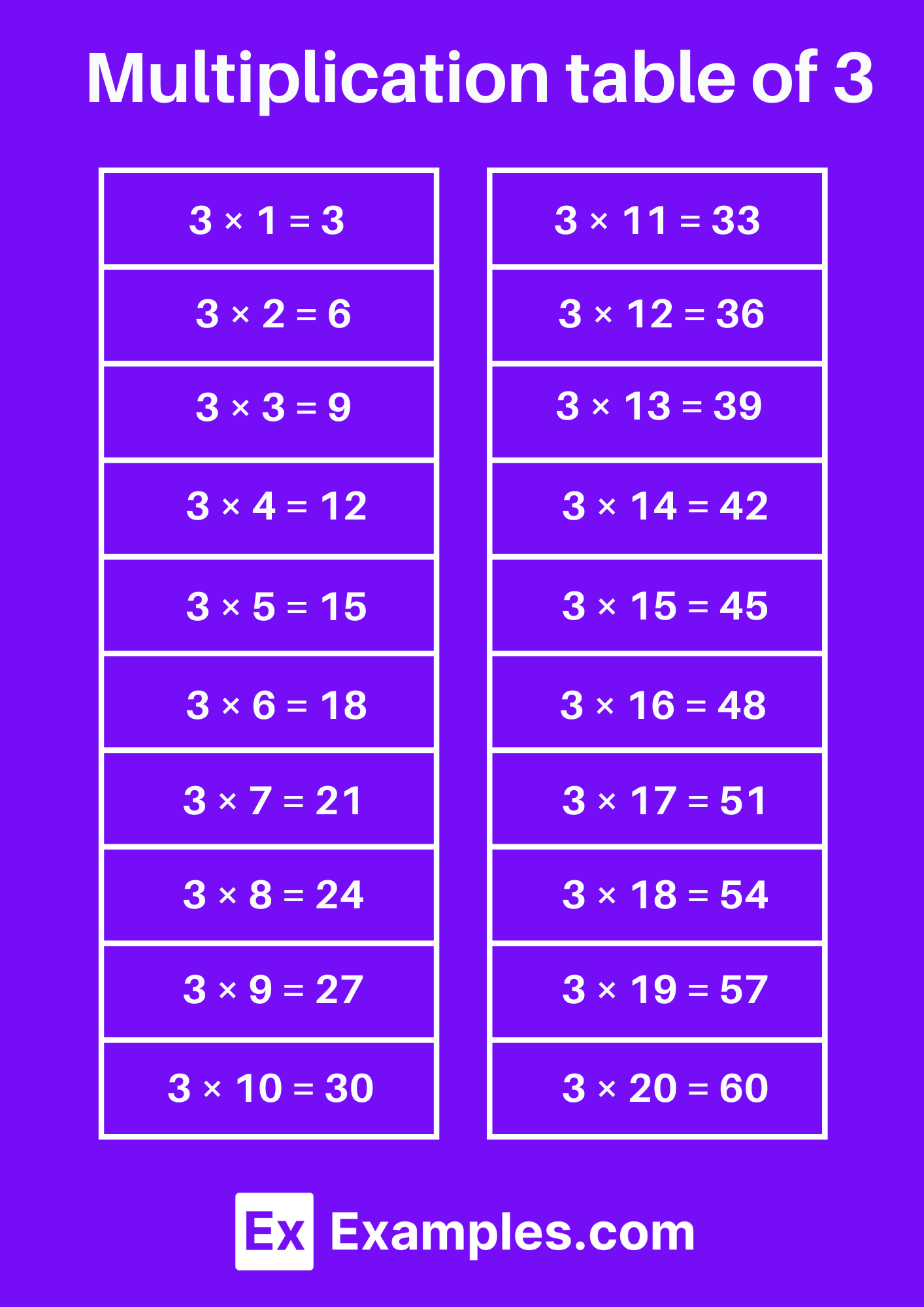
A multiple of 3 is any number that can be evenly divided by 3 without leaving a remainder. Examples include 3, 6, 9, 12, and so on.
How can I determine if a number is a multiple of 3?
To determine if a number is a multiple of 3, divide the number by 3. If the result is a whole number with no remainder, then it is a multiple of 3.
What are the first five multiples of 3?
The first five multiples of 3 are 3, 6, 9, 12, and 15.
Are all multiples of 3 also multiples of other numbers?
Not necessarily. While some multiples of 3 might also be multiples of other numbers (e.g., 6 is a multiple of both 3 and 2), others may not be (e.g., 9 is only a multiple of 3).
Why are multiples of 3 important in mathematics?
Multiples of 3 are important in mathematics for understanding patterns, solving problems involving divisibility, and finding the least common multiple (LCM) and greatest common divisor (GCD).
Can negative numbers be multiples of 3?
Yes, negative numbers can also be multiples of 3. Examples include -3, -6, -9, and so on.
How are multiples of 3 used in real life?
Multiples of 3 are used in various real-life applications such as event planning, packaging, cooking, and financial planning, where grouping or dividing items into sets of 3 is practical.
Is zero considered a multiple of 3?
Yes, zero is considered a multiple of 3 because 0÷3=0 with no remainder.
What is the relationship between multiples of 3 and the number 9?
Multiples of 9 are also multiples of 3. This is because 9 itself is a multiple of 3 ( 9 = 3×3). Thus, any multiple of 9 can be evenly divided by 3.
What is the rule for finding multiples of 3 using digit sum?
A number is a multiple of 3 if the sum of its digits is a multiple of 3. For example, for 123, the sum of digits is 1+2+3 = 6, which is a multiple of 3, so 123 is a multiple of 3.
Share :
