What is the 4th multiple of 330?
1210
1320
1350
1240
of 10
Multiples of 330 – 100+ List, Examples
Multiples of 330 – 100+ List, Examples
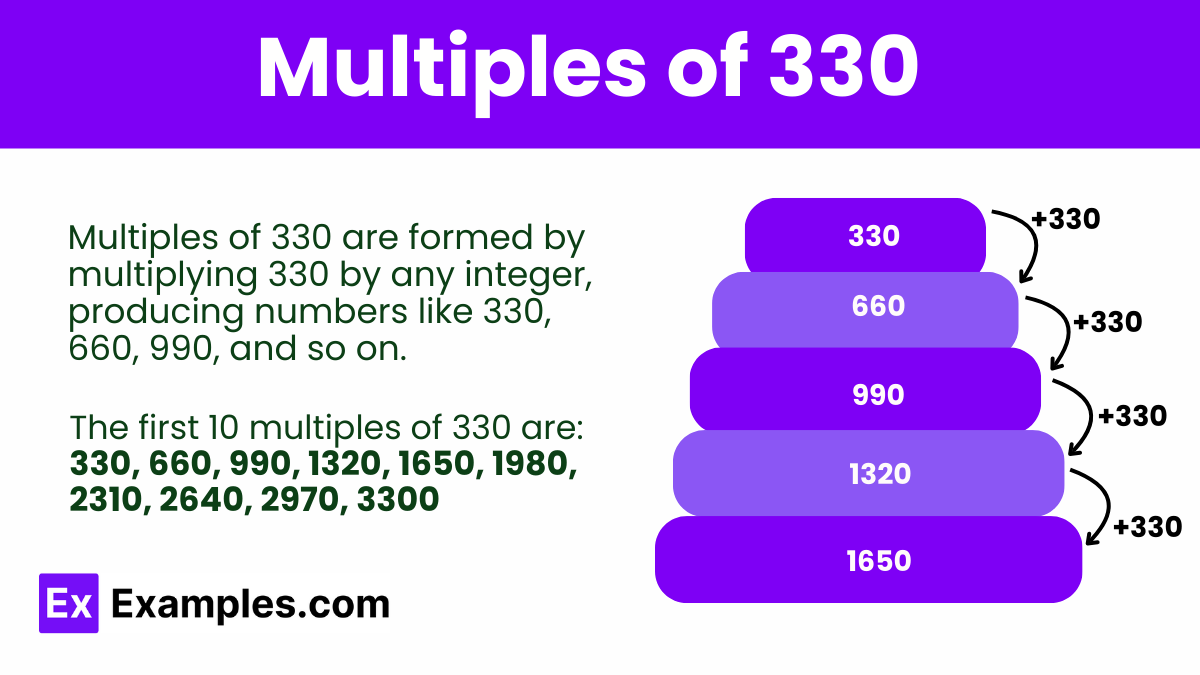
Multiples of 330 are numbers that can be expressed as 330×n, where 𝑛n is an integer. These multiples increase by 330 each time (e.g., 330, 660, 990, 1320, 1650) and are not necessarily even. Multiples of 330 are crucial in mathematics, especially in algebra, squares, square roots, and fractions. They help in understanding the properties of numbers and performing arithmetic operations efficiently. Recognizing these multiples aids in grasping complex mathematical ideas and solving algebraic equations. Multiples serve as essential building blocks in number theory, allowing the exploration of patterns, relationships, and the behavior of numbers within mathematical frameworks. They are fundamental in understanding the structure and properties of numbers.
What are Multiples of 330?
Multiples of 330 are numbers that can be expressed as 330×n, where n is an integer. These numbers are always even and include values like 330, 660, 990, 1320, and so on.
First 10 Multiples of 330 are 330, 660, 990, 1320, 1650, 1980, 2310, 2640, 2970, 3300
First 50 Multiples of 330 are 330, 660, 990, 1320, 1650, 1980, 2310, 2640, 2970, 3300, 3630, 3960, 4290, 4620, 4950, 5280, 5610, 5940, 6270, 6600, 6930, 7260, 7590, 7920, 8250, 8580, 8910, 9240, 9570, 9900, 10230, 10560, 10890, 11220, 11550, 11880, 12210, 12540, 12870, 13200, 13530, 13860, 14190, 14520, 14850, 15180, 15510, 15840, 16170, 16500.
For example, 330 , 660, 990 and 1320 are all multiples of 330, 655 is not a multiple of 330 for the following reasons:
| Number | Reason | Remainder |
|---|---|---|
| 330 | 330×1=330330×1=330, thus it is a multiple of 330 | 0 |
| 660 | 330×2=660330×2=660, thus it is a multiple of 330 | 0 |
| 990 | 330×3=990330×3=990, thus it is a multiple of 330 | 0 |
| 1320 | 330×4=1320330×4=1320, thus it is a multiple of 330 | 0 |
| 655 | 655÷330=1655÷330=1 with a remainder of 325, thus it is not a multiple of 330 | 325 |
List of First 100 Multiples of 330 with Remainders
| Number | Reason | Remainder |
|---|---|---|
| 330 | 330×1=330330×1=330, thus it is a multiple of 330 | 0 |
| 660 | 330×2=660330×2=660, thus it is a multiple of 330 | 0 |
| 990 | 330×3=990330×3=990, thus it is a multiple of 330 | 0 |
| 1320 | 330×4=1320330×4=1320, thus it is a multiple of 330 | 0 |
| 1650 | 330×5=1650330×5=1650, thus it is a multiple of 330 | 0 |
| 1980 | 330×6=1980330×6=1980, thus it is a multiple of 330 | 0 |
| 2310 | 330×7=2310330×7=2310, thus it is a multiple of 330 | 0 |
| 2640 | 330×8=2640330×8=2640, thus it is a multiple of 330 | 0 |
| 2970 | 330×9=2970330×9=2970, thus it is a multiple of 330 | 0 |
| 3300 | 330×10=3300330×10=3300, thus it is a multiple of 330 | 0 |
| 3630 | 330×11=3630330×11=3630, thus it is a multiple of 330 | 0 |
| 3960 | 330×12=3960330×12=3960, thus it is a multiple of 330 | 0 |
| 4290 | 330×13=4290330×13=4290, thus it is a multiple of 330 | 0 |
| 4620 | 330×14=4620330×14=4620, thus it is a multiple of 330 | 0 |
| 4950 | 330×15=4950330×15=4950, thus it is a multiple of 330 | 0 |
| 5280 | 330×16=5280330×16=5280, thus it is a multiple of 330 | 0 |
| 5610 | 330×17=5610330×17=5610, thus it is a multiple of 330 | 0 |
| 5940 | 330×18=5940330×18=5940, thus it is a multiple of 330 | 0 |
| 6270 | 330×19=6270330×19=6270, thus it is a multiple of 330 | 0 |
| 6600 | 330×20=6600330×20=6600, thus it is a multiple of 330 | 0 |
| 6930 | 330×21=6930330×21=6930, thus it is a multiple of 330 | 0 |
| 7260 | 330×22=7260330×22=7260, thus it is a multiple of 330 | 0 |
| 7590 | 330×23=7590330×23=7590, thus it is a multiple of 330 | 0 |
| 7920 | 330×24=7920330×24=7920, thus it is a multiple of 330 | 0 |
| 8250 | 330×25=8250330×25=8250, thus it is a multiple of 330 | 0 |
| 8580 | 330×26=8580330×26=8580, thus it is a multiple of 330 | 0 |
| 8910 | 330×27=8910330×27=8910, thus it is a multiple of 330 | 0 |
| 9240 | 330×28=9240330×28=9240, thus it is a multiple of 330 | 0 |
| 9570 | 330×29=9570330×29=9570, thus it is a multiple of 330 | 0 |
| 9900 | 330×30=9900330×30=9900, thus it is a multiple of 330 | 0 |
| 10230 | 330×31=10230330×31=10230, thus it is a multiple of 330 | 0 |
| 10560 | 330×32=10560330×32=10560, thus it is a multiple of 330 | 0 |
| 10890 | 330×33=10890330×33=10890, thus it is a multiple of 330 | 0 |
| 11220 | 330×34=11220330×34=11220, thus it is a multiple of 330 | 0 |
| 11550 | 330×35=11550330×35=11550, thus it is a multiple of 330 | 0 |
| 11880 | 330×36=11880330×36=11880, thus it is a multiple of 330 | 0 |
| 12210 | 330×37=12210330×37=12210, thus it is a multiple of 330 | 0 |
| 12540 | 330×38=12540330×38=12540, thus it is a multiple of 330 | 0 |
| 12870 | 330×39=12870330×39=12870, thus it is a multiple of 330 | 0 |
| 13200 | 330×40=13200330×40=13200, thus it is a multiple of 330 | 0 |
| 13530 | 330×41=13530330×41=13530, thus it is a multiple of 330 | 0 |
| 13860 | 330×42=13860330×42=13860, thus it is a multiple of 330 | 0 |
| 14190 | 330×43=14190330×43=14190, thus it is a multiple of 330 | 0 |
| 14520 | 330×44=14520330×44=14520, thus it is a multiple of 330 | 0 |
| 14850 | 330×45=14850330×45=14850, thus it is a multiple of 330 | 0 |
| 15180 | 330×46=15180330×46=15180, thus it is a multiple of 330 | 0 |
| 15510 | 330×47=15510330×47=15510, thus it is a multiple of 330 | 0 |
| 15840 | 330×48=15840330×48=15840, thus it is a multiple of 330 | 0 |
| 16170 | 330×49=16170330×49=16170, thus it is a multiple of 330 | 0 |
| 16500 | 330×50=16500330×50=16500, thus it is a multiple of 330 | 0 |
| 16830 | 330×51=16830330×51=16830, thus it is a multiple of 330 | 0 |
| 17160 | 330×52=17160330×52=17160, thus it is a multiple of 330 | 0 |
| 17490 | 330×53=17490330×53=17490, thus it is a multiple of 330 | 0 |
| 17820 | 330×54=17820330×54=17820, thus it is a multiple of 330 | 0 |
| 18150 | 330×55=18150330×55=18150, thus it is a multiple of 330 | 0 |
| 18480 | 330×56=18480330×56=18480, thus it is a multiple of 330 | 0 |
| 18810 | 330×57=18810330×57=18810, thus it is a multiple of 330 | 0 |
| 19140 | 330×58=19140330×58=19140, thus it is a multiple of 330 | 0 |
| 19470 | 330×59=19470330×59=19470, thus it is a multiple of 330 | 0 |
| 19800 | 330×60=19800330×60=19800, thus it is a multiple of 330 | 0 |
| 20130 | 330×61=20130330×61=20130, thus it is a multiple of 330 | 0 |
| 20460 | 330×62=20460330×62=20460, thus it is a multiple of 330 | 0 |
| 20790 | 330×63=20790330×63=20790, thus it is a multiple of 330 | 0 |
| 21120 | 330×64=21120330×64=21120, thus it is a multiple of 330 | 0 |
| 21450 | 330×65=21450330×65=21450, thus it is a multiple of 330 | 0 |
| 21780 | 330×66=21780330×66=21780, thus it is a multiple of 330 | 0 |
| 22110 | 330×67=22110330×67=22110, thus it is a multiple of 330 | 0 |
| 22440 | 330×68=22440330×68=22440, thus it is a multiple of 330 | 0 |
| 22770 | 330×69=22770330×69=22770, thus it is a multiple of 330 | 0 |
| 23100 | 330×70=23100330×70=23100, thus it is a multiple of 330 | 0 |
| 23430 | 330×71=23430330×71=23430, thus it is a multiple of 330 | 0 |
| 23760 | 330×72=23760330×72=23760, thus it is a multiple of 330 | 0 |
| 24090 | 330×73=24090330×73=24090, thus it is a multiple of 330 | 0 |
| 24420 | 330×74=24420330×74=24420, thus it is a multiple of 330 | 0 |
| 24750 | 330×75=24750330×75=24750, thus it is a multiple of 330 | 0 |
| 25080 | 330×76=25080330×76=25080, thus it is a multiple of 330 | 0 |
| 25410 | 330×77=25410330×77=25410, thus it is a multiple of 330 | 0 |
| 25740 | 330×78=25740330×78=25740, thus it is a multiple of 330 | 0 |
| 26070 | 330×79=26070330×79=26070, thus it is a multiple of 330 | 0 |
| 26400 | 330×80=26400330×80=26400, thus it is a multiple of 330 | 0 |
| 26730 | 330×81=26730330×81=26730, thus it is a multiple of 330 | 0 |
| 27060 | 330×82=27060330×82=27060, thus it is a multiple of 330 | 0 |
| 27390 | 330×83=27390330×83=27390, thus it is a multiple of 330 | 0 |
| 27720 | 330×84=27720330×84=27720, thus it is a multiple of 330 | 0 |
| 28050 | 330×85=28050330×85=28050, thus it is a multiple of 330 | 0 |
| 28380 | 330×86=28380330×86=28380, thus it is a multiple of 330 | 0 |
| 28710 | 330×87=28710330×87=28710, thus it is a multiple of 330 | 0 |
| 29040 | 330×88=29040330×88=29040, thus it is a multiple of 330 | 0 |
| 29370 | 330×89=29370330×89=29370, thus it is a multiple of 330 | 0 |
| 29700 | 330×90=29700330×90=29700, thus it is a multiple of 330 | 0 |
| 30030 | 330×91=30030330×91=30030, thus it is a multiple of 330 | 0 |
| 30360 | 330×92=30360330×92=30360, thus it is a multiple of 330 | 0 |
| 30690 | 330×93=30690330×93=30690, thus it is a multiple of 330 | 0 |
| 31020 | 330×94=31020330×94=31020, thus it is a multiple of 330 | 0 |
| 31350 | 330×95=31350330×95=31350, thus it is a multiple of 330 | 0 |
| 31680 | 330×96=31680330×96=31680, thus it is a multiple of 330 | 0 |
| 32010 | 330×97=32010330×97=32010, thus it is a multiple of 330 | 0 |
| 32340 | 330×98=32340330×98=32340, thus it is a multiple of 330 | 0 |
| 32670 | 330×99=32670330×99=32670, thus it is a multiple of 330 | 0 |
| 33000 | 330×100=33000330×100=33000, thus it is a multiple of 330 | 0 |
Important Notes
- Even Numbers: All multiples of 330 are even numbers, meaning they end in 0.
- Divisibility: A number is a multiple of 330 if it can be divided by 330 with no remainder.
- Factors: Multiples of 330 have 330 as one of their factors.
- Infinite Sequence: There are infinitely many multiples of 330, extending indefinitely as 330, 660, 990, 1320, and so on.
- Arithmetic Pattern: The difference between consecutive multiples of 330 is always 330.
Examples on Multiples of 330
Simple Multiples
- 330: 330×1=330330×1=330
- 660: 330×2=660330×2=660
- 990: 330×3=990330×3=990
Larger Multiples
- 1650: 330×5=1650330×5=1650
- 3300: 330×10=3300330×10=3300
- 6600: 330×20=6600330×20=6600
Real-Life Examples
- Time: 19800 seconds in 5.5 hours is a multiple of 330 because 330×60=19800330×60=19800.
- Money: $3300 is a multiple of 330 because 330×10=3300330×10=3300.
- Measurements: 11880 inches in 330 yards is a multiple of 330 because 330×36=11880330×36=11880.
Practical Examples of Multiples of 330
- Work Hours: A project requiring 660 hours can be divided into 330×2330×2 work hours.
- Travel Distance: A round trip of 990 miles can be planned using 330×3330×3 miles each way.
- Budgeting: A budget of $3300 can be allocated in 330×10330×10 dollar increments.
- Event Planning: Seating for 1320 guests can be arranged using 330×4330×4 seats per section.
- Storage: A warehouse holding 1650 items can store 330×5330×5 items per unit.
Practical Applications
- Counting by 330: When counting by 330 (330, 660, 990, 1320…), you are listing the multiples of 330.
- Large Quantities: Any large quantity that is a multiple of 330, such as 6600 or 9900, can be divided evenly by 330.
Can a multiple of 330 be odd?
No, a multiple of 330 cannot be odd since 330 is even.
What is the 15th multiple of 330?
The 15th multiple of 330 is 330×15=4950
Is 990 a multiple of 330?
Yes, 990 is a multiple of 330 because 330×3=990
How many multiples of 330 are there between 1000 and 5000?
There are 12 multiples of 330 between 1000 and 5000 (1320, 1650, 1980, 2310, 2640, 2970, 3300, 3630, 3960, 4290, 4620, 4950).
Is 1980 a multiple of 330?
Yes, 1980 is a multiple of 330 because 330×6=1980
What is the largest multiple of 330 less than 10000?
The largest multiple of 330 less than 10000 is 9900.
Is 13200 a multiple of 330?
Yes, 13200 is a multiple of 330 because 330×40=13200
Are multiples of 330 also multiples of 110?
Yes, multiples of 330 are also multiples of 110 because 330 is a multiple of 110.
What is the 25th multiple of 330?
The 25th multiple of 330 is 330×25=8250
Is 2970 a multiple of 330?
Yes, 2970 is a multiple of 330 because 330×9=2970
What is the next multiple of 330 after 4620?
The next multiple of 330 after 4620 is 4950.
Share :
Practice Test
Which of the following numbers is a multiple of 330?
990
985
995
1000
of 10
What is the product of 330 and 6?
1980
1990
1970
1960
of 10
If you add 330 to 1650, what do you get?
1970
1980
1990
2000
of 10
What is the 8th multiple of 330?
2620
2630
2640
2650
of 10
Which of the following is a multiple of 330 closest to 3000?
2970
2980
2990
3000
of 10
If 330 is multiplied by 11, what is the product?
3610
3620
3630
3640
of 10
What is the next multiple of 330 after 2310?
2630
2640
2650
2660
of 10
Which of the following is the sum of two multiples of 330?
660
670
680
690
of 10
How many multiples of 330 are there between 1000 and 4000?
8
9
10
11
of 10