Multiples of 87

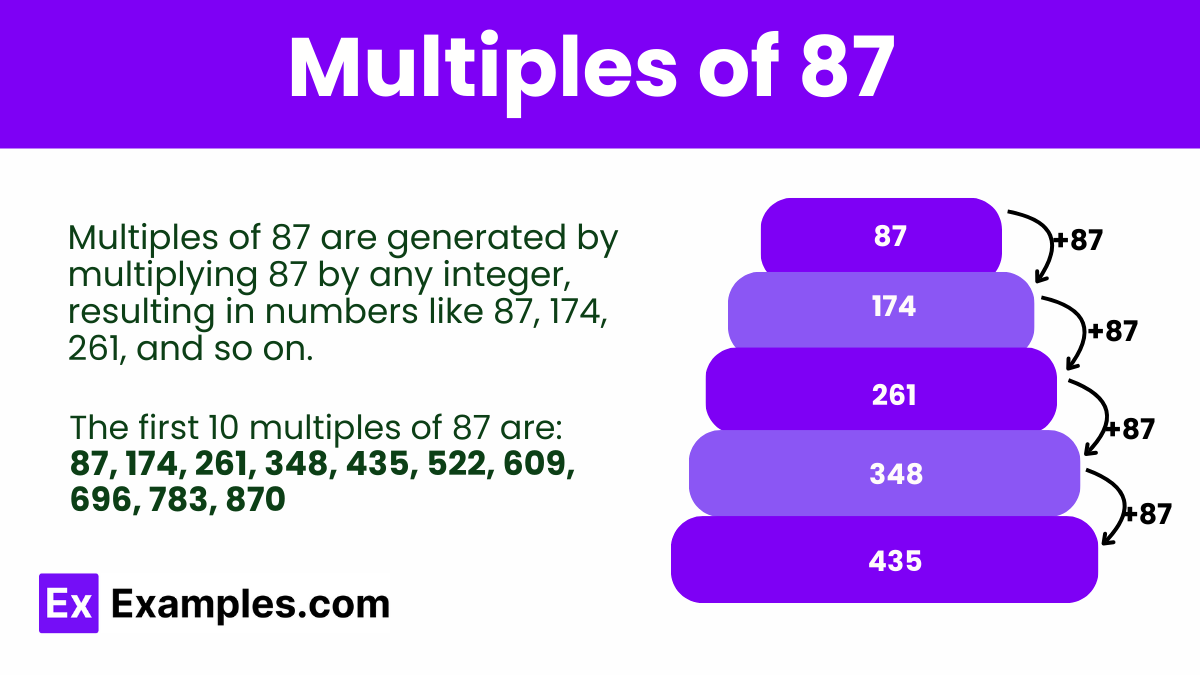
Multiples of 87 are numbers that can be expressed as (87 x n), where (n) is an integer. These multiples increase by 87 each time (e.g., 87, 174, 261, 348, 435). They are not necessarily even but follow a consistent pattern. Multiples of 87 are essential in mathematics, particularly in algebraic concepts, squares, square roots, and fractions. They play a fundamental role in understanding the properties of numbers and performing various arithmetic operations efficiently. Recognizing these multiples aids in grasping more complex mathematical ideas and solving algebraic equations. Multiples serve as essential building blocks in number theory, helping to explore patterns, relationships, and the behavior of numbers within mathematical frameworks, thereby enhancing a student’s comprehension of mathematical principles.

What are Multiples of 87?

Multiples of 87 are numbers that can be expressed as 87×n, where n is an integer. These numbers are always even and include values like 87, 174, 261, 348, and so on.

Prime Factorization of 87: 3 x 29

First 10 Multiples of 87 are 2, 4, 6, 8, 10, 12, 14, 16, 18, 20.

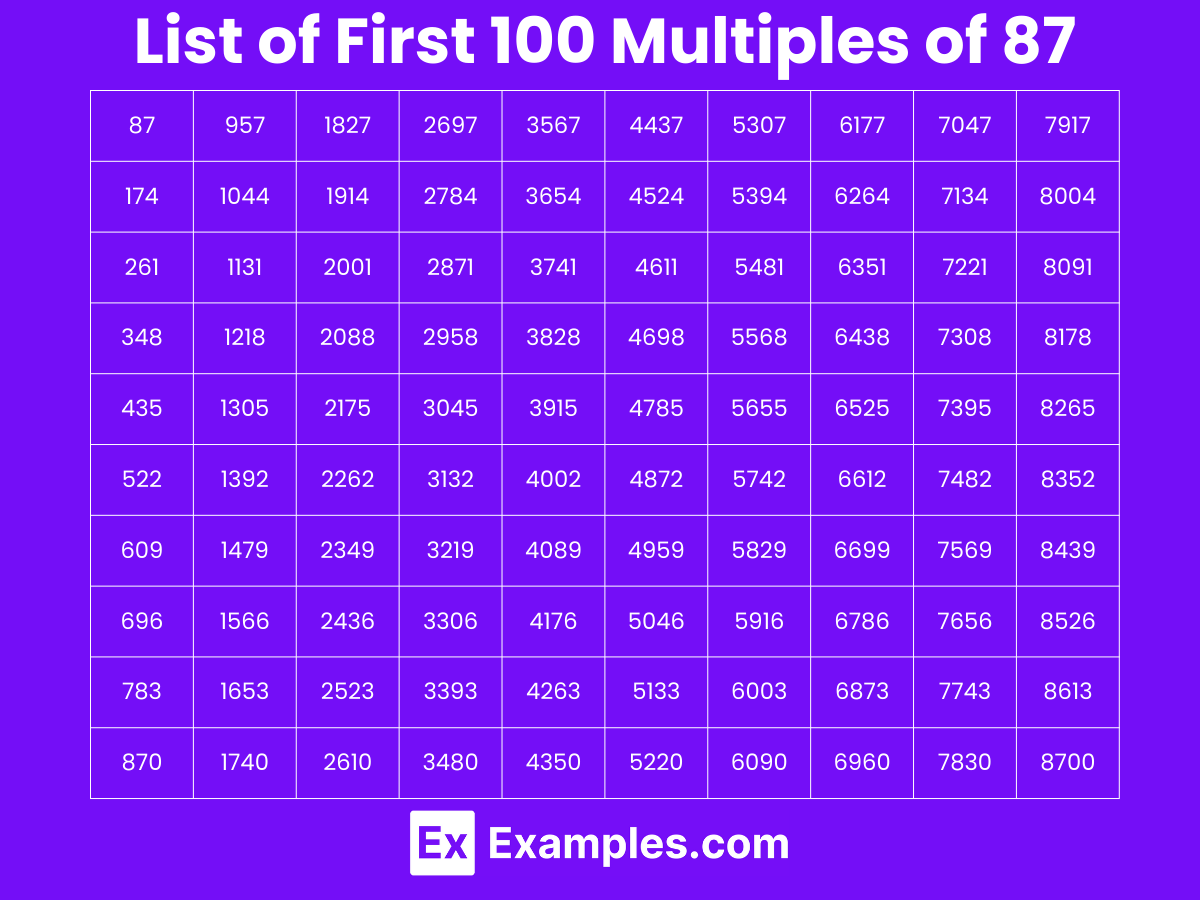
First 50 Multiples of 87 are 87, 174, 261, 348, 435, 522, 609, 696, 783, 870, 957, 1044, 1131, 1218, 1305, 1392, 1479, 1566, 1653, 1740, 1827, 1914, 2001, 2088, 2175, 2262, 2349, 2436, 2523, 2610, 2697, 2784, 2871, 2958, 3045, 3132, 3219, 3306, 3393, 3480, 3567, 3654, 3741, 3828, 3915, 4002, 4089, 4176, 4263, 4350

For example, 87, 174, 261and 348 are all multiples of 87, 133 is not a multiple of 87 for the following reasons:

Number	Reason	Remainder
87	87 × 1 = 87, which is exactly divisible by 87	0
174	87 × 2 = 174, which is exactly divisible by 87	0
261	87 × 3 = 261, which is exactly divisible by 87	0
348	87 × 4 = 348, which is exactly divisible by 87	0
133	133 ÷ 87 = 1 with a remainder, not exactly divisible	46

List of First 100 Multiples of 87 with Remainders

Number	Reason	Remainder
87	87 × 1 = 87, which is exactly divisible by 87	0
174	87 × 2 = 174, which is exactly divisible by 87	0
261	87 × 3 = 261, which is exactly divisible by 87	0
348	87 × 4 = 348, which is exactly divisible by 87	0
435	87 × 5 = 435, which is exactly divisible by 87	0
522	87 × 6 = 522, which is exactly divisible by 87	0
609	87 × 7 = 609, which is exactly divisible by 87	0
696	87 × 8 = 696, which is exactly divisible by 87	0
783	87 × 9 = 783, which is exactly divisible by 87	0
870	87 × 10 = 870, which is exactly divisible by 87	0
957	87 × 11 = 957, which is exactly divisible by 87	0
1044	87 × 12 = 1044, which is exactly divisible by 87	0
1131	87 × 13 = 1131, which is exactly divisible by 87	0
1218	87 × 14 = 1218, which is exactly divisible by 87	0
1305	87 × 15 = 1305, which is exactly divisible by 87	0
1392	87 × 16 = 1392, which is exactly divisible by 87	0
1479	87 × 17 = 1479, which is exactly divisible by 87	0
1566	87 × 18 = 1566, which is exactly divisible by 87	0
1653	87 × 19 = 1653, which is exactly divisible by 87	0
1740	87 × 20 = 1740, which is exactly divisible by 87	0
1827	87 × 21 = 1827, which is exactly divisible by 87	0
1914	87 × 22 = 1914, which is exactly divisible by 87	0
2001	87 × 23 = 2001, which is exactly divisible by 87	0
2088	87 × 24 = 2088, which is exactly divisible by 87	0
2175	87 × 25 = 2175, which is exactly divisible by 87	0
2262	87 × 26 = 2262, which is exactly divisible by 87	0
2349	87 × 27 = 2349, which is exactly divisible by 87	0
2436	87 × 28 = 2436, which is exactly divisible by 87	0
2523	87 × 29 = 2523, which is exactly divisible by 87	0
2610	87 × 30 = 2610, which is exactly divisible by 87	0
2697	87 × 31 = 2697, which is exactly divisible by 87	0
2784	87 × 32 = 2784, which is exactly divisible by 87	0
2871	87 × 33 = 2871, which is exactly divisible by 87	0
2958	87 × 34 = 2958, which is exactly divisible by 87	0
3045	87 × 35 = 3045, which is exactly divisible by 87	0
3132	87 × 36 = 3132, which is exactly divisible by 87	0
3219	87 × 37 = 3219, which is exactly divisible by 87	0
3306	87 × 38 = 3306, which is exactly divisible by 87	0
3393	87 × 39 = 3393, which is exactly divisible by 87	0
3480	87 × 40 = 3480, which is exactly divisible by 87	0
3567	87 × 41 = 3567, which is exactly divisible by 87	0
3654	87 × 42 = 3654, which is exactly divisible by 87	0
3741	87 × 43 = 3741, which is exactly divisible by 87	0
3828	87 × 44 = 3828, which is exactly divisible by 87	0
3915	87 × 45 = 3915, which is exactly divisible by 87	0
4002	87 × 46 = 4002, which is exactly divisible by 87	0
4089	87 × 47 = 4089, which is exactly divisible by 87	0
4176	87 × 48 = 4176, which is exactly divisible by 87	0
4263	87 × 49 = 4263, which is exactly divisible by 87	0
4350	87 × 50 = 4350, which is exactly divisible by 87	0
4437	87 × 51 = 4437, which is exactly divisible by 87	0
4524	87 × 52 = 4524, which is exactly divisible by 87	0
4611	87 × 53 = 4611, which is exactly divisible by 87	0
4698	87 × 54 = 4698, which is exactly divisible by 87	0
4785	87 × 55 = 4785, which is exactly divisible by 87	0
4872	87 × 56 = 4872, which is exactly divisible by 87	0
4959	87 × 57 = 4959, which is exactly divisible by 87	0
5046	87 × 58 = 5046, which is exactly divisible by 87	0
5133	87 × 59 = 5133, which is exactly divisible by 87	0
5220	87 × 60 = 5220, which is exactly divisible by 87	0
5307	87 × 61 = 5307, which is exactly divisible by 87	0
5394	87 × 62 = 5394, which is exactly divisible by 87	0
5481	87 × 63 = 5481, which is exactly divisible by 87	0
5568	87 × 64 = 5568, which is exactly divisible by 87	0
5655	87 × 65 = 5655, which is exactly divisible by 87	0
5742	87 × 66 = 5742, which is exactly divisible by 87	0
5829	87 × 67 = 5829, which is exactly divisible by 87	0
5916	87 × 68 = 5916, which is exactly divisible by 87	0
6003	87 × 69 = 6003, which is exactly divisible by 87	0
6090	87 × 70 = 6090, which is exactly divisible by 87	0
6177	87 × 71 = 6177, which is exactly divisible by 87	0
6264	87 × 72 = 6264, which is exactly divisible by 87	0
6351	87 × 73 = 6351, which is exactly divisible by 87	0
6438	87 × 74 = 6438, which is exactly divisible by 87	0
6525	87 × 75 = 6525, which is exactly divisible by 87	0
6612	87 × 76 = 6612, which is exactly divisible by 87	0
6699	87 × 77 = 6699, which is exactly divisible by 87	0
6786	87 × 78 = 6786, which is exactly divisible by 87	0
6873	87 × 79 = 6873, which is exactly divisible by 87	0
6960	87 × 80 = 6960, which is exactly divisible by 87	0
7047	87 × 81 = 7047, which is exactly divisible by 87	0
7134	87 × 82 = 7134, which is exactly divisible by 87	0
7221	87 × 83 = 7221, which is exactly divisible by 87	0
7308	87 × 84 = 7308, which is exactly divisible by 87	0
7395	87 × 85 = 7395, which is exactly divisible by 87	0
7482	87 × 86 = 7482, which is exactly divisible by 87	0
7569	87 × 87 = 7569, which is exactly divisible by 87	0
7656	87 × 88 = 7656, which is exactly divisible by 87	0
7743	87 × 89 = 7743, which is exactly divisible by 87	0
7830	87 × 90 = 7830, which is exactly divisible by 87	0
7917	87 × 91 = 7917, which is exactly divisible by 87	0
8004	87 × 92 = 8004, which is exactly divisible by 87	0
8091	87 × 93 = 8091, which is exactly divisible by 87	0
8178	87 × 94 = 8178, which is exactly divisible by 87	0
8265	87 × 95 = 8265, which is exactly divisible by 87	0
8352	87 × 96 = 8352, which is exactly divisible by 87	0
8439	87 × 97 = 8439, which is exactly divisible by 87	0
8526	87 × 98 = 8526, which is exactly divisible by 87	0
8613	87 × 99 = 8613, which is exactly divisible by 87	0
8700	87 × 100 = 8700, which is exactly divisible by 87	0

Read More About Multiples of 87

Table of 87

Important Notes

• Even Numbers: Not all multiples of 87 are even numbers. They can end in any digit, such as 0, 1, 2, 3, 4, 5, 6, 7, 8, or 9.
• Divisibility: A number is a multiple of 87 if it can be divided by 87 with no remainder.
• Factors: Multiples of 87 have 87 as one of their factors.
• Infinite Sequence: There are infinitely many multiples of 87, extending indefinitely as 87, 174, 261, 348, 435, and so on.
• Arithmetic Pattern: The difference between consecutive multiples of 87 is always 87.

Examples on Multiples of 87

Simple Multiples

174: 87 × 2 = 174

435: 87 × 5 = 435

696: 87 × 8 = 696

Larger Multiples

2175: 87 × 25 = 2175

4350: 87 × 50 = 4350

8700: 87 × 100 = 8700

Real-Life Examples

Time: 5220 seconds in an hour and 27 seconds is a multiple of 87 because 87 × 60 = 5220.

Money: $4350 is a multiple of 87 because 87 × 50 = 4350.

Measurements: 3132 inches is a multiple of 87 because 87 × 36 = 3132.

Practical Examples of Multiples of 87

1. Time Calculation: 5220 seconds in an hour and 27 seconds is a multiple of 87 because 87 × 60 = 5220.
2. Money Calculation: $4350 is a multiple of 87 because 87 × 50 = 4350.
3. Distance Measurement: 3132 inches is a multiple of 87 because 87 × 36 = 3132.
4. Event Planning: If an event occurs every 87 days, then in 870 days, it has occurred 10 times because 87 × 10 = 870.
5. Inventory Management: If a product is stocked in multiples of 87, then 2610 units represent 30 batches because 87 × 30 = 2610.

Practical Applications

Counting by Eighty-Sevens: When counting by eighty-sevens (87, 174, 261, 348…), you are listing the multiples of 87.

Multiple of 87: Any number that is a multiple of 87, such as 174 or 261, is a multiple of 87 because it can be divided evenly by 87.