Which of the following is a multiple of 5?
22
27
30
33

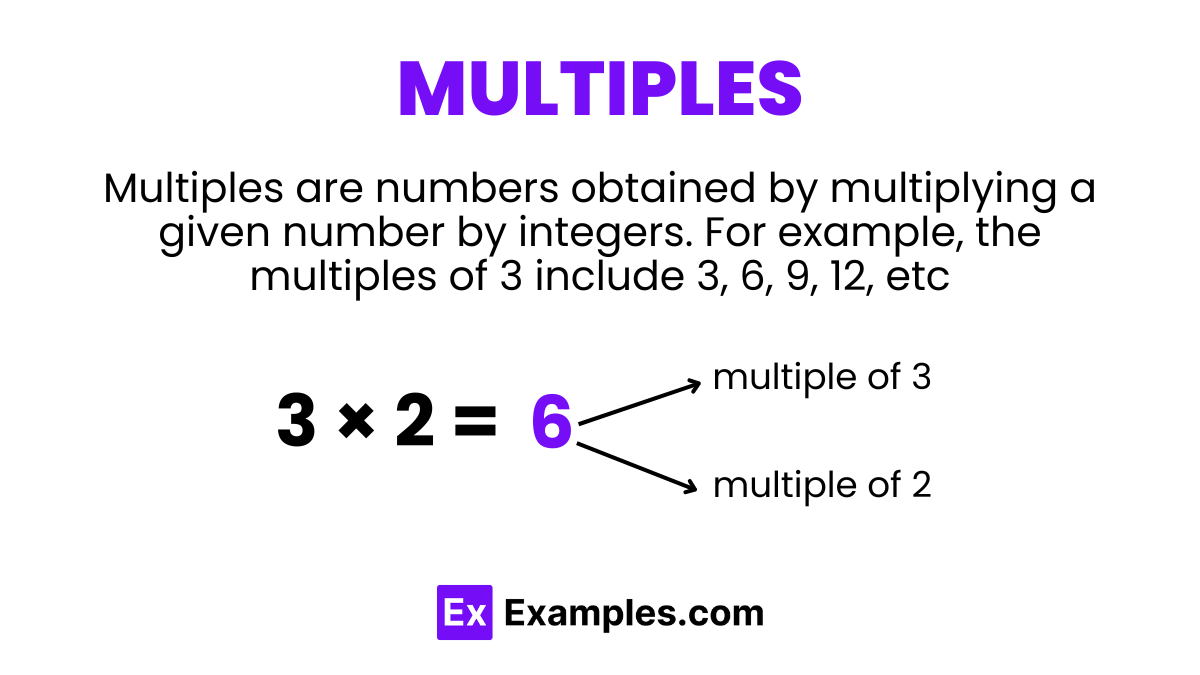
Discover the fascinating world of multiples in mathematics with our comprehensive guide. Ideal for educators, students, and math enthusiasts, this article demystifies the concept of multiples through clear explanations and engaging examples. Whether you’re tackling homework, preparing for exams, or just curious about math, our guide provides the insights you need. Dive into the world of multiples and enhance your mathematical understanding today.
Multiples in maths are the result of multiplying a number by an integer. Simply put, when you take any number and multiply it by 1, 2, 3, 4, and so on, the products you get are known as the multiples of that number. For instance, the multiples of 3 include 3 (3×1), 6 (3×2), 9 (3×3), 12 (3×4), and continue in this pattern. Essentially, multiples are what you count by when you skip count by a particular number, making them a fundamental concept in both arithmetic and algebra.
let’s a look of few examples here.
Multiples of 2: 2, 4, 6, 8, 10, 12, 14, 16, 18, 20
Multiples of 3: 3, 6, 9, 12, 15, 18, 21, 24, 27, 30
Multiples of 4: 4, 8, 12, 16, 20, 24, 28, 32, 36, 40
Multiples of 5: 5, 10, 15, 20, 25, 30, 35, 40, 45, 50
Multiples of 6: 6, 12, 18, 24, 30, 36, 42, 48, 54, 60
Multiples of 7: 7, 14, 21, 28, 35, 42, 49, 56, 63, 70
Multiples of 8: 8, 16, 24, 32, 40, 48, 56, 64, 72, 80
Multiples of 9: 9, 18, 27, 36, 45, 54, 63, 72, 81, 90
Multiples of 10: 10, 20, 30, 40, 50, 60, 70, 80, 90, 100
Understanding the properties of multiples is crucial for grasping how numbers interact within the mathematical universe. These properties not only simplify computations but also enable us to recognize patterns and solve problems more efficiently. Here, we delve into the fundamental characteristics that define the nature of multiples.
One of the most intriguing aspects of multiples is their infinite nature. For any given number, there exists an endless series of multiples. This is because you can keep multiplying the number by increasingly larger integers, extending the series indefinitely. For example, the multiples of 2 (2, 4, 6, 8, 10…) continue endlessly as you multiply 2 by larger numbers.
A lesser-known fact is that zero (0) is considered a multiple of every number. This is due to the property that any number multiplied by zero equals zero. Hence, zero uniquely stands as a universal multiple, underscoring the foundational role it plays in mathematics.
A number is a multiple of another if it can be divided by that number without leaving a remainder. This property of divisibility is fundamental to understanding multiples. For instance, 15 is a multiple of 3 and 5 because it can be divided evenly by these numbers (15 ÷ 3 = 5).
When two or more numbers share a multiple, it is known as a common multiple. The least common multiple (LCM) is particularly important in various mathematical operations, especially in solving fractions and equations. The LCM of two numbers is the smallest number that is a multiple of both.
Prime numbers, which are numbers greater than 1 that have no divisors other than 1 and themselves, have a unique relationship with multiples. Each prime number’s multiples are exclusively generated by multiplying the prime number by integers. For example, the multiples of 5 are all the numbers that can only be obtained by multiplying 5 by an integer.
Multiples often form recognizable patterns, which can be particularly helpful in mental math and problem-solving. For example, the multiples of 2 always end in 0, 2, 4, 6, or 8, reflecting the pattern of even numbers. Similarly, the multiples of 5 end in either 0 or 5. Recognizing these patterns can simplify computations and enhance number sense.
A multiple of a number is a number that is the product of a given number and some other natural number. Finding multiples of a number is a fundamental skill in mathematics that lays the groundwork for more complex operations and concepts. This guide will walk you through the steps to identify multiples, enhancing your mathematical proficiency and understanding.
Before diving into the process, it’s essential to grasp what multiples are. A multiple of a number is what you get when you multiply that number by an integer. Multiples are like the building blocks in the world of mathematics, forming patterns and sequences that are foundational to various mathematical concepts.
For this example, we’ve chosen the number 4.
We begin by multiplying 4 by integers (whole numbers) starting from 1. Here’s how it looks:
As we multiply, we list the products, which are the multiples of 4: 4, 8, 12, 16, 20, 24, 28, 32, 36, 40…
In this case, you might notice that all multiples of 4 are even numbers. This pattern can help you quickly identify multiples of 4, even without doing the multiplication explicitly.
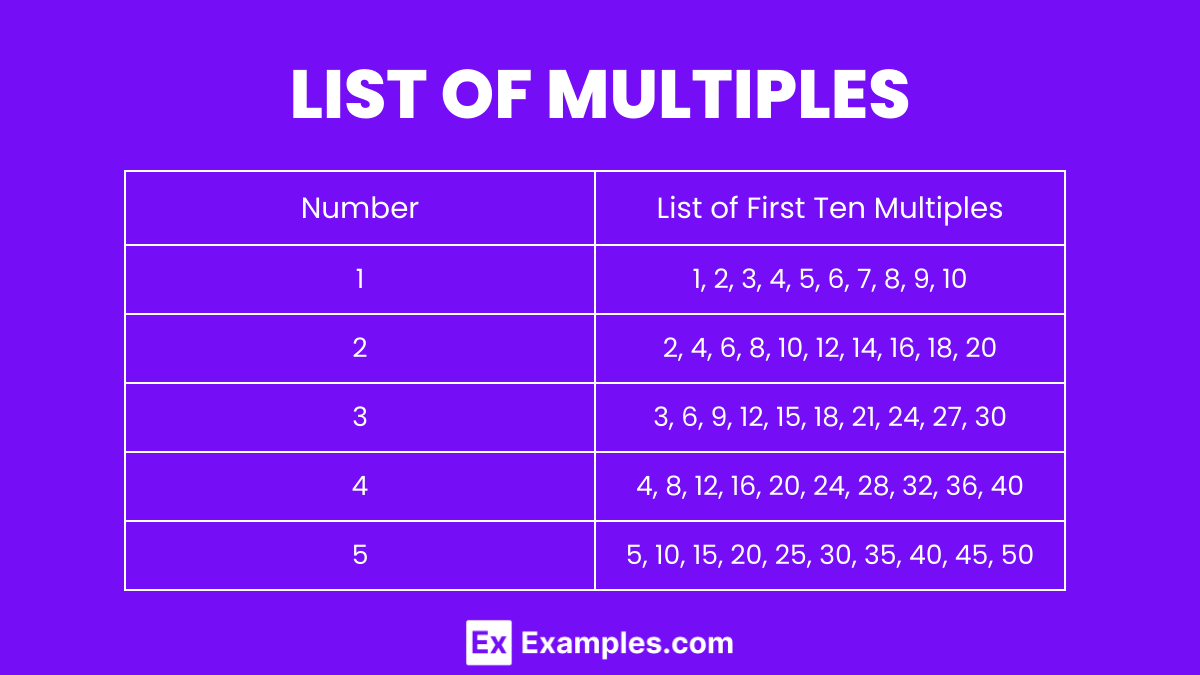 Creating a list of multiples in a table format is an effective way to visualize and understand the pattern of multiples for any given number. This approach is particularly useful in educational settings, aiding both teachers and students in exploring the concept of multiplication and its applications. Below, we provide a guide on how to create and interpret a multiples table, followed by an example for clarity.
Creating a list of multiples in a table format is an effective way to visualize and understand the pattern of multiples for any given number. This approach is particularly useful in educational settings, aiding both teachers and students in exploring the concept of multiplication and its applications. Below, we provide a guide on how to create and interpret a multiples table, followed by an example for clarity.
| Number | List of First Ten Multiples |
|---|---|
| 1 | 1, 2, 3, 4, 5, 6, 7, 8, 9, 10 |
| 2 | 2, 4, 6, 8, 10, 12, 14, 16, 18, 20 |
| 3 | 3, 6, 9, 12, 15, 18, 21, 24, 27, 30 |
| 4 | 4, 8, 12, 16, 20, 24, 28, 32, 36, 40 |
| 5 | 5, 10, 15, 20, 25, 30, 35, 40, 45, 50 |
| 6 | 6, 12, 18, 24, 30, 36, 42, 48, 54, 60 |
| 7 | 7, 14, 21, 28, 35, 42, 49, 56, 63, 70 |
| 8 | 8, 16, 24, 32, 40, 48, 56, 64, 72, 80 |
| 9 | 9, 18, 27, 36, 45, 54, 63, 72, 81, 90 |
| 10 | 10, 20, 30, 40, 50, 60, 70, 80, 90, 100 |
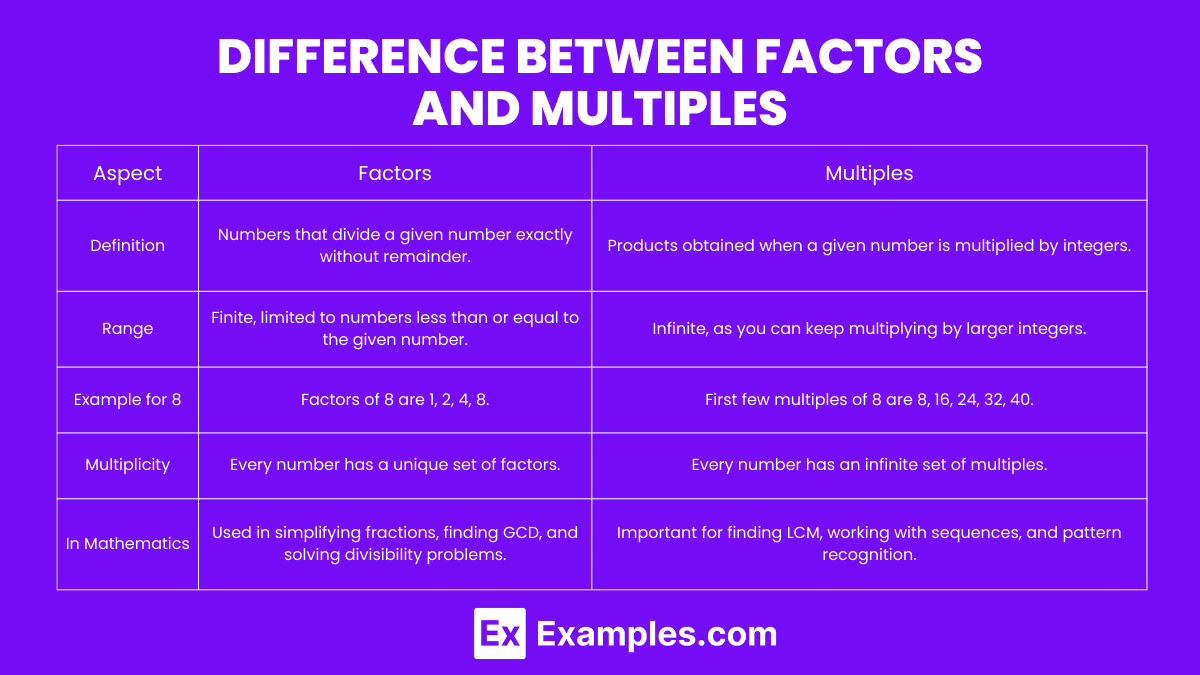 In the realm of mathematics, factors and multiples are fundamental concepts that often confuse learners due to their interconnected nature. However, understanding the distinction between these two is crucial for mastering arithmetic and algebra. This guide aims to elucidate the differences with clear definitions and illustrative examples.
In the realm of mathematics, factors and multiples are fundamental concepts that often confuse learners due to their interconnected nature. However, understanding the distinction between these two is crucial for mastering arithmetic and algebra. This guide aims to elucidate the differences with clear definitions and illustrative examples.
| Aspect | Factors | Multiples |
|---|---|---|
| Definition | Numbers that divide a given number exactly without remainder. | Products obtained when a given number is multiplied by integers. |
| Range | Finite, limited to numbers less than or equal to the given number. | Infinite, as you can keep multiplying by larger integers. |
| Example for 8 | Factors of 8 are 1, 2, 4, 8. | First few multiples of 8 are 8, 16, 24, 32, 40. |
| Multiplicity | Every number has a unique set of factors. | Every number has an infinite set of multiples. |
| In Mathematics | Used in simplifying fractions, finding GCD, and solving divisibility problems. | Important for finding LCM, working with sequences, and pattern recognition. |
| Pattern | Factors decrease in number as the given number increases. | Multiples increase as the given number increases. |
| Visibility | Factors are always less than or equal to the given number. | Multiples are always equal to or greater than the given number. |
In the mathematical landscape, the concept of common multiples plays a pivotal role in various arithmetic and algebraic operations. Understanding common multiples is essential for solving problems related to least common multiples (LCM), simplifying fractions, and finding solutions to equations involving multiple terms. This guide aims to shed light on what common multiples are, how to find them, and their practical applications, enhancing your mathematical toolkit.
Common multiples of two or more numbers are multiples that the numbers share. These are numbers that can be divided evenly by all the original numbers without any remainder. For instance, if you’re considering the numbers 4 and 6, some of their common multiples would be 12, 24, 36, and so on. Each of these common multiples can be divided evenly by both 4 and 6.
The multiples of 2 represent the essence of even numbers. Each product in this series is a result of multiplying 2 by integers ranging from 1 to 10. This sequence is fundamental in mathematics, illustrating the basic concept of even numbers, which are divisible by 2 without any remainder. Understanding these multiples is crucial for grasping the properties of even numbers, which play a significant role in various arithmetic operations and number theory.
The table showcasing the multiples of 3 highlights a pattern where each number is divisible by 3. This series is particularly important for understanding the concept of divisibility and for identifying numbers that are multiples of 3. These multiples are integral to various mathematical concepts, including factors, multiples, and patterns within the number system. Recognizing the sequence of multiples of 3 aids in developing arithmetic skills and enhances problem-solving capabilities.
The multiples of 4 table demonstrates how numbers increase when multiplied by 4. Each step in this series doubles the even number sequence, further emphasizing the structure within the number system. This table is useful for understanding the multiplication process and for recognizing patterns associated with the number 4. It serves as a valuable tool for students learning basic multiplication and for anyone working with numbers divisible by 4.
he multiples of 5 are easily recognizable by their ending digits, either 0 or 5. This pattern is not only simple to remember but also crucial for various arithmetic calculations, especially in mental math. The table of multiples of 5 is particularly useful in real-world applications, such as counting by fives, dealing with currency, and measuring time
The table of multiples of 6 combines the properties of even numbers and the divisibility by 3. This series is essential for understanding more complex patterns in the number system and for solving problems related to least common multiples and divisibility.
In conclusion, multiples form an essential part of mathematics, offering a foundation for understanding arithmetic patterns and solving complex problems. By mastering the concept of multiples and recognizing their patterns, learners can enhance their mathematical skills, facilitating easier computation and a deeper comprehension of numerical relationships, crucial for academic success and daily applications.

Discover the fascinating world of multiples in mathematics with our comprehensive guide. Ideal for educators, students, and math enthusiasts, this article demystifies the concept of multiples through clear explanations and engaging examples. Whether you’re tackling homework, preparing for exams, or just curious about math, our guide provides the insights you need. Dive into the world of multiples and enhance your mathematical understanding today.
Multiples in maths are the result of multiplying a number by an integer. Simply put, when you take any number and multiply it by 1, 2, 3, 4, and so on, the products you get are known as the multiples of that number. For instance, the multiples of 3 include 3 (3×1), 6 (3×2), 9 (3×3), 12 (3×4), and continue in this pattern. Essentially, multiples are what you count by when you skip count by a particular number, making them a fundamental concept in both arithmetic and algebra.
let’s a look of few examples here.
Multiples of 2: 2, 4, 6, 8, 10, 12, 14, 16, 18, 20
Multiples of 3: 3, 6, 9, 12, 15, 18, 21, 24, 27, 30
Multiples of 4: 4, 8, 12, 16, 20, 24, 28, 32, 36, 40
Multiples of 5: 5, 10, 15, 20, 25, 30, 35, 40, 45, 50
Multiples of 6: 6, 12, 18, 24, 30, 36, 42, 48, 54, 60
Multiples of 7: 7, 14, 21, 28, 35, 42, 49, 56, 63, 70
Multiples of 8: 8, 16, 24, 32, 40, 48, 56, 64, 72, 80
Multiples of 9: 9, 18, 27, 36, 45, 54, 63, 72, 81, 90
Multiples of 10: 10, 20, 30, 40, 50, 60, 70, 80, 90, 100
Understanding the properties of multiples is crucial for grasping how numbers interact within the mathematical universe. These properties not only simplify computations but also enable us to recognize patterns and solve problems more efficiently. Here, we delve into the fundamental characteristics that define the nature of multiples.
One of the most intriguing aspects of multiples is their infinite nature. For any given number, there exists an endless series of multiples. This is because you can keep multiplying the number by increasingly larger integers, extending the series indefinitely. For example, the multiples of 2 (2, 4, 6, 8, 10…) continue endlessly as you multiply 2 by larger numbers.
A lesser-known fact is that zero (0) is considered a multiple of every number. This is due to the property that any number multiplied by zero equals zero. Hence, zero uniquely stands as a universal multiple, underscoring the foundational role it plays in mathematics.
A number is a multiple of another if it can be divided by that number without leaving a remainder. This property of divisibility is fundamental to understanding multiples. For instance, 15 is a multiple of 3 and 5 because it can be divided evenly by these numbers (15 ÷ 3 = 5).
When two or more numbers share a multiple, it is known as a common multiple. The least common multiple (LCM) is particularly important in various mathematical operations, especially in solving fractions and equations. The LCM of two numbers is the smallest number that is a multiple of both.
Prime numbers, which are numbers greater than 1 that have no divisors other than 1 and themselves, have a unique relationship with multiples. Each prime number’s multiples are exclusively generated by multiplying the prime number by integers. For example, the multiples of 5 are all the numbers that can only be obtained by multiplying 5 by an integer.
Multiples often form recognizable patterns, which can be particularly helpful in mental math and problem-solving. For example, the multiples of 2 always end in 0, 2, 4, 6, or 8, reflecting the pattern of even numbers. Similarly, the multiples of 5 end in either 0 or 5. Recognizing these patterns can simplify computations and enhance number sense.
A multiple of a number is a number that is the product of a given number and some other natural number. Finding multiples of a number is a fundamental skill in mathematics that lays the groundwork for more complex operations and concepts. This guide will walk you through the steps to identify multiples, enhancing your mathematical proficiency and understanding.
Before diving into the process, it’s essential to grasp what multiples are. A multiple of a number is what you get when you multiply that number by an integer. Multiples are like the building blocks in the world of mathematics, forming patterns and sequences that are foundational to various mathematical concepts.
Start with the Number: Begin by choosing the number for which you want to find multiples. This number is your base or starting point.
Multiply by Integers: The simplest way to find multiples is to multiply your chosen number by integers (whole numbers). Start with 1, then 2, 3, 4, and so on. For example, if you’re finding multiples of 3, you’ll multiply 3 by 1 to get 3, by 2 to get 6, by 3 to get 9, and continue this pattern.
List the Products: As you multiply, list down the products. These products are the multiples of your chosen number. Continuing with the example of 3, your list will start with 3, 6, 9, 12, 15, and so on.
Recognize Patterns: As you list the multiples, you might start to notice patterns. For instance, multiples of 2 always end in 0, 2, 4, 6, or 8, while multiples of 5 end in 0 or 5. Identifying these patterns can make finding multiples quicker and more intuitive.
Use Multiplication Tables: If you’re familiar with multiplication tables, you can use them as a shortcut to find multiples. The row or column corresponding to your number in the table will list its multiples.
Incorporate Technology: For larger numbers or for a more extensive list of multiples, consider using calculators or online tools. These can save time and ensure accuracy, especially when dealing with numbers outside the typical multiplication table range.
For this example, we’ve chosen the number 4.
We begin by multiplying 4 by integers (whole numbers) starting from 1. Here’s how it looks:
4 × 1 = 4
4 × 2 = 8
4 × 3 = 12
4 × 4 = 16
4 × 5 = 20
4 × 6 = 24
4 × 7 = 28
4 × 8 = 32
4 × 9 = 36
4 × 10 = 40
As we multiply, we list the products, which are the multiples of 4: 4, 8, 12, 16, 20, 24, 28, 32, 36, 40…
In this case, you might notice that all multiples of 4 are even numbers. This pattern can help you quickly identify multiples of 4, even without doing the multiplication explicitly.
 Creating a list of multiples in a table format is an effective way to visualize and understand the pattern of multiples for any given number. This approach is particularly useful in educational settings, aiding both teachers and students in exploring the concept of multiplication and its applications. Below, we provide a guide on how to create and interpret a multiples table, followed by an example for clarity.
Creating a list of multiples in a table format is an effective way to visualize and understand the pattern of multiples for any given number. This approach is particularly useful in educational settings, aiding both teachers and students in exploring the concept of multiplication and its applications. Below, we provide a guide on how to create and interpret a multiples table, followed by an example for clarity.
Number | List of First Ten Multiples |
|---|---|
1 | 1, 2, 3, 4, 5, 6, 7, 8, 9, 10 |
2 | 2, 4, 6, 8, 10, 12, 14, 16, 18, 20 |
3 | 3, 6, 9, 12, 15, 18, 21, 24, 27, 30 |
4 | 4, 8, 12, 16, 20, 24, 28, 32, 36, 40 |
5 | 5, 10, 15, 20, 25, 30, 35, 40, 45, 50 |
6 | 6, 12, 18, 24, 30, 36, 42, 48, 54, 60 |
7 | 7, 14, 21, 28, 35, 42, 49, 56, 63, 70 |
8 | 8, 16, 24, 32, 40, 48, 56, 64, 72, 80 |
9 | 9, 18, 27, 36, 45, 54, 63, 72, 81, 90 |
10 | 10, 20, 30, 40, 50, 60, 70, 80, 90, 100 |
 In the realm of mathematics, factors and multiples are fundamental concepts that often confuse learners due to their interconnected nature. However, understanding the distinction between these two is crucial for mastering arithmetic and algebra. This guide aims to elucidate the differences with clear definitions and illustrative examples.
In the realm of mathematics, factors and multiples are fundamental concepts that often confuse learners due to their interconnected nature. However, understanding the distinction between these two is crucial for mastering arithmetic and algebra. This guide aims to elucidate the differences with clear definitions and illustrative examples.
Aspect | Factors | Multiples |
|---|---|---|
Definition | Numbers that divide a given number exactly without remainder. | Products obtained when a given number is multiplied by integers. |
Range | Finite, limited to numbers less than or equal to the given number. | Infinite, as you can keep multiplying by larger integers. |
Example for 8 | Factors of 8 are 1, 2, 4, 8. | First few multiples of 8 are 8, 16, 24, 32, 40. |
Multiplicity | Every number has a unique set of factors. | Every number has an infinite set of multiples. |
In Mathematics | Used in simplifying fractions, finding GCD, and solving divisibility problems. | Important for finding LCM, working with sequences, and pattern recognition. |
Pattern | Factors decrease in number as the given number increases. | Multiples increase as the given number increases. |
Visibility | Factors are always less than or equal to the given number. | Multiples are always equal to or greater than the given number. |
In the mathematical landscape, the concept of common multiples plays a pivotal role in various arithmetic and algebraic operations. Understanding common multiples is essential for solving problems related to least common multiples (LCM), simplifying fractions, and finding solutions to equations involving multiple terms. This guide aims to shed light on what common multiples are, how to find them, and their practical applications, enhancing your mathematical toolkit.
Common multiples of two or more numbers are multiples that the numbers share. These are numbers that can be divided evenly by all the original numbers without any remainder. For instance, if you’re considering the numbers 4 and 6, some of their common multiples would be 12, 24, 36, and so on. Each of these common multiples can be divided evenly by both 4 and 6.
List Multiples: Start by listing the first few multiples of the numbers you’re considering. For example, if you’re working with 3 and 4, list their multiples:
Multiples of 3: 3, 6, 9, 12, 15, 18, 21, 24, 27, 30…
Multiples of 4: 4, 8, 12, 16, 20, 24, 28, 32, 36, 40…
Identify Common Multiples: Look through the lists to find multiples that appear in all lists. In the example above, 12 and 24 are common multiples of 3 and 4.
Least Common Multiple (LCM): The smallest of the common multiples is known as the Least Common Multiple (LCM). For 3 and 4, the LCM is 12, as it is the smallest number that both 3 and 4 can divide into without a remainder.
The multiples of 2 represent the essence of even numbers. Each product in this series is a result of multiplying 2 by integers ranging from 1 to 10. This sequence is fundamental in mathematics, illustrating the basic concept of even numbers, which are divisible by 2 without any remainder. Understanding these multiples is crucial for grasping the properties of even numbers, which play a significant role in various arithmetic operations and number theory.
2 × 1 = 2
2 × 2 = 4
2 × 3 = 6
2 × 4 = 8
2 × 5 = 10
2 × 6 = 12
2 × 7 = 14
2 × 8 = 16
2 × 9 = 18
2 × 10 = 20
The table showcasing the multiples of 3 highlights a pattern where each number is divisible by 3. This series is particularly important for understanding the concept of divisibility and for identifying numbers that are multiples of 3. These multiples are integral to various mathematical concepts, including factors, multiples, and patterns within the number system. Recognizing the sequence of multiples of 3 aids in developing arithmetic skills and enhances problem-solving capabilities.
3 × 1 = 3
3 × 2 = 6
3 × 3 = 9
3 × 4 = 12
3 × 5 = 15
3 × 6 = 18
3 × 7 = 21
3 × 8 = 24
3 × 9 = 27
3 × 10 = 30
The multiples of 4 table demonstrates how numbers increase when multiplied by 4. Each step in this series doubles the even number sequence, further emphasizing the structure within the number system. This table is useful for understanding the multiplication process and for recognizing patterns associated with the number 4. It serves as a valuable tool for students learning basic multiplication and for anyone working with numbers divisible by 4.
4 × 1 = 4
4 × 2 = 8
4 × 3 = 12
4 × 4 = 16
4 × 5 = 20
4 × 6 = 24
4 × 7 = 28
4 × 8 = 32
4 × 9 = 36
4 × 10 = 40
he multiples of 5 are easily recognizable by their ending digits, either 0 or 5. This pattern is not only simple to remember but also crucial for various arithmetic calculations, especially in mental math. The table of multiples of 5 is particularly useful in real-world applications, such as counting by fives, dealing with currency, and measuring time
5 × 1 = 5
5 × 2 = 10
5 × 3 = 15
5 × 4 = 20
5 × 5 = 25
5 × 6 = 30
5 × 7 = 35
5 × 8 = 40
5 × 9 = 45
5 × 10 = 50
The table of multiples of 6 combines the properties of even numbers and the divisibility by 3. This series is essential for understanding more complex patterns in the number system and for solving problems related to least common multiples and divisibility.
6 × 1 = 6
6 × 2 = 12
6 × 3 = 18
6 × 4 = 24
6 × 5 = 30
6 × 6 = 36
6 × 7 = 42
6 × 8 = 48
6 × 9 = 54
6 × 10 = 60
In conclusion, multiples form an essential part of mathematics, offering a foundation for understanding arithmetic patterns and solving complex problems. By mastering the concept of multiples and recognizing their patterns, learners can enhance their mathematical skills, facilitating easier computation and a deeper comprehension of numerical relationships, crucial for academic success and daily applications.
Text prompt
Add Tone
Properties of Multiples
How to Find Multiples of a Number
Which of the following is a multiple of 5?
22
27
30
33
Which number is a multiple of 8?
45
48
50
52
Find the smallest multiple of 7 greater than 50.
49
51
56
63
Which of the following is not a multiple of 9?
27
36
45
50
What is the least common multiple (LCM) of 4 and 6?
10
12
14
16
Find the greatest multiple of 13 less than 150.
130
143
156
169
Which of the following numbers is a multiple of 15?
65
70
75
80
Find the smallest multiple of 8 greater than 70.
72
74
76
78
Which number is a multiple of 4?
23
24
25
26
Which of the following numbers is not a multiple of 2?
14
16
18
19
Before you leave, take our quick quiz to enhance your learning!

