Perfect Square Trinomial

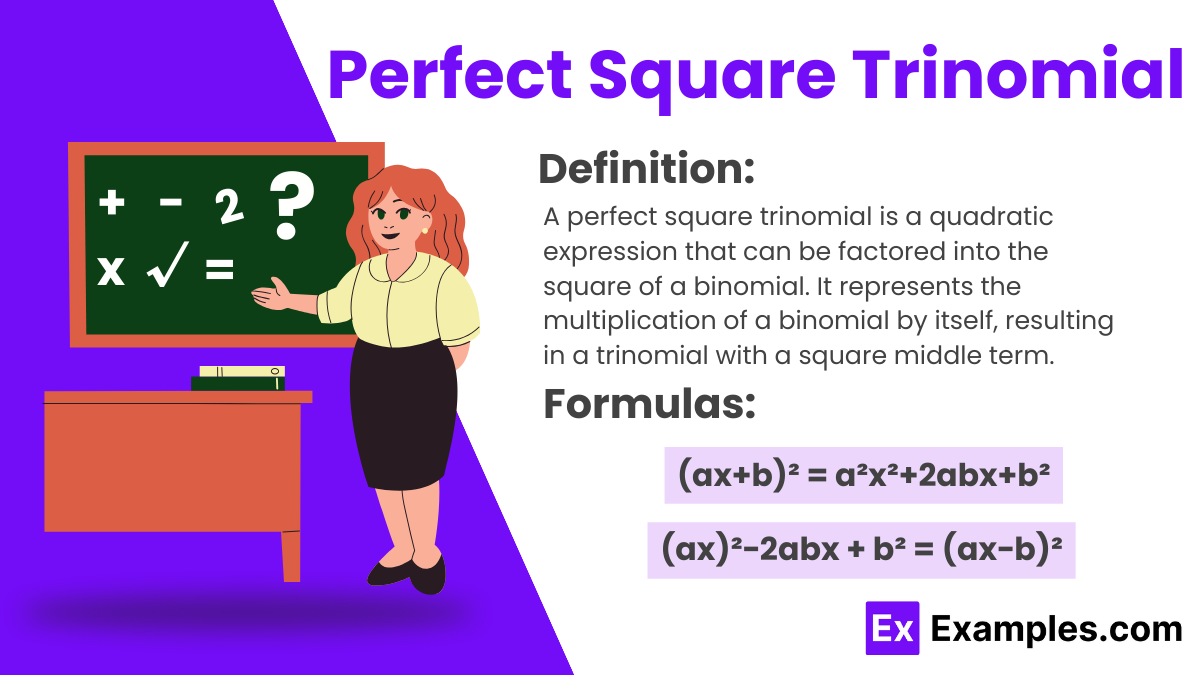
Exploring Perfect Square Trinomials unveils essential principles of algebra, encompassing rational and irrational numbers, squares, and square roots. These trinomials represent quadratic expressions that can be factored into identical binomial factors, showcasing the symmetry and structure inherent in quadratic equations. Understanding Perfect Square Trinomials is crucial in algebraic manipulation, number theory, and statistical analysis, providing insights into least square methods and polynomial functions. Mastery of these trinomials enhances proficiency in mathematical reasoning and problem-solving across various domains.

Whats is Perfect Square Trinomial – Definition

A Perfect Square Trinomial is a quadratic expression that can be factored into the square of a binomial, where each term is a perfect square. It follows the form (𝑎𝑥+𝑏)², where 𝑎 and 𝑏 are integers, representing a quadratic expression with a square leading coefficient and twice the product of the square root of the first and last terms. This special form allows for easy identification and manipulation, often employed in algebraic simplification, solving quadratic equations, and exploring polynomial functions.

Perfect Square Trinomial Formula

The formulas for a Perfect Square Trinomial is:

(ax+b)² = a²x²+2abx+b²

(ax)²−2abx + b² = (ax−b)²

Where 𝑎 and 𝑏 are integers, s of the quadratic expression, and 𝑥 is the variable. This formula represents the expansion of a squared binomial, showcasing the symmetrical structure of the trinomial and its relationship to the original expression. It is a fundamental tool in algebraic manipulation, factorization, and solving quadratic equations.

• The first term (𝑎𝑥)² represents the square of the first term of the binomial.
• The last term 𝑏² represents the square of the last term of the binomial.
• The middle term −2𝑎𝑏𝑥 represents twice the product of the square roots of the first and last terms of the binomial.

Perfect Square Trinomial Pattern

• Symmetrical Structure: Perfect Square Trinomials exhibit a symmetrical structure when expanded, with the first and last terms being perfect squares.
• Binomial Squaring: They result from squaring a binomial expression, producing a quadratic polynomial with three terms.
• Specific Form: Perfect Square Trinomials follow the form (ax+b)² = a²x²+2abx+b², where 𝑎 and 𝑏 are integers.
• Easy Identification: They can be easily identified by checking whether the first and last terms are perfect squares, and the middle term is twice the product of the square root of the first and last terms.
• Factorization: Perfect Square Trinomials can be factored into the square of a binomial, facilitating simplification and solution of quadratic equations.
• Applications: They are commonly encountered in algebraic expressions, quadratic equations, and polynomial functions, playing a crucial role in various mathematical problems and applications.

How to Factor a Perfect Square Trinomial

• Identify the Trinomial: Determine if the quadratic expression is a perfect square trinomial, meaning it can be factored into the square of a binomial.
• Check the Form: Verify that the trinomial follows the form (ax+b)², where 𝑎a and 𝑏b are integers.
• Identify the Square Terms: Recognize the first and last terms of the trinomial, which must be perfect squares.
• Determine the Middle Term: Calculate the middle term by taking twice the product of the square roots of the first and last terms.
• Write the Binomial: Write the binomial expression as (ax+b), where 𝑎a is the square root of the first term and 𝑏b is the square root of the last term.
• Factor: Express the Perfect Square Trinomial as the square of the binomial, i.e., (ax+b)².
• Verify: Multiply the binomial expression to ensure it yields the original trinomial.

Perfect Square Trinomial Examples

Example 1

• Trinomial: 𝑥²+6𝑥+9
• Explanation: This trinomial is a perfect square because the first term (𝑥²) and the last term (9) are perfect squares, and the middle term (6𝑥6x) is twice the product of the square root of the first and last terms (2×3×𝑥2×3×).
• Factored Form: (𝑥+3)²
• Trinomial: 4𝑥²−12𝑥+9

Example 2

• Let’s examine the expression 9𝑦²+12𝑦+4. In this case, 𝑎=3 and 𝑏=2. Using the formula, we get (3𝑦)²−2(3)(2)𝑦+2²=(3𝑦−2)², which simplifies to (3𝑦−2)², confirming the Perfect Square Trinomial.

Example 3

• Explanation: This trinomial is also a perfect square because the first term (4𝑥²) and the last term (9) are perfect squares, and the middle term (−12𝑥) is twice the product of the square root of the first and last terms (2×3×𝑥).
• Factored Form: (2𝑥−3)²

Example 4

• Trinomial: 9𝑦²+12𝑦+Explanation: This trinomial is a perfect square because the first term (9𝑦²) and the last term (4) are perfect squares, and the middle term (12𝑦) is twice the product of the square root of the first and last terms (2×3×𝑦).
• Factored Form: (3𝑦+2)²

Example 5

• Consider the expression 4𝑥²−12𝑥+9. Here, 𝑎=2 and 𝑏=3. Applying the formula, we have (2𝑥)²−2(2)(3)𝑥+32 = (2𝑥−3)², which simplifies to (2𝑥−3)², verifying the Perfect Square Trinomial.

FAQs

How do you recognize a Perfect Square Trinomial?

• You can identify a Perfect Square Trinomial by checking if it follows the form (ax+b)², where 𝑎 and 𝑏 are integers, and the middle term is twice the product of the square roots of the first and last terms.

What are some applications of Perfect Square Trinomials?

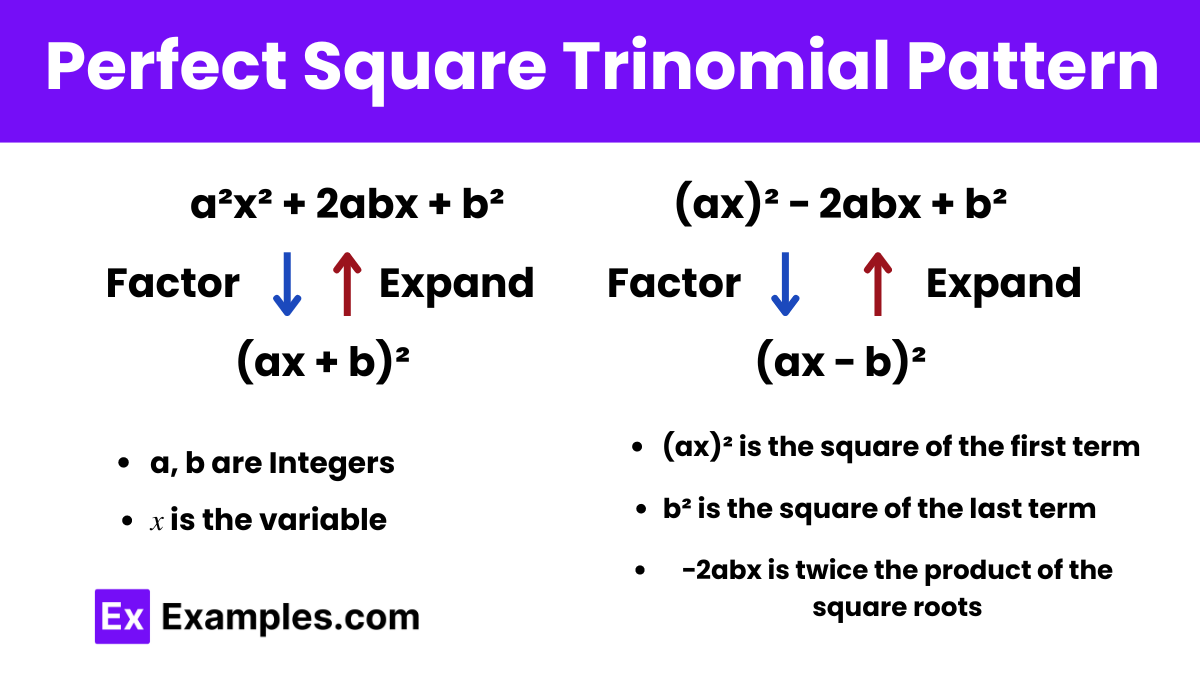
Perfect Square Trinomials are used in various mathematical applications, including geometry, physics, and engineering. They are employed in solving problems involving areas, volumes, distances, and optimization.

Can Perfect Square Trinomials be called Quadratic Equations?

erfect Square Trinomials are a type of quadratic expression, not necessarily a quadratic equation, as they represent a specific form of quadratic polynomial that can be factored into the square of a binomial. They are a tool for solving quadratic equations and understanding quadratic functions but are distinct from equations, which involve expressions set equal to one another.