What is the square of 109?
11,881
12,321
11,321
12,099

Hence, the square number of 109 is 11881. Mastering the process of squaring numbers is crucial in mathematics, especially in fields like algebra and geometry. It serves as a foundation for tackling more intricate problems and understanding advanced mathematical theories.
√109 = 10.4403065089
or
√109 = 10.440 up to three decimals
The square root of 109 is an irrational number, similar to the square roots of other non-perfect squares such as 24, because it cannot be exactly expressed as a fraction of two integers. Its approximate value is 10.4403065089. This value has importance in various mathematical fields, including geometry, where it can be used to determine the length of the side of a square that has an area of 109 square units. Furthermore, the square root of 109 is utilized in algebraic equations, engineering calculations, and physics formulas. It plays an essential role in understanding spatial relationships and achieving numerical approximations.
irrational number cannot be expressed as a simple fraction, where both the numerator and denominator are integers. The square root of 109, like that of most non-perfect squares, does not result in a whole number or a simple fraction, indicating its irrational nature. This characteristic is crucial in fields such as algebra and geometry, impacting calculations and theories involving square roots.
Rational numbers are those that can be expressed as a fraction with both the numerator and denominator being integers. This category includes a variety of numbers, such as simple fractions like 1/2, negative fractions like −3/4
Examples:4/3,3/5
Irrational numbers, in contrast, cannot be written as a simple fraction. This means there are no two integers that can form a fraction to express these numbers exactly. Irrational numbers have non-terminating, non-repeating decimal expansions. Many of them are found by taking the square root of numbers that aren’t perfect squares.
Examples:√3,√5
The simplest and most accurate method for most people is to use a calculator. By entering 109 and pressing the square root function, you can quickly obtain the value of √109.
For an estimation without a calculator, you can find two perfect squares between which 109 falls (100 and 121, which are squares of 10 and 11, respectively). Since 109 is closer to 100, you can estimate √109 to be slightly under 10.5.
This method uses calculus and is very effective for finding more precise values of square roots. You start with a guess (x0, say 10) and use the formula:
xₙ₊₁ = 1/2(xₙ+109/xₙ)
Repeatedly apply this formula until the value stabilizes. This method converges very quickly to the actual square root.
Similar to Newton’s method, this ancient technique, also known as the “Hero’s method,” involves making a guess and refining it. It uses the same iterative formula as Newton’s method and is particularly useful when a high degree of accuracy is not required.
This method is akin to traditional long division but adapted for square roots. It involves dividing the number into pairs of digits starting from the decimal point and subtracting squares of increasing digits until you get a result. This method can be cumbersome without practice but provides a step-by-step breakdown of the square root.
Programming languages such as Python, Java, or C++ can be used to calculate square roots to many decimal places using built-in functions or algorithms similar to the Newton’s method.
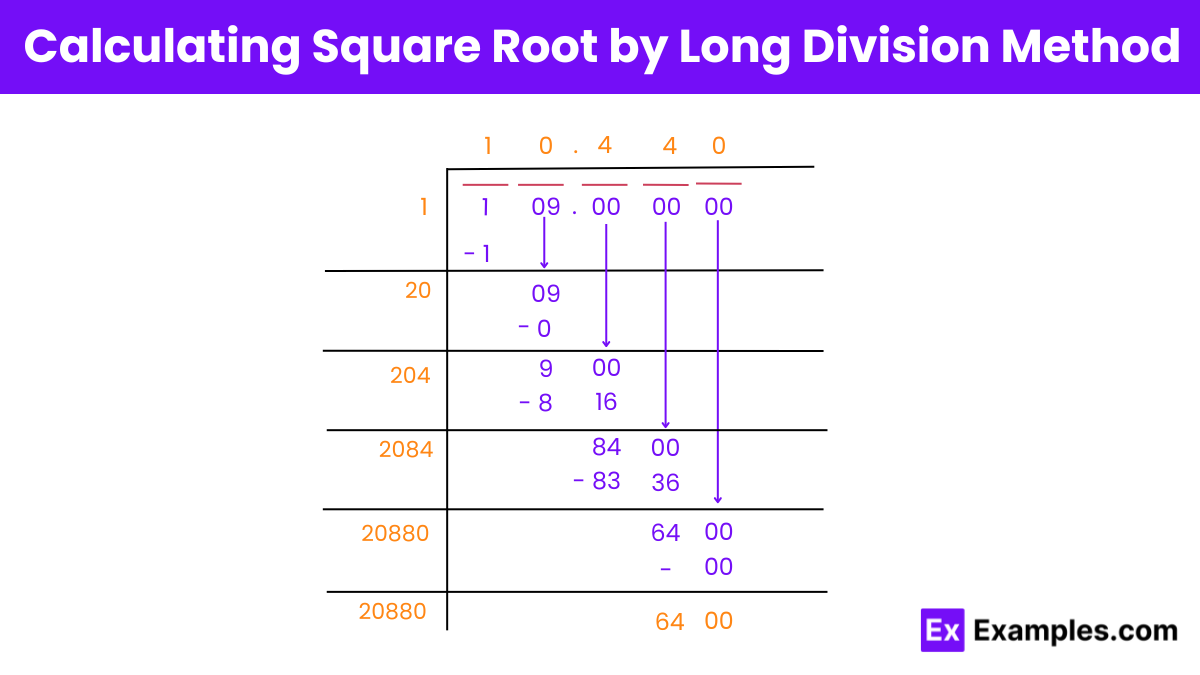
Start by pairing the digits of 109 from the right. This looks like: 1 | 09. Use a horizontal bar to show the pairing.
Find a number which squared gives a result ≤ 1. Since 1 × 1 = 1, the first quotient digit is 1.
Bring down the next pair of digits (00) and double the current quotient (1) which gives 2. This 2 is the starting digit of our new divisor.
Put a 0 in the one’s place of the new divisor to make 20, because 20 × 0 = 0. Subtract this from 9, bring down the next pair of zeros, and the new remainder is 900.
Now, multiply the current quotient (10) by 2 to get 20, which is the starting digit of the next divisor.
Place a 4 at the one’s place to make the new divisor 204, because 204 × 4 = 816. Subtract this from 900 to get 84, and bring down the next pair of zeros.
The quotient 104, when doubled, gives 208. This is the new starting digit of the divisor.
Again, place a 4 in the one’s place to get 2084. Multiplying 2084 by 4 gives 8336. Subtracting this from 8400, the new remainder is 64. Bring down the next pair of zeros.
Double the latest quotient (1044) to get 2088, which is the starting digit of the next divisor.
Place a 0 at the one’s place of the new divisor (20880) because 20880 × 0 = 0. Subtract this from 6400 to get the final remainder of 6400.
A perfect square is a number that can be expressed as the square of an integer. For instance, numbers like 100 (10²) and 121 (11²) are perfect squares because they result from squaring whole numbers. However, 109 does not have an integer as its square root; the square root of 109 is approximately 10.44, which is not a whole number. Thus, 109 is not a perfect square.
Refers to the square root of 109, which is an irrational number approximately equal to 10.44. It represents the non-integer root of the number 109.
The number 109 is a prime number, meaning it is greater than 1, has no positive divisors other than 1 and itself, and is an odd number.

109² (109×109) = 11881
Hence, the square number of 109 is 11881. Mastering the process of squaring numbers is crucial in mathematics, especially in fields like algebra and geometry. It serves as a foundation for tackling more intricate problems and understanding advanced mathematical theories.
√109 = 10.4403065089
or
√109 = 10.440 up to three decimals
The square root of 109 is an irrational number, similar to the square roots of other non-perfect squares such as 24, because it cannot be exactly expressed as a fraction of two integers. Its approximate value is 10.4403065089. This value has importance in various mathematical fields, including geometry, where it can be used to determine the length of the side of a square that has an area of 109 square units. Furthermore, the square root of 109 is utilized in algebraic equations, engineering calculations, and physics formulas. It plays an essential role in understanding spatial relationships and achieving numerical approximations.
Approximate Decimal Form: 10.4403065089
Exponential Form: 109^0.5 or 109¹/²
Radical Form: √109
The square root of 109 is irrational.
irrational number cannot be expressed as a simple fraction, where both the numerator and denominator are integers. The square root of 109, like that of most non-perfect squares, does not result in a whole number or a simple fraction, indicating its irrational nature. This characteristic is crucial in fields such as algebra and geometry, impacting calculations and theories involving square roots.
Rational numbers are those that can be expressed as a fraction with both the numerator and denominator being integers. This category includes a variety of numbers, such as simple fractions like 1/2, negative fractions like −3/4
Examples:4/3,3/5
Irrational numbers, in contrast, cannot be written as a simple fraction. This means there are no two integers that can form a fraction to express these numbers exactly. Irrational numbers have non-terminating, non-repeating decimal expansions. Many of them are found by taking the square root of numbers that aren’t perfect squares.
Examples:√3,√5
The simplest and most accurate method for most people is to use a calculator. By entering 109 and pressing the square root function, you can quickly obtain the value of √109.
For an estimation without a calculator, you can find two perfect squares between which 109 falls (100 and 121, which are squares of 10 and 11, respectively). Since 109 is closer to 100, you can estimate √109 to be slightly under 10.5.
This method uses calculus and is very effective for finding more precise values of square roots. You start with a guess (x0, say 10) and use the formula:
xₙ₊₁ = 1/2(xₙ+109/xₙ)
Repeatedly apply this formula until the value stabilizes. This method converges very quickly to the actual square root.
Similar to Newton’s method, this ancient technique, also known as the “Hero’s method,” involves making a guess and refining it. It uses the same iterative formula as Newton’s method and is particularly useful when a high degree of accuracy is not required.
This method is akin to traditional long division but adapted for square roots. It involves dividing the number into pairs of digits starting from the decimal point and subtracting squares of increasing digits until you get a result. This method can be cumbersome without practice but provides a step-by-step breakdown of the square root.
Programming languages such as Python, Java, or C++ can be used to calculate square roots to many decimal places using built-in functions or algorithms similar to the Newton’s method.
Start by pairing the digits of 109 from the right. This looks like: 1 | 09. Use a horizontal bar to show the pairing.
Find a number which squared gives a result ≤ 1. Since 1 × 1 = 1, the first quotient digit is 1.
Bring down the next pair of digits (00) and double the current quotient (1) which gives 2. This 2 is the starting digit of our new divisor.
Put a 0 in the one’s place of the new divisor to make 20, because 20 × 0 = 0. Subtract this from 9, bring down the next pair of zeros, and the new remainder is 900.
Now, multiply the current quotient (10) by 2 to get 20, which is the starting digit of the next divisor.
Place a 4 at the one’s place to make the new divisor 204, because 204 × 4 = 816. Subtract this from 900 to get 84, and bring down the next pair of zeros.
The quotient 104, when doubled, gives 208. This is the new starting digit of the divisor.
Again, place a 4 in the one’s place to get 2084. Multiplying 2084 by 4 gives 8336. Subtracting this from 8400, the new remainder is 64. Bring down the next pair of zeros.
Double the latest quotient (1044) to get 2088, which is the starting digit of the next divisor.
Place a 0 at the one’s place of the new divisor (20880) because 20880 × 0 = 0. Subtract this from 6400 to get the final remainder of 6400.
No, 109 is not a perfect square.
A perfect square is a number that can be expressed as the square of an integer. For instance, numbers like 100 (10²) and 121 (11²) are perfect squares because they result from squaring whole numbers. However, 109 does not have an integer as its square root; the square root of 109 is approximately 10.44, which is not a whole number. Thus, 109 is not a perfect square.
Refers to the square root of 109, which is an irrational number approximately equal to 10.44. It represents the non-integer root of the number 109.
The number 109 is a prime number, meaning it is greater than 1, has no positive divisors other than 1 and itself, and is an odd number.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
What is the square of 109?
11,881
12,321
11,321
12,099
Find the square root of 109.
10.44
10.54
10.49
10.33
What is the value of 109 squared?
11,881
12,109
11,541
12,321
If x² = 109, what is the value of x?
±10.44
±10.54
±10.49
±10.33
What is 109 raised to the power of 2?
11,881
12,109
11,541
12,321
What is the square root of 121 minus the square root of 109?
1.50
2.50
3.00
4.00
What is the square of the square root of 109?
109
121
100
144
Find the number whose square root is approximately 10.44.
100
109
123
144
Calculate the square root of 121 plus the square root of 109.
21.44
20.54
22.00
19.44
What is the difference between the square of 110 and the square of 109?
219
220
121
1
Before you leave, take our quick quiz to enhance your learning!

