What is the square of 20?
200
400
800
1000
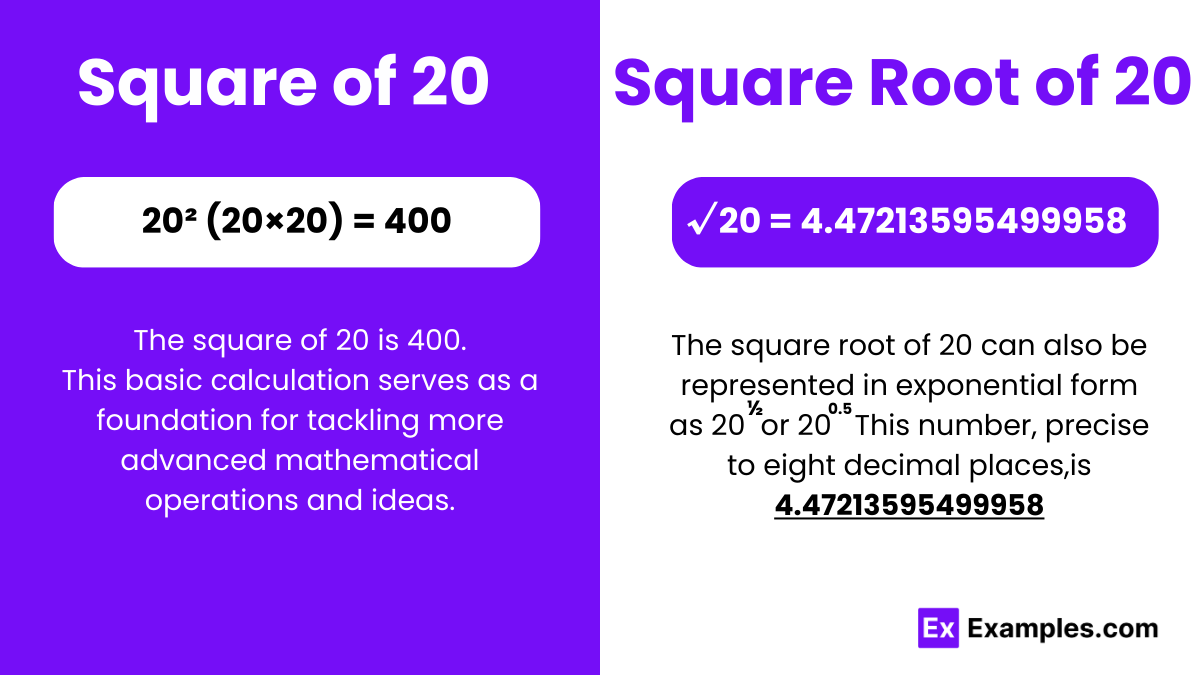
The “square of 20” refers to the result obtained when the number 20 is multiplied by itself.
In mathematical terms, it is denoted as 20² or simply 20 squared. To calculate the square of 20, you simply multiply 20 by 20. This can be done using basic arithmetic operations:
Mathematically, 20² (20×20) = 400
The concept of the square of 20 is fundamental in mathematics and arithmetic. It represents the area of a square with side length 20 units. Understanding the square of 20 is crucial in various mathematical applications, such as geometry, algebra, and physics. By knowing how to calculate the square of 20, one can solve problems involving area, volume, and other geometric properties with ease.
√20 = 4.47213595499958
or
√20=4.472 up to three places of decimal
The square root of 20, denoted as √20, is a value that, when multiplied by itself, equals 20. In simpler terms, it’s the number that, when squared, gives the result 20. To calculate the square root of 20, we seek a number that, when multiplied by itself, equals 20. While the square root of 20 is not a whole number, it is an irrational number. Mathematically, the square root of 20 is approximately 4.47213595499958. Calculating the square root of 20 involves various methods such as long division, approximation techniques like Newton’s method, or using calculators with square root functions. Understanding the square root of 20 is crucial in mathematics, especially in geometry, algebra, and calculus, where it is used to solve equations and find unknown sides or dimensions in geometric shapes.
Square Root of 20: 4.47213595499958
Exponential Form: 20^½ or 20^0.5
Radical Form: √20
The square root of 20 is an irrational number.
To understand why the square root of 20 is irrational, let’s delve into the definitions of rational and irrational numbers.
Firstly, let’s define these terms:
Square Root of 20 as Irrational:
When we calculate the square root of 20, we find that it cannot be expressed as a fraction of two integers.
Its decimal expansion, approximately 4.47213595499958, goes on infinitely without repeating a pattern.
To understand why the square root of 20 is irrational, let’s simplify it:
√20 = √(16 + 4) = √16 ×√4 = 4 × 2 = 8
Now, we know that 8 is a rational number. However, √4 is famously rational. It cannot be represented as a fraction of two integers, and its decimal form goes on forever without repeating. Therefore, 8 is also irrational because the product of a rational number (8) and an irrational number (√4) is always irrational.
In summary, the square root of 20 is irrational because it simplifies to a rational number (8), and when combined with the irrational √4, the result is always irrational.
Finding the value of the square root of 20 involves various methods, each with its own approach to determine an approximate value of √20. Here are some common methods explained:
Long Division Method: In the long division method, we iteratively refine an initial guess through a series of divisions until reaching a satisfactory level of precision. We identify a perfect square close to 20 and perform long division to obtain the square root.
Approximation Techniques: Techniques such as Newton’s method or the Babylonian method can be employed to approximate the square root of 20. These methods involve iteratively refining an initial guess to converge towards the actual value of √20.
Using Calculators or Software: Modern calculators and mathematical software programs come equipped with built-in functions to directly compute the square root of a number. This offers a quick and accurate way to find the value of √20 without manual calculation.
Factorization: Another method involves the prime factorization of 20 and grouping the factors into pairs. By extracting one factor from each pair, we can obtain an approximation of the square root of 20.

Finding the square root of 20 using the long division method involves a similar procedure:
Step 1: Preparation
Write 20 as 20.00 00 00, grouping digits in pairs from the decimal point. For 20, it looks like “20”.
Step 2: Find the Largest Square
Identify the largest square smaller than or equal to 20, which is 16 (4²). Place 4 above the line as the first digit of the root.
Step 3: Subtract and Bring Down
Subtract 16 from 20 to get 4, then bring down the next pair of zeros to make it 400.
Step 4: Double and Find the Next Digit
Double the current result (4) to get 8. Now, find a digit (X) such that 48 multiplied by X is less than or equal to 400. Here, X is 5, because 485×5=240.
Step 5: Repeat with Precision
Subtract 240 from 400 to get 160, bring down the next zeros to get 1600, then double the quotient (45) to get 90. Choose a digit (Y) so that 459 multiplied by Y is just under 1600.
Step 6: Finish at Desired Accuracy
Continue the process until reaching the desired level of accuracy. For the square root of 20, this method gives us about 4.472 as we extend the division.
A perfect square root is a number that can be expressed as the product of an integer multiplied by itself. For instance, 4 (2 × 2) and 9 (3 × 3) are perfect square roots. To determine whether 20 is a perfect square root, we examine if it can be expressed as the square of an integer.
However, when we try to find an integer that, when multiplied by itself, equals 20, we realize that there are no such integers. In other words, there is no whole number x such that x × x = 20.
Since 20 cannot be expressed as the product of two identical integers, it doesn’t have an integer square root. While 20 does have a square root (√20), it’s an irrational number and not the result of multiplying any whole number by itself. Therefore, we conclude that 20 is not a perfect square root.
Is √20 a Real Number?
Yes, the square root of 20, denoted as √20, is a real number. A real number is any number that can be found on the number line, including both rational and irrational numbers. Since √20 exists on the number line and is not an imaginary number (which involve the square root of negative numbers), it is classified as a real number.
Is 20 an Integer? Yes or No?
No, 20 is not an integer. An integer is a whole number that can be positive, negative, or zero. Since 20 is not a whole number and includes a fractional part, it does not fall under the category of integers.
What Square Root is Between 20?
The square root of 20 lies between the integers 4 and 5. While the exact value of √20 is approximately 4.47213595499958, it’s important to note that it falls between the perfect squares of 4 (which is 16) and 5 (which is 25). Therefore, we can say that the square root of 20 is greater than 4 but less than 5.

The “square of 20” refers to the result obtained when the number 20 is multiplied by itself.
In mathematical terms, it is denoted as 20² or simply 20 squared. To calculate the square of 20, you simply multiply 20 by 20. This can be done using basic arithmetic operations:
Mathematically, 20² (20×20) = 400
The concept of the square of 20 is fundamental in mathematics and arithmetic. It represents the area of a square with side length 20 units. Understanding the square of 20 is crucial in various mathematical applications, such as geometry, algebra, and physics. By knowing how to calculate the square of 20, one can solve problems involving area, volume, and other geometric properties with ease.
√20 = 4.47213595499958
or
√20=4.472 up to three places of decimal
The square root of 20, denoted as √20, is a value that, when multiplied by itself, equals 20. In simpler terms, it’s the number that, when squared, gives the result 20. To calculate the square root of 20, we seek a number that, when multiplied by itself, equals 20. While the square root of 20 is not a whole number, it is an irrational number. Mathematically, the square root of 20 is approximately 4.47213595499958. Calculating the square root of 20 involves various methods such as long division, approximation techniques like Newton’s method, or using calculators with square root functions. Understanding the square root of 20 is crucial in mathematics, especially in geometry, algebra, and calculus, where it is used to solve equations and find unknown sides or dimensions in geometric shapes.
Square Root of 20: 4.47213595499958
Exponential Form: 20^½ or 20^0.5
Radical Form: √20
The square root of 20 is an irrational number.
To understand why the square root of 20 is irrational, let’s delve into the definitions of rational and irrational numbers.
Firstly, let’s define these terms:
Rational Number: A rational number can be expressed as the quotient of two integers, where the denominator is not zero. Rational numbers have either terminating or repeating decimal representations.
Irrational Number: An irrational number cannot be expressed as a simple fraction of two integers. Their decimal representations are non-repeating and non-terminating.
Square Root of 20 as Irrational:
When we calculate the square root of 20, we find that it cannot be expressed as a fraction of two integers.
Its decimal expansion, approximately 4.47213595499958, goes on infinitely without repeating a pattern.
To understand why the square root of 20 is irrational, let’s simplify it:
√20 = √(16 + 4) = √16 ×√4 = 4 × 2 = 8
Now, we know that 8 is a rational number. However, √4 is famously rational. It cannot be represented as a fraction of two integers, and its decimal form goes on forever without repeating. Therefore, 8 is also irrational because the product of a rational number (8) and an irrational number (√4) is always irrational.
In summary, the square root of 20 is irrational because it simplifies to a rational number (8), and when combined with the irrational √4, the result is always irrational.
Finding the value of the square root of 20 involves various methods, each with its own approach to determine an approximate value of √20. Here are some common methods explained:
Long Division Method: In the long division method, we iteratively refine an initial guess through a series of divisions until reaching a satisfactory level of precision. We identify a perfect square close to 20 and perform long division to obtain the square root.
Approximation Techniques: Techniques such as Newton’s method or the Babylonian method can be employed to approximate the square root of 20. These methods involve iteratively refining an initial guess to converge towards the actual value of √20.
Using Calculators or Software: Modern calculators and mathematical software programs come equipped with built-in functions to directly compute the square root of a number. This offers a quick and accurate way to find the value of √20 without manual calculation.
Factorization: Another method involves the prime factorization of 20 and grouping the factors into pairs. By extracting one factor from each pair, we can obtain an approximation of the square root of 20.
Finding the square root of 20 using the long division method involves a similar procedure:
Step 1: Preparation
Write 20 as 20.00 00 00, grouping digits in pairs from the decimal point. For 20, it looks like “20”.
Step 2: Find the Largest Square
Identify the largest square smaller than or equal to 20, which is 16 (4²). Place 4 above the line as the first digit of the root.
Step 3: Subtract and Bring Down
Subtract 16 from 20 to get 4, then bring down the next pair of zeros to make it 400.
Step 4: Double and Find the Next Digit
Double the current result (4) to get 8. Now, find a digit (X) such that 48 multiplied by X is less than or equal to 400. Here, X is 5, because 485×5=240.
Step 5: Repeat with Precision
Subtract 240 from 400 to get 160, bring down the next zeros to get 1600, then double the quotient (45) to get 90. Choose a digit (Y) so that 459 multiplied by Y is just under 1600.
Step 6: Finish at Desired Accuracy
Continue the process until reaching the desired level of accuracy. For the square root of 20, this method gives us about 4.472 as we extend the division.
A perfect square root is a number that can be expressed as the product of an integer multiplied by itself. For instance, 4 (2 × 2) and 9 (3 × 3) are perfect square roots. To determine whether 20 is a perfect square root, we examine if it can be expressed as the square of an integer.
However, when we try to find an integer that, when multiplied by itself, equals 20, we realize that there are no such integers. In other words, there is no whole number x such that x × x = 20.
Therefore, 20 is not a perfect square root
Since 20 cannot be expressed as the product of two identical integers, it doesn’t have an integer square root. While 20 does have a square root (√20), it’s an irrational number and not the result of multiplying any whole number by itself. Therefore, we conclude that 20 is not a perfect square root.
Is √20 a Real Number?
Yes, the square root of 20, denoted as √20, is a real number. A real number is any number that can be found on the number line, including both rational and irrational numbers. Since √20 exists on the number line and is not an imaginary number (which involve the square root of negative numbers), it is classified as a real number.
Is 20 an Integer? Yes or No?
No, 20 is not an integer. An integer is a whole number that can be positive, negative, or zero. Since 20 is not a whole number and includes a fractional part, it does not fall under the category of integers.
What Square Root is Between 20?
The square root of 20 lies between the integers 4 and 5. While the exact value of √20 is approximately 4.47213595499958, it’s important to note that it falls between the perfect squares of 4 (which is 16) and 5 (which is 25). Therefore, we can say that the square root of 20 is greater than 4 but less than 5.
Text prompt
Add Tone
10 Examples of Public speaking
20 Examples of Gas lighting
What is the square of 20?
200
400
800
1000
Which of the following numbers is the square root of 400?
10
15
20
25
Find the value of 20².
200
300
400
500
If √x = 20 , what is the value of x?
200
300
400
500
What is the approximate value of √20?
4.47
5.47
6.47
7.47
Which of the following is closest to the value of 20²?
380
390
400
410
If y = √400, what is the value of y?
19
20
21
22
Which number, when squared, equals 400?
15
17
19
20
What is the value of 20 × 20?
300
350
400
450
If n = √20, what is the value of n?
380
390
400
410
Before you leave, take our quick quiz to enhance your learning!

